AP Physics Workbook 5.I Momentum Representations

Mr.S ClassRoom
14 Nov 202009:38
EducationalLearning
32 Likes 10 Comments

TLDRThis transcript from an AP Physics workbook tutorial focuses on the concept of momentum in Unit 5, Section 5.1, where a scenario of a ball thrown upwards is used to illustrate the principles of motion, including velocity, acceleration, and the forces at play. The discussion covers the ball's journey from its initial positive velocity to reaching zero at the peak height, then becoming negative as it descends. The tutorial also delves into the potential and kinetic energy of the system, emphasizing the conservation of mechanical energy in a closed system without air resistance. The concepts are further clarified through the analysis of graphs representing velocity, position, momentum, net external force, and energy over time, highlighting the mathematical relationships that govern these physical quantities.

Takeaways
  • 🚀 The scenario describes a ball thrown upwards with an initial speed, reaching a maximum height with zero velocity before falling back down, neglecting air resistance.
  • 📉 The acceleration of the ball is consistently negative (-9.8 m/s²), directed downwards due to gravity.
  • 📈 The velocity-time graph starts with a positive velocity, goes to zero at the peak, and then becomes negative as the ball descends.
  • 📊 The position-time graph is a curve that starts flat, hits a zero slope at the peak, and then curves downwards as the ball falls.
  • 🌐 The potential energy of the ball-earth system is given by mgh and changes over time as the height changes, curving like the position graph but inverted.
  • 🔄 The momentum of the ball, represented as mv, matches the shape of the velocity-time graph since mass is constant.
  • ⚖️ The net external force on the ball is equal to the gravitational force (mass times the gravitational acceleration), directed downwards.
  • 🔢 The area under the acceleration vs. time curve equals the change in velocity (delta v), while the area under the velocity vs. time curve equals the change in position.
  • 🌟 The momentum vs. time graph has the same shape as the velocity vs. time graph, reflecting the direct relationship between momentum and velocity.
  • 💥 The net force graph vs. time is similar to the acceleration graph since net force equals mass times acceleration.
  • 🔄 The total mechanical energy of the ball-earth system remains constant due to the conservation of energy, as there are no external forces and the system is closed.
Q & A

  • What is the initial condition of the ball's velocity when thrown upwards?

    -The initial condition of the ball's velocity is positive, with an initial speed v-not, indicating that the ball is moving upwards at the start.

  • What is the acceleration experienced by the ball during its flight?

    -The acceleration experienced by the ball is constant at -9.8 m/s^2, directed downwards, due to gravitational force.

  • How does the velocity of the ball change over time during its flight?

    -The velocity of the ball starts positive, decreases to zero at the maximum height, and then becomes negative as it descends back to the ground.

  • What is the shape of the position versus time graph for the ball?

    -The position versus time graph for the ball starts at zero, increases to a maximum height, and then decreases back to zero, curving downward at every point since the slope represents the decreasing velocity.

  • How does the potential energy of the ball change over time?

    -The potential energy starts at zero, increases linearly with height as the ball rises, reaches a maximum at the peak height, and then decreases linearly as the ball descends, converting back into kinetic energy.

  • What is the momentum of the ball at different points during its flight?

    -The momentum of the ball is mv, where m is the mass and v is the velocity. It starts positive, goes to zero at the peak, and then becomes negative as the ball descends.

  • What is the net external force acting on the ball?

    -The net external force on the ball is equal to its mass times the gravitational acceleration (ma), which is -9.8 N downwards.

  • How does the conservation of energy principle apply to the ball's motion?

    -Since air resistance is neglected, the total mechanical energy of the ball-Earth system remains constant. The sum of kinetic and potential energy remains the same throughout the motion due to the closed system.

  • What is the kinetic energy of the ball at different points during its flight?

    -The kinetic energy, given by the formula (1/2)mv^2, starts high when the ball is thrown upwards, becomes zero at the peak, and then increases as the ball descends, reflecting the squaring of the velocity values.

  • How are the graphs of momentum versus time and velocity versus time related?

    -The graph of momentum versus time has the same shape as the velocity versus time graph because momentum is the product of mass and velocity (mv).

  • What can be inferred about the total mechanical energy graph over time?

    -The total mechanical energy graph remains constant over time because the system is closed, and there is no loss of energy due to the absence of external forces or work done.

Outlines
00:00
📚 Introduction to Momentum and Ball's Trajectory

This paragraph introduces the concept of momentum in the context of a physics workbook tutorial. It describes a scenario where a ball is thrown upwards with an initial speed and its motion is analyzed through various physical properties such as velocity, acceleration, and potential energy. The paragraph explains that air resistance is neglected, and the constant acceleration due to gravity is -9.8 m/s². The discussion includes the graphical representation of the ball's position, velocity, and acceleration over time, as well as the potential and kinetic energy of the ball-earth system. The emphasis is on the conservation of mechanical energy in a closed system.

05:03
📈 Graph Analysis and Energy Conservation

The second paragraph delves into the analysis of various graphs related to the ball's motion. It explains the relationship between the slope of the position versus time graph and average velocity, and how the velocity versus time graph reflects the average acceleration. The paragraph also discusses how the area under the acceleration versus time curve correlates with the change in velocity. It further explores the concept of momentum and its graphical representation, which parallels the velocity graph. The net force and its relation to the acceleration graph are also covered. The discussion concludes with the conservation of total mechanical energy, highlighting that the sum of kinetic and potential energy remains constant in a closed system without external forces.

Mindmap
Keywords
💡Momentum
Momentum, represented as the product of mass (m) and velocity (v), is a crucial concept in physics that describes the quantity of motion an object has. In the video, it is discussed in the context of a ball thrown upwards, where the momentum changes as the velocity changes over time. The momentum graph mirrors the velocity graph, highlighting that the ball's momentum is directly related to its speed and direction of motion.
💡Acceleration
Acceleration is the rate of change of velocity of an object with respect to time. In the video, the constant acceleration of the ball due to gravity is mentioned as -9.8 m/s^2, indicating that the ball is slowing down as it moves upwards and speeding up as it falls back down. This concept is essential for understanding changes in the ball's momentum and velocity.
💡Potential Energy
Potential energy is the stored energy an object has due to its position in a force field, such as the gravitational field of the Earth. In the video, the potential energy of the ball-Earth system is discussed in relation to the height of the ball above the ground. As the ball moves upwards, its potential energy increases, and as it falls back down, this potential energy is converted back into kinetic energy.
💡Kinetic Energy
Kinetic energy is the energy of motion an object possesses due to its movement. It is given by the formula 0.5 * m * v^2, where m is the mass and v is the velocity of the object. In the video, the kinetic energy of the ball is discussed in relation to its velocity, with the energy being entirely kinetic when the ball is thrown upwards and converting to potential energy at the peak of its trajectory.
💡Conservation of Energy
The principle of conservation of energy states that the total amount of energy in a closed system remains constant, as energy can neither be created nor destroyed, only converted from one form to another. In the video, this principle is used to explain that the total mechanical energy of the ball-Earth system, which includes both kinetic and potential energy, remains constant throughout the ball's motion, assuming no air resistance.
💡Net External Force
The net external force on an object is the vector sum of all external forces acting on it. In the video, the net external force on the ball is equated to the gravitational force, which is the product of the object's mass and the gravitational acceleration (m * g). This force is responsible for the ball's acceleration and deceleration during its trajectory.
💡Velocity
Velocity is a vector quantity that describes the rate of change of an object's position with respect to time, including both speed and direction. In the video, the velocity of the ball is central to understanding its motion as it is thrown upwards, reaches its peak, and falls back down.
💡Position
Position refers to the location of an object in space relative to a reference point. In the context of the video, the position of the ball changes as it moves upwards from the ground, reaches its highest point, and then returns to the ground. The position graph is used to illustrate the ball's vertical motion over time.
💡Mechanical Energy
Mechanical energy is the sum of potential and kinetic energy in a system. It is a conserved quantity in a closed system with no external work being done. In the video, the mechanical energy of the ball-Earth system is constant because energy is transferred between potential and kinetic forms without loss.
💡Gravitational Acceleration
Gravitational acceleration, often denoted as 'g', is the acceleration experienced by an object due to the gravitational pull of the Earth. In the video, the gravitational acceleration is given as -9.8 m/s^2, indicating that objects near the Earth's surface are pulled downwards with this acceleration.
💡Air Resistance
Air resistance is the force that opposes the motion of an object through the air. It is often neglected in idealized physical models to simplify the analysis. In the video, air resistance is neglected, allowing for a focus on the effects of gravity alone on the ball's motion.
Highlights

The scenario describes a ball thrown upwards with initial speed v-not.

The ball's motion involves going up to a maximum height where its velocity becomes zero, then falling back down.

Air resistance is neglected in this scenario, simplifying the analysis.

The acceleration of the ball is a constant -9.8 m/s^2, directed downwards.

The velocity graph starts positively, hits zero at the maximum height, and then becomes negative as the ball falls.

The position graph has a decreasing slope, indicating the ball's upward and downward motion.

The potential energy of the ball is represented by mgh over time, with m and g being constants.

The height of the ball increases, reaches a maximum, and then decreases, curving the potential energy graph.

Momentum is represented by mv, and the graph of momentum over time matches the velocity graph.

The net external force on the ball is equal to the mass times the gravitational acceleration (ma = mg).

The total mechanical energy of the ball-earth system remains constant due to the conservation of energy principle.

The kinetic energy of the ball is given by 1/2 mv^2, which does not reach a negative value due to the squared term.

The slope of the position versus time graph represents the average velocity.

The area under the acceleration versus time curve equals the change in velocity (delta v).

The graph of momentum versus time has the same shape as the velocity versus time graph.

The net force graph versus time mirrors the acceleration versus time graph.

The area under the net external force versus time graph equals the change in momentum or the impulse.

The potential energy versus time graph mirrors the vertical position versus time graph.

The kinetic energy versus time graph is related to the velocity versus time graph due to the formula for kinetic energy (1/2 mv^2).

The total kinetic energy of the graph is constant, representing the sum of the kinetic and potential energy graphs.

Transcripts

Browse More Related Video

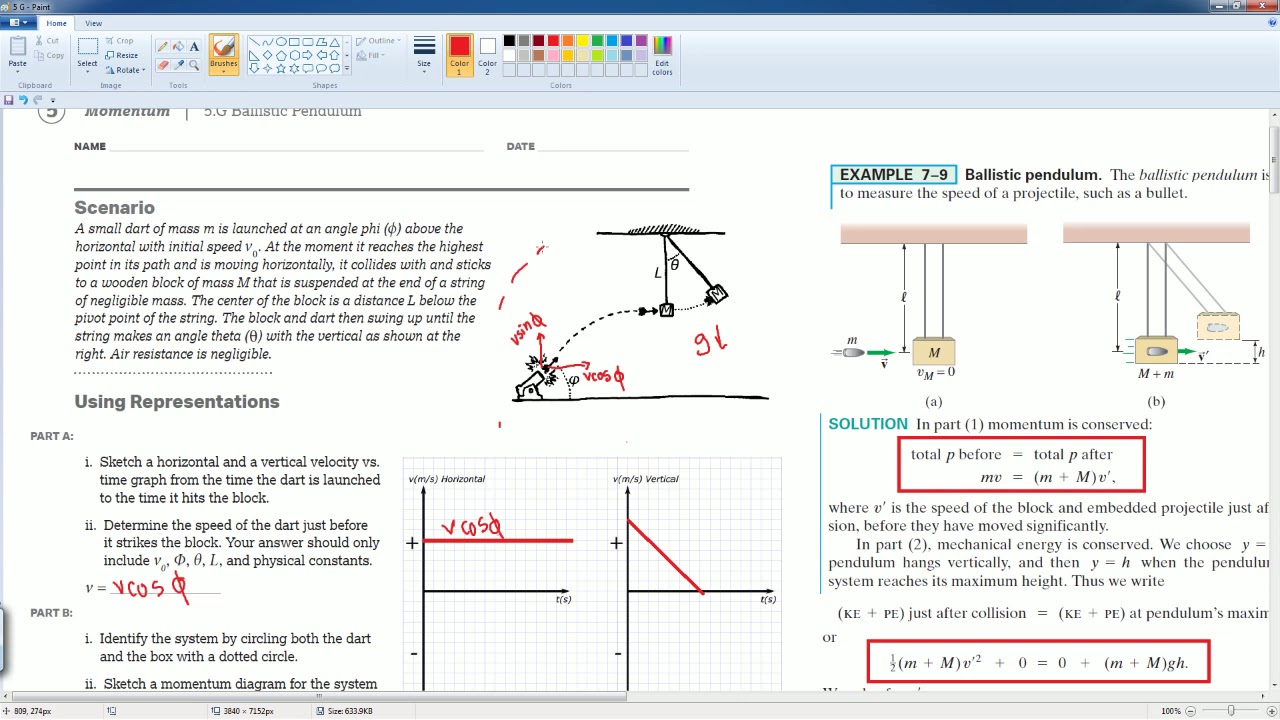
AP Physics Workbook 5.G Ballistic Pendulum

AP Physics Workbook 7.L Angular Momentum

AP Physics 1 review of Torque and Angular momentum | Physics | Khan Academy

AP Physics Workbook 5.J Explosions

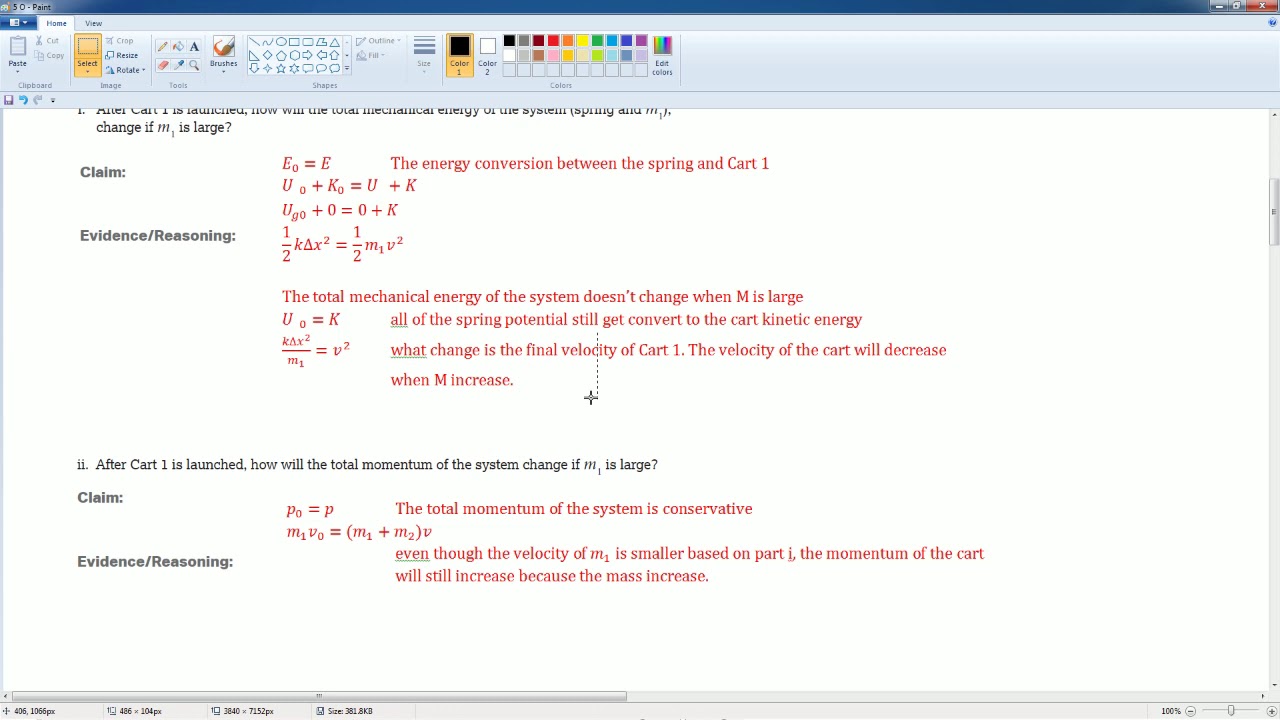
AP Physics Workbook 5.O Conservation of Energy and Momentum

2 | FRQ | Practice Sessions | AP Physics C: Mechanics

Rate This

5.0 / 5 (0 votes)

Thanks for rating:

Related Tags
PhysicsTutorialMomentumAnalysisEnergyConservationBallTrajectoryAccelerationGraphPotentialEnergyKineticEnergyClosedSystemMechanicalEnergyForceCalculation