What Is The Difference Between Odds and Probability? | Statistics

Learn2Stats
8 Jan 202104:27
EducationalLearning
32 Likes 10 Comments

TLDRThe video script clearly distinguishes between probability and odds, emphasizing that they are not interchangeable despite common misuse. It explains the formulas for calculating each, using the simple example of flipping a coin to illustrate the concepts. The video also covers how to convert between probability and odds, highlighting the different ranges for each. It concludes by noting the importance of using these terms correctly to avoid mathematical impossibilities and societal misunderstandings.

Takeaways
  • 📌 Probability and odds are not the same, and using them interchangeably is incorrect.
  • 📈 Probability is calculated as x over n, representing the number of desired outcomes over the total number of outcomes.
  • 🔄 Odds are expressed as one probability over another, simplified to x over (n - x), representing the ratio of the desired outcome to all other outcomes.
  • 🪙 An example to illustrate the difference is flipping a coin, where the probability of getting heads is 1/2 or 0.5, and the odds are 1 to 1.
  • 🔄 Converting from probability to odds is done by dividing the probability (p) by (1 - p).
  • 🔄 Converting from odds to probability is done by dividing the odds by (1 + the odds).
  • 📈 Probability ranges from 0 to 1, while odds can range from 0 to infinity.
  • 🤔 Smaller probabilities tend to converge with their odds, making them look similar.
  • 📊 Larger probabilities show a greater difference between the probability and odds, with odds being a larger decimal than the probability.
  • ⚠️ Mistakes such as stating a probability of 1.2 occur due to the misuse of probability and odds, which is a fundamental error.
  • 💡 Understanding the relationship and differences between probability and odds is essential for accurate calculations and interpretations.
Q & A

  • What is the main difference between probability and odds?

    -Probability is represented as x over n, a straightforward calculation of the desired outcome over the total number of outcomes. Odds, on the other hand, are expressed as one probability over another, which simplifies to x over (n - x) or in ratio form, x to (n - x).

  • Why is it incorrect to use probability and odds interchangeably?

    -Using probability and odds interchangeably can lead to misunderstandings and errors because they represent different aspects of the same event. Probability is the measure of the likelihood of an event occurring, while odds compare the likelihood of an event to the likelihood of its complement.

  • How do you calculate the odds of getting a head when flipping a coin?

    -The odds of getting a head when flipping a coin are calculated by taking the probability of heads (1/2 or 0.5) and dividing it by the probability of tails (also 1/2 or 0.5), which simplifies to 1 over 1, resulting in odds of 1 to 1.

  • What is the formula to convert probability to odds?

    -To convert probability to odds, you use the formula p over (1 - p), where p is the probability of the event occurring.

  • How do you convert odds back to probability?

    -To convert odds back to probability, you use the formula odds divided by (1 + odds), which gives you the probability of the event occurring.

  • What are the ranges of probability and odds?

    -The range of probability is from 0 to 1, representing the likelihood of an event occurring. The range of odds is from 0 to infinity, reflecting the ratio of the event occurring to the event not occurring.

  • Why do smaller probabilities tend to have odds that are closer to their value?

    -Smaller probabilities have odds that are closer to their value because as the probability decreases, the odds become a slightly larger representation of that probability, but still closely aligned in value.

  • How do larger probabilities affect the difference between their odds and the probability itself?

    -Larger probabilities have odds that are significantly larger than the probability itself. As the probability approaches 1, the odds approach infinity, while as the probability gets closer to 0, the odds approach 0.

  • What is a common mistake made when using probability and odds?

    -A common mistake is assigning a probability value of over 1, which is impossible. This error likely stems from the confusion between probability and odds, as odds can theoretically extend to infinity.

  • How do the concepts of probability and odds relate to each other?

    -Probability and odds are related in that odds contain the formula for probability within them. Understanding both concepts allows for a more nuanced understanding of the likelihood and comparison of events.

  • Why is it important to understand the difference between probability and odds?

    -Understanding the difference is crucial for accurate analysis and prediction of events. It helps avoid mathematical errors and ensures clear communication of statistical information.

Outlines
00:00
📊 Understanding the Distinction Between Probability and Odds

This paragraph explains the common misconception of using probability and odds interchangeably. It clarifies that while probability is represented as a fraction (x over n), odds are expressed as one probability over another (x over n minus x). The video aims to correct this misunderstanding and provide a clear distinction between the two concepts.

Mindmap
Keywords
💡Probability
Probability refers to the measure of the likelihood that a particular event will occur. It is quantified as a number between 0 and 1, with 0 indicating impossibility and 1 indicating certainty. In the video, the example of flipping a coin and getting heads has a probability of 1/2 or 0.5, meaning there's a 50% chance of this event occurring.
💡Odds
Odds are a way of expressing the ratio of the likelihood of an event occurring compared to it not occurring. They are expressed as a fraction, and unlike probability, odds can range from 0 to positive infinity. In the context of the video, the odds of getting heads when flipping a coin are calculated as 1/(2-1) or 1 to 1, which simplifies to 1.
💡Interchangeable
The term 'interchangeable' suggests that two things can be switched or replaced with one another without affecting the outcome. However, the video emphasizes that probability and odds are not interchangeable, despite common misuse. They have distinct calculations and interpretations, which is crucial for understanding and applying them correctly.
💡Coin Flip
A coin flip is a classic example used to illustrate probability and odds. In the video, it is used to demonstrate the calculation of both concepts. The probability of getting heads is 1/2, while the odds are expressed as 1 to 1, highlighting the difference between the two and how they are related.
💡Conversion
Conversion in the context of the video refers to the process of changing probability into odds or vice versa. The video provides formulas for converting probability (p) to odds (p / (1 - p)) and odds back to probability (odds / (1 + odds)). This allows for a deeper understanding of the relationship between these two statistical measures.
💡Ranges
Ranges indicate the possible values that a variable can take. In the video, it is mentioned that probability ranges from 0 to 1, while odds can range from 0 to infinity. Understanding these ranges is important for correctly interpreting and applying probability and odds in various scenarios.
💡Slight Differences
The video points out that smaller probabilities tend to have odds that are only slightly larger, such as the example of 1 in 10,000, which has an odds ratio of 1 to 9,999. This illustrates how closely related probability and odds can be in certain cases, despite their distinct definitions and calculations.
💡Significant Differences
The video also discusses significant differences between probability and odds when the probability is high. For instance, a probability of 9/10 or 0.9 has odds of 9 to 1, showing a more pronounced difference between the two measures. This highlights the importance of using the correct statistical measure for accurate analysis.
💡Misuse
Misuse in the context of the video refers to the incorrect use of probability and odds, such as stating a probability of 1.2, which is impossible. The video emphasizes the need to understand and correctly apply these terms to avoid such errors and to communicate effectively in statistical discussions.
💡Statistical Measures
Statistical measures are mathematical methods used to summarize and analyze data. In the video, probability and odds are discussed as two such measures that are often used to predict and understand the likelihood of events. Understanding their differences and proper application is crucial for accurate statistical analysis and interpretation.
💡Nerdy
The term 'nerdy' in the video is used in a light-hearted way to describe the enthusiasm and interest in learning about probability and odds. It reflects the video's aim to engage viewers in the sometimes complex, but fascinating, world of statistics and mathematics.
Highlights

The main difference between probability and odds is clarified, emphasizing that they are not interchangeable terms.

Probability is defined as a straightforward calculation, represented as x over n.

Odds are explained as one probability over another, simplifying to x over (n - x).

A simple example using a coin flip illustrates the relationship between probability and odds.

The probability of getting a head in a coin flip is 1 over 2 or 0.5.

The odds of getting a head in a coin flip are calculated as 1 to 1, highlighting the conversion process.

The method for converting probability to odds is presented as p over (1 - p).

The formula for converting odds back to probability is given as odds divided by (1 + odds).

The range of probabilities is from 0 to 1, whereas the range of odds is from 0 to infinity.

Incorrect usage of probability, such as stating a probability of 1.2, is criticized and attributed to the misuse of the terms.

As probabilities approach zero, they become more similar to their odds.

Larger probabilities exhibit greater differences when compared to their odds.

The odds will always be a larger decimal than the probability.

The video aims to correct common misconceptions about probability and odds through education.

The content is designed to help viewers understand the distinct calculations and applications of probability and odds.

The video encourages viewers to like and subscribe for more informative content.

Transcripts

Browse More Related Video

Medical Statistics - Part 4: Calculating Odds

Statistics Lecture 4.2: Introduction to Probability

4.1.5 Basics of Probability - Odds and Their Relation to Probability

Theoretical Probability, Permutations and Combinations

4.1.0 Basics of Probability - Lesson Overview, Key Concepts and Learning Outcomes

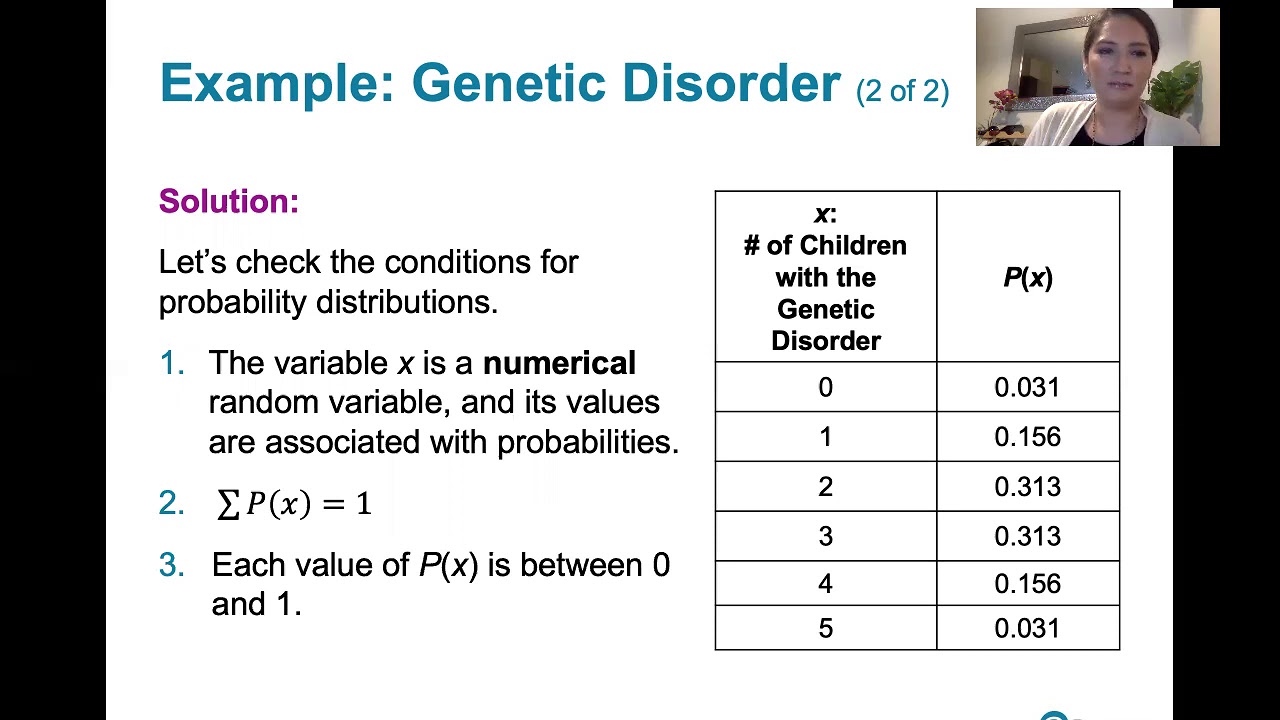
5.1.2 Discrete Probability Distributions - Probability Distributions and Probability Histograms

Rate This

5.0 / 5 (0 votes)

Thanks for rating:

Related Tags
ProbabilityOddsMathematicsEducationComparisonCoin FlipStatisticsUnderstandingNumerical AnalysisConcept Clarification