Solid of Revolution (part 1)

Khan Academy

25 Apr 200810:05

EducationalLearning

32 Likes 10 Comments

TLDRThe video script explains the concept of using definite integrals to calculate the volumes of rotational solids. It begins by discussing how integrals can determine areas under curves, then extends this to rotational volumes by rotating a function around the x-axis, transforming rectangles into disks. The volume of each disk is found using the formula pi * (f(x))^2 * dx, where f(x) represents the function. The total volume of the solid is obtained by integrating this expression over the interval [a, b]. The explanation emphasizes understanding the concepts behind calculus rather than just memorizing formulas.

Takeaways

📈 The concept of definite integrals can be applied to find volumes of rotational solids, in addition to calculating areas under curves.
🌀 To find the volume of a solid created by rotating a function around the x-axis, you calculate the volume of infinitesimally thin disks and sum them up.
📊 The volume of each small disk is found by using the formula for the area of a circle (π times the radius squared) and multiplying by the width element (dx).
🎨 The radius of the disk corresponds to the value of the function f(x) at a specific x-value, and the width is the differential dx.
🔄 The process of finding the volume of a rotational solid involves rotating rectangles (or small sections of the curve) around the x-axis to form disks.
📚 The mathematical representation of the volume of a rotational solid is the definite integral from a to b of πf(x)^2 dx, where f(x) is the function being rotated and a and b are the interval limits.
🖌️ The script provides a visual example of rotating y=√x around the x-axis to create a solid, which would resemble a sideways copper vase.
🤔 Understanding the geometric interpretation of calculus problems is emphasized as crucial for problem-solving, rather than just memorizing formulas.
👀 Visualization is identified as the most challenging part of these problems, so it's important to first imagine the process of rotation and the resulting solid.
🚀 The video script serves as an introduction to the concept, with a promise of a practical application in the next video.

Q & A

How do definite integrals relate to finding areas under curves?
-Definite integrals help in calculating the areas under curves by summing up an infinite number of infinitely small rectangles, each with a width dx and height equal to the function value f(x) at that point.
What is the significance of rotating a function around the x-axis?
-Rotating a function around the x-axis creates a rotational solid, and the volume of this solid can be determined using principles similar to those used for calculating definite integrals for areas.
What does the function y equal the square root of x represent?
-The function y equals the square root of x represents a curve where the y-value is the square root of the x-value. It is used as an example in the script to illustrate the concepts of areas under curves and volumes of rotational solids.
How is the area under a curve between two points calculated?
-The area under a curve between two points is calculated by determining the definite integral from the lower bound (a) to the upper bound (b) of the function f(x) multiplied by dx.
What is the shape of the solid formed by rotating y equals the square root of x around the x-axis?
-Rotating y equals the square root of x around the x-axis forms a shape resembling a sideways copper vase or a circular solid with two circular ends and a curved surface in between.
How do you find the volume of a rotational solid created by rotating a function around the x-axis?
-The volume of a rotational solid is found by summing the volumes of infinitesimally thin disks created by rotating rectangles under the curve around the x-axis. The volume of each disk is calculated using the formula pi * (f(x)^2) * dx.
What is the formula for the volume of a disk?
-The formula for the volume of a disk is pi * r^2, where r is the radius of the disk.
How is the volume of a rotational solid expressed mathematically?
-The volume of a rotational solid is expressed as the definite integral from a to b of pi * (f(x)^2) * dx, where f(x) represents the function being rotated and a and b are the bounds of rotation.
Why is visualization important in problems involving rotational solids?
-Visualization is crucial in understanding the shape and structure of the rotational solid, which helps in applying the correct mathematical principles to find the volume.
What is the relationship between the height of a small rectangle in the area calculation and the radius of a disk in the volume calculation?
-In the area calculation, the height of a small rectangle is the function value f(x) at a specific x-value. In the volume calculation, this height corresponds to the radius of the disk created by rotating the rectangle around the x-axis.
How does the concept of definite integrals extend to finding volumes of rotational solids?
-The concept of definite integrals extends to finding volumes of rotational solids by summing the volumes of infinitesimally thin disks instead of rectangles. This sum is represented by a definite integral involving the function of x squared, multiplied by dx, and multiplied by pi.

Outlines

00:00

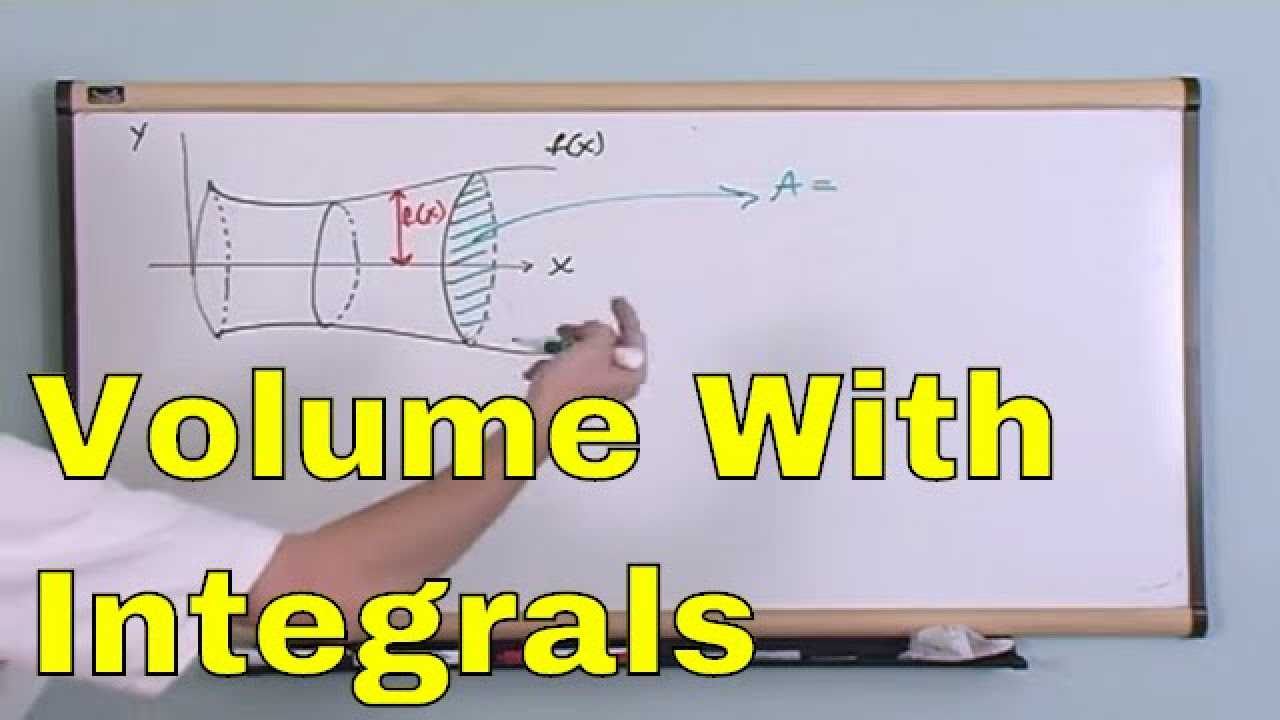
📊 Understanding Definite Integrals for Areas and Rotational Volumes

This paragraph introduces the concept of using definite integrals to calculate areas under curves and extends this principle to find the volumes of rotational solids. It begins with a review of how to find the area under a curve, such as y equals the square root of x, using the fundamental idea of summing up small rectangles with width dx and height f(x). The speaker then transitions to the idea of rotating the function around the x-axis to form a solid, like a sideways copper vase, and explains that the volume of this solid can be found by summing up the volumes of disks created by rotating these rectangles around the x-axis.

05:01

🎨 Visualizing Rotational Solids and Calculating Disk Volumes

In this paragraph, the focus is on visualizing the process of rotating the curve around the x-axis to form a solid and calculating the volume of the resulting solid. The speaker emphasizes the challenge of visualizing the 3D shape created by rotating the function and explains that each small rectangle on the curve, when rotated around the x-axis, becomes a disk with radius f(x) and width dx. The volume of each disk is then found using the formula for the area of a circle, pi times the radius squared, resulting in pi times f(x) squared. The total volume of the rotational solid is obtained by integrating this volume formula over the interval from a to b.

10:01

👋 Conclusion and Future Application

The paragraph concludes the discussion on calculating the volumes of rotational solids using definite integrals. The speaker briefly reiterates the process and formula used to find the volume of a rotational solid and encourages understanding the underlying concepts rather than just memorizing formulas. The speaker also mentions that the next video will apply the discussed concepts to solve an actual problem, indicating a continuation of the topic in future content.

Mindmap

Keywords

💡Definite Integrals

Definite integrals are a fundamental concept in calculus that allow us to calculate the area under a curve or between curves. In the context of the video, they are introduced as a way to understand the foundational principles that will later be applied to find the volumes of rotational solids. The definite integral from point 'a' to 'b' of a function 'f(x)' is denoted as the integral from 'a' to 'b' of 'f(x) dx', and it represents the signed area between the curve and the x-axis over the given interval.

💡Rotational Solids

Rotational solids are three-dimensional shapes that are created by rotating a two-dimensional shape, called a cross-section, around an axis. In the video, the concept is used to explain how the volume of such solids can be calculated using the principles of definite integrals. The process involves rotating a function around an axis, such as the x-axis, and then summing up the volumes of all the infinitesimally thin disks created by this rotation.

💡Volumes

Volumes refer to the amount of space occupied by a solid object. In the context of the video, calculating volumes is the primary application of the rotational solids concept. The video explains that by rotating a function around an axis and summing the volumes of the resulting cross-sectional disks, one can find the total volume of the created solid.

💡Cross-Sections

Cross-sections are the shapes that would be left if the rotational solid were sliced by a plane. In the context of the video, the cross-sections are the circles formed by the rotation of the rectangles (with width dx and height f(x)) around the x-axis. These cross-sections are crucial for calculating the volume of the rotational solid, as their areas are used in the integral to find the volume of each infinitesimally thin disk, which is then summed up to find the total volume.

💡Disks

Disks, in this context, refer to the cross-sectional shapes that result from rotating a rectangle around the x-axis. Each rectangle, with width dx and height f(x), becomes a disk with radius f(x) and thickness dx when rotated. The area of each disk is calculated using the formula pi times the radius squared, which is then used to find the volume of each disk by multiplying by the thickness dx.

💡Area

Area is a measure of the extent of a two-dimensional surface. In the video, the area is a concept that is first introduced in relation to finding the area under a curve, which is then extended to finding the volume of solids formed by rotation. The area of a shape is fundamental to understanding how to calculate the volume of rotational solids since the volume is found by summing the areas of the cross-sectional disks.

💡Pi (π)

Pi, often denoted by the Greek letter 'π', is a mathematical constant that represents the ratio of a circle's circumference to its diameter. In the context of the video, pi is used in the formula for calculating the area of a disk, which is pi times the radius squared. This constant is crucial when calculating the volume of a rotational solid formed by rotating a function around the x-axis, as each cross-section is a disk and the volume of each disk is found by multiplying the area by the thickness dx.

💡Integration

Integration is a process in calculus that involves finding the accumulated quantity, such as area or volume, by summing up infinitesimally small parts. In the video, integration is used to find the volume of a rotational solid by summing up the volumes of all the infinitesimally thin disks created by rotating the cross-sections around the x-axis.

💡Visualization

Visualization is the process of creating a mental or graphical representation of a mathematical concept or problem. In the video, visualization is essential for understanding how a two-dimensional curve becomes a three-dimensional rotational solid when rotated around an axis. The ability to visualize the rotation and the resulting solid helps in grasping the concept of calculating volumes using definite integrals.

💡Square Root

A square root is a mathematical operation that finds the value that, when multiplied by itself, gives the original number. In the video, the function y = sqrt(x) represents a curve where the y-value is the square root of the x-value. This function is used as an example to demonstrate how to find the area under the curve and how to calculate the volume of the rotational solid formed by rotating the curve around the x-axis.

Highlights

Definite integrals can be used to find areas under or between curves.

The principles of definite integrals can be applied to calculate the volumes of rotational solids.

The function y equals the square root of x is used as an example to illustrate the concept.

Area under a curve is found by summing up small rectangles with width dx and height f(x).

The area under a curve between two points a and b is given by the definite integral from a to b of f(x) dx.

Understanding the concepts behind calculus is emphasized over mere mechanical application.

Rotating a function about the x-axis creates a 3D shape, like a sideways copper vase.

The volume of a rotational solid is found by summing the volumes of infinitely small disks created by rotating rectangles around the x-axis.

Each rectangle with width dx and height f(x) becomes a disk with radius f(x) when rotated around the x-axis.

The volume of a single disk is calculated as the area of the disk (pi times the radius squared) times dx.

The area of a disk obtained from rotation is pi times f(x) squared.

The volume of the entire rotational solid is the definite integral from a to b of pi f(x) squared dx.

Visualization is key to solving problems involving rotational solids and volumes.

The process of finding the volume of a rotational solid involves an infinite sum of small disks.

The height of the rectangle (f(x)) becomes the radius of the disk, and its width (dx) represents the thickness.

The volume of the rotational solid is the sum of the volumes of the disks, which is an integral of the area of the disks times dx.

The formula for the volume of a rotational solid around the x-axis is V = pi * (f(x)^2) * dx, integrated from a to b.

Transcripts

Browse More Related Video

Disc method around x-axis | Applications of definite integrals | AP Calculus AB | Khan Academy

Solid of Revolution (part 2)

Lecture 20: Several Variables Volumes Galore

2011 Calculus AB free response #3 (c) | AP Calculus AB | Khan Academy

Solid of Revolution (part 5)

Lesson 12 - Calculating Volume With Integrals (Calculus 1 Tutor)

Solid of Revolution (part 1)

Takeaways

Q & A

How do definite integrals relate to finding areas under curves?

What is the significance of rotating a function around the x-axis?

What does the function y equal the square root of x represent?

How is the area under a curve between two points calculated?

What is the shape of the solid formed by rotating y equals the square root of x around the x-axis?

How do you find the volume of a rotational solid created by rotating a function around the x-axis?

What is the formula for the volume of a disk?

How is the volume of a rotational solid expressed mathematically?

Why is visualization important in problems involving rotational solids?

What is the relationship between the height of a small rectangle in the area calculation and the radius of a disk in the volume calculation?

How does the concept of definite integrals extend to finding volumes of rotational solids?