Solid of Revolution (part 5)

Khan Academy
27 Apr 200809:29
EducationalLearning
32 Likes 10 Comments

TLDRThe video script discusses the concept of rotating a function around the y-axis to find the volume of the resulting solid. It introduces the shell method as an alternative to the disk method used for x-axis rotations. The example function, y = x^2, is rotated around the y-axis from x = 0 to x = 1. The script explains calculating the surface area of a ring, which is then used to find the volume of the shell. The final volume is determined by integrating the expression 2Ο€x * f(x) over the given interval, with a specific example yielding a volume of Ο€/2.

Takeaways
  • πŸ“ Rotating a function around the y-axis can create a new shape, different from the disk method used for x-axis rotations.
  • πŸŒ€ The volume of a shape obtained by rotating a function around the y-axis can be calculated using the shell method.
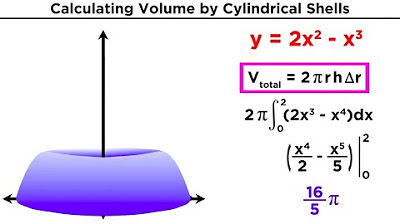
  • πŸ“Š The shell method involves considering the shape as a series of cylindrical shells, each with a height equal to the function's value at a given x point.
  • πŸ”„ The base of the rotated shape resembles a cylinder, but the inside is hollow, creating a 'bowl-like' appearance.
  • 🎨 The surface area of each shell is calculated as the circumference of the ring (2Ο€ times the radius) times the height of the shell.
  • πŸ“ The radius of each shell is the distance from the y-axis to the point on the function, which is x.
  • πŸ“ˆ The volume of each shell is found by multiplying the surface area of the shell by its width (dx).
  • 🧩 To find the total volume of the entire shape, integrate the volume of each shell over the interval of interest.
  • 🌠 For the specific function y = x^2, the volume when rotated around the y-axis from 0 to 1 is calculated as ∫(from 0 to 1) of 2Ο€x * x^2 dx, which simplifies to 2Ο€ * (x^3/3) from 0 to 1.
  • πŸ”’ The antiderivative of x^3 is x^4/4, and evaluating this from 1 to 0 gives the volume as 2Ο€(1/4 - 0), which equals Ο€/2.
  • πŸŽ“ Understanding the geometry and calculus concepts involved is crucial for visualizing and calculating the volume of rotated shapes.
Q & A

  • What is the main topic of the video script?

    -The main topic of the video script is the concept of rotating a function around the y-axis to find the volume of the resulting solid figure, specifically using the shell method.

  • What function is used as an example in the script?

    -The function used as an example in the script is y = x squared.

  • Why can't the disk method be used for rotation around the y-axis?

    -The disk method cannot be used for rotation around the y-axis because it is designed for rotation around the x-axis, where disks can be formed. For rotation around the y-axis, the shell method is more appropriate as it accounts for the cylindrical shape formed by the rotation.

  • What is a shell in the context of the shell method?

    -In the context of the shell method, a shell is a thin cylindrical slice of the solid figure formed by rotating a function around the y-axis. It has a height equal to the function value at a particular x value and a radius equal to the x value.

  • How is the surface area of a shell calculated?

    -The surface area of a shell is calculated by multiplying the circumference of the circular base of the shell (which is 2 pi times the radius) by the height of the shell (which is the function value at a particular x value).

  • What is the volume of a single shell in the script's explanation?

    -The volume of a single shell is calculated by multiplying the surface area of the shell by its width (dx), resulting in the formula 2 pi * x * f(x) * dx.

  • How is the total volume of the rotated figure found?

    -The total volume of the rotated figure is found by integrating the volume of a single shell over the entire range of x values, in this case from 0 to 1.

  • What is the final volume of the rotated figure when the function is x squared?

    -The final volume of the rotated figure when the function is x squared is pi/2, calculated by integrating x^3 from 0 to 1 and evaluating the antiderivative at the bounds.

  • What is the significance of the boundaries 0 and 1 in the integral?

    -The boundaries 0 and 1 in the integral represent the range of x values over which the function is being rotated around the y-axis. The lower bound is 0 and the upper bound is 1.

  • How does the script emphasize the importance of drawing in understanding the problem?

    -The script emphasizes the importance of drawing in understanding the problem by showing that it helps visualize the shape and structure of the solid formed by the rotation, which is crucial for applying the correct method (shell method in this case) to find the volume.

Outlines
00:00
πŸ“ Exploring Rotation Around the Y-Axis

The paragraph introduces the concept of rotating a function around the y-axis, using the specific example of y equals x squared. The speaker begins by drawing the axes and explaining the symmetry of the function, which allows for a general approach to the problem. The focus is on visualizing the resulting shape when the function is rotated around the y-axis, which is described as a hollowed-out cylinder or a bowl on the inside and a cylinder on the outside. The speaker emphasizes the challenge of drawing the shape effectively to aid understanding. The method of calculating the volume is then transitioned from the disk method used for rotation around the x-axis to the shell method, which is applicable for rotation around the y-axis. The shell method involves considering the volume of individual 'shells' formed by rotating thin rectangles around the y-axis, with the height of each shell being the function's value at a given x point.

05:02
πŸ“š Calculating the Surface Area and Volume of the Shells

This paragraph delves into the specifics of the shell method, focusing on how to calculate the surface area and volume of the individual shells formed by the rotation. The surface area of a shell is determined by the circumference of the ring (2 pi times the radius) multiplied by the height of the shell (the function's value at a given x point). The volume of a shell is calculated by multiplying the surface area by the width of the shell, which is represented by the differential dx. The speaker then explains how to find the total volume of the entire rotated figure by summing up the volumes of all the shells, which involves integrating the expression for the volume of a single shell over the interval from 0 to 1. The example provided uses the function x squared, and the calculation results in a volume of pi over 2, demonstrating the application of the shell method for finding the volume of a solid created by rotating a function around the y-axis.

Mindmap
Keywords
πŸ’‘rotation
Rotation refers to the process of turning a shape around a central point or axis in a specific direction and for a certain angle. In the context of the video, the main theme revolves around rotating a function around the y-axis to create a 3D shape, which is a fundamental concept in calculus for determining volumes of solids of revolution.
πŸ’‘y-axis
The y-axis is one of the two principal axes used in the Cartesian coordinate system to define a point in a two-dimensional space. In the video, the y-axis is the central axis around which the function is being rotated, signifying a shift from the usual x-axis rotations to explore new geometrical possibilities.
πŸ’‘x-axis
The x-axis, also known as the horizontal axis, is the other principal axis in the Cartesian coordinate system that intersects with the y-axis at the origin. In the video, the x-axis is used as a reference to define the shape of the function being rotated and to establish the coordinate system for the 3D shape.
πŸ’‘f(x)
f(x) represents a function of x, which is a mathematical relationship that assigns a unique output value to each input value of x. In the video, f(x) is used to denote the function being rotated around the y-axis, and the specific function given is f(x) = x^2, which is a quadratic function.
πŸ’‘volume
Volume refers to the amount of space occupied by a three-dimensional object. In the context of the video, calculating volume involves using calculus to determine the space enclosed by the solid formed when a 2D shape is rotated around the y-axis.
πŸ’‘disk method
The disk method is a technique used in calculus to find the volume of a solid of revolution by rotating a function around the x-axis. It involves integrating the cross-sectional area of the solid, which is approximated as a series of disks, over the interval of rotation.
πŸ’‘shell method
The shell method is an alternative technique in calculus for finding the volume of a solid of revolution when rotating around the y-axis. It involves integrating the surface area of cylindrical 'shells' formed by rotating narrow vertical strips of the 2D shape, which is then multiplied by the width of each strip (dx).
πŸ’‘circumference
Circumference is the length of the edge of a circle or any circular curve. In the video, circumference is used to calculate the surface area of each cylindrical shell that makes up the 3D shape when a function is rotated around the y-axis.
πŸ’‘antiderivative
An antiderivative, also known as an indefinite integral, is a function whose derivative is equal to the given function. In the context of the video, finding the antiderivative of a function is crucial for calculating the volume of the solid formed by rotation using the shell method.
πŸ’‘integral
An integral is a mathematical concept that represents the accumulation of a quantity, often used to calculate the area under a curve or the volume of a solid. In the video, the integral is used to sum up the volumes of infinitesimally thin shells to find the total volume of the solid formed by rotating the function around the y-axis.
πŸ’‘calculus
Calculus is a branch of mathematics that deals with rates of change and accumulations. It is used to solve problems involving functions and their derivatives and integrals. In the video, calculus is the foundation for the methods discussed, such as the disk and shell methods, to calculate volumes of solids of revolution.
Highlights

Introduction to rotating around the y-axis, as opposed to the x-axis.

Drawing the axes for visual reference, with the y-axis and x-axis clearly defined.

Using the general function f(x) to make the example adaptable to various functions.

Selection of the specific function y = x^2 for the rotation example.

Exploration of the concept of volume when rotating around the y-axis, as opposed to the x-axis.

Introduction to the concept of 'area' when discussing rotation around the y-axis.

Visualization of the rotation's outcome as a cylinder-like shape with a hollow interior.

Explanation of the shell method as an alternative to the disk method for calculating volume.

Description of a shell as a geometric figure and its relation to the original function f(x).

Calculation of the surface area of a shell using the circumference and height.

Derivation of the volume of a single shell and its components.

Integration of the shell volumes over the interval to find the total volume.

Use of calculus principles to understand the infinitely small contributions to the volume.

Application of the shell method to the specific function f(x) = x^2.

Calculation of the volume of the rotated figure using integration and the antiderivative.

Final result of the volume calculation, demonstrating the practical use of the shell method.

Anticipation of further discussion in the next video, creating a continuity in the learning process.

Transcripts

Browse More Related Video

Solid of Revolution (part 7)

Disc method around x-axis | Applications of definite integrals | AP Calculus AB | Khan Academy

Disc method rotating around vertical line | AP Calculus AB | Khan Academy

Solid of Revolution (part 2)

Calculating Volume by Cylindrical Shells

Generalizing the washer method | Applications of definite integrals | AP Calculus AB | Khan Academy

Rate This

5.0 / 5 (0 votes)

Thanks for rating:

Related Tags
CalculusVolume of RevolutionShell Methody-axis RotationMathematical ConceptsGeometryIntegrationx^2 FunctionEducational ContentVisual Explanation