Uniform Circular Motion Formulas and Equations - College Physics
TLDRThis video script offers a comprehensive review of the key concepts and formulas related to uniform circular motion, emphasizing the importance of centripetal acceleration and force. It explains how velocity, period, and frequency are interconnected and demonstrates how to calculate the tension in a rope when an object is moving in a circular path. The script also addresses the calculation of normal force on an inclined plane, highlighting the conditions under which an object may lose contact with the ground due to excessive speed. The video aims to prepare students for tests by reinforcing their understanding of these fundamental principles through practical examples and problem-solving techniques.
Takeaways
- 📚 The video is a review for uniform circular motion test preparation.
- 🏃♂️ Objects in uniform circular motion move at a constant speed along a circular path.
- 🔄 Velocity vector direction changes continuously, while speed remains constant.
- 🌀 Centripetal acceleration always points towards the center of the circle and is given by the formula: $a_c = \frac{v^2}{r}$.
- 🔧 Newton's Second Law applied to circular motion: $F_c = m a_c = m \frac{v^2}{r}$.
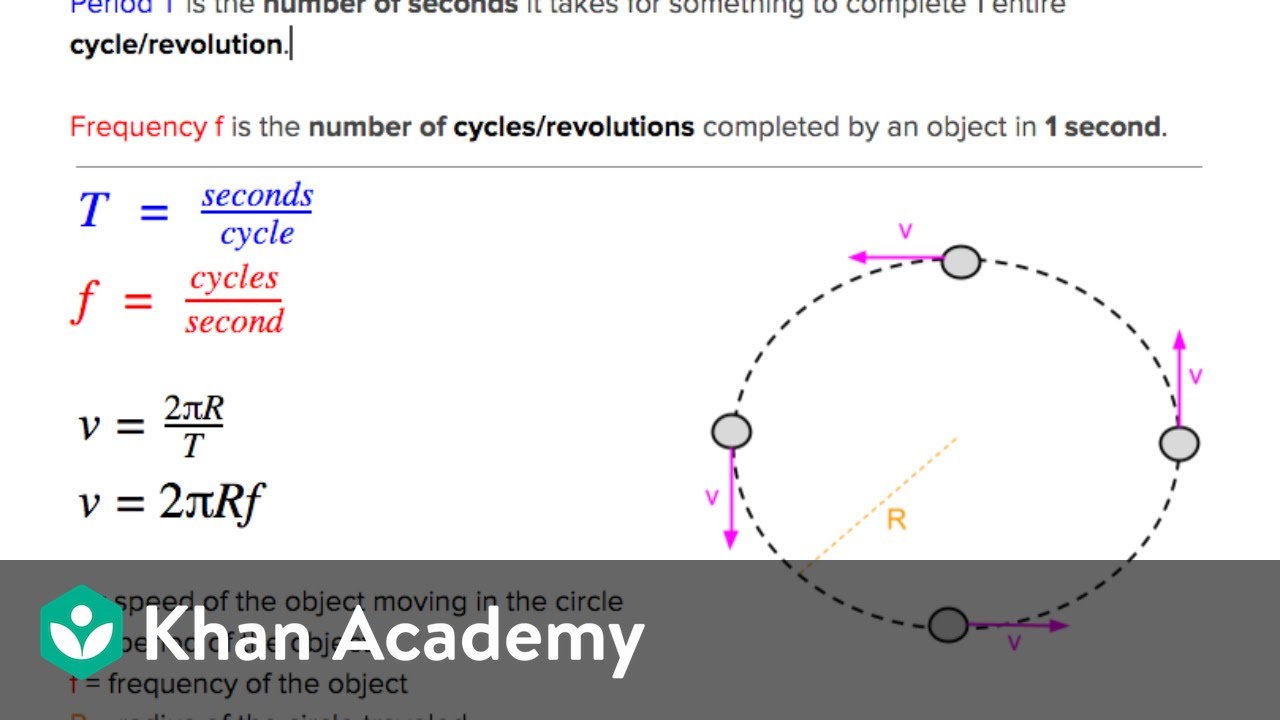
- 📏 Circumference of a circle is $2\pi r$, and the period (T) is the time for one complete revolution.
- 🚶♂️ Frequency (f) is the reciprocal of the period: $f = \frac{1}{T}$, measured in hertz (Hz).
- 🌐 At points A and C in a vertical circle, tension equals centripetal force: $T = m v^2 / r$.
- 🔽 At the bottom of a vertical circle (point D), tension equals the sum of centripetal force and weight (mg).
- 🔼 At the top of a vertical circle (point B), tension is at its minimum, equal to the difference between weight and centripetal force.
- 📊 For an object on an inclined plane, the normal force can be calculated based on the object's speed and the geometry of the situation.
Q & A
What is uniform circular motion?
-Uniform circular motion is when an object moves in a circular path at a constant speed. The speed is constant, but the velocity changes direction continuously, which means there is a centripetal acceleration towards the center of the circle.
What is the direction of the velocity vector in uniform circular motion?
-The direction of the velocity vector is tangential to the circle at the object's position and changes as the object moves. If the object is moving counterclockwise, the velocity vector will point upwards at the top of the circle.
What is centripetal acceleration, and how is it calculated?
-Centripetal acceleration is the acceleration that keeps an object moving in a circular path. It is always directed towards the center of the circle. It is calculated using the formula a_c = v^2/r, where v is the velocity of the object and r is the radius of the circle.
How does the centripetal force relate to centripetal acceleration?
-The centripetal force is the net force acting on an object in circular motion, directed towards the center of the circle. It is equal to the mass of the object times the centripetal acceleration (F_c = m * a_c) and can be calculated using the formula F_c = mv^2/r.
What is the relationship between velocity and the period of circular motion?
-The velocity of an object in uniform circular motion is related to the period (the time it takes to complete one revolution) by the formula v = 2πr/T, where r is the radius of the circle and T is the period.
How does the tension force in a rope change at different points of circular motion?
-At points A and C (top and bottom of the circle), the tension force in the rope is approximately equal to the centripetal force (F_t ≈ mv^2/r). At point D (bottom of a vertical circle), the tension force is the sum of the centripetal force and the weight of the object. At point B (the lowest point of a horizontal circle), the tension force is the weakest and is the difference between the centripetal force and the weight force.
What is the formula for calculating the normal force on an object moving on a hill?
-At the bottom of the hill, the normal force is the sum of the centripetal force and the weight force, while at the top, it is the difference between the weight force and the centripetal force. The exact formulas depend on the speed of the object and its position on the hill.
What happens when the normal force becomes negative in the context of an object moving on a hill?
-If the normal force becomes negative, it means that the object loses contact with the ground and is essentially flying off. A positive normal force indicates that the object remains in contact with the ground.
How can you calculate the tension in a rope when an object is moving in a horizontal circle?
-In a horizontal circle, the tension force in the rope can be calculated by considering both the horizontal (TX) and vertical (TY) components. The formula for the tension force is √(TX^2 + TY^2), where TX provides the centripetal force and TY supports the weight of the object.
What is the significance of the angle in the context of calculating tension in a rope?
-The angle is significant when calculating the tension in a rope because it affects the magnitude of the horizontal (TX) and vertical (TY) components of the tension force. If the object is moving fast enough, the angle is close to zero, and TX is approximately equal to the centripetal force. If the object is not moving very fast, the angle is significant, and both TX and TY components must be considered.
Where can one find example problems related to the formulas discussed in the script?
-Example problems related to the formulas discussed can be found in the description section below the video or by checking out the links provided. There are other videos in the chapter where these equations are practiced.
Outlines
📚 Introduction to Uniform Circular Motion
This paragraph introduces the concept of uniform circular motion, emphasizing objects moving in a circle at a constant speed. It explains the difference between velocity and acceleration in this context, highlighting that while speed is constant, the direction changes, leading to centripetal acceleration. The paragraph also introduces the formula for centripetal acceleration (a = v^2/r) and relates it to Newton's Second Law, explaining the concept of centripetal force (Fc = ma). The importance of understanding these concepts and formulas for a test on the subject is stressed.
🔄 Calculating Velocity and Tension in Circular Motion
The second paragraph delves into the calculation of velocity and tension in uniform circular motion. It explains how to determine the period (T) and frequency of an object in circular motion and uses these to derive the velocity (v = 2πr/T) and centripetal acceleration (a = 4π^2r/T^2). The paragraph further discusses the tension force in a rope when an object is moving in a circular path, especially at different points such as at the top and bottom of a vertical circle, and how it changes depending on the position and speed. It also touches on the horizontal circular motion and the components of tension force in such scenarios.
🏞️ Normal Force on an Incline: Hill Example
The final paragraph discusses the application of concepts from the previous sections to a real-world scenario involving an object moving on an incline, specifically a hill. It explains how to calculate the normal force exerted by the ground on the object at the bottom and top of the hill. The paragraph clarifies that the normal force at the bottom is at its maximum, supporting both the weight of the object and providing the centripetal force for turning. Conversely, at the top, the normal force is at its minimum, and if the speed is too high, resulting in a negative normal force, the object loses contact with the ground. The video ends with a prompt to check out additional resources for further understanding and practice.
Mindmap
Keywords
💡Uniform Circular Motion
💡Centripetal Acceleration
💡Centripetal Force
💡Velocity
💡Circumference
💡Period
💡Tension Force
💡Normal Force
💡Newton's Second Law
💡Tangential Force
💡Frequency
Highlights
The video is a review of formulas for uniform circular motion.
Uniform circular motion involves objects moving in a circle at a constant speed.
The velocity vector direction changes continuously, even though the speed is constant.
Centripetal acceleration always points towards the center of the circle and is responsible for the change in direction.
The formula for centripetal acceleration is a = v^2 / r, where 'a' is the acceleration, 'v' is the velocity, and 'r' is the radius.
Doubling the velocity results in a fourfold increase in centripetal acceleration.
Acceleration is the change in velocity divided by the change in time.
According to Newton's Second Law, the net force on an object is equal to mass times acceleration, leading to the concept of centripetal force.
The formula for centripetal force is Fc = m*v^2 / r, where 'Fc' is the centripetal force.
The velocity of an object in uniform circular motion can be calculated using the formula v = 2πr/T, where 'T' is the period.
The period 'T' is the time taken for one complete revolution and is related to frequency, which is 1/T.
Tension force in a rope provides the centripetal force at points A and C in a circular path.
At the bottom of a vertical circle (point D), the tension force equals the sum of the centripetal force and the weight of the object.
At point B, the tension force is the weakest and equals the difference between the centripetal force and the weight force.
In a horizontal circle, the tension force is calculated as the square root of (Tx^2 + Ty^2), with Tx providing the centripetal force.
The normal force on an object moving on a hill can be calculated by considering the balance between gravitational force and centripetal force.
At the top of the hill, if the normal force becomes negative, the object loses contact with the ground.
The video provides a comprehensive overview of the physics concepts and formulas needed for understanding uniform circular motion.
Additional example problems and practice exercises are available in the video description for further understanding.
Transcripts
Browse More Related Video
Introduction to Centripetal Acceleration - Period, Frequency, & Linear Speed - Physics Problems
Uniform Circular Motion - IB Physics
Dynamics of Uniform Circular Motion
Centripetal Force Physics Problems - Calculate Tension & Maximum Speed - Uniform Circular Motion

AP Physics 1 - Simple Harmonic Motion
AP Physics 1 review of Centripetal Forces | Physics | Khan Academy
5.0 / 5 (0 votes)
Thanks for rating: