AP Physics 1 review of Centripetal Forces | Physics | Khan Academy
TLDRThis transcript delves into fundamental concepts of physics, including period, frequency, centripetal acceleration, and gravitational forces. It explains how the period and frequency are inversely related, detailing their relationship with speed and radius in circular motion. The concept of centripetal acceleration, which alters the direction of velocity, is contrasted with tangential acceleration, which changes speed. The role of centripetal forces in circular motion, the universal law of gravitation, and the concept of gravitational fields and orbits are also elucidated, providing examples to illustrate these principles in practical scenarios.
Takeaways
- 📈 The period is the time taken for a complete cycle of a repeating process and is measured in seconds.
- 🔄 Frequency is the number of cycles completed in one second and is measured in hertz (Hz).
- 🔄 The relationship between period and frequency is inverse; the period is one over the frequency, and vice versa.
- 📊 Speed, radius, and period are interrelated in circular motion, with speed calculated as two pi R times the frequency.
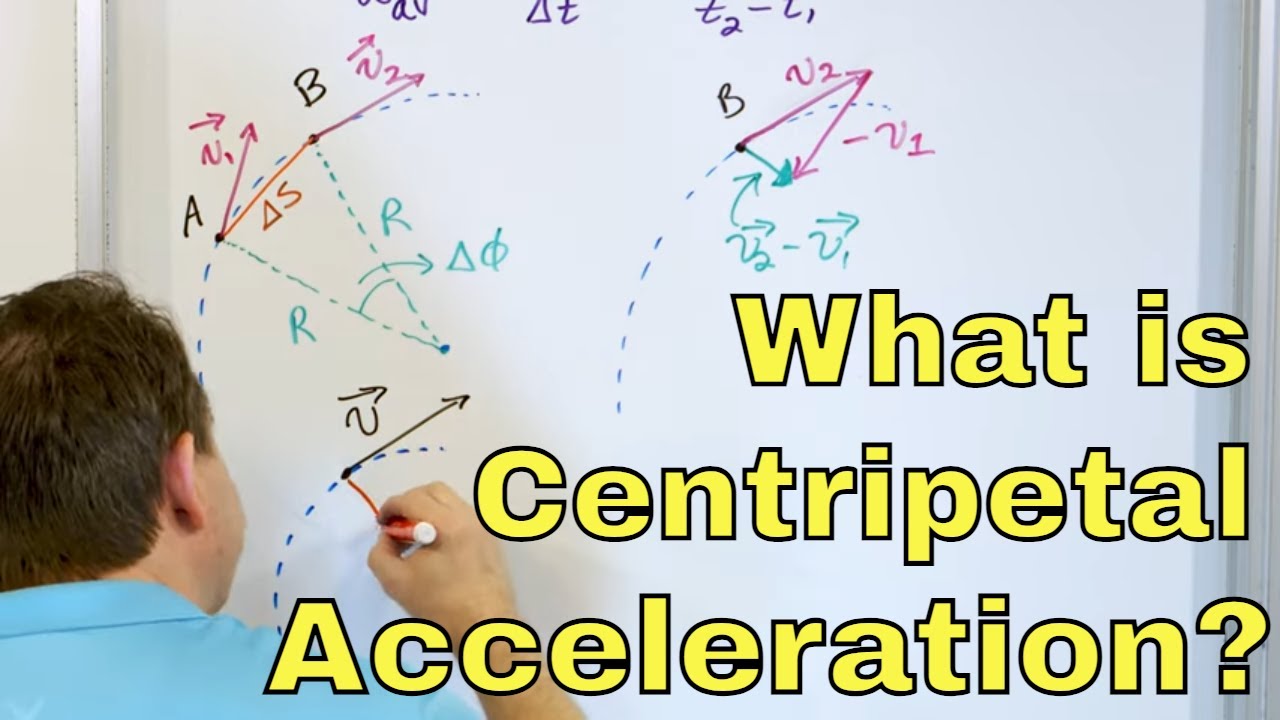
- 🌐 Centripetal acceleration is the acceleration that keeps an object moving in a circular path, directed towards the center of the circle.
- 📉 The formula for centripetal acceleration is speed squared divided by the radius, with units in meters per second squared.
- 🌟 Centripetal acceleration changes the direction of velocity, not its magnitude, while tangential acceleration does.
- 🔧 Centripetal forces are not a unique type of force but are any force directed towards the center of a circular path.
- 🌍 Newton's universal law of gravity states that every mass attracts every other mass with a force proportional to their masses and inversely proportional to the square of the distance between them.
- 🌌 Gravitational field is the acceleration due to gravity near an object, with the formula little G equals big G times mass divided by distance.
- 🚀 Gravitational orbits are determined by the gravitational force and can be calculated using centripetal acceleration principles.
Q & A
What is the definition of period in the context of this script?
-The period is the number of seconds it takes for a process to complete a full cycle, circle, or revolution.
How is frequency related to period?
-Frequency is the number of cycles, circles, or revolutions completed in one second. It is the inverse of the period, meaning the period is one over the frequency and the frequency is one over the period.
What is the unit of frequency and how is it derived?
-The unit of frequency is hertz, which comes from the relationship that frequency has units of one over second (1/s) because it is the number of cycles per second.
How can you relate speed, radius, and period in the motion of an object in a circular path?
-For an object in circular motion at a constant speed, the speed can be related to the radius and period by the formula where speed is equal to two pi times the radius (2πR) divided by the period.
What is centripetal acceleration and how is it directed?
-Centripetal acceleration is the acceleration that causes an object to move in a circular path. It is always directed toward the center of the circle.
What is the formula to calculate centripetal acceleration?
-The formula to calculate centripetal acceleration is speed squared (v^2) divided by the radius (R) of the circle the object is traveling in.
How does centripetal acceleration affect the velocity of an object?
-Centripetal acceleration does not cause the object to speed up or slow down. It only changes the direction of the velocity, not its magnitude.
What is the role of centripetal force in circular motion?
-Centripetal force is the force that keeps an object moving in a circular path. It is not a new type of force but any force that happens to be pointing towards the center of the circle.
How does Newton's universal law of gravity relate to the gravitational force between two masses?
-Newton's universal law of gravity states that all masses in the universe attract every other mass with a gravitational force that is proportional to the product of their masses and inversely proportional to the square of the distance between their centers.
What is the gravitational field and how is it represented?
-The gravitational field is the acceleration due to gravity near an object. It can be visualized as vectors pointing radially in toward a mass and is represented by the formula G = (G * M) / r^2, where G is the gravitational constant, M is the mass creating the field, and r is the distance from the center of the mass.
How can you calculate the gravitational acceleration on a planet with known density and radius?
-The gravitational acceleration on a planet can be calculated using the formula g = (G * ρ * 4/3 * π * R^3) / R^2, where G is the gravitational constant, ρ is the density of the planet, and R is the radius of the planet.
What is the relationship between the speed of an orbiting object and the mass and radius of the larger object it is orbiting?
-The speed of an orbiting object is related to the mass of the larger object it is orbiting and the distance between their centers by the formula v = √(G * M_larger / r), where G is the gravitational constant, M_larger is the mass of the larger object, and r is the center-to-center distance between the objects.
Outlines
📊 Understanding Period and Frequency
This paragraph introduces the concepts of period and frequency, which are fundamental in the study of periodic phenomena. The period is defined as the time taken for a complete cycle of a repeating process and is measured in seconds. Frequency, on the other hand, is the number of cycles completed in one second and is measured in hertz (Hz). The relationship between period and frequency is inversely proportional, meaning the period is one over the frequency and vice versa. The example provided involves an object moving in a circular path at a constant speed, where the relationship between speed, radius, and period of motion is discussed. The concept is further extended to discuss centripetal acceleration, which is the acceleration required for an object to move in a circular path, and the centripetal force that keeps an object in circular motion.
🔄 Centripetal Acceleration and Forces
This section delves deeper into the concept of centripetal acceleration, which is the acceleration that keeps an object moving in a circular path. The formula for centripetal acceleration is derived as the square of the speed divided by the radius of the circle. It is emphasized that centripetal acceleration only changes the direction of the velocity, not its magnitude. The paragraph also discusses the difference between centripetal and tangential acceleration, with the latter affecting the speed of the object. The example given compares the centripetal acceleration of two particles moving in circles with different speeds and radii, highlighting the relationship between their accelerations. Additionally, the concept of centripetal force is introduced, explaining that it is not a new type of force but rather an existing force directed towards the center of the circle.
🌍 Newton's Laws in Gravitational Context
This paragraph discusses the application of Newton's laws in the context of gravitational forces. Newton's universal law of gravity is introduced, stating that every mass attracts every other mass with a force proportional to the product of their masses and inversely proportional to the square of the distance between them. The example provided calculates the change in gravitational force when the mass and distance between two objects are altered. The concept of gravitational field is also explained as the acceleration due to gravity near an object, with its formula and vector nature discussed. An example problem involving the gravitational field of a hypothetical planet with Earth's density and radius is presented, showing how to calculate the acceleration due to gravity on this planet.
🚀 Gravitational Orbits and Space Stations
The final paragraph focuses on gravitational orbits, which occur when an object orbits another object due to gravitational force. The relationship between the speed of an orbiting object, the mass of the larger object, and the distance between them is derived using Newton's second law and centripetal acceleration. The example provided compares the speeds of two space stations orbiting at different altitudes above planets with different masses. The solution involves calculating the center to center distances and applying the derived formula to find the speed ratio between the two space stations, demonstrating the principles of gravitational orbits.
Mindmap
Keywords
💡Period
💡Frequency
💡Centripetal Acceleration
💡Centripetal Force
💡Newton's Universal Law of Gravity
💡Gravitational Field
💡Density
💡Gravitational Orbits
💡Vector
💡Newton's Second Law
💡Tangential Acceleration
Highlights
The period is the time taken for a process to complete a full cycle.
Frequency is the number of cycles completed in one second, measured in hertz.
Period and frequency are inversely related, with the formula: frequency = 1/period and period = 1/frequency.
An object in circular motion at constant speed has a period related to its speed and radius.
Centripetal acceleration is the acceleration that keeps an object moving in a circular path and always points toward the center of the circle.
The formula for centripetal acceleration is v^2/r, where v is the speed and r is the radius.
Centripetal acceleration changes the direction of velocity, not its magnitude.
The ratio of accelerations for two particles in circular motion depends on their speeds and radii.
Centripetal forces are not a new type of force but are existing forces directed towards the center of the circle.
Newton's universal law of gravity states that every mass attracts every other mass with a force proportional to their masses and inversely proportional to the square of the distance between them.
The gravitational field is the acceleration due to gravity near an object and is a vector quantity.
The gravitational field can be calculated using the formula G*M/R^2, where M is the mass of the object creating the field and R is the distance from the center of the mass.
In problems involving density, the mass of an object can be found by multiplying its density by its volume.
Gravitational orbits are a special case of centripetal acceleration where an object orbits another due to gravitational force.
The speed of an orbiting object is independent of its mass and depends on the mass of the larger object and the distance between the centers of the two objects.
In circular motion, the speed can be expressed as the product of the radius of the circle and the frequency of motion.
The gravitational force between two objects is a vector and has a direction that attracts each other mass.
The gravitational field strength is equivalent to the gravitational force exerted on a mass placed at a point in space, measured in newtons per kilogram.
Transcripts
Browse More Related Video
Centripetal Acceleration & Force - Circular Motion, Banked Curves, Static Friction, Physics Problems
Uniform Circular Motion - IB Physics
AP Physics 1 Circular Motion and Gravitation Review
Introduction to Centripetal Acceleration - Period, Frequency, & Linear Speed - Physics Problems

What Is Circular Motion? | Physics in Motion
What is Circular Motion & Centripetal Acceleration in Physics? - [1-4-14]
5.0 / 5 (0 votes)
Thanks for rating: