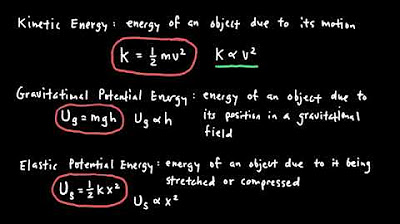What is Energy?
TLDRThe script explores the concept of energy in physics, highlighting its evolution from an intuitive idea to a powerful mathematical tool. It discusses the contributions of historical figures like Leibniz and du Chatelet in establishing energy conservation. The importance of energy as a measure of motion and potential, and its role in various forms like kinetic and potential energy, is emphasized. The script also touches on the broader implications of energy conservation in the universe, including its relationship with fundamental symmetries and the intriguing aspects of energy in an expanding universe.
Takeaways
- 🌟 Energy in physics is a quantity, not a tangible substance or mystical force, but a mathematical relationship between more fundamental quantities.
- 📈 The concept of energy was inspired by our intuitive sense of being energetic, leading to the discovery of powerful concepts in physics.
- 🔄 Gottfried Leibniz first formulated the mathematical form of kinetic energy, termed 'vis viva' or living force, which is conserved in systems without friction and perfect bounciness.
- 🏆 Emilie du Chatelet expanded the conservation of energy to include gravitational potential energy, putting it on equal footing with the conservation of momentum.
- 🔋 The law of conservation of energy states that energy can never be destroyed, only changed in form, and is a powerful tool in physics.
- 🎾 Energy conservation is demonstrated by the reversibility in the conversion between kinetic and potential energy, such as a ball bouncing without energy loss.
- 🚀 The concept of energy is crucial in understanding the behavior of the universe, as it allows us to predict outcomes and solve problems that would otherwise be impossible.
- 🌀 Energy calculations involve accounting for all forms of energy storage, including the effects of air resistance and friction, which are considered non-conservative forces.
- 📚 The conservation of energy is linked to fundamental symmetries in the universe, as discovered by Emmy Noether, and is not necessarily conserved on cosmic scales or in general relativity.
- 🌌 The universe's expansion leads to effects like dark energy and the accelerating expansion of the universe, where energy is not conserved on large scales.
- 📈 The concept of energy has evolved and is central to advanced mechanics and quantum mechanics, providing a versatile tool for describing complex systems and interactions.
Q & A
What is the concept of energy in physics?
-In physics, energy is not a substance or a mystical concept; it is a number or quantity that represents a mathematical relationship between other more fundamental quantities. It is a powerful tool for understanding and predicting the behavior of the physical universe.
Who first figured out the mathematical form of kinetic energy?
-17th century polymath Gottfried Leibniz was the first to figure out the mathematical form of what we now call kinetic energy, the energy of motion.
What did Leibniz call the early incarnation of energy?
-Leibniz called the early incarnation of energy 'vis viva', which translates to 'the living force'.
How does the law of conservation of energy relate to momentum?
-Leibniz's vis viva was seen as a competitor to Newton's idea of the conservation of momentum. While momentum was conserved, vis viva was not in the event of friction, leading to further development in the understanding of energy conservation.
Who demonstrated that energy is conserved and can never be destroyed?
-Emilie du Chatelet showed that vis viva, or energy, is conserved and can never be destroyed, only changed in form.
What is the significance of the law of conservation of energy?
-The law of conservation of energy is an incredibly powerful tool in physics. It states that energy is always conserved but only if you account for all types of energy, allowing for the prediction of the behavior of the universe in ways that would otherwise be impossible.
How does the concept of energy apply to a ball falling and bouncing?
-In the case of a ball falling and bouncing, gravitational potential energy gets converted to kinetic energy during the fall and then back to the same amount of potential energy during the rise, assuming no energy is lost to friction or air resistance.
What is a conservative force?
-A conservative force is one where the conversion between kinetic and potential energy is perfectly reversible, and every path taken between two points within the force field takes the same amount of work, the same shift between kinetic and potential energy.
How does energy calculation work in the presence of non-conservative forces?
-In the presence of non-conservative forces like friction or air resistance, energy calculations must account for the energy sapped from the system, which often results in the kinetic energy transfer to other forms of energy, such as heat.
What are the implications of the law of conservation of energy in the context of an expanding universe?
-In the context of an expanding universe, the law of conservation of energy does not hold on cosmic scales where expansion becomes significant. This leads to effects like dark energy and the accelerating expansion of the universe.
What is Noether's theorem and its significance in physics?
-Noether's theorem, discovered by mathematician Emmy Noether, states that for every symmetry in our universe, there exists a conserved quantity. This relationship between conservation laws and symmetries is fundamental to understanding the deeper properties of spacetime and the conservation of energy.
How has the concept of energy evolved beyond Newtonian mechanics?
-The concept of energy has led to new types of mechanics that have supplanted Newtonian mechanics for certain applications, such as Lagrange mechanics and Hamiltonian mechanics. These approaches use energy to describe the evolution of systems in ways that do not require tracking of numerous force vectors, and they have been adapted to quantum mechanics and high-energy particle physics.
Outlines
🌟 Understanding Energy in Physics
This paragraph delves into the concept of energy in physics, explaining that it is not a tangible substance but rather a measurable quantity representing a mathematical relationship between more fundamental quantities. It discusses the historical development of the concept of energy, from Gottfried Leibniz's introduction of kinetic energy (vis viva) to the conservation of energy as demonstrated by Emilie du Chatelet. The paragraph also explores the law of conservation of energy, emphasizing its power as a tool for understanding and predicting physical phenomena, and the importance of accounting for all types of energy in the system.
🔄 The Conservation and Transformation of Energy
This section focuses on the conservation and transformation of energy within a conservative force field, where every path between two points requires the same amount of work and energy is efficiently exchanged between kinetic and potential forms. It highlights the impact of non-conservative forces, such as friction and air resistance, on this energy exchange. The paragraph further discusses the fundamental forces being conservative when considering all particles involved and how energy calculations involve balancing the energy stored in various forms. It also touches on the broader implications of energy conservation in the universe and its role in the development of new mechanics frameworks like Lagrange and Hamiltonian mechanics.
🌌 The Cosmic and Quantum Implications of Energy
The final paragraph explores the deeper implications of energy conservation in the universe, linking it to the concept of symmetry and Noether's theorem. It ponders the effects of an expanding universe on energy conservation and introduces the idea that energy is not fundamental but rather a clue to more fundamental properties of spacetime. The paragraph concludes with a nod to the Great Courses Plus, a digital learning service that supports the video series and offers a wide range of educational content, including a course on physics and the universe, as a resource for further learning about these concepts.
Mindmap
Keywords
💡Potential Energy
💡Kinetic Energy
💡Conservation of Energy
💡Vis Viva
💡Emilie du Chatelet
💡Friction
💡Newtonian Mechanics
💡Lagrangian Mechanics
💡Hamiltonian Mechanics
💡Noether's Theorem
💡General Relativity
💡Quantum Mechanics
Highlights
The concept of energy in physics is not a substance but a number or quantity.
Energy is a mathematical relationship between other more fundamental quantities.
Gottfried Leibniz first figured out the mathematical form of kinetic energy.
Leibniz's concept of 'vis viva', the living force, is an early incarnation of energy.
Emilie du Chatelet showed that energy, or 'vis viva', is conserved and can never be destroyed, only changed in form.
The law of conservation of energy states that energy can only be changed in form, not destroyed.
James Prescott Joule and others extended the idea of energy to include heat energy.
The law of conservation of energy is a powerful tool in physics.
Gravitational potential energy is calculated as mass times gravitational acceleration times height.
The reversibility in the conversion between kinetic and potential energy is a key aspect of energy's power.
Energy is the currency of the trade between motion and the potential for motion.
Non-conservative forces, like friction or air resistance, can sap energy from a system.
All fundamental forces are conservative when considering all particles involved.
Energy calculations involve balancing the books and accounting for all forms of energy storage.
The concept of energy allows us to write equations describing the evolution of entire systems.
Energy conservation arises due to symmetry, particularly time translational symmetry.
Noether's theorem links every symmetry in the universe to a conserved quantity.
In an expanding universe, energy is not conserved on cosmic scales, leading to phenomena like dark energy.
Energy is not fundamental but a clue to the deeper, truly fundamental properties of spacetime.
Transcripts
5.0 / 5 (0 votes)
Thanks for rating: