3 Step Continuity Test, Discontinuity, Piecewise Functions & Limits | Calculus
TLDRThe video script explains the three-step continuity test to determine if a function is continuous at a specific point 'a'. It involves showing the function is defined at 'a', the limit of the function as x approaches 'a' exists and is equal from both sides, and finally, the limit equals the function's value at 'a'. The script uses piecewise functions as examples to illustrate how to apply the test, demonstrating continuity at x=2 and discontinuity at x=3, identifying it as a jump discontinuity. Additional examples explore removable and infinite discontinuities.
Takeaways
- 📌 The three-step continuity test is used to prove if a function is continuous at a certain point.
- 🔢 The first step is to confirm that the function is defined at the point 'a', meaning f(a) exists and has a specific value.
- 🌟 The second step involves showing that the limit as x approaches 'a' exists, which requires the left-sided limit to equal the right-sided limit.
- 🔄 The third step is to demonstrate that the limit of f(x) as x approaches 'a' from either side is equal to f(a).
- 🌰 An example function was given with different expressions for x < 2, 2 ≤ x ≤ 3, and x ≥ 3.
- 📈 At x = 2, the function was found continuous because the left and right limits both equaled the function's value at that point.
- 🚫 At x = 3, the function was discontinuous due to the left and right limits not matching, indicating a jump discontinuity.
- 🔍 For another example at x = -1, the function was found discontinuous with a removable hole because the left and right limits equaled but did not match the function's value at that point.
- ❗️ If the left and right limits are different, it typically indicates a jump discontinuity.
- 💡 If the left and right limits are the same but do not match the function's value at that point, it usually results in a removable discontinuity.
- 🌀 An infinite discontinuity only occurs if the limits themselves are equal to infinity.
Q & A
What is the first step in proving a function is continuous at a certain point?
-The first step is to show that the function is defined at that point, meaning f(a) must exist and equal a certain value.
How do you demonstrate the existence of a limit as x approaches a in the second step of the continuity test?
-You need to show that the left-sided limit (as x approaches a from the left) is equal to the right-sided limit (as x approaches a from the right).
What does the third step of the continuity test require you to prove?
-The third step requires proving that the limit as x approaches a from either side is equal to f(a).
What is the function f(x) at x equals two and three according to the given example?
-At x equals two, f(x) is x squared minus two, and at x equals three, f(x) is two x plus five.
Is the function continuous or discontinuous at x equals two in the provided example?
-The function is continuous at x equals two because the left and right limits both equal two, which is equal to f(2).
What type of discontinuity does the function have at x equals three?
-The function has a jump discontinuity at x equals three because the left and right limits do not match.
How does the value of f(x) change when x is equal to negative one in the second example?
-When x is equal to negative one, f(x) is five, regardless of the value of x being less than or equal to negative one.
Is the function continuous or discontinuous at x equals negative one in the second example?
-The function is discontinuous at x equals negative one because the limit as x approaches negative one does not equal f(negative one).
What type of discontinuity is present at x equals negative one in the second example?
-There is a removable discontinuity at x equals negative one because the limit exists but does not match the function's value at that point.
What is the difference between a jump discontinuity and a removable discontinuity?
-A jump discontinuity occurs when the left and right limits at a point are different and non-removable, while a removable discontinuity occurs when the limit exists but does not equal the function's value at that point, and it can be 'removed' by redefining the function at that point.
How can you identify an infinite discontinuity?
-An infinite discontinuity is identified when the left and right limits at a point both approach infinity, but the function's value at that point does not.
Outlines
📚 Understanding the Three-Step Continuity Test
This paragraph introduces the concept of the three-step continuity test for functions. It explains that to prove a function is continuous at a certain point 'a', one must first show the function is defined at 'a', meaning f(a) exists. The second step involves demonstrating that the limit of f(x) as x approaches 'a' exists, which requires the left-sided limit to equal the right-sided limit. The third step is to prove that the limit from both sides is equal to f(a). An example function is provided to illustrate applying the test at points x=2 and x=3, showing the function is continuous at 2 and discontinuous at 3 due to a jump discontinuity.
🔍 Applying the Continuity Test to Specific Functions
This paragraph continues the discussion on the continuity test, focusing on applying it to specific functions. It provides a detailed example where the function changes its definition based on the value of x. The example involves determining the continuity or discontinuity at x=-1. The function is found to be discontinuous at this point due to a removable discontinuity, as the left and right limits match but differ from the function's value at x=-1. The explanation also distinguishes between jump and removable discontinuities and mentions infinite discontinuities in the context of limits equaling infinity.
Mindmap
Keywords
💡Continuous Function
💡Three-Step Continuity Test
💡Limit
💡Piecewise Function
💡Jump Discontinuity
💡Removable Discontinuity
💡Existence of Limits
💡Square Root
💡X Approaching A
💡Function Value
💡Left-Sided and Right-Sided Limits
Highlights
The three-step continuity test for proving the continuity of a function at a certain point.
The first step is to show that the function is defined at the point 'a', meaning f(a) exists and has a certain value.
The second step involves demonstrating that the limit as x approaches 'a' of f(x) exists, which requires the left-sided limit to equal the right-sided limit.
The third step is to prove that the limit as x approaches 'a' from either side is equal to f(a).
Applying the three-step continuity test to a piecewise function defined differently at intervals of x < 2, 2 ≤ x ≤ 3, and x ≥ 3.
At x = 2, the function is continuous because the left-sided limit equals the right-sided limit, both being equal to f(2).
At x = 3, the function is discontinuous due to a mismatch between the left-sided and right-sided limits, indicating a jump discontinuity.
For the function defined differently at x < -1, -1 ≤ x ≤ 1, and x = 1, the continuity at x = -1 is analyzed.
At x = -1, the function is discontinuous with a removable hole because the limit exists but does not equal f(-1).
A jump discontinuity occurs when the left-sided and right-sided limits are different, and it is non-removable.
A removable discontinuity, or hole, exists when the left-sided and right-sided limits are the same but do not match the function's value at that point.
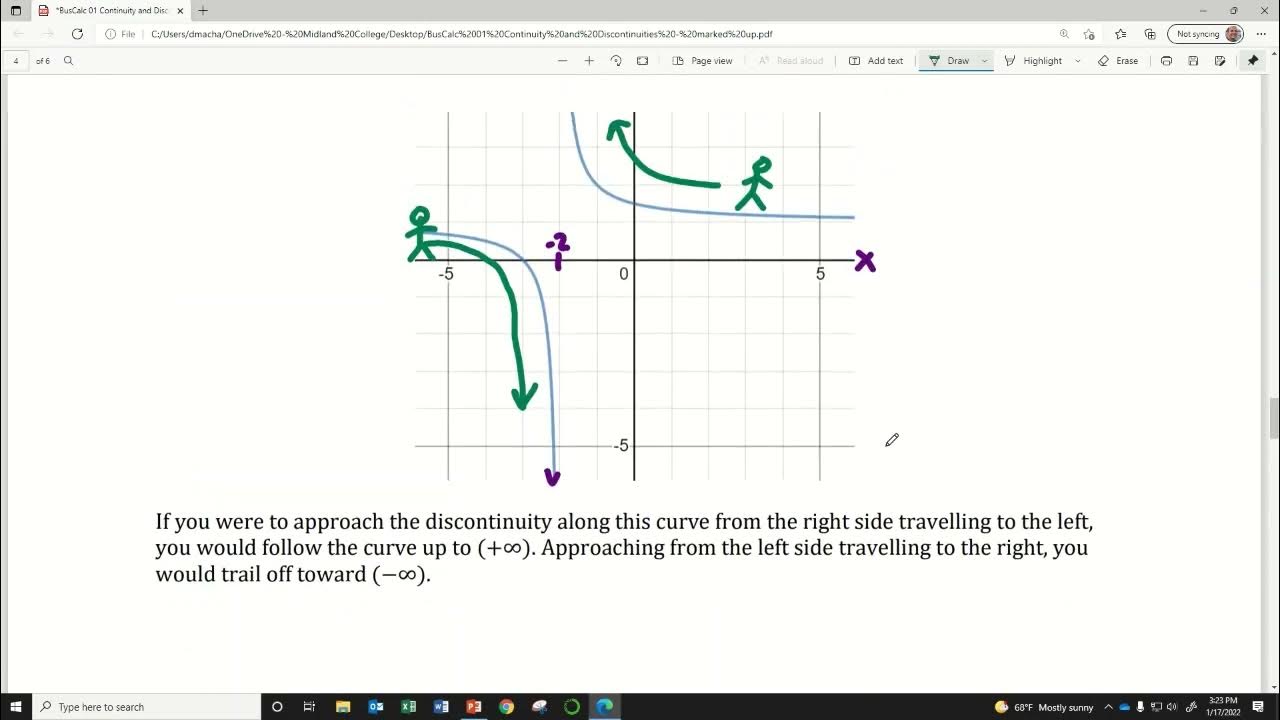
An infinite discontinuity is characterized by the limit values being infinity, which is not the case in the provided examples.
The importance of the three-step continuity test in determining the type of discontinuity: jump or removable hole.
The method for calculating the left-sided and right-sided limits to apply the three-step continuity test.
The significance of the function's definition at the point 'a' in the first step of the continuity test.
The role of the limit as x approaches 'a' from either side in the third step of the continuity test.
The practical application of the three-step continuity test to piecewise functions with different expressions in intervals.
The process of determining continuity or discontinuity at specific points using the three-step continuity test.
Transcripts
5.0 / 5 (0 votes)
Thanks for rating: