Physics - Ch 66 Ch 4 Quantum Mechanics: Schrodinger Eqn (27 of 92) Expectation Value=? 1-D Box n=1

Michel van Biezen
27 Nov 201706:09
EducationalLearning
32 Likes 10 Comments

TLDRThis lecture delves into the concept of expectation value for a particle in a one-dimensional box well, clarifying that it represents the average position rather than the probability of finding the particle at a specific location. The process of calculating the expectation value involves integrating the product of the particle's wave function and its position variable. For a particle in the ground state, the expectation value is found to be at the midpoint of the box (L/2), reflecting the symmetric probability distribution of the particle's position, indicating equal likelihood of it being to the left or right of the center.

Takeaways
  • πŸ“Œ The expectation value of a particle in a one-dimensional box well refers to its average position.
  • πŸ”„ To find the expectation value, integrate the product of the probability density function and the position variable (x) over the entire range of the box.
  • 🌐 The probability density function for a particle in a one-dimensional box is derived from the wave equation.
  • πŸ“ˆ The integral for the expectation value involves a combination of x squared, x, and the cosine of a multiple of the angle (2Ο€x/L).
  • 🎯 For the ground state (n=1), the integral simplifies due to specific wave function properties.
  • πŸŒ€ The integration constant 'a' in the general case is Ο€/L for a one-dimensional box.
  • πŸ“Š The integral results in a quadratic equation in terms of x, which simplifies after applying the limits of integration (0 to L).
  • πŸ”’ The final result of the expectation value calculation is L/2, indicating symmetry in the system.
  • 🌟 The value L/2 represents the central point of the box where the particle is equally likely to be found on either side.
  • πŸ” The symmetry of the wave function about the midpoint (L/2) confirms the expectation value's position.
  • πŸ’‘ This exercise demonstrates the application of quantum mechanics principles to understand the behavior of particles in defined potentials.
Q & A

  • What is the expectation value of a particle in a one-dimensional box well?

    -The expectation value refers to the average position of the particle within the box, which, for a particle in a one-dimensional box well, is found to be at the center of the box (L/2).

  • How is the expectation value different from the probability of finding a particle at a specific location?

    -The expectation value is the average position of the particle, considering its entire probability density function, not just the likelihood of finding the particle at any particular point in space.

  • What mathematical approach is used to calculate the expectation value of the particle's position?

    -The expectation value is calculated by integrating the product of the particle's wave function (probability density function) and its position variable (x) over the entire range of the box.

  • What is the ground state in the context of a one-dimensional box well?

    -The ground state refers to the lowest energy state of a particle in a one-dimensional box well, which is the state we're considering for this example.

  • How does the wave equation of a particle in a one-dimensional box well help in finding the expectation value?

    -The wave equation provides the probability density function, which is essential for calculating the expectation value through integration as it represents the distribution of the particle's presence within the box.

  • What is the significance of the symmetry of the probability density function in determining the expectation value?

    -The symmetry of the probability density function about the midpoint (L/2) of the box indicates that there is an equal probability of finding the particle on either side of this point, leading to an expectation value at the center (L/2).

  • What is the mathematical expression for the integral of x times the sine squared function?

    -The integral of x times the sine squared function (x * sin^2(a * x)) is (a/4) * (x^3 - 3 * x) / (4 * a^3), where 'a' is a constant.

  • Why does the term involving the sine function in the expectation value calculation evaluate to zero?

    -The term involving the sine function evaluates to zero because the sine of 2Ο€ (or any multiple of Ο€) is zero, which occurs at the upper and lower limits of integration (0 and L).

  • How do the terms involving the cosine function in the expectation value calculation simplify?

    -The terms involving the cosine function simplify because the cosine of 2Ο€ and the cosine of 0 are the same, leading to their cancellation when the limits of integration are applied.

  • What is the final result of the expectation value calculation for a particle in a one-dimensional box well?

    -The final result of the expectation value calculation is L/2, indicating that the average position of the particle is at the center of the box.

  • What does the expectation value of L/2 signify about the particle's distribution within the box?

    -The expectation value of L/2 signifies that there is an equal probability for the particle to be found on either side of the midpoint of the box, showing no preference for either side.

  • How does the expectation value relate to the particle's wave function and energy levels?

    -The expectation value is directly related to the particle's wave function, which in turn determines its energy levels. For a particle in a one-dimensional box well, the ground state wave function leads to an expectation value at the center of the box, reflecting the particle's lowest energy state.

Outlines
00:00
πŸ“ Understanding Expectation Value in a One-Dimensional Box

This paragraph introduces the concept of expectation value for a particle in a one-dimensional box well. It explains that the expectation value represents the average position of the particle, not the probability of finding the particle at a specific location or the most likely position. The lecturer uses the wave equation for a particle in a box to derive the probability density function and then applies an integral involving x times this function to find the expectation value. The calculation is demonstrated for the ground state (n=1), and the integral is worked out step by step, leading to the conclusion that the expectation value for the particle's position is at L/2, signifying an equal probability distribution across the box.

05:01
πŸ“Š Symmetry and Average Position in Quantum Mechanics

The second paragraph delves into the implications of the expectation value calculated in the previous section. It highlights the symmetry of the probability distribution graph about the midpoint (L/2) of the box, indicating that there is no preferred side for the particle's location. The average position being at L/2 is attributed to this perfect symmetry, where the probability of finding the particle on the left side of L/2 is equal to the probability of finding it on the right side. The expectation value, therefore, is precisely at the center of the box, demonstrating the principle of equal probability distribution in quantum mechanics.

Mindmap
Keywords
πŸ’‘Expectation Value
The expectation value is a fundamental concept in quantum mechanics that represents the average value of a physical quantity for a system. In the context of this video, it specifically refers to the average position of a particle within a one-dimensional box well. The expectation value is calculated by taking the integral of the product of the particle's position and its probability density function over the entire space. The video emphasizes that the expectation value does not describe the most probable location of the particle but rather the mean position based on the distribution of its probability density.
πŸ’‘One-Dimensional Box Well
A one-dimensional box well, also known as an infinite potential well or a particle in a box, is a theoretical model in quantum mechanics where a particle is confined within a one-dimensional space between two infinite potential barriers. This model is used to illustrate the quantization of energy levels and the wave-like behavior of particles. The video uses this model to explain how to calculate the expectation value of a particle's position within the box.
πŸ’‘Wave Equation
A wave equation is a mathematical equation that describes the evolution of a wave's shape and its propagation through space and time. In quantum mechanics, the wave equation is used to determine the wave function of a particle, which contains information about the probability distribution of the particle's position. The video uses the wave equation for a particle in a one-dimensional box well to derive the probability density function necessary for finding the expectation value.
πŸ’‘Probability Density Function
The probability density function (PDF) is a function that describes the distribution of probabilities for a particle's position in space. In the context of the video, the PDF is derived from the wave function of the particle in the one-dimensional box well. The integral of the PDF over all space gives the total probability, which must equal 1. The expectation value is found by integrating the product of the position and the PDF over all space.
πŸ’‘Integral
In mathematics, an integral represents the accumulation of a quantity over a range or region. In the context of this video, the integral is used to calculate the expectation value by summing up the product of the particle's position and its probability density function over the entire one-dimensional box. The integral is a key mathematical tool in quantum mechanics for determining expectation values and other physical quantities.
πŸ’‘Ground State
In quantum mechanics, the ground state is the lowest energy state of a quantum system. For the particle in a one-dimensional box well, the ground state corresponds to the lowest energy level that the particle can occupy. The video specifically calculates the expectation value for the ground state, which is the simplest and most fundamental state of the system.
πŸ’‘Quantum Number
Quantum numbers are integers or half-integers that describe the energy levels and other properties of particles in quantum systems. In the context of the one-dimensional box well, the quantum number (n) determines the energy level and the wave function of the particle. The video focuses on the ground state, which corresponds to n equals 1.
πŸ’‘Sine Squared
The sine squared function is a trigonometric function that represents the square of the sine of an angle. In the context of the video, the sine squared function is part of the wave function for the particle in the one-dimensional box well. It is used in the integral calculation to find the expectation value of the particle's position.
πŸ’‘Cosine Function
The cosine function is a trigonometric function that describes the ratio of the adjacent side to the hypotenuse in a right-angled triangle, or equivalently, the horizontal position of a point on a wave at a given time. In the video, the cosine function appears in the integral calculation for the expectation value, where it is used to account for the wave-like behavior of the particle's probability density function.
πŸ’‘Symmetry
Symmetry in this context refers to the balanced distribution of a physical quantity or property across a system. The video highlights the symmetry of the particle's probability density function about the midpoint of the box (L/2), which leads to the expectation value being at the center of the box. This symmetry implies that there is an equal likelihood of finding the particle at any position within the box, contributing to the average position at L/2.
πŸ’‘Quantization
Quantization is the process of restricting a physical quantity to discrete values or levels. In quantum mechanics, energy levels are quantized, meaning particles can only occupy certain allowed energy states. The one-dimensional box well model demonstrates this concept by showing that the particle's energy is quantized into different levels based on its wave function.
Highlights

The lecture explains how to find the expectation value of a single particle in a one-dimensional box well.

The expectation value is defined as the average position of the particle, not the probability of finding the particle at a specific location.

The expectation value is calculated using the integral of the product of the wave function and the position variable (x) over the entire range of possible positions.

For the ground state, the wave function of a particle in a one-dimensional box is used to find the probability density function.

The integral to find the expectation value involves the product of x and the sine squared of (Ο€x/L), where L is the length of the box.

The integral is solved by applying the general case formula for integrating x times the sine squared function.

In the specific case of a one-dimensional box, 'a' equals Ο€/L, simplifying the integral expression.

The limits of integration are from 0 to L, which corresponds to the length of the box.

The calculated expectation value for the average position of the particle is L/2, indicating symmetry about the center of the box.

The result L/2 demonstrates that there is an equal probability for the particle to be on either side of the midpoint of the box.

The expectation value being at L/2 signifies that the particle is equally likely to be found on the left or right side of the midpoint.

The symmetry of the wave function about the midpoint L/2 leads to the expectation value being at the center of the box.

The expectation value calculation provides insight into the behavior of particles in quantum systems.

The method used in the lecture can be applied to other quantum systems to understand their properties.

The lecture provides a clear example of how to apply mathematical techniques to quantum mechanics problems.

The integral calculus used in the calculation is a fundamental tool in quantum physics.

The expectation value calculation is a practical application of the principles of quantum mechanics.

The lecture illustrates the importance of understanding the wave function in determining the properties of quantum systems.

The expectation value is a key concept in quantum mechanics that describes the average behavior of particles.

The lecture demonstrates the step-by-step process of calculating the expectation value, making it accessible for learners.

Transcripts

Browse More Related Video

Physics - Ch 66 Ch 4 Quantum Mechanics: Schrodinger Eqn (25 of 92) Prob. of a Particle 1-D Box n=1

Physics - Ch 66 Ch 4 Quantum Mechanics: Schrodinger Eqn (29 of 92) Expect. Value Momentum=? 1-D Box

Physics - Ch 66 Ch 4 Quantum Mechanics: Schrodinger Eqn (22 of 92) Prob. of Finding Particle 2

Physics - Ch 66 Ch 4 Quantum Mechanics: Schrodinger Eqn (30 of 92) Momentum Eigenvalue=? n=1

21. Quantum Mechanics III

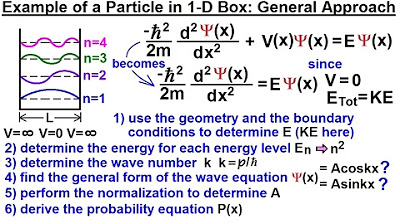
Physics - Ch 66 Ch 4 Quantum Mechanics: Schrodinger Eqn (18 of 92) Particle in 1-D Box: Gen. Appr.

Rate This

5.0 / 5 (0 votes)

Thanks for rating:

Related Tags
QuantumMechanicsExpectationValueParticlePhysicsOneDimensionalBoxProbabilityDensityWaveEquationSymmetryAnalysisGroundStateQuantumBehaviorPhysicsLecture