Physics - Ch 66 Ch 4 Quantum Mechanics: Schrodinger Eqn (30 of 92) Momentum Eigenvalue=? n=1

Michel van Biezen
30 Nov 201703:54
EducationalLearning
32 Likes 10 Comments

TLDRThis video delves into the concept of eigenvalues for momentum in the context of a particle within a one-dimensional box. It explains that while the expectation value of momentum is zero due to equal contributions from right and left-moving components, individual eigenvalues for momentum can be determined. These eigenvalues are given by Β±nΟ€Δ§/L, representing the magnitude of momentum for motion in either direction. The video clarifies that the average momentum, as expected, remains zero, aligning with the concept of eigenvalues in quantum mechanics.

Takeaways
  • πŸ“Œ Eigenvalues are values that represent the outcome of a measurement without depending on direction.
  • 🌟 The eigenvalues for momentum in a particle in a box are direction-independent and can be positive or negative.
  • πŸ“ˆ The momentum eigenvalues are derived from the energy levels of the particle in a one-dimensional box.
  • πŸ”’ The formula for momentum eigenvalues is ±√(2m(Ο€^2Δ§^2)/2ml^2), where m is the mass, l is the length of the box, and Δ§ is h-bar.
  • πŸŒ€ The expectation value of momentum for a particle in a box is zero due to equal probabilities of right and left motion.
  • πŸ”„ The average of the positive and negative momentum eigenvalues equals zero, confirming the expectation value.
  • 🎯 The positive eigenvalue represents momentum in the right direction, and the negative represents the left.
  • πŸ“ The individual momentum eigenvalues provide the magnitude of momentum for the particle moving to the right or left.
  • 🌊 The momentum eigenvalues are related to the energy levels and can be expressed in terms of the energy.
  • πŸ”§ The process of finding momentum eigenvalues involves plugging in the energy level, mass, box length, and h-bar into the momentum formula.
  • πŸ“Š The momentum eigenvalues are squared and then taken under the square root, simplifying the expression for analysis.
Q & A

  • What is the topic of the video?

    -The topic of the video is finding the eigenvalues of momentum for a particle in a one-dimensional box.

  • How are the eigenvalues related to the momentum of a particle in a box?

    -Eigenvalues represent the possible values for the momentum of a particle in a box that do not depend on direction.

  • What is the significance of the expectation value for momentum being zero?

    -The expectation value for momentum being zero indicates that on average, the momentum to the right and to the left cancel each other out, which is the case for a particle in a one-dimensional box.

  • What is the expression for calculating the eigenvalues of momentum?

    -The eigenvalues of momentum are given by Β±(nΟ€Δ§)/L, where n is the level, Δ§ is the reduced Planck constant, and L is the length of the box.

  • What do the plus and minus signs in the eigenvalues of momentum represent?

    -The plus sign represents the momentum for motion to the right, while the minus sign represents the momentum for motion to the left.

  • How does the average momentum of a particle in a one-dimensional box compare to the expectation value?

    -The average momentum is equal to the expectation value, which is zero, as the positive and negative momenta cancel each other out.

  • What is the implication of the eigenvalues for the energy levels in a one-dimensional box?

    -The eigenvalues for momentum are directly related to the energy levels, as both are quantized and depend on the same factors such as the level, mass, length of the box, and Δ§.

  • Why is the momentum in a one-dimensional box direction independent?

    -The momentum is direction independent because the particle is confined to move within the box, and the average momentum to the right and left over time is zero.

  • How does the script relate the concept of eigenvalues to the physical scenario of a particle in a box?

    -The script demonstrates that the eigenvalues of momentum can be calculated for a particle in a box and that these values provide insight into the particle's behavior, specifically its momentum in the context of the box's constraints.

  • What is the role of the reduced Planck constant (Δ§) in the expression for momentum eigenvalues?

    -The reduced Planck constant (Δ§) is a fundamental constant in quantum mechanics that relates the momentum eigenvalues to the energy levels and the physical dimensions of the box.

  • How does the length of the box (L) affect the eigenvalues of momentum?

    -The length of the box (L) is inversely proportional to the magnitude of the momentum eigenvalues, meaning that as the box size increases, the possible momentum values decrease.

Outlines
00:00
πŸ“ Quantum Mechanics: Finding Eigenvalues of Momentum in a Particle in a Box

This paragraph introduces the topic of finding the eigenvalues of momentum for a particle confined within a one-dimensional box. It explains that eigenvalues are the values we seek that do not depend on direction, indicating they are direction-independent. The discussion revolves around the concept that the expectation value for momentum is zero due to the equal and opposite momenta canceling each other out. The calculation for the eigenvalues of momentum is described, leading to the expression involving the square root of 2m, the particle's mass, Planck's reduced constant (h-bar), and the box's length (L). The paragraph concludes by highlighting that the average value of the two eigenvalues (positive and negative) is zero, which aligns with the expectation value for momentum.

Mindmap
Keywords
πŸ’‘Eigenvalues
Eigenvalues are the special values of a mathematical function or operator that do not change the function's properties. In the context of this video, eigenvalues refer to the specific values of momentum associated with different energy levels of a particle in a one-dimensional box. These eigenvalues are direction-independent, meaning they represent the magnitude of momentum without considering the direction of motion.
πŸ’‘Momentum
Momentum is a fundamental concept in physics that represents the quantity of motion of an object. It is the product of an object's mass and its velocity. In the video, the focus is on the momentum of a particle confined within a one-dimensional box, where the momentum is related to the energy levels and is found to have two possible values, positive and negative, representing motion to the right and left, respectively.
πŸ’‘Particle in a Box
A particle in a box is a theoretical model in quantum mechanics that describes a particle, often an electron, confined within a one-dimensional box or potential well with impenetrable walls. The video uses this model to explore the quantization of energy levels and momentum for the confined particle, highlighting the discrete nature of these properties in the quantum realm.
πŸ’‘Energy Levels
Energy levels are the quantized, discrete values that energy can take within a quantum system. In the context of the video, energy levels refer to the different allowed energy states a particle can occupy within a one-dimensional box. These levels are determined by the wave-like properties of the particle and the boundaries of the box.
πŸ’‘Expectation Value
The expectation value is a statistical measure that represents the average value of a random variable or quantum observable. In quantum mechanics, it is used to calculate the average outcome of a measurement for a quantum state. In the video, the expectation value for momentum is found to be zero, indicating that on average, the particle's momentum in the box is equally distributed in both positive and negative directions.
πŸ’‘Quantum Mechanics
Quantum mechanics is a fundamental theory in physics that describes the behavior of matter and energy at the atomic and subatomic scales. It introduces concepts such as quantization of energy and wave-particle duality, which are essential for understanding the behavior of particles in a one-dimensional box as discussed in the video.
πŸ’‘One-Dimensional Box
A one-dimensional box, in the context of quantum mechanics, refers to an idealized model where a particle is confined to move only along a straight line, with the space restricted between two impenetrable walls. This model is used to illustrate the principles of quantization and wave-like behavior of particles at the quantum level.
πŸ’‘Reduced Planck Constant (H bar)
The reduced Planck constant, denoted as H bar, is a fundamental constant in quantum mechanics that relates the energy of a photon to its frequency. It is used in the equations describing quantum systems, such as the one-dimensional box model, to calculate energy levels and other properties like momentum.
πŸ’‘Direction Independence
Direction independence refers to a property of certain physical quantities that means their values do not depend on the direction of measurement. In the video, the eigenvalues of momentum for a particle in a one-dimensional box are direction-independent, meaning the magnitude of momentum is the same regardless of whether the particle is moving to the right or to the left.
πŸ’‘Wave Function
A wave function is a mathematical description in quantum mechanics that provides information about the probability amplitude of a particle's location or other physical properties. It is essential for calculating expectation values and understanding the behavior of particles in quantum systems like the one-dimensional box.
πŸ’‘Quantization
Quantization is the process of restricting physical quantities to discrete values, as opposed to continuous ones. In quantum mechanics, energy, momentum, and other properties are quantized, meaning they can only take on certain specific values. The video demonstrates quantization by showing that the momentum and energy of a particle in a one-dimensional box can only have certain eigenvalues.
Highlights

The video discusses finding the eigenvalues of momentum for a particle in a one-dimensional box.

The energy levels in the previous video were expressed with a specific mathematical expression.

The momentum for the energy levels can be expressed in terms of energy.

Eigenvalues are values that do not depend on direction, making them direction independent.

The expectation value for momentum is equal to zero due to the averaging of right and left momenta.

The individual values for right and left momentum can be found and compared to the expectation value.

The formula for momentum involves the level, mass, length of the box, and h-bar.

The twos and Ms in the formula cancel out, simplifying the expression.

The momentum eigenvalues are given by n PI H bar over L, with positive and negative values representing right and left motion.

The average value of the eigenvalues results in zero, which is the expectation value for momentum.

The eigenvalues provide the magnitude of momentum for a particle in a one-dimensional box moving to the right or left.

The process of finding the eigenvalues involves plugging in the given values into the momentum formula.

The video demonstrates a straightforward method for calculating momentum eigenvalues.

The theoretical approach to finding momentum eigenvalues is explained in a clear and accessible manner.

The video provides a comprehensive understanding of the concept of eigenvalues in quantum mechanics.

The practical application of the eigenvalue concept is shown in the context of a particle in a one-dimensional box.

The video is an educational resource for those interested in quantum mechanics and eigenvalue calculations.

Transcripts

Browse More Related Video

Physics - Ch 66 Ch 4 Quantum Mechanics: Schrodinger Eqn (29 of 92) Expect. Value Momentum=? 1-D Box

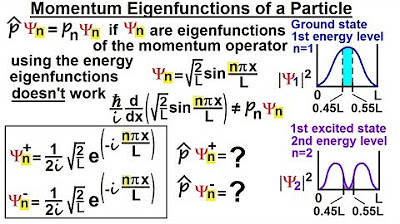
Physics - Ch 66 Ch 4 Quantum Mechanics: Schrodinger Eqn (31 of 92) Momentum Eigenfunction Particle

Lecture 16: Eigenstates of the Angular Momentum Part 2

Ch 7: How are observables operators? | Maths of Quantum Mechanics

26.1 Integral Equations for Quantum Bound States

EASIEST question on the International Physics Olympiad?

Rate This

5.0 / 5 (0 votes)

Thanks for rating:

Related Tags
Quantum MechanicsEigenvaluesMomentumParticle in a BoxPhysics EducationDirection IndependenceZero MomentumQuantum StatesWave FunctionsEducational Video