Physics - Ch 66 Ch 4 Quantum Mechanics: Schrodinger Eqn (21 of 72) Prob. of Finding Particle 1

Michel van Biezen
19 Feb 201707:13
EducationalLearning
32 Likes 10 Comments

TLDRThe video script discusses the calculation of the probability of finding a particle in a one-dimensional infinite well within a specific range. It explains the process of squaring the wave function, integrating over the interval from 0 to 1/4 of the well's length, and applying the sine squared identity to simplify the integral. The result shows that there's approximately a 10% chance of finding the particle in the first and third quarters of the well, and about 80% in the middle half. This provides a clear understanding of the particle's likely locations within the well.

Takeaways
  • ๐ŸŒŸ The video discusses calculating the probability of finding a particle in a one-dimensional infinite well within a specific range.
  • ๐Ÿ“ The wave equation derived from the general concept is used to determine the probability by squaring the function and integrating over the interval of interest.
  • ๐Ÿ”ข The probability is found by integrating the squared wave function from x=0 to x=L/4, where L is the width of the well.
  • ๐ŸŒ€ The wave function for a particle in a one-dimensional well is sine squared in form, involving the variable nฯ€x/L.
  • ๐Ÿ“Œ The integration process simplifies by taking constants outside the integral and using the trigonometric identity for sine squared.
  • ๐Ÿงฎ The integral of sine squared is transformed into an integral of (1 - cos(2nฯ€x/L)) and then split into two parts for easier computation.
  • ๐Ÿ The first integral evaluates to L/4, and the second integral involves the cosine function, which simplifies the process.
  • ๐Ÿ“Š The final result of the probability calculation is 0.0908, or approximately 10%, for finding the particle in the first quarter of the well.
  • ๐Ÿ”„ Due to symmetry, a similar 10% probability is expected for the particle to be found in the last quarter of the well.
  • ๐Ÿ”ด The highest probability, around 80%, is calculated for finding the particle in the middle half of the well (from L/4 to 3L/4).
  • ๐ŸŽฒ The problem demonstrates the application of wave functions, integration, and trigonometric identities in quantum mechanics.
Q & A

  • What is the main topic of the video?

    -The main topic of the video is calculating the probability of finding a particle in a specific portion of a one-dimensional infinite well.

  • What is the equation derived for the particle in a one-dimensional well?

    -The wave equation derived for the particle in a one-dimensional well is based on the general concept of what the wave equation should look like, and it is used to find the probability of the particle's location.

  • How is the probability of finding a particle calculated?

    -The probability is calculated by squaring the wave function (since there's no imaginary part) and integrating it over the interval of interest, which in this case is from x=0 to x=L/4.

  • What is the significance of the sine squared function in the calculation?

    -The sine squared function represents the squared wave function, which is used to calculate the probability density of the particle within the well.

  • How is the integral of the sine squared function evaluated?

    -The integral of the sine squared function is evaluated using the trigonometric identity, which transforms it into an integral of (1 - cos(2nฯ€x/L)) over the interval from 0 to L/4.

  • What is the role of the integral in determining the probability?

    -The integral is used to sum up the probabilities over the interval from 0 to L/4, which gives the total probability of finding the particle within that interval.

  • Why is the constant factor 2/L taken outside the integral?

    -The constant factor 2/L is taken outside the integral because it does not depend on the variable of integration (x), and this simplifies the integral expression.

  • What is the final probability of finding the particle in the first quarter of the well?

    -The final probability of finding the particle in the first quarter of the well is approximately 10%.

  • How does symmetry contribute to the probability distribution in the well?

    -Due to symmetry, the probability of finding the particle is about 10% in the first and third quarters, and about 80% in the middle half of the well.

  • What is the significance of the calculated probabilities for quantum particles in an infinite well?

    -The calculated probabilities provide insights into the behavior of quantum particles within a one-dimensional infinite well, which is fundamental for understanding quantum mechanics and the properties of quantum systems.

  • How can the results from this video be applied in practical scenarios?

    -The results can be applied in various areas of quantum physics, such as understanding the behavior of electrons in quantum dots or designing nanoscale electronic devices.

Outlines
00:00
๐ŸŒŸ Quantum Mechanics: Probability Calculation in an Infinite Well

This paragraph delves into the quantum mechanics concept of calculating the probability of finding a particle within a specific region of a one-dimensional infinite well. The discussion begins with a recap of previous videos on the particle's motion equation and the probability of finding it in an infinite well. The focus then shifts to the actual calculation of the probability for a particle to be found between x=0 and x=1/4L away from the left side of the well. The wave equation derived for the particle is used, and the process involves squaring the function, integrating over the interval of interest, and applying the sine squared function. The integration is carried out using the trigonometric identity, leading to a final expression that can be evaluated to find the probability.

05:02
๐Ÿ“ˆ Probability Distribution in a Quantum Well: Interpreting the Results

The second paragraph continues the quantum mechanics discussion by interpreting the calculated probability distribution of a particle in a one-dimensional well. The calculation leads to a result that indicates a nearly 10% chance of finding the particle in the first quarter of the well (from 0 to L/4). Due to the symmetry of the system, a similar 10% probability is expected in the last quarter. The remaining 80% probability is associated with finding the particle in the middle half of the well (from L/4 to 3L/4). The arithmetic is worked out to provide a clear understanding of the probability distribution, which is a fundamental aspect of quantum behavior in such systems.

Mindmap
Keywords
๐Ÿ’กElectron Line
The term 'Electron Line' likely refers to the series of videos that the transcript is a part of, focusing on quantum mechanics and electron behavior. It is the central theme around which the educational content is structured, aiming to teach viewers about the motion of particles and wave functions.
๐Ÿ’กWave Equation
A wave equation is a mathematical representation that describes the behavior of waves, such as the propagation of particles in quantum mechanics. In the context of the video, it is used to model the probability of finding a particle in a one-dimensional well, which is a fundamental concept in understanding quantum states.
๐Ÿ’กProbability
In quantum mechanics, probability is used to describe the likelihood of finding a particle in a particular state or location. It is a statistical measure that reflects the uncertainty principle, where the exact position and momentum of a particle cannot be known simultaneously.
๐Ÿ’กOne-Dimensional Infinite Well
A one-dimensional infinite well, also known as a particle in a box, is a thought experiment in quantum mechanics where a particle is confined to move within an infinite potential well along a single dimension. This model helps in understanding the quantization of energy levels and wave functions.
๐Ÿ’กWave Function
A wave function is a mathematical function that describes the quantum state of a particle or system. It contains all the information about the system and is used to calculate probabilities of various outcomes. In the video, the wave function is squared to find the probability distribution of the particle.
๐Ÿ’กSine Squared
The sine squared function is a trigonometric function that represents the square of the sine function. In the context of the video, it is used in the integration process to find the probability distribution of the particle. The sine squared term arises from the square of the wave function in the one-dimensional well.
๐Ÿ’กTrigonometric Identity
A trigonometric identity is a relationship between trigonometric functions that is always true. In the video, the 'traumatic identity' (likely a typo for 'trigonometric identity') is used to simplify the integration of the sine squared function, which is a common technique in solving physics and engineering problems.
๐Ÿ’กIntegration
Integration is a mathematical process that finds the area under a curve or calculates the accumulated quantity over a given interval. In the video, integration is used to determine the probability of finding a particle within a specific range in the one-dimensional well.
๐Ÿ’กCosine Function
The cosine function is a trigonometric function that describes periodic changes in a wave. In the context of the video, the cosine function is used in the integration process after applying the trigonometric identity, to help simplify the calculation of the probability distribution.
๐Ÿ’กQuantum States
Quantum states are the various conditions that a quantum system can be in, each characterized by a unique wave function. These states determine the probabilities of different outcomes when measurements are made, such as the position of a particle in the one-dimensional well discussed in the video.
๐Ÿ’กSymmetry
Symmetry in physics often refers to the balanced or mirror-like properties of a system. In the context of the video, symmetry is implied in the probability distribution of the particle's location, suggesting that the likelihood of finding the particle is evenly distributed across symmetrical sections of the well.
Highlights

Calculating the probability of finding a particle in a one-dimensional infinite well

Using the derived wave equation for the particle in the well

Squaring the wave function to find probability, due to the absence of an imaginary part

Integrating the squared wave function over the interval from 0 to 1/4 of L

Applying the integral of sine squared using the trigonometric identity

Integrating the expression to separate it into two parts

Evaluating the first integral to get 1/4

Evaluating the second integral involving the cosine function

Calculating the probability as 1/4 - (1/2 * pi) / (2 * pi * n)

Interpreting the result as approximately 10% probability of finding the particle in the first quarter of the well

Due to symmetry, a similar 10% probability is expected in the third quarter

An 80% probability of finding the particle in the middle half of the well

The method demonstrates a practical application of wave equations in quantum mechanics

The process illustrates the mathematical approach to solving quantum mechanical problems

The use of trigonometric identities simplifies complex integrals in physics

The video provides a clear step-by-step explanation of the calculation

The results have direct implications for understanding particle behavior in quantum wells

The explanation is accessible, making complex quantum mechanics concepts understandable

Transcripts

Browse More Related Video

Physics - Ch 66 Ch 4 Quantum Mechanics: Schrodinger Eqn (22 of 92) Prob. of Finding Particle 2

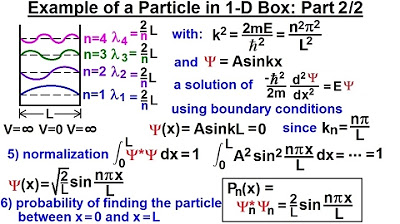
Physics - Ch 66 Ch 4 Quantum Mechanics: Schrodinger Eqn (20 of 92) Particle in 1-D Box: Example 2/2

Physics - Ch 66 Ch 4 Quantum Mechanics: Schrodinger Eqn (10 of 92) What is Normalization? Ex. 1

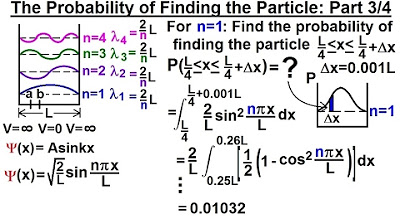
Physics - Ch 66 Ch 4 Quantum Mechanics: Schrodinger Eqn (23 of 92) Prob. of Finding Particle 3

Physics - Ch 66 Ch 4 Quantum Mechanics: Schrodinger Eqn (27 of 92) Expectation Value=? 1-D Box n=1

Physics - Ch 66 Ch 4 Quantum Mechanics: Schrodinger Eqn (25 of 92) Prob. of a Particle 1-D Box n=1

Rate This

5.0 / 5 (0 votes)

Thanks for rating:

Related Tags
Quantum MechanicsWave EquationProbability CalculationOne-Dimensional WellParticle MotionSine Squared FunctionIntegrationTrigonometric IdentityPhysics EducationMathematical Analysis