Using Substitutions to Solve Equations (Precalculus - College Algebra 23)
TLDRThis video script delves into the technique of substitution to solve non-quadratic equations by transforming them into quadratic form. The process involves identifying a common factor in the equation that can be raised to the power of two in one term and to the power of one in another, thus allowing the substitution of a new variable. The video provides step-by-step examples, illustrating how to perform the substitution, factor the resulting quadratic equation, and then revert back to the original variable to find the solutions. It emphasizes the importance of careful checking when dealing with square roots and negative exponents to avoid false solutions. The script serves as a comprehensive guide for those looking to master the substitution method for solving a wider range of polynomial equations.
Takeaways
- 📚 Substitution is a technique used to solve non-quadratic equations by transforming them into a quadratic form that is easier to factor and solve.
- ✅ The substitution method is particularly useful when the equation has a term that can be squared and another term with the same base raised to the first power.
- 🔍 To apply substitution, look for an expression common to two terms in the equation, and replace it with a new variable, often represented as 'u' or 'y'.
- 🧩 After transforming the equation into a quadratic using substitution, solve it for the new variable. Then, use the original substitution to revert back to the original variable.
- ❗ Be cautious when dealing with square roots or fractional exponents, as squaring both sides of an equation can introduce non-real or extraneous solutions.
- 🤔 If the quadratic equation is not factorable, use the quadratic formula to find the solutions in terms of the substitution variable.
- 🔢 When reverting back to the original variable, be mindful of the domain of the original function to avoid including invalid or complex solutions.
- ✋ Remember that the substitution method is not limited to simple quadratics and can be extended to more complex equations, including those with radicals or higher-degree polynomials.
- 📉 The method can also be used to find y-intercepts instead of x-intercepts, which requires reciprocation and potentially rationalizing the denominator.
- 📈 Practice is key to mastering the substitution method, especially for more complex equations that may not factor easily.
- 📝 Always show your work and maintain a clear trail of substitutions to ensure the process is understandable and reversible.
Q & A
What is the main topic discussed in the video?
-The video discusses how to solve non-quadratic equations using quadratic techniques by employing a method called substitution.
What is the purpose of making a substitution in solving equations?
-The purpose of making a substitution is to transform a non-quadratic equation into a simpler quadratic form that is easier to factor and solve.
What is a common mistake students make when using substitution with variables other than X?
-A common mistake is to forget that the substituted variable represents the original expression and to incorrectly solve for X by substituting the variable back into the original equation without considering the original form.
How does the substitution method help in finding the roots of a polynomial?
-The substitution method helps in finding the roots of a polynomial by simplifying the polynomial into a quadratic form, which is easier to factor and from which the roots can be found more readily.
What is the significance of the first term's exponent being twice the second term's exponent in the substitution method?
-The significance is that it allows the first term to be written as the square of the second term, which is a requirement for the substitution method to work effectively in transforming the equation into a quadratic form.
Why is it important to check the solutions found using substitution against the original equation?
-It is important to check the solutions against the original equation to ensure that the substitution process was done correctly and that the solutions obtained are valid for the original non-quadratic equation.
What is the role of the quadratic formula in solving equations that are not easily factorable?
-The quadratic formula can be used to find the solutions for equations that are not easily factorable, especially when the substitution method has transformed the equation into a standard quadratic form.
How does the substitution method handle complex solutions?
-The substitution method can still yield complex solutions when the factored form of the quadratic equation results in imaginary numbers. These complex solutions are important in a mathematical context but may not correspond to real-world x-intercepts.
What is the 'breadcrumbs' analogy used to describe in the video?
-The 'breadcrumbs' analogy is used to describe the process of using substitution to guide you through the problem, just as Hansel and Gretel used breadcrumbs to find their way through the forest. It helps to remember the path taken to return to the original variable after substitution.
Why is it necessary to be cautious when squaring both sides of an equation involving square roots?
-Squaring both sides of an equation involving square roots can lead to false solutions if the square root originally represented a negative number, as the square root of a real number cannot be negative.
How does the video script guide the viewer through the process of solving a cubic equation using substitution?
-The video script guides the viewer through identifying when a substitution is possible (when the first term's exponent is twice the second term's), making the substitution, factoring the quadratic form, solving for the substituted variable, and then transforming the solutions back into terms of the original variable.
Outlines
😀 Introduction to Quadratic Substitution
This paragraph introduces the concept of solving non-quadratic equations using quadratic techniques through a method called substitution. The focus is on identifying factorable expressions that can be transformed into a quadratic form by substituting a variable, thus simplifying the process of finding solutions.
🧐 Common Pitfalls and Solutions with Substitution
The second paragraph discusses common mistakes made by students when using substitution, particularly the tendency to work with the substituted variable rather than converting back to the original variable, x. It emphasizes the importance of recognizing when to revert from the substituted variable to x to find the actual solutions to the equation.
🔍 Identifying When Substitution is Applicable
This part of the script explains how to determine if a substitution will be effective. It involves checking if the first exponent is twice the second, allowing the equation to be rewritten in a form that resembles a quadratic, which can then be factored or solved more easily.
🤔 Dealing with Complex Solutions and Roots
The fourth paragraph delves into the process of finding x-intercepts for polynomials of higher degrees, which may result in complex solutions. It covers how to handle situations where the substitution leads to complex numbers and the importance of distinguishing between real and complex solutions when finding x-intercepts.
📚 Advanced Substitution with Fractional Exponents
This section illustrates a more advanced use of substitution with fractional exponents, transforming them into radical expressions and then applying the substitution method. It also addresses the potential complexity that can arise when solutions involve square roots within square roots.
🚧 Cautions When Squaring and Unsquaring Equations
The sixth paragraph warns about the potential pitfalls of squaring both sides of an equation, especially when dealing with square roots and negative values. It emphasizes the need for careful consideration to avoid introducing false solutions based on invalid mathematical operations.
🔢 Techniques for Solving Cubic Equations
The seventh paragraph discusses the approach to solving cubic equations using substitution. It contrasts the process with that of quadratic equations and highlights that cube roots can accept negative values, unlike square roots, which presents a key difference in solving the equations.
🛑 Special Considerations for Negative Exponents
The eighth paragraph focuses on the special considerations required when dealing with negative exponents in the context of substitutions. It explains how to transform the equation to make it quadratic and the importance of ensuring the first term's exponent is twice that of the second.
🔁 The Process of Substitution and Re-substitution
The final paragraph summarizes the process of making a substitution to transform a non-quadratic equation into a quadratic form, solving it, and then reversing the substitution to find the original variable's values. It also touches on the potential need for rationalizing denominators when the substitution results in complex fractions.
Mindmap
Keywords
💡Substitution
💡Quadratic
💡Factorable
💡Exponents
💡Polynomial
💡X-intercepts
💡Radicals
💡Complex Solutions
💡Real Solutions
💡Quadratic Formula
💡Reciprocals
Highlights
The video discusses solving non-quadratic equations using quadratic techniques through a method called substitution.
Substitution is typically applied when the equation has a factorable nature but does not immediately appear factorable.
A simple substitution can transform a non-quadratic equation into a quadratic form, allowing for easier factoring.
The substitution method involves setting the equation equal to zero and looking for a pattern where the first term is the second term raised to the power of two.
The substitution variable, often denoted as 'u', should not be the variable already in use (e.g., 'x') to avoid confusion.
After transforming the equation into a quadratic form and solving for 'u', the original variable 'x' can be found by reversing the substitution.
The video uses the analogy of Hansel and Gretel's breadcrumbs to describe the process of substitution and reverting back to the original variable.
A common mistake is to forget that the substitution variable 'u' represents an expression in terms of 'x', not just 'x' itself.
The video demonstrates how to handle equations that may not factor easily, using the quadratic formula and dealing with complex or imaginary solutions.
For polynomials with real coefficients, the fundamental theorem of algebra ensures that there will be as many roots as the degree of the polynomial, including complex roots.
When applying the substitution method to cubic or higher degree polynomials, it's important to remember that the first term's exponent must be twice the second term's exponent.
The video provides a detailed example of solving a quartic function using substitution to find both real and complex x-intercepts.
The presenter cautions against automatically squaring both sides of an equation when dealing with square roots, as it can lead to invalid real-number solutions.
The video concludes with a reminder that the substitution technique is powerful and versatile, applicable to a range of polynomial equations beyond simple quadratics.
A comprehensive understanding of exponents, radicals, and the domain of real numbers is crucial for successfully applying the substitution method.
The video emphasizes the importance of checking work and being mindful of the mathematical rules when manipulating equations, especially with square roots and negatives.
The presenter provides a step-by-step guide on how to handle complex solutions and how to determine which are valid x-intercepts for the given function.
The video also addresses the potential need to rationalize denominators when dealing with solutions involving square roots, to ensure they are expressed in a simplified form.
Transcripts
Browse More Related Video
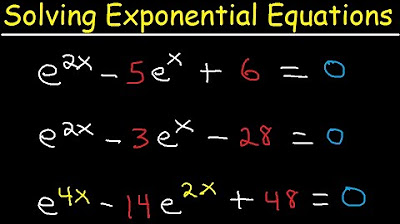
Solving Exponential Equations In Quadratic Form - Using Logarithms, With e
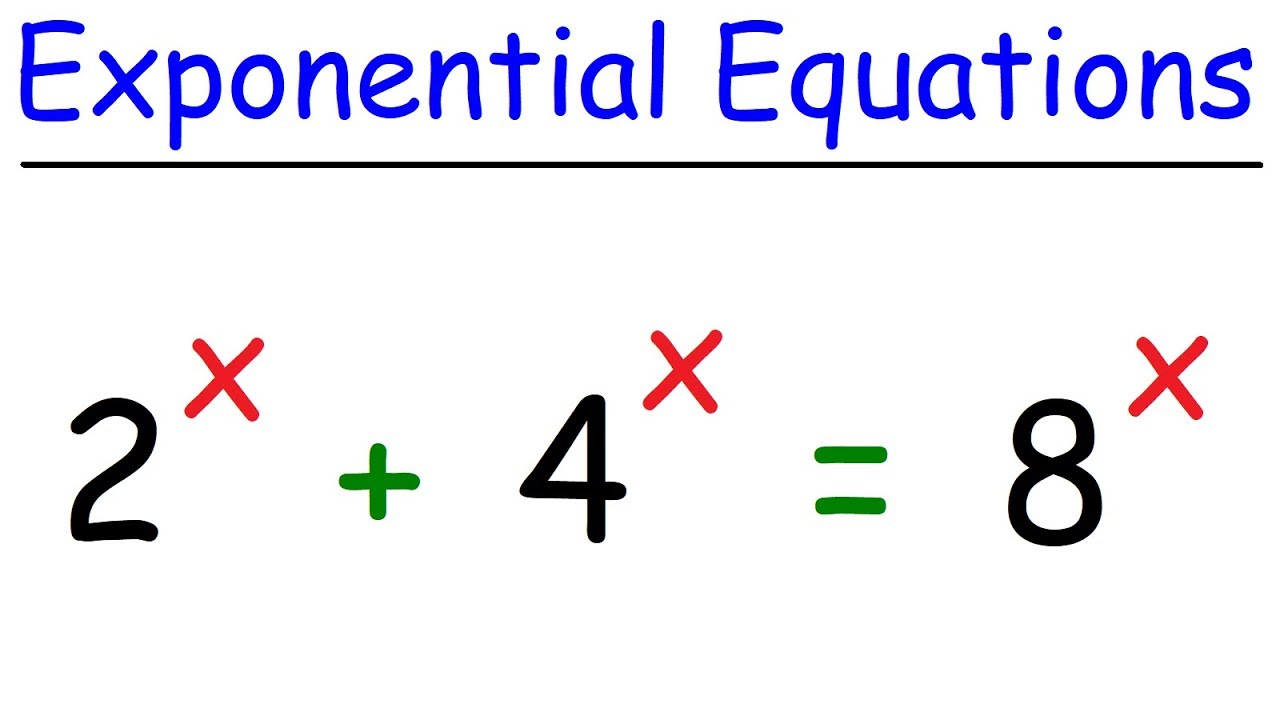
Solving Exponential Equations Using Logarithms & The Quadratic Formula

Solving Exponential Equations with Common Bases (Precalculus - College Algebra 54)
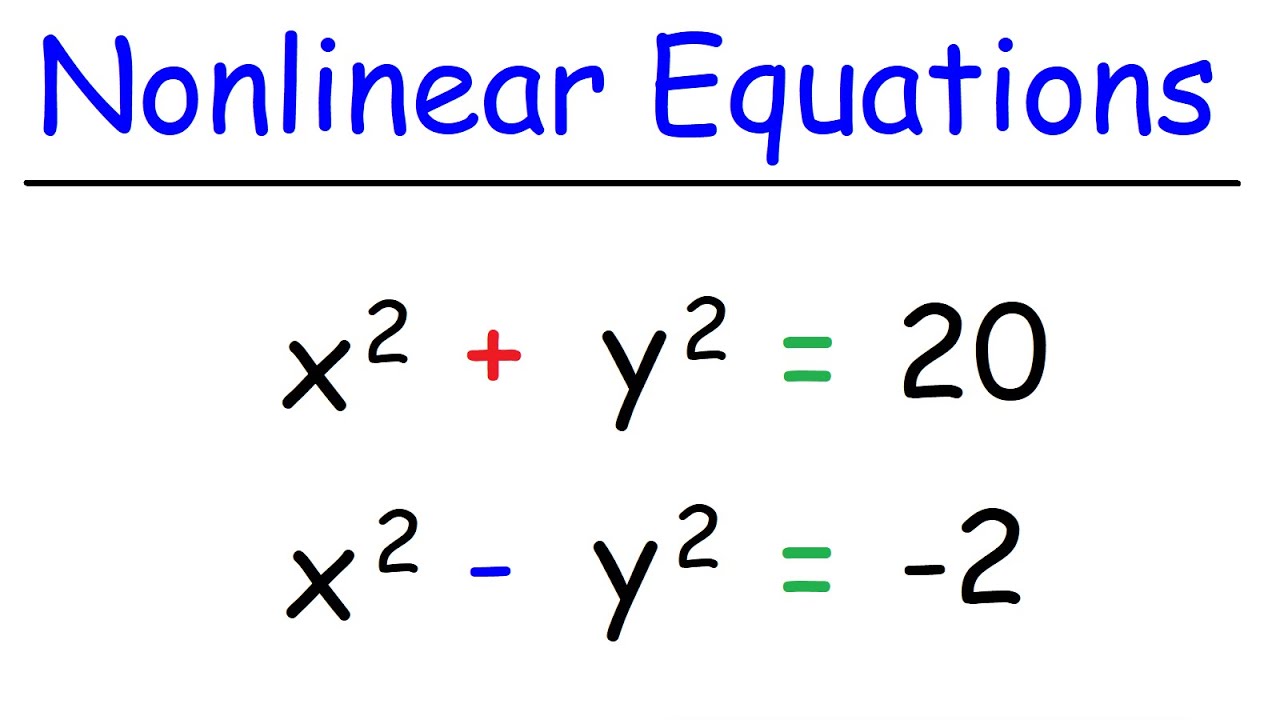
How To Solve Systems of Nonlinear Equations
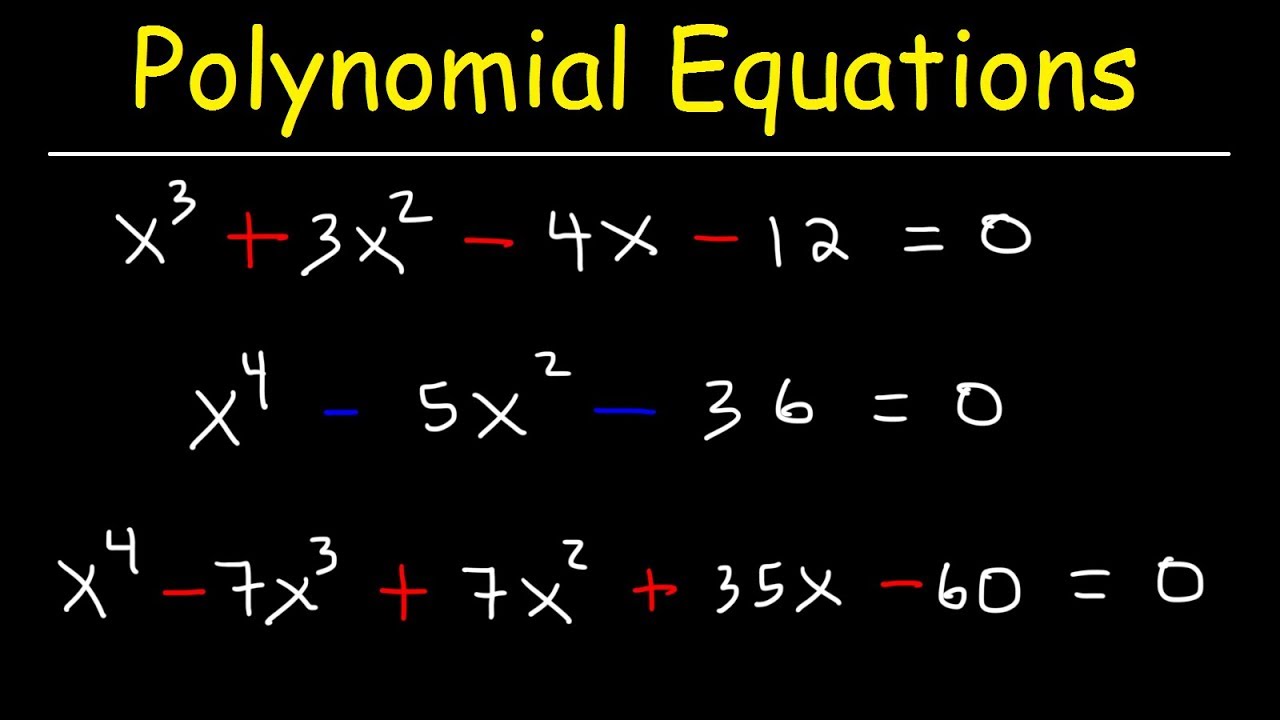
Solving Polynomial Equations By Factoring and Using Synthetic Division
Solving Systems of Equations By Elimination & Substitution With 2 Variables
5.0 / 5 (0 votes)
Thanks for rating: