First Principles Example: Square Root of x

Eddie Woo

22 Feb 201303:46

EducationalLearning

32 Likes 10 Comments

TLDRThe video script discusses a method for finding the derivative of a function involving square roots. It emphasizes starting with the limit definition and manipulating the expression to eliminate the variable 'h' from the denominator. The script introduces the concept of conjugates and the difference of squares to simplify the numerator. By rationalizing the expression, the 'h' term is canceled, allowing for the substitution of 'h' with zero. The result is simplified to a form involving the square root of 'x', illustrating the power rule for derivatives, which applies to both whole and fractional exponents.

Takeaways

📚 Start with the limit: The speaker emphasizes the importance of beginning with the limit when solving calculus problems.
🔍 Recognize the function: The function \( f(x+h) \) is identified as the square root of \( x + h \) and \( f(x) \) as the square root of \( x \).
🧩 Subtract the function: The process involves subtracting \( f(x) \) from \( f(x+h) \) to set up the limit expression.
🌐 Simplify the expression: The goal is to simplify the expression to eliminate the variable \( h \) from the denominator.
🔄 Use of conjugates: Conjugates are introduced to facilitate the manipulation of the square roots in the numerator.
🔢 Difference of squares: The script explains using the difference of squares to simplify the expression involving square roots.
✂️ Cancel out terms: Terms are canceled out in the numerator to simplify the expression further.
🎯 Focus on the numerator: The focus shifts to the numerator after simplifying the denominator, aiming to get rid of the \( h \) term.
🚫 Eliminate the variable \( h \): The script demonstrates how to eliminate \( h \) by setting \( h = 0 \) after simplification.
📈 Final result: The final result of the limit as \( h \) approaches zero is \( \frac{1}{\sqrt{x} + x\sqrt{x}} \), which simplifies to \( x^{-\frac{1}{2}} \).
📘 Power rule application: The script concludes with the application of the power rule \( nx^{n-1} \), which applies to both whole numbers and fractions.

Q & A

What is the initial step recommended when dealing with limits involving square roots?
-The initial step recommended is to always start with the limit notation, which involves writing down the function f(x+h) minus f(x), all divided by h.
Why is it important to eliminate the variable h from the denominator in the limit calculation?
-Eliminating h from the denominator is important because it simplifies the expression and allows us to evaluate the limit as h approaches zero.
What is the purpose of using conjugates in the context of this script?
-Conjugates are used to create a difference of squares, which can then be factorized and simplified, making it easier to work with the numerator of the limit expression.
How does the script suggest simplifying the expression involving square roots in the numerator?
-The script suggests using the difference of squares factorization, which allows the square roots to interact and be simplified more easily.
What is the difference between rationalizing and irrationalizing the denominator in this context?
-Rationalizing the denominator typically involves eliminating radicals from the denominator, whereas irrationalizing, as mentioned in the script, involves creating a form that allows for easier manipulation of the numerator.
What happens when the common terms in h cancel out in the numerator?
-When the common terms in h cancel out, it simplifies the expression and allows for the evaluation of the limit by substituting h with zero.
Why is it possible to substitute h with zero after the simplification process described in the script?
-After simplification, the h terms that were in common cancel out, leaving an expression that no longer contains h, thus allowing for the substitution of h with zero to evaluate the limit.
What is the final expression obtained after substituting h with zero in the script?
-The final expression obtained is one over the square root of x plus the square root of x, which simplifies to one over two times the square root of x.
How does the script relate the final expression to an index form?
-The script relates the final expression to an index form by expressing it as x to the power of -1/2, which is equivalent to one over the square root of x.
What rule does the script mention that applies to the power of a variable?
-The script mentions the rule n * x to the power of n minus one, which applies to both whole numbers and fractions as the exponent n.

Outlines

00:00

📚 Calculus: Understanding Limits with Square Roots

The speaker is addressing a common challenge in calculus when dealing with limits involving square roots. They suggest starting with the limit and writing the function f(x+h) as the square root of (x+h), then subtracting f(x), which is the square root of x, all over h. The goal is to eliminate h from the denominator. The speaker explains that expanding and manipulating the numerator to make h common is a key step. They introduce the concept of conjugates to simplify the square roots by using the difference of squares, which allows for cancellation in the numerator. The process leads to a simplified form where h can be set to zero, resulting in 1/(√x + x√), which simplifies further to x^(-1/2), illustrating the power rule for derivatives.

Mindmap

Keywords

💡Limit

In calculus, the limit is a fundamental concept that describes the behavior of a function as its input approaches a certain value. It is used to define continuity, derivatives, and integrals. In the video, the instructor emphasizes starting with the limit to understand the behavior of the function as 'h' approaches zero, which is a common approach in finding derivatives.

💡Square root

A square root is a value that, when multiplied by itself, gives the original number. It is represented as \( \sqrt{x} \). In the script, square roots are central to the function being analyzed, and the instructor discusses manipulating expressions involving square roots to simplify the limit process.

💡Conjugate

A conjugate, in the context of algebra, is a pair of complex numbers that differ by a sign in at least one of their components. However, in the script, the term is used to describe a technique for simplifying expressions involving square roots by creating a difference of squares, which is a useful strategy in the manipulation of the function under the limit.

💡Difference of squares

The difference of squares is an algebraic identity stating that for any two terms 'a' and 'b', the expression \( a^2 - b^2 \) can be factored into \( (a + b)(a - b) \). The instructor uses this identity to simplify the numerator of the expression after introducing conjugates, which helps in canceling out terms and solving the limit.

💡Numerator

The numerator is the top part of a fraction. In the video, the instructor manipulates the numerator of the expression to create common terms that can be canceled out with the denominator, which is a crucial step in solving the limit.

💡Denominator

The denominator is the bottom part of a fraction. The script mentions 'irrationalizing the denominator' as a strategy to simplify the expression, which is the opposite of the more common 'rationalizing the denominator' and is done to facilitate the cancellation of terms.

💡Factorize

Factorization is the process of breaking down a polynomial or expression into a product of its factors. In the context of the video, the instructor factorizes the expression involving square roots to create a difference of squares, which simplifies the process of finding the limit.

💡Continuity

Continuity in calculus refers to a property of functions where the limit of the function as x approaches a certain value is equal to the function's value at that point. While not explicitly mentioned in the script, the concept of limits inherently involves continuity, as the instructor is finding the derivative, which is related to the function's continuity.

💡Derivative

A derivative in calculus represents the rate at which a function changes with respect to its independent variable. It is often found using limits, as the instructor is doing in the script. The process described is a method for finding the derivative of the square root function.

💡Index form

Index form, also known as exponential notation, is a way of expressing a number multiplied by itself a certain number of times. In the script, the instructor translates the expression involving square roots into index form to show the result of the limit process, which simplifies the understanding of the function's behavior.

💡Fraction

A fraction represents a part of a whole, expressed as one integer divided by another. In the context of the video, the instructor mentions that the rule for derivatives applies to any number, including fractions, as part of the explanation for the general rule \( \frac{d}{dx}[x^n] = nx^{n-1} \).

Highlights

Starting with the limit when solving calculus problems.

The importance of not forgetting to include the limit at the beginning of the problem.

The function f(x+h) is defined as the square root of (x+h).

The goal is to eliminate the variable h from the denominator.

Using the conjugate to facilitate the elimination of h from the numerator.

Difference of squares technique is applied to simplify the expression.

The factorization of the expression results in a squared minus b squared form.

The conjugate does not affect the denominator, which is unconventional in rationalization.

Cancellation of terms in the numerator simplifies the expression.

The h terms cancel out, allowing for h to be set to zero.

Substituting h with zero simplifies the expression to one over the square root of x.

Expressing the result in index form, showing x to the power of negative half.

The rule nx^(n-1) applies to both whole numbers and fractions.

The process demonstrates a method to solve calculus problems involving square roots.

The approach emphasizes the manipulation of the numerator to simplify the expression.

The transcript provides a step-by-step guide on how to handle square roots in calculus.

Transcripts

Browse More Related Video

2.2 - Problem #4 (Calc)

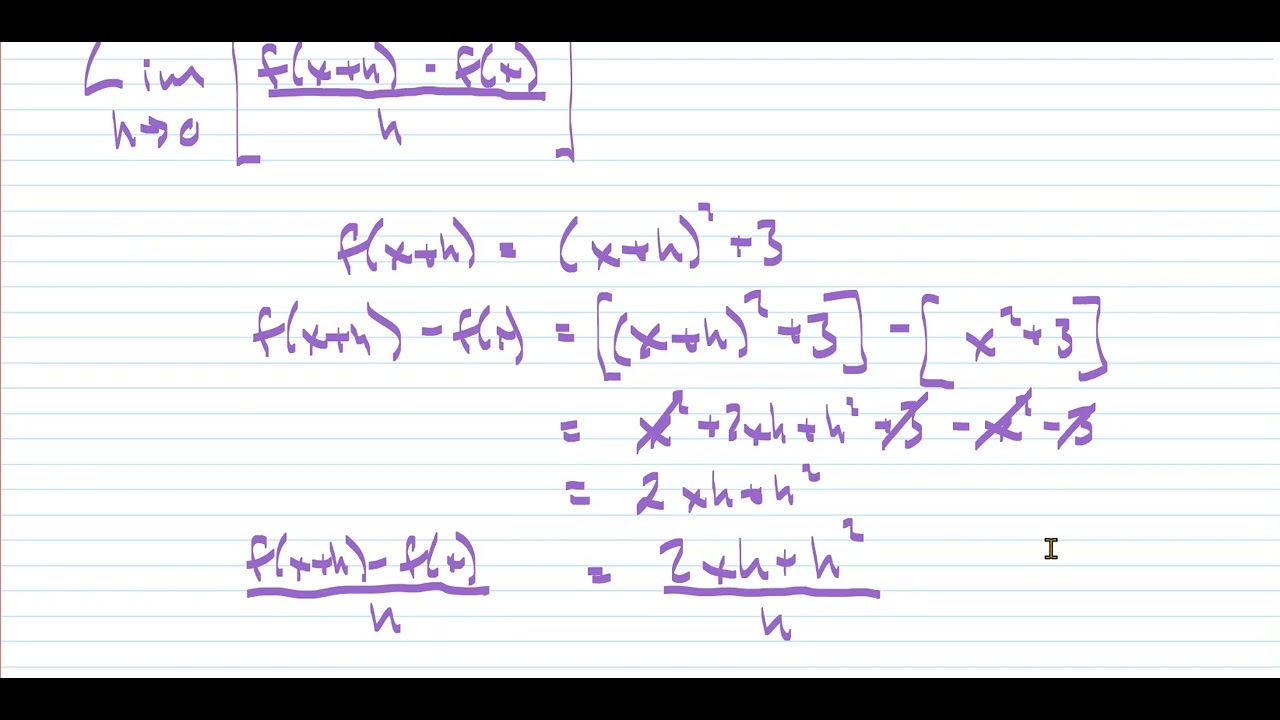
Finding Derivative Using Limit Definition

First principles grade 12: Practice

Limits at infinity of quotients with square roots (even power) | AP Calculus AB | Khan Academy

Applying the Definition of the Derivative to 1/x

Limits by rationalizing | Limits and continuity | AP Calculus AB | Khan Academy

First Principles Example: Square Root of x

Takeaways

Q & A

What is the initial step recommended when dealing with limits involving square roots?

Why is it important to eliminate the variable h from the denominator in the limit calculation?

What is the purpose of using conjugates in the context of this script?

How does the script suggest simplifying the expression involving square roots in the numerator?

What is the difference between rationalizing and irrationalizing the denominator in this context?

What happens when the common terms in h cancel out in the numerator?

Why is it possible to substitute h with zero after the simplification process described in the script?

What is the final expression obtained after substituting h with zero in the script?

How does the script relate the final expression to an index form?

What rule does the script mention that applies to the power of a variable?