Tensors as a Sum of Symmetric and Antisymmetric Tensors
TLDRThis video script delves into the concept of tensor calculus in physics, specifically focusing on the decomposition of any second-rank tensor into a sum of symmetric and antisymmetric tensors. It defines the properties of symmetry and anti-symmetry by examining the behavior of tensors under index transposition. The script then provides a proof for this decomposition, demonstrating how to derive symmetric and antisymmetric components from a given tensor. It also offers a visual representation of the matrix elements, illustrating the zero diagonal in antisymmetric tensors. The video concludes with an example of the electromagnetic field strength tensor, highlighting the minus sign characteristic of anti-symmetric tensors.
Takeaways
- 📚 The video discusses the concept of expressing any second rank tensor as a sum of a symmetric and an antisymmetric tensor.
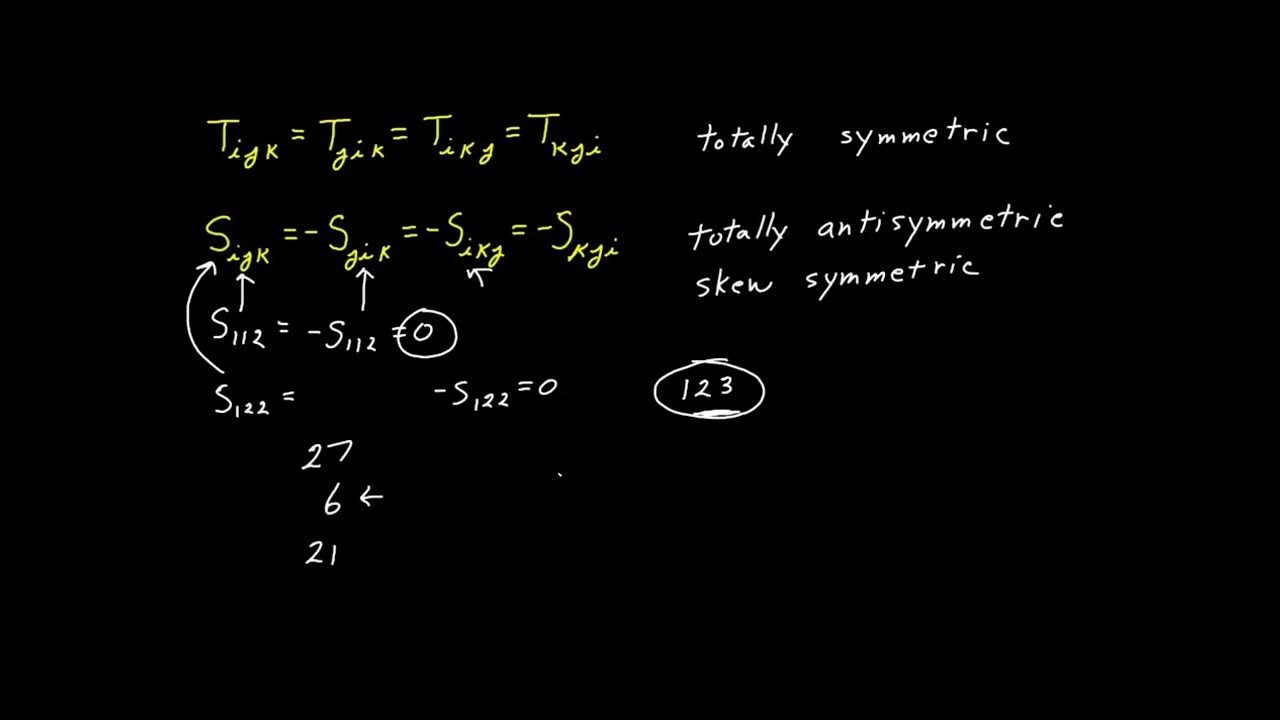
- 🔍 It defines what a symmetric tensor is, explaining that it remains unchanged when indices are swapped (Tμν = Tνμ).
- 🔍 It also defines an antisymmetric tensor, which changes sign when indices are swapped (Tμν = -Tνμ), and results in a zero diagonal in matrix representation.
- 🌐 The script provides examples of symmetric tensors, such as the metric tensor and the inertia tensor.
- 🌐 It gives the electromagnetic field strength tensor as an example of an antisymmetric tensor.
- 📝 The proof begins by manipulating the original tensor Tμν by adding and subtracting the same terms to separate symmetric and antisymmetric components.
- 🔢 The symmetric part of the tensor is obtained by adding Tμν and Tνμ, which results in a tensor that remains the same when transposed.
- 🔢 The antisymmetric part is derived by subtracting Tνμ from Tμν, which leads to a tensor that changes sign upon transposition.
- 📊 The script visually represents the matrix form of both symmetric and antisymmetric tensors to illustrate their properties.
- 🧲 The characteristic minus sign in the definition of an antisymmetric tensor is highlighted, particularly in the context of the electromagnetic field strength tensor.
- 📑 The video concludes with a simple proof that any second rank tensor can be decomposed into symmetric (Sμν) and antisymmetric (Aμν) parts, with Tμν = Sμν + Aμν.
- 🎓 The presenter encourages viewers to apply this knowledge and share examples of how tensors can be decomposed into symmetric and antisymmetric components.
Q & A
What is the main topic of the video script?
-The main topic of the video script is the proof that any arbitrary second rank tensor can be expressed as the sum of a symmetric tensor and an antisymmetric tensor.
What does it mean for a tensor to be symmetric?
-A tensor is symmetric if, when its indices are swapped, the value of the tensor remains the same, i.e., T_mu_nu equals T_nu_mu.
What is an example of a symmetric tensor?
-Examples of symmetric tensors include the metric tensor and the inertia tensor.
What does it mean for a tensor to be antisymmetric?
-A tensor is antisymmetric if, when its indices are swapped, the value of the tensor is the negative of the original, i.e., T_mu_nu equals -T_nu_mu.
Why do antisymmetric tensors have a diagonal of zeros?
-Antisymmetric tensors have a diagonal of zeros because T_mu_mu equals -T_mu_mu, which can only be true if the diagonal elements are zero.
What is an example of an antisymmetric tensor?
-An example of an antisymmetric tensor is the electromagnetic field strength tensor, F_mu_nu.
How does the proof show that any second rank tensor can be split into symmetric and antisymmetric parts?
-The proof shows this by adding and subtracting the same terms from the original tensor T_mu_nu, creating a new expression that separates the symmetric and antisymmetric components.
What is the significance of the transpose operation in the context of this proof?
-The transpose operation is significant because it is used to determine the symmetry or antisymmetry of a tensor by comparing the tensor with its transpose.
How does the script demonstrate the symmetric part of the tensor?
-The script demonstrates the symmetric part by showing that when the indices are swapped, the expression remains unchanged, indicating symmetry.
How does the script demonstrate the antisymmetric part of the tensor?
-The script demonstrates the antisymmetric part by showing that when the transpose of the expression is taken, it results in the negative of the original expression, indicating antisymmetry.
What is the final expression for the original tensor T_mu_nu in terms of its symmetric and antisymmetric parts?
-The final expression is T_mu_nu equals S_mu_nu plus A_mu_nu, where S_mu_nu is the symmetric part and A_mu_nu is the antisymmetric part.
Outlines
📚 Introduction to Tensor Decomposition
This paragraph introduces the concept of tensor decomposition in the context of physics. The speaker explains that any second-rank tensor can be decomposed into a sum of a symmetric and an antisymmetric tensor. The explanation begins with defining the terms 'symmetric' and 'antisymmetric' by describing what happens to a tensor when its indices are swapped. A symmetric tensor remains unchanged when transposed, whereas an antisymmetric tensor changes sign. Examples of symmetric tensors include the metric tensor and the inertia tensor, while the electromagnetic field strength tensor is given as an example of an antisymmetric tensor. The paragraph sets the stage for proving the decomposition theorem.
🔍 Proving Tensor Decomposition Theorem
The speaker delves into proving the tensor decomposition theorem by manipulating the original tensor equation. The process involves adding and subtracting terms to separate the symmetric and antisymmetric components. The symmetric part of the tensor is identified as the sum of the original tensor and its transpose, divided by two, which remains unchanged upon transposition. Conversely, the antisymmetric part is derived by taking half the difference between the original tensor and its transpose, which results in a tensor that changes sign upon transposition. The proof is visually reinforced by representing the tensors in matrix form, illustrating the zero diagonal elements for antisymmetric tensors. The paragraph concludes with the final expression for the decomposition of an arbitrary tensor into its symmetric and antisymmetric components.
Mindmap
Keywords
💡Second rank tensor
💡Symmetric tensor
💡Antisymmetric tensor
💡Transpose
💡Metric tensor
💡Inertia tensor
💡Electromagnetic field strength tensor
💡Decomposition
💡Matrix representation
💡Diagonal elements
💡Proof
Highlights
Introduction to the concept that any second rank tensor can be expressed as the sum of a symmetric and an antisymmetric tensor.
Definition of tensor symmetry and antisymmetry in relation to index swapping and transposition.
Explanation of symmetric tensors, such as the metric tensor and inertia tensor, and their properties.
Clarification of antisymmetric tensors, including the electromagnetic field strength tensor, and their unique characteristics.
Demonstration of how to prove the decomposition of a tensor into symmetric and antisymmetric parts using mathematical manipulation.
Use of addition and subtraction of the tensor and its transpose to achieve the decomposition.
Factorization of the symmetric part of the tensor, showing how it remains unchanged under index swapping.
Matrix representation of the symmetric tensor, illustrating the process of gathering terms to form a symmetric matrix.
Introduction of the antisymmetric part of the tensor and its properties, including the zero diagonal terms.
Matrix representation of the antisymmetric tensor, showing the negative relationship between transposed elements.
Proof completion by demonstrating the antisymmetric property through matrix element manipulation.
Naming of the symmetric and antisymmetric parts of the tensor for clarity, s_mu_nu for symmetric and a_mu_nu for antisymmetric.
Final expression of the tensor as the sum of its symmetric and antisymmetric components.
Discussion of the practical applications of antisymmetric tensors, such as in the electromagnetic field strength tensor.
Encouragement for viewers to apply the concepts learned to write a tensor as the sum of symmetric and antisymmetric parts.
Closing remarks and invitation for feedback on the video's content and presentation.
Transcripts
Browse More Related Video
5.0 / 5 (0 votes)
Thanks for rating: