AP Calculus AB - 1.6b Determining Limits Using Algebraic Manipulation

Daniel Bortnick

4 Oct 202024:12

EducationalLearning

32 Likes 10 Comments

TLDRIn this AP Calculus AB video, Mr. Bortnick discusses Section 1.6b on determining limits using algebraic manipulation. He emphasizes the importance of direct substitution as the first step in finding limits, and then demonstrates how to handle indeterminate forms like 0/0 through factoring and simplification. Special trigonometric limits are introduced, which are crucial for the AP exam, and several examples are worked through to illustrate the process of simplifying limits involving sine and cosine functions. The video concludes with practice problems for students to apply these concepts.

Takeaways

📘 Always start with direct substitution when attempting to find a limit in a function, as it is the simplest method to try first.
📚 Direct substitution involves substituting the value you're approaching into the function to see if it yields a numerical answer.
🚫 If direct substitution results in an indeterminate form like 0/0, it means you need to simplify the expression further before finding the limit.
🔍 For horizontal lines or constant functions, direct substitution will always yield the constant value as the limit, regardless of the approach value.
📉 In cases where direct substitution is not possible or yields an indeterminate form, use algebraic manipulation such as factoring to simplify the expression.
🔄 After factoring, cancel out common factors in the numerator and denominator, then try direct substitution again to find the limit.
❗ Be cautious not to write an expression as 'equals zero over zero', as this is a common mistake that can lead to points being deducted on exams.
📊 Special trigonometric limits, such as sin(x)/x approaching 1 as x approaches 0, must be memorized as they are frequently tested on the AP exam.
📐 The difference of squares factoring technique can be applied to trigonometric functions to simplify expressions involving cosine squared x - 1.
📝 When dealing with limits involving trigonometric functions, use properties of limits to manipulate the expression into a form that matches memorized limits.
📉 For limits that do not exist, such as when the direct substitution results in an undefined expression like 15/0, it's a signal that the limit does not exist.

Q & A

What is the first step Mr. Bortnick recommends when trying to find a limit in calculus?
-The first step Mr. Bortnick recommends is direct substitution, where you substitute the value of x directly into the function and see what happens.
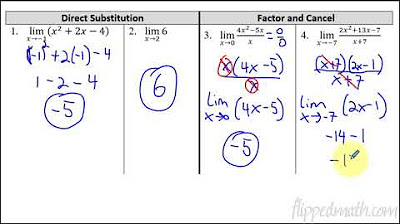
What is the result of the limit as x approaches -1 for the function x^2 + 2x - 4?
-By substituting x with -1, the result of the limit is -1 squared plus 2 times -1 minus 4, which simplifies to 1 - 2 - 4, giving a result of -5.
Why is direct substitution not applicable for the limit as x approaches 2 for the function f(x) = 6?
-Direct substitution is not applicable because the function is a constant (6), and the value of x does not affect the output, making the limit equal to 6 itself.
What is the term used for a limit that results in 0/0 in calculus?
-A limit that results in 0/0 is referred to as an indeterminate form in calculus.
How does Mr. Bortnick simplify the limit as x approaches 0 for the function (4x^2 - 5x) / x?
-He factors the numerator to x(4x - 5), cancels out the x terms, and then applies direct substitution with x = 0, resulting in the limit being -5.
What is the common mistake Mr. Bortnick warns against when dealing with indeterminate forms like 0/0?
-The common mistake is to incorrectly write that the expression equals zero over zero, which is not allowed as it implies division by zero.
What is the result of the limit as x approaches -7 for the function (2x^2 + 13x - 7) / (x + 7)?
-After factoring and canceling out the common terms, the limit simplifies to -2x - 1, and substituting x with -7 gives a result of -15.
Why does Mr. Bortnick say the limit does not exist for the function (x^2 - 6x + 3) / (x + 6) as x approaches -6?
-The limit does not exist because direct substitution results in an undefined expression (15/0), and no factors can be canceled out to simplify the expression further.
What are the special trigonometric limits that Mr. Bortnick mentions should be memorized for the AP exam?
-The limits to memorize are: lim (x->0) sin(x)/x = 1, lim (x->0) x/sin(x) = 1, lim (x->0) (1 - cos(x))/x = 0, and lim (x->0) (x/(1 - cos(x))) = 0.
How does Mr. Bortnick solve the limit as x approaches 0 for the function sin(3x) / x?
-He multiplies both the numerator and the denominator by 3, then uses the special trig limit lim (x->0) sin(x)/x = 1, resulting in the limit being 3.
What technique does Mr. Bortnick use to solve the limit as x approaches 0 for the function sin(7x) / sin(9x)?
-He uses the property of limits that allows splitting the limit statement and then manipulates the fractions to have the same terms in the numerator and denominator, resulting in the limit being 7/9.
How does the difference of two squares factoring technique apply to the limit as x approaches 0 for the function (cos^2(x) - 1) / (x * (cos(x) + 1))?
-The numerator (cos^2(x) - 1) factors into (cos(x) + 1) * (cos(x) - 1), which cancels out with the denominator, leaving a simplified expression that results in the limit being 0.

Outlines

00:00

📚 Introduction to Algebraic Manipulation in Limits

Mr. Bortnick introduces the topic of limits in calculus, specifically focusing on algebraic manipulation to determine limits. He emphasizes the importance of direct substitution as the first step in finding limits, and provides examples where this method is applicable, such as in the function x^2 + 2x - 4 as x approaches -1. He also discusses cases where direct substitution results in an indeterminate form, like 0/0, and how to resolve these by factoring and simplifying the equation.

05:00

🔍 Dealing with Indeterminate Forms and Factoring Techniques

The paragraph delves deeper into handling indeterminate forms that arise from direct substitution. Mr. Bortnick illustrates how to factor expressions to simplify limits, using the example of 4x^2 - 5x / x as x approaches 0. He also cautions against incorrectly stating that an expression equals zero over zero, which is a common mistake that can lead to points being deducted on exams. The solution involves factoring and canceling out terms to find the limit.

10:03

🚫 Understanding Limits That Do Not Exist

This section addresses scenarios where the limit does not exist, as indicated by an expression that does not simplify to an indeterminate form. Mr. Bortnick demonstrates this with an example where direct substitution results in a non-zero value divided by zero. He explains that this is a signal that factoring may not resolve the limit and concludes that the limit does not exist for the given function.

15:03

📘 Memorizing Special Trigonometric Limits for AP Exams

Mr. Bortnick highlights the necessity for students to memorize specific trigonometric limits that are frequently tested on the AP exam. These limits involve sine and cosine functions as x approaches zero, such as sin(x)/x and x/sin(x) both tending to 1, and (1 - cos(x))/x tending to 0. He stresses that these rules apply exclusively when x approaches zero and that students should be prepared to apply these memorized limits in problem-solving.

20:07

🌐 Applying Trigonometric Limits in Algebraic Contexts

The paragraph explores the application of the previously mentioned trigonometric limits in more complex algebraic contexts. Mr. Bortnick shows how to manipulate functions involving sine and cosine to fit the form required for these special limits. He uses the example of sin(3x)/x as x approaches 0, demonstrating how to adjust the function to utilize the known limit of sin(x)/x equaling 1, resulting in the limit being 3.

🔄 Creative Approaches to Trigonometric Limit Problems

In this section, Mr. Bortnick discusses creative methods to tackle trigonometric limit problems that do not directly fit the special limit forms. He uses the example of sin(7x)/sin(9x) as x approaches 0, showing how to rewrite the expression to separate the limit statements and apply the known trigonometric limits to find the solution, which in this case is 7/9.

📐 Factoring Techniques with Trigonometric Functions

The final paragraph focuses on applying factoring techniques, such as the difference of squares, to trigonometric functions within limits. Mr. Bortnick demonstrates this with the function cos^2(x) - 1 / (x * (cos(x) + 1)) as x approaches 0. He shows how factoring the numerator and recognizing the special trigonometric limit can simplify the problem and lead to the conclusion that the limit is 0.

Mindmap

Keywords

💡Direct Substitution

Direct substitution is the initial method suggested in the script for finding limits in calculus. It involves substituting the value that the variable approaches directly into the function to see if a determinate value results. This method is fundamental to the theme of the video, which is about determining limits using algebraic manipulation. For example, when finding the limit as x approaches -1 for the function x^2 + 2x - 4, direct substitution results in the value -5.

💡Limit

A limit in calculus is the value that a function or sequence approaches as the input approaches some value. The concept is central to the video's theme, which focuses on finding these values using algebraic techniques. The script discusses various methods to determine limits, such as direct substitution and factoring, especially when direct substitution is not sufficient due to an indeterminate form like 0/0.

💡Indeterminate Form

An indeterminate form, such as 0/0, arises when direct substitution results in a division by zero, which is undefined. The script emphasizes that when this occurs, additional algebraic manipulation is necessary to find the limit. The concept is crucial for understanding the limitations of direct substitution and the need for further techniques.

💡Factoring

Factoring is an algebraic technique used to break down a polynomial into a product of simpler polynomials. In the context of the video, factoring is used to simplify expressions and solve indeterminate forms, such as transforming 4x^2 - 5x into x(4x - 5) to cancel out the x term in the denominator and find the limit as x approaches 0.

💡Algebraic Manipulation

Algebraic manipulation refers to the process of transforming algebraic expressions using various mathematical rules and techniques. The video's main theme revolves around using such manipulations to determine limits that cannot be found through direct substitution. Examples include factoring and simplifying fractions to find determinate limit values.

💡Trigonometric Limits

Trigonometric limits are specific limits involving trigonometric functions that the AP exam expects students to memorize. The script mentions several of these, such as the limit of sin(x)/x as x approaches 0, which is equal to 1. These limits are essential for solving more complex problems and are a key part of the video's educational content.

💡Sine Function

The sine function is a fundamental trigonometric function that relates the angle of a right triangle to the ratio of the length of the side opposite the angle to the length of the hypotenuse. In the script, the sine function is used in various limit problems, such as sin(3x)/x, which requires manipulation to apply the special trigonometric limit sin(x)/x as x approaches 0.

💡Cosine Function

The cosine function, like the sine function, is a trigonometric function used in the context of right triangles and periodic phenomena. The script discusses the limit involving the cosine function squared minus one over x, which can be factored using the difference of squares technique to find the limit as x approaches 0.

💡Difference of Squares

The difference of squares is an algebraic identity that states a^2 - b^2 = (a + b)(a - b). The script applies this identity to factor expressions like cos^2(x) - 1 into cos(x) + 1 and cos(x) - 1, which simplifies the process of finding the limit as x approaches 0.

💡Horizontal Line

A horizontal line in the context of the script refers to a constant function, such as y = 6, where the output does not change regardless of the input value. The script uses this concept to explain that the limit as x approaches 2 for the function 6 is simply 6, as it represents a horizontal line at y = 6.

Highlights

Introduction to the topic of determining limits using algebraic manipulation in calculus.

Emphasis on the importance of direct substitution as the first step in finding limits.

Explanation of the process for direct substitution and its application in example problems.

Identification of indeterminate forms, such as 0/0, in limits calculations.

Technique of factoring to resolve indeterminate forms and simplify limit expressions.

Demonstration of how to handle horizontal lines in limit problems where direct substitution is not applicable.

Use of the 'big x' factoring method to simplify expressions involving trigonometric limits.

Special trigonometric limits that must be memorized for the AP exam, specifically limits involving sine and cosine functions.

Application of the difference of squares factoring technique to trigonometric functions in limits.

The concept of limits not existing when the left and right limits do not converge to the same value.

Creative algebraic manipulation to align with special trigonometric limits for solving complex problems.

Avoiding common mistakes such as equating indeterminate forms to zero over zero in exam responses.

The significance of the convention for writing squared trigonometric functions to avoid ambiguity.

Practical examples of how to apply algebraic manipulation to find limits in various scenarios.

The process of simplifying complex limit expressions step by step, including factoring and direct substitution.

The importance of correctly identifying and applying special trigonometric limits in limit calculations.

The final practice problems provided for students to apply the concepts learned in the lesson.

Transcripts

Browse More Related Video

AP Calculus AB - 1.7 Selecting Procedures for Determining Limits

Calculus AB/BC – 1.6 Determining Limits Using Algebraic Manipulation

Limits Involving Sine and Cosine & The Squeeze Theorem!

AP Calculus AB - 1.5 Determining Limits Using Algebraic Properties of Limits

AP Calculus AB - 1.8 Determining Limits Using the Squeeze Theorem

AP Calculus AB: Introduction to Limits

Related Tags

Calculus Techniques AP Calculus Limits Determination Algebraic Manipulation Direct Substitution Indeterminate Forms Factoring Methods Trigonometric Limits Educational Content Mathematics Tutorial AP Exam Prep