AP Calculus AB - 1.7 Selecting Procedures for Determining Limits

Daniel Bortnick

4 Oct 202026:30

EducationalLearning

32 Likes 10 Comments

TLDRIn this AP Calculus AB video by Mr. Bortnick, the focus is on Unit 1, specifically topic 1.7, which discusses selecting procedures for determining limits. The video provides a comprehensive review of rationalizing functions and techniques for simplifying limits, including the use of algebraic manipulation and the conjugate method. Examples are worked through to illustrate these concepts, emphasizing the importance of direct substitution and handling indeterminate forms in limit problems.

Takeaways

📚 The lesson is focused on 'Selecting Procedures for Determining Limits' in the context of AP Calculus AB, emphasizing algebraic techniques to simplify limits.
📐 The instructor introduces the concept of rationalizing the denominator, which is a technique used to eliminate radicals from the denominator of a fraction.
🔍 An example provided is simplifying the expression 1/√2 by multiplying the numerator and denominator by √2, resulting in √2/2.
📝 The script reviews the process of rationalizing more complex expressions, such as 5/√3, and demonstrates the steps to simplify it to 5√3/6.
🤔 The instructor explains that higher-level math typically does not leave final answers with radicals in the denominator, hence the need for rationalization.
🤓 The use of the conjugate is introduced as a method to simplify expressions involving square roots, particularly when adding or subtracting square roots.
🧩 The concept of 'difference of two squares' is applied to multiply the numerator and denominator by the conjugate of the radical to eliminate the square root.
📉 The script includes a step-by-step walkthrough of solving limit problems by rationalizing and using algebraic manipulation, such as in the example limit as x approaches 5 of √(x+4) - 3 / (x-5).
📌 The importance of direct substitution is highlighted as the first step in solving limit problems, followed by algebraic techniques if an indeterminate form is encountered.
📈 The lesson also covers complex fractions and how to handle them by making the denominators the same and combining fractions.
📚 Finally, the instructor encourages students to practice the techniques learned with the provided problems and to check their answers for understanding.

Q & A

What is the main topic of the video lecture?
-The main topic of the video lecture is 'Selecting Procedures for Determining Limits' in the context of AP Calculus AB, specifically focusing on techniques for simplifying limits using algebraic methods.
Why is it important to rationalize the denominator in higher-level math?
-In higher-level math, it is important to rationalize the denominator to avoid leaving final answers with radicals in the denominator, which is considered an unsimplified form.
What is the process of rationalizing the denominator?
-The process of rationalizing the denominator involves multiplying both the numerator and the denominator of a fraction by the radical part in the denominator, which eliminates the radical and simplifies the expression.
Can you provide an example of rationalizing the denominator with a square root?
-An example given in the script is rationalizing the expression 1/√2 by multiplying both the numerator and the denominator by √2, resulting in (1*√2)/(√2*√2) which simplifies to √2/2.
What is the difference of two squares formula and how is it used in the script?
-The difference of two squares formula is a^2 - b^2 = (a + b)(a - b). In the script, it is used to simplify expressions involving square roots by multiplying the numerator and denominator by the conjugate of the radical part.
How does the script handle the addition of square roots in an expression?
-The script suggests using the difference of two squares to handle the addition of square roots in an expression, by multiplying by the conjugate to eliminate the square roots and simplify the expression.
What is an indeterminate form and how does it relate to finding limits?
-An indeterminate form, such as 0/0, occurs when direct substitution results in a non-existent or undefined value. It often indicates that an algebraic technique may be needed to simplify the expression before finding the limit.
Can you explain the technique of multiplying by the conjugate to find limits?
-Multiplying by the conjugate is a technique used to simplify expressions with radicals or complex fractions. By multiplying both the numerator and the denominator by the conjugate, terms can cancel out, potentially leading to a determinate form that allows for limit evaluation.
How does the script address complex fractions in limit problems?
-The script addresses complex fractions by first trying direct substitution. If that results in an indeterminate form, it suggests using algebraic techniques to simplify the expression, such as making the denominators the same and then combining the fractions.
What is the purpose of combining fractions with the same denominator in the script?
-Combining fractions with the same denominator simplifies the process of finding limits by allowing the numerator to be simplified first, and then canceling out common factors with the denominator before direct substitution.
Can you provide an example of simplifying a complex fraction from the script?
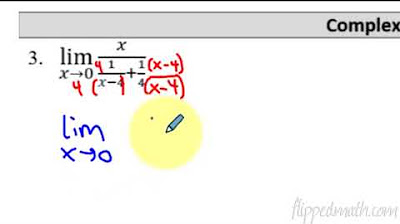
-An example from the script is simplifying the limit as x approaches 0 of x/(1/(x-4) + 1/4) by making the denominators the same and then combining the fractions into a single fraction that can be simplified before finding the limit.
How does the script suggest simplifying a fraction divided by another fraction?
-The script suggests simplifying a fraction divided by another fraction by multiplying by the reciprocal of the denominator, which allows for the cancellation of common factors and simplification of the expression.
What is the final step in finding the limit after simplifying the expression?
-The final step in finding the limit after simplifying the expression is to perform direct substitution, plugging in the value at which the limit is being approached, and simplifying the resulting expression to find the limit.

Outlines

00:00

📚 Introduction to Rationalizing Denominators

In this introductory segment, Mr. Bortnick begins by discussing the importance of rationalizing denominators in higher-level mathematics, specifically in the context of calculus. He explains that radicals in the denominator are not acceptable and must be rationalized using algebraic techniques. The segment includes a step-by-step demonstration of rationalizing the denominator for the expression 1 over the square root of 2, transforming it into a simplified form without radicals in the denominator. This process is crucial for simplifying limits and understanding the fundamental algebraic principles that are essential for more advanced mathematical concepts.

05:01

🔍 Advanced Rationalization Techniques

This paragraph delves deeper into the rationalization process, showcasing how to handle more complex expressions involving radicals. Mr. Bortnick demonstrates the technique of multiplying by the conjugate to rationalize the denominator of expressions like 5 over 2 root 3. He also addresses the use of the difference of squares formula to simplify expressions with radicals in the numerator and denominator, such as 2 over (3 plus the square root of 7). The explanation includes a detailed walkthrough of the algebraic manipulations required to achieve a simplified form, emphasizing the importance of recognizing and applying these techniques in higher-level math problems.

10:03

📘 Applying Rationalization to Limits

The focus shifts to applying rationalization techniques to determine limits in calculus. Mr. Bortnick outlines the process of using rationalization to simplify expressions that arise when evaluating limits, starting with a review of direct substitution and its limitations. He then illustrates how multiplying by the conjugate can help resolve indeterminate forms, such as 0/0, by providing a step-by-step solution to a limit problem involving square roots. This approach not only simplifies the process of finding limits but also reinforces the connection between algebraic skills and their application in calculus.

15:04

📙 Complex Fractions and Limit Simplification

This segment addresses the challenge of evaluating limits involving complex fractions. Mr. Bortnick explains the initial step of direct substitution and its potential to lead to indeterminate forms. He then demonstrates how to simplify complex fractions by creating a common denominator, allowing for the combination of fractions into a single expression. The summary includes a detailed example of transforming a complex fraction into a simpler form and then finding the limit as x approaches a specific value, highlighting the algebraic manipulations necessary to achieve a determinate result.

20:07

📒 Further Exploration of Complex Fractions

Continuing the discussion on complex fractions, Mr. Bortnick provides additional examples to illustrate the process of simplifying these expressions. He shows how to manipulate the numerator and denominator to create a common denominator, which simplifies the process of finding the limit. The segment includes a step-by-step guide on how to multiply by the reciprocal of the denominator to further simplify the expression, ultimately leading to a determinate form that can be evaluated as x approaches zero.

25:07

📕 Concluding Techniques for Limits and Algebra

In the final segment, Mr. Bortnick wraps up the lesson by summarizing the two key algebraic techniques discussed: rationalizing the denominator using the conjugate and working with complex fractions. He emphasizes the importance of these techniques in finding limits and encourages students to practice these methods with the provided problems. The summary serves as a concise review of the material covered, reinforcing the steps and strategies for successfully determining limits in calculus.

Mindmap

Keywords

💡Rationalizing the Denominator

Rationalizing the denominator is a mathematical technique used to eliminate radicals (square roots, cube roots, etc.) from the denominator of a fraction. This is important in higher-level mathematics because expressions with radicals in the denominator are typically not considered simplified. In the video, the instructor demonstrates this by multiplying the numerator and denominator by the square root that appears in the denominator, effectively converting the radical into a rational number. This technique is used multiple times throughout the script to simplify expressions involving limits.

💡Algebraic Manipulation

Algebraic manipulation refers to the process of transforming algebraic expressions using various mathematical operations to simplify them or to make them more suitable for further analysis. In the context of the video, the instructor uses algebraic manipulation to simplify limits by rationalizing denominators and combining fractions. This is a key technique in the study of calculus, especially when dealing with limits that involve radicals or complex fractions.

💡Limits

In calculus, a limit is the value that a function or sequence 'approaches' as the input or index approaches some value. The concept of limits is fundamental to understanding continuity, derivatives, and integrals. The video's theme revolves around determining limits using different algebraic procedures. The script provides several examples of how to find limits by first attempting direct substitution and then applying algebraic techniques when the direct substitution results in an indeterminate form.

💡Indeterminate Form

An indeterminate form occurs when an expression simplifies to a form such as 0/0 or ∞/∞, which does not provide a clear value for the limit. In the script, the instructor encounters indeterminate forms during direct substitution and uses algebraic techniques to resolve them. For example, multiplying by the conjugate is a method to eliminate the indeterminate form and find the actual limit of the expression.

💡Direct Substitution

Direct substitution is the initial approach to finding the limit of a function by simply substituting the value the function is approaching into the function itself. The script emphasizes that the first step in solving limit problems is to attempt direct substitution. If this results in an indeterminate form, as it often does in the video, then further algebraic techniques are applied to find the limit.

💡Conjugate

In the context of the video, the conjugate refers to a pair of expressions that differ only in sign. The instructor uses the concept of conjugates to rationalize expressions and to simplify limits by multiplying the numerator and denominator by the conjugate of the radical in the denominator. This technique is particularly useful when dealing with square roots and other radicals in the denominator of a fraction.

💡Complex Fractions

Complex fractions are fractions that have other fractions in their numerator, denominator, or both. The script discusses techniques for dealing with complex fractions when calculating limits. The instructor shows how to simplify complex fractions by finding a common denominator or by multiplying by the reciprocal of the denominator, which allows for the cancellation of terms and simplification of the expression.

💡Difference of Squares

The difference of squares is an algebraic identity that states that the difference between the squares of two numbers is equal to the product of the sum and the difference of the two numbers (a^2 - b^2 = (a+b)(a-b)). In the script, the instructor uses this identity to simplify expressions involving square roots, specifically when adding terms with radicals by multiplying by the conjugate of the radical to eliminate the square root in the denominator.

💡Reciprocal

A reciprocal of a number is what you multiply that number by to get 1. In the context of the video, the instructor uses the concept of reciprocals to simplify complex fractions by multiplying by the reciprocal of the denominator, which allows for the cancellation of terms and simplification of the expression. This technique is particularly useful when dealing with fractions within fractions.

💡Simplification

Simplification in mathematics refers to the process of making an expression easier to understand or work with by reducing it to its simplest form. Throughout the script, the instructor focuses on simplifying complex expressions, especially those involving limits, by applying various algebraic techniques such as rationalizing the denominator, using the difference of squares, and working with complex fractions.

Highlights

Introduction to the topic of selecting procedures for determining limits in calculus.

Emphasis on two special algebraic techniques for simplifying limits.

Review of rationalizing functions to avoid radicals in the denominator in higher-level math.

Demonstration of rationalizing the denominator for the expression 1 over the square root of 2.

Technique of multiplying by the conjugate to eliminate radicals in the denominator.

Example of rationalizing the denominator for the expression 5 over 2 root 3.

Use of the difference of two squares formula in simplifying expressions with radicals.

Method of multiplying by the conjugate to simplify expressions involving addition of radicals.

Explanation of how to handle complex fractions in limit problems.

Approach to direct substitution in limit problems and handling indeterminate forms.

Application of algebraic techniques to find limits when direct substitution results in indeterminate forms.

Example problem demonstrating the use of conjugates to simplify and find a limit.

Process of simplifying complex fractions by making common denominators.

Technique of dividing by a fraction by multiplying by its reciprocal.

Example of simplifying a complex fraction involving x in the numerator and denominator.

Final problem walkthrough involving complex fractions and direct substitution.

Summary of the two special algebraic techniques for finding limits: rationalizing and working with complex fractions.

Encouragement to practice the techniques with provided problems and check answers.

Transcripts

Browse More Related Video

AP Calculus AB - 1.6b Determining Limits Using Algebraic Manipulation

Calculus AB/BC – 1.7 Selecting Procedures for Determining Limits

AP Calculus AB - 1.5 Determining Limits Using Algebraic Properties of Limits

AP Calculus AB - 1.6a Review of Expanding and Factoring Techniques

Calculus AB/BC – 1.6 Determining Limits Using Algebraic Manipulation

Limits Involving Sine and Cosine & The Squeeze Theorem!

Related Tags

Calculus Tutorial Limits Continuity Algebraic Techniques Rationalizing Conjugate Multiplication Complex Fractions Direct Substitution Math Education AP Calculus Educational Content