Evaluate all the limits from a given graph
TLDRThe speaker explains the concept of limits in calculus, focusing on left-hand and right-hand limits and how they determine the behavior of a function as it approaches specific x-values. The discussion covers various scenarios, such as approaching negative and positive values, discontinuities, and asymptotes. The speaker emphasizes standard notation and highlights that a general limit exists only if the function approaches the same value from both sides. Key examples illustrate how limits are identified and interpreted, particularly when dealing with infinite values and undefined limits.
Takeaways
- 🔍 Understanding limits involves analyzing the value that a function approaches as x gets closer to a specific point.
- ➡️ A negative sign indicates the left-hand limit, meaning the value the function approaches from the left side.
- ⬅️ A positive sign indicates the right-hand limit, meaning the value the function approaches from the right side.
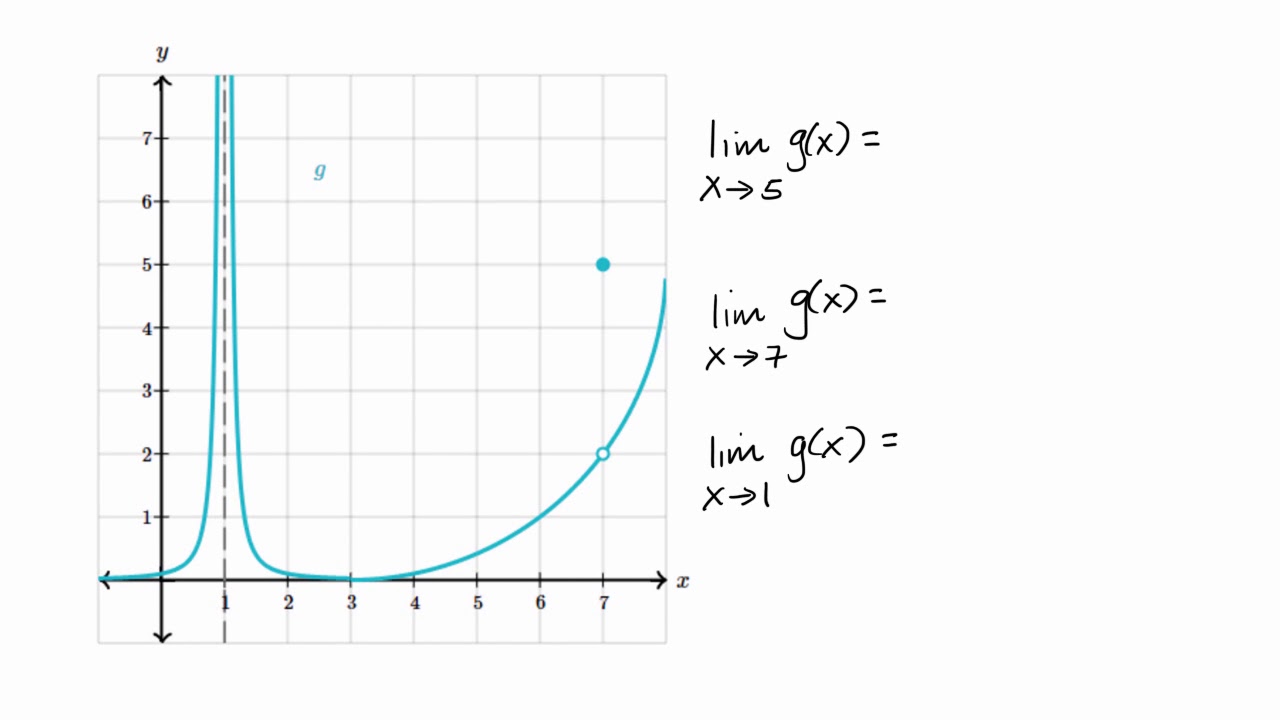
- 📉 When approaching -4 from the left, the function value approaches 4.
- 📈 When approaching -4 from the right, the function value approaches -2.
- ❌ If left-hand and right-hand limits are not equal, the general limit does not exist.
- 0️⃣ At x = 0, if both left and right limits approach 2, the limit exists and equals 2.
- ↔️ For x = -6, if there is no value to the left, the limit does not exist.
- ⚫ Approaching 2 from the right results in a value of 0, regardless of any hole or discontinuity.
- ♾️ For asymptotes, the direction of the function (e.g., approaching infinity) is noted, even though technically the limit does not exist.
Q & A
What is the significance of the notation \( g(x) \) in the context of this lesson?
-The notation \( g(x) \) represents a function whose limits are being analyzed as \( x \) approaches different values from the left and right.
What does the little negative sign (superscript) indicate in limit notation?
-The little negative sign indicates that the limit is being approached from the left side.
What is the value that \( g(x) \) approaches as \( x \) approaches -4 from the left?
-As \( x \) approaches -4 from the left, \( g(x) \) approaches the value 4.
Why can't we use non-standard notation for limits, such as approaching \( x \) from the left without the negative sign?
-Non-standard notation should be avoided because it may not be understood by others; standard notation ensures clarity and consistency.
What is the value that \( g(x) \) approaches as \( x \) approaches -4 from the right?
-As \( x \) approaches -4 from the right, \( g(x) \) approaches the value -2.
Why does the general limit of \( g(x) \) as \( x \) approaches -4 not exist?
-The general limit does not exist because the values approached from the left and the right are not the same.
Can limits occur at points of continuity, such as \( x = 0 \) in this lesson?
-Yes, limits can occur at points of continuity. In the lesson, as \( x \) approaches 0, \( g(x) \) approaches the value 2, which is continuous at that point.
What happens to \( g(x) \) as \( x \) approaches -6 from the right, given that there's no function on the left?
-As \( x \) approaches -6 from the right, \( g(x) \) approaches the value 0. Since there is no function on the left, it’s not possible to approach from the left, and the limit does not exist.
What is the value that \( g(x) \) approaches as \( x \) approaches 2 from the right, even if there’s a hole or a point?
-As \( x \) approaches 2 from the right, \( g(x) \) approaches the value 0, irrespective of holes or points.
How do we interpret the behavior of \( g(x) \) as \( x \) approaches 4 with an asymptote?
-As \( x \) approaches 4 from the right, \( g(x) \) goes to infinity, and as it approaches 4 from the left, it goes to negative infinity. Since they go in different directions, the limit does not exist.
Outlines
📚 Understanding Limits and One-Sided Limits
The first paragraph introduces the concept of limits in calculus, specifically focusing on the function labeled as 'g of x'. The explanation covers how to determine the y-value a function approaches as x approaches various values, including both left-hand and right-hand limits. The importance of notation is emphasized, explaining the difference between approaching a value from the left and from the right. The paragraph also discusses the conditions under which a general limit does or does not exist, using the function's behavior at specific points as examples. It clarifies that limits do not always occur at discontinuities and can approach different values from each side, indicating the non-existence of a general limit in such cases.
🔍 Analyzing Directional Behavior Near Asymptotes
The second paragraph delves into the behavior of the function near asymptotes and points of discontinuity. It discusses how to interpret the direction of the function's approach to certain values, such as infinity or negative infinity, and the importance of this direction in determining the limit. The paragraph provides examples of how to identify and express the limit when the function approaches a value from both sides, emphasizing that the direction of approach can be more specific than simply stating 'does not exist'. It also touches on the technicality that infinity is not a value but rather a direction, and how this is often simplified in practice for the sake of clarity.
Mindmap
Keywords
💡Function
💡Limit
💡Left-hand limit
💡Right-hand limit
💡Discontinuity
💡General limit
💡Infinity
💡Asymptote
💡Notation
💡Approaching
Highlights
Introduction to limits, including left-hand and right-hand limits.
Explanation of approaching x-values from the left and right, and how the corresponding y-value is determined.
Clarification of standard notation in calculus for expressing limits.
Example of finding the limit as x approaches -4 from the left, with the result being 4.
Illustration of the difference between left-hand and right-hand limits, and how they can lead to different y-values.
Discussion on general limits and when they do not exist, particularly when left-hand and right-hand limits do not match.
Explanation of limits at discontinuities and the misconception that limits always occur at these points.
Example of finding the limit as x approaches 0, where the limit is equal to 2 despite there being no discontinuity.
Determination of the limit as x approaches -6 from the right, resulting in a limit of 0.
Clarification that if there is no graph to the left of a point, the limit from the left does not exist.
Discussion on why limits must approach the same value from both the left and right for the limit to exist.
Example of a limit as x approaches 2 from the right, resulting in a limit of 0 even though there is a hole in the graph.
Explanation of asymptotic behavior and how limits are expressed when approaching infinity.
Clarification that infinity is not an actual value, but often used to indicate the direction a graph is heading.
Example of how a limit might not exist if the graph approaches different infinities (positive vs. negative) from different directions.
Transcripts
Browse More Related Video

Limits! Part 1

One-sided limits from tables | Limits and continuity | AP Calculus AB | Khan Academy
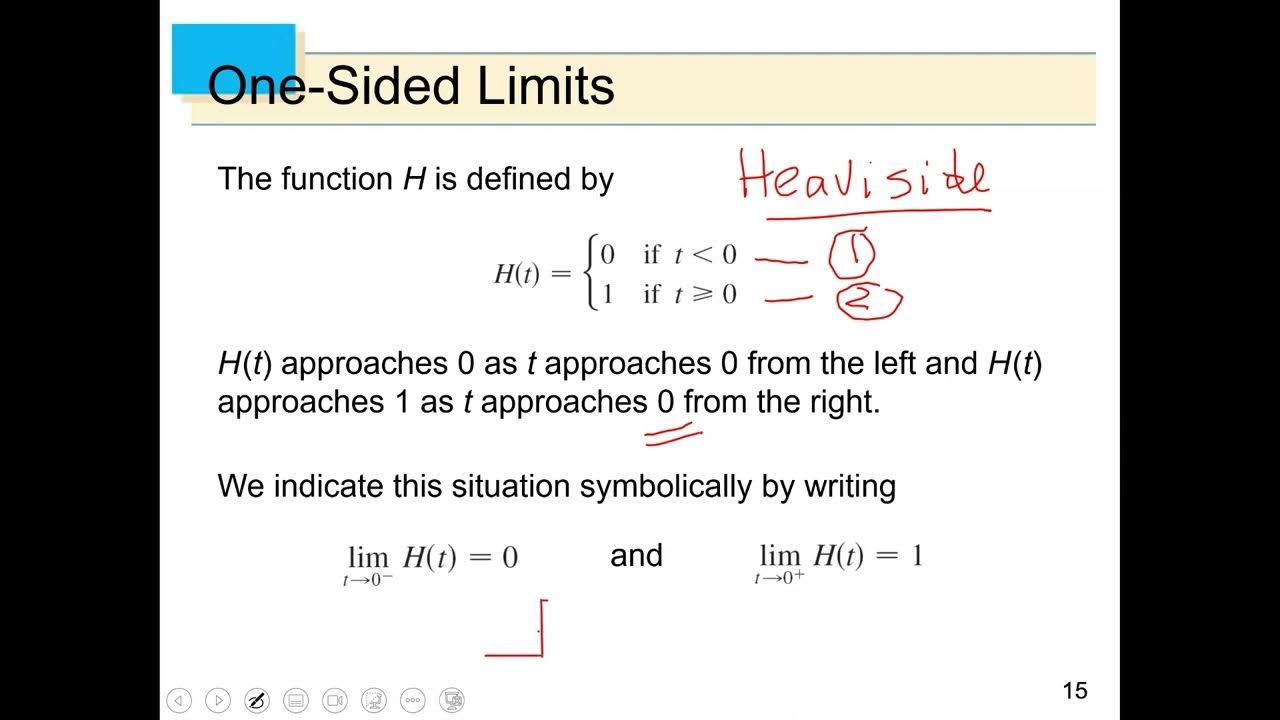
Limits from graphs | Limits and continuity | AP Calculus AB | Khan Academy
Calculus Math 133 - Lecture 2.2
How To Evaluate Limits From a Graph

Worked example: Continuity at a point | Limits and continuity | AP Calculus AB | Khan Academy
5.0 / 5 (0 votes)
Thanks for rating: