Graph of r = 1 + cos(theta/2) | MIT 18.01SC Single Variable Calculus, Fall 2010
TLDRIn this educational video, the instructor guides viewers through graphing the polar curve r = 1 + cos(θ/2) over the range 0 to 4π. They first plot the curve in an r-θ plane to understand its behavior before translating it into the Cartesian xy-plane. The focus then shifts to calculating areas of specific regions within the graph, emphasizing the importance of correctly identifying bounds and using symmetry to simplify integration for area calculations.
Takeaways
- 📚 The video is a recitation session focused on graphing the polar curve \( r = 1 + \cos(\theta / 2) \) over the range \( \theta \) from 0 to \( 4\pi \) and calculating areas of certain components in the xy-plane.
- 📈 The instructor emphasizes understanding the relationship between \( r \) and \( \theta \) as the main goal of graphing the curve.
- 🔍 Initially, the curve is graphed in an \( r, \theta \) plane to simplify understanding before translating it to the xy-plane.
- 🌀 The transformation applied to the cosine function includes stretching it horizontally by a factor of 2 and shifting it upwards by 1 unit.
- 📉 The period of the cosine function is doubled to \( 4\pi \) due to the division by 2 in the argument of the cosine function.
- 📍 The curve starts and ends at a height of 2 in the \( r, \theta \) plane, and it only reaches down to 0 instead of the usual -1 due to the +1 addition.
- 🤔 The instructor encourages students to pause the video to visualize the curve before continuing with the graphing process.
- 📝 The instructor explains the process of translating points from the \( r, \theta \) plane to the xy-plane, highlighting the periodic nature and specific points like (2, 0).
- 🔄 The symmetry of the curve is used to simplify the graphing process, as drawing half of the curve allows for the completion by reflection.
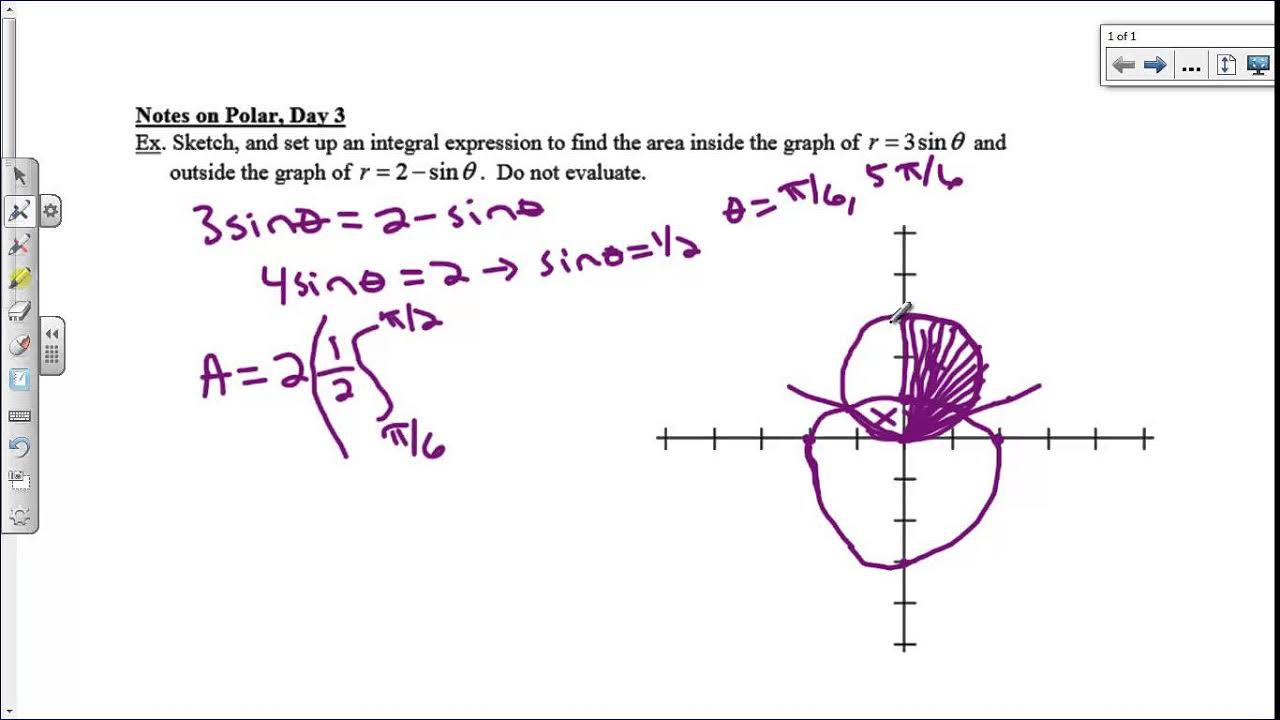
- 🌐 The instructor discusses the process of finding areas of regions in the curve, emphasizing the importance of correct bounds in theta for the integrals.
- ✂️ To find the area of the blue region, the instructor explains that one must calculate the total area from 0 to \( 4\pi \) and then subtract the area of the pink region to avoid double counting.
Q & A
What is the main goal of the video?
-The main goal of the video is to graph the curve r = 1 + cos(θ/2) for θ between 0 and 4π in the xy-plane and then calculate the area of some components of that curve.
What is the first step the instructor asks the students to do?
-The first step the instructor asks the students to do is to get a good picture of the curve in the xy-plane and pause the video to do so.
How does the instructor initially approach the problem of graphing the curve?
-The instructor initially approaches the problem by looking at the curve in the r, θ plane, treating r as a function of θ, before translating it to the xy-plane.
What transformation is applied to the normal cosine function in the given curve equation?
-The transformation applied to the normal cosine function is a horizontal stretch by a factor of 2 and a vertical shift up by 1, resulting in the equation r = 1 + cos(θ/2).
Why does the period of the cosine function change when θ is divided by 2?
-Dividing θ by 2 in the cosine function doubles the period from 2π to 4π because the input value for θ that would normally complete one cycle of the cosine function now only completes half a cycle.
How does the instructor describe the starting and ending points of the curve in the r, θ plane?
-The instructor describes the starting point as r = 2 when θ = 0 and the ending point also as r = 2 when θ = 4π, indicating the periodic nature of the curve.
What is the significance of the point (2, 0) in the xy-plane in relation to the curve?
-The point (2, 0) in the xy-plane is significant because it represents the starting and ending point of the curve when θ is 0, 2π, and 4π, showing a closed loop.
How does the instructor suggest finding the area of the pink region in the xy-plane?
-The instructor suggests finding the area of the pink region by integrating the square of r, which is (1 + cos(θ/2))^2, with respect to θ over the bounds of the pink region.
What is the strategy for calculating the area of the blue region, according to the instructor?
-The strategy for calculating the area of the blue region is to find the total area from 0 to π, subtract the area of the pink region from π to 3π, and then double the result due to symmetry.
Why is it necessary to subtract the area of the pink region when calculating the area of the blue region?
-It is necessary to subtract the area of the pink region because the blue region excludes the pink area, and the integral from 0 to π would include the pink area if not accounted for.
What mathematical concept is highlighted in the video for calculating areas in polar coordinates?
-The mathematical concept highlighted is that dA = (r^2)/2 dθ, which is used to calculate the area in polar coordinates by integrating over the appropriate bounds.
Outlines
📚 Introduction to Graphing the Curve r = 1 + cos(θ/2)
In this segment, Christine Breiner introduces the task of graphing the polar curve r = 1 + cos(θ/2) over the range of θ from 0 to 4π in the xy-plane. The goal is to understand the relationship between r and θ, and to visualize the curve before moving on to calculate the area of certain components. She emphasizes the importance of graphing in the r-θ plane first to grasp the relationship between r and θ, and then translating it into the xy-plane. Key points include the horizontal stretching of the cosine function by a factor of 2, which doubles its period, and the vertical shift upwards by 1, which changes the range of the function from -1 to 1 to 0 to 2.
📈 Translating the r-θ Graph to the xy-Plane
Christine explains the process of translating the graph from the r-θ plane to the xy-plane. She discusses the periodic nature of the curve and how the r-value changes as θ rotates from 0 to 2π and then to 4π. The symmetry of the curve is highlighted, and the points where the curve intersects itself are identified. The explanation includes the transformation of the curve as θ varies, and how to determine the corresponding x and y coordinates in the xy-plane. The importance of understanding the symmetry and periodicity of the curve is emphasized to assist in graphing and later calculating areas.
🔍 Calculating Areas with Integrals in Polar Coordinates
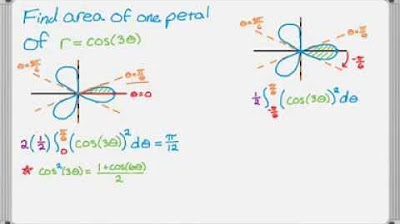
This paragraph delves into the calculation of areas in polar coordinates, focusing on the integral form dA = (r^2)/2 dθ. Christine illustrates how to find the area of the pink region by squaring the r function and integrating over the appropriate bounds of θ. She also discusses the strategy for integrating the terms that arise, including using substitution and trigonometric identities. The symmetry of the problem is noted, allowing for the area calculation from one segment to be doubled to find the total area of interest.
📐 Dealing with Symmetry and Subtracting Areas in the xy-Plane
Christine concludes the video script by addressing the complexity of calculating the area of the blue region, which involves subtracting the area of the pink region from the total area under the curve. She explains the importance of correctly identifying the bounds of θ for each region and the need to subtract the inner area to find the area of the blue region. The summary emphasizes the method of calculating the total area and then removing the overlapping area to isolate the desired region. The concept of symmetry is again highlighted as a tool to simplify the calculation process.
Mindmap
Keywords
💡Recitation
💡Graphing
💡Curve
💡Polar Coordinates
💡Theta (θ)
💡Cosine Function
💡Period
💡Area Calculation
💡Integral
💡Symmetry
💡U-Substitution
💡Double Angle Formula
Highlights
Introduction to the task of graphing the curve r = 1 + cos(θ)/2 in the xy-plane for θ between 0 and 4π.
The importance of understanding how r depends on θ for the graphing process.
Initial approach to graph the equation in the r, θ plane before translating to the xy-plane.
Explanation of the transformation applied to the cosine function by dividing the input value by 2, effectively doubling the period.
Graphical representation of the curve in the r, θ plane, emphasizing the stretch and shift transformations.
Transition from the r, θ plane to the xy-plane by identifying points and their coordinates.
Identification of symmetry in the curve and its implications for graphing and area calculations.
Method to calculate the area of the pink region using the integral form and bounds on theta.
Use of symmetry to simplify the calculation of the area by considering the region from pi to 2π and multiplying by 2.
Integration strategy for the area calculation involving r squared and the application of trigonometric identities.
Complexity in calculating the blue area by subtracting the pink area from the total area obtained from 0 to π.
The necessity to account for symmetry and the exclusion of overlapping regions when determining areas.
Final visualization of the curve in the xy-plane with emphasis on the correct interpretation of the r and θ relationship.
Summary of the process for graphing and area calculation, highlighting the importance of understanding the curve's behavior in the xy-plane.
Emphasis on the careful selection of integral bounds to avoid double-counting areas in the calculation.
Conclusion that highlights the importance of understanding the curve's symmetry and the correct application of integrals for area calculation.
Transcripts
5.0 / 5 (0 votes)
Thanks for rating: