AP Precalculus Practice Exam Question 28

NumWorks
23 May 202307:10
EducationalLearning
32 Likes 10 Comments

TLDRIn this educational video, the presenter explores the polar function R = f(θ) = 1 + 2sin(θ) within the range 0 ≤ θ ≤ 2π. They create a table of values for θ and plot the graph, revealing a spiral pattern. The presenter then solves for when the radius is zero, finding the points where the graph touches the pole at 7π/6 and 11π/6. The video concludes by evaluating statements about the distance between the graph and the origin, with the correct answer being that the distance increases from zero to 2π, as R is positive and increasing on this interval.

Takeaways
  • 📚 The video discusses the polar graph of a function \( R = f(\theta) \) where \( f(\theta) = 1 + 2\sin(\theta) \) within the range \( 0 \leq \theta \leq 2\pi \).
  • 📝 The presenter begins by creating a table of values for \( \theta \) and \( R \) to sketch the graph, starting from \( \theta = 0 \) to \( 2\pi \).
  • 📈 At \( \theta = 0 \), the value of \( R \) is 1, indicating a point on the polar graph at a distance of 1 from the origin.
  • 📉 The graph's behavior is analyzed at specific angles, including \( \pi/2 \), \( \pi \), and \( 3\pi/2 \), revealing increases and decreases in the radius.
  • 🔍 The presenter identifies that the graph will hit the pole at certain angles, specifically between \( \pi \) and \( 3\pi/2 \), and then from \( 3\pi/2 \) to \( 2\pi \).
  • 🧩 The solution to \( 1 + 2\sin(\theta) = 0 \) is found to be at \( \theta = 7\pi/6 \) and \( 11\pi/6 \), indicating points where the graph touches the pole.
  • 📊 The graph is described as having a spiral motion, with specific points analyzed for their distance from the origin.
  • 🔎 The presenter evaluates several statements about the distance between the point with polar coordinate \( (f(\theta), \theta) \) and the origin.
  • ✅ Statement A is identified as true, suggesting the distance increases from 0 to \( 2\pi \) because \( f(\theta) \) is positive and increasing on the interval.
  • ❌ Statement B is refuted as it suggests the distance increases from \( 3\pi/2 \) to \( 11\pi/6 \), which is incorrect since the distance is decreasing.
  • ❌ Statement C is also incorrect, as it contradicts the increasing distance from 0 to \( 2\pi \) identified in Statement A.
  • ❌ Statement D is incorrect because it suggests the distance decreases from \( 3\pi/2 \) to \( 11\pi/6 \), but the presenter's analysis shows an increase.
Q & A

  • What is the polar function given in the script?

    -The polar function given is R = f(θ) = 1 + 2sin(θ).

  • What is the range of θ for the polar function?

    -The range of θ is from 0 to 2π.

  • What is the initial value of R when θ is 0?

    -When θ is 0, the value of R is 1 because sin(0) is 0, so R = 1 + 2(0) = 1.

  • What happens to the radius R when θ is π?

    -When θ is π, sin(π) is 0, so the radius R remains 1, as R = 1 + 2(0) = 1.

  • At what angle does the radius R become negative?

    -The radius R becomes negative when θ is 3π/2, because sin(3π/2) is -1, making R = 1 + 2(-1) = -1.

  • What is the significance of the negative radius in the polar plot?

    -A negative radius indicates that the point is located on the positive y-axis, as the radius is the distance from the origin, and a negative value suggests a reflection across the x-axis.

  • What are the θ values where the curve hits the pole (R = 0)?

    -The curve hits the pole at θ values of 7π/6 and 11π/6, where sin(θ) equals -1/2.

  • Why does the curve not complete a full cycle at 2π according to the script?

    -The curve does not complete a full cycle at 2π because the function has points where R = 0 (at 7π/6 and 11π/6), which interrupts the continuity of the spiral motion.

  • What is the correct statement about the distance between the point with polar coordinate (R, θ) and the origin?

    -Statement A is correct: The distance is increasing from 0 to 2π because R is positive and increases on the interval.

  • Why is statement B incorrect according to the script?

    -Statement B is incorrect because the distance is not increasing from 3π/2 to 11π/6; it is actually decreasing as the radius goes from -1 to 0.

  • What makes statement C incorrect?

    -Statement C is the contradiction of A, claiming the distance is decreasing from 0 to 2π, which is false as previously established that the distance is increasing.

  • Why is statement D also incorrect?

    -Statement D is incorrect because, although the radius is negative, the distance to the origin is not decreasing but increasing as the radius approaches zero from a negative value.

Outlines
00:00
📚 Analyzing the Polar Function Graph

The speaker begins by addressing a question about the polar function R = 1 + 2sin(θ), with θ ranging from 0 to 2π. The approach involves sketching the graph by creating a table of values for θ and calculating the corresponding R values. The process includes plotting these points on a polar coordinate system to visualize the spiral-like pattern. The speaker identifies critical points where the graph touches the pole at specific angles, determined by setting the function equal to zero and solving for θ. This results in finding angles 7π/6 and 11π/6 where the radius is zero, indicating the points of intersection with the pole.

05:01
🔍 Evaluating Statements on Distance Variation

The speaker proceeds to evaluate several statements regarding the distance between points on the polar curve and the origin. Statement A suggests that the distance is increasing from 0 to 2π, which the speaker initially agrees with, based on the observed increase in radius values. Statement B claims the distance increases from 3π/2 to 11π/6, which is refuted as the radius decreases, indicating the distance to the origin is getting smaller. Statement C, the contradiction of A, is dismissed as false since the distance was established to be increasing. Statement D asserts the distance decreases from 3π/2 to 11π/6, which the speaker corrects, noting that the negative radius values are actually approaching zero, signifying an increase in distance, not a decrease. After the analysis, the speaker concludes that the only true statement is A, confirming the initial observation of increasing distance along the curve.

Mindmap
Keywords
💡Polar Function
A polar function is a mathematical representation of a relationship between a radius (R) and an angle (theta) in a polar coordinate system. In the video, the polar function R = f(theta) = 1 + 2sin(theta) is used to describe the graph of a curve. The function is central to the video's theme as it dictates the shape and behavior of the graph being analyzed.
💡Polar Coordinates
Polar coordinates are a two-dimensional coordinate system where each point is determined by a radius from a fixed origin and an angle from a fixed direction. In the context of the video, the polar coordinate system is used to plot the graph of the given polar function, illustrating the points' positions relative to the origin.
💡Theta (θ)
Theta, often denoted by the symbol θ, is the angle in a polar coordinate system, measured from a fixed reference direction to the line segment connecting the origin to the point. In the video, theta is the variable input for the polar function, and its values range from 0 to 2π, determining the position of points on the graph.
💡Sine Function
The sine function is a trigonometric function that relates the ratio of the opposite side to the hypotenuse in a right-angled triangle. In the video, the sine function is used within the polar function to create a wave-like pattern in the graph, with 'sin(theta)' contributing to the variation of the radius based on the angle theta.
💡Graph Sketching
Graph sketching is the process of visually representing a mathematical function or equation. The video involves sketching the graph of the polar function by creating a table of values and plotting them on a polar coordinate grid, which helps in understanding the shape and behavior of the curve.
💡Radius (R)
In polar coordinates, the radius (R) is the distance from the origin to a point on the graph. In the video, the radius is given by the polar function R = f(theta), and its values are calculated for different theta values to plot the graph, showing how the distance from the origin changes with the angle.
💡Unit Circle
A unit circle is a circle with a radius of one, used in trigonometry to define the sine and cosine functions. The video refers to the unit circle when determining the values of sine for specific angles, such as 7π/6 and 11π/6, which are crucial for finding points where the graph intersects the pole.
💡Pole
In the context of polar coordinates, the pole is the origin or the fixed point from which all other points are measured. The video discusses the pole in relation to the points where the graph intersects the origin, indicating a radius of zero.
💡Increasing Function
An increasing function is one where the output value increases as the input value increases. In the video, the statement 'the distance is increasing from zero to two Pi' refers to the behavior of the radius as theta increases, indicating that the points on the graph are moving further away from the origin.
💡Decreasing Function
A decreasing function is the opposite of an increasing function, where the output value decreases as the input value increases. The video evaluates the statement 'the distance is decreasing from 3π/2 to 11π/6' to determine if the radius values are getting smaller as theta increases, which is a key aspect of analyzing the graph's behavior.
💡Trigonometric Values
Trigonometric values are the ratios of the sides of a right-angled triangle that define the sine, cosine, and tangent functions. The video uses specific trigonometric values, such as sine of π/2 being 1 and sine of 3π/2 being -1, to calculate the radius at different angles and to plot the graph accurately.
Highlights

Introduction to the polar function R = f(θ) with f(θ) = 1 + 2sin(θ).

Exploration of the graph by creating a table of values for θ from 0 to 2π.

Calculation of R values at specific angles: 0, π/2, π, and 3π/2.

Observation of a spiral-like motion in the graph's plot.

Identification of the polar curve's pole-hitting points between π and 3π/2.

Solution for when the radius is zero by setting 1 + 2sin(θ) = 0.

Inclusion of θ values 7π/6 and 11π/6 where the curve hits the pole.

Analysis of the distance between the point with polar coordinate (f(θ), θ) and the origin.

Statement A: The distance increases from 0 to 2π due to positive and increasing R values.

Statement B: Incorrect, as the distance decreases from 3π/2 to 11π/6, not increasing.

Statement C: Contradiction of A, claiming the distance decreases from 0 to 2π, which is false.

Statement D: Incorrect, as the distance is not decreasing but increasing from 3π/2 to 11π/6.

Conclusion that the only true statement is A, confirming the increasing distance.

Discussion of the significance of positive and negative R values in the context of the graph.

Explanation of how the graph's spiral pattern affects the perceived distance from the origin.

Final summary emphasizing the importance of understanding polar functions and their graphical behavior.

Transcripts

Browse More Related Video

AP Precalculus Practice Exam Question 25

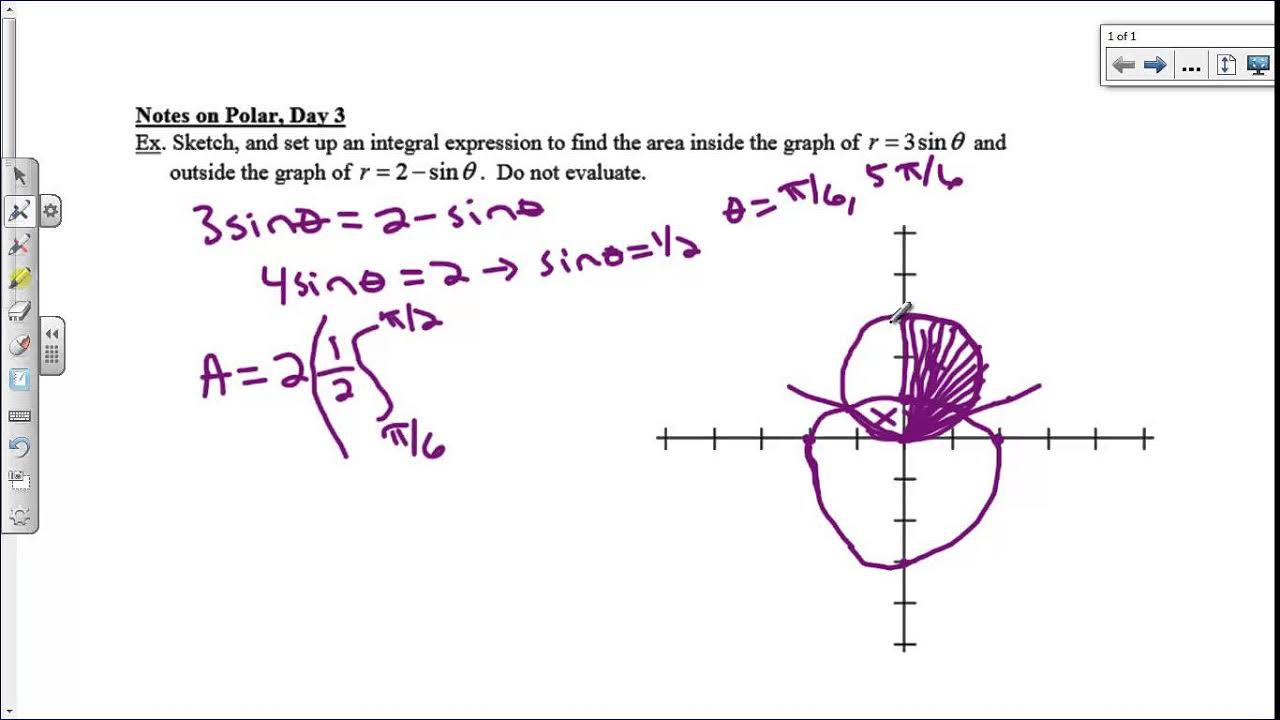
Notes on Polar, Day 3

Graph of r = 1 + cos(theta/2) | MIT 18.01SC Single Variable Calculus, Fall 2010

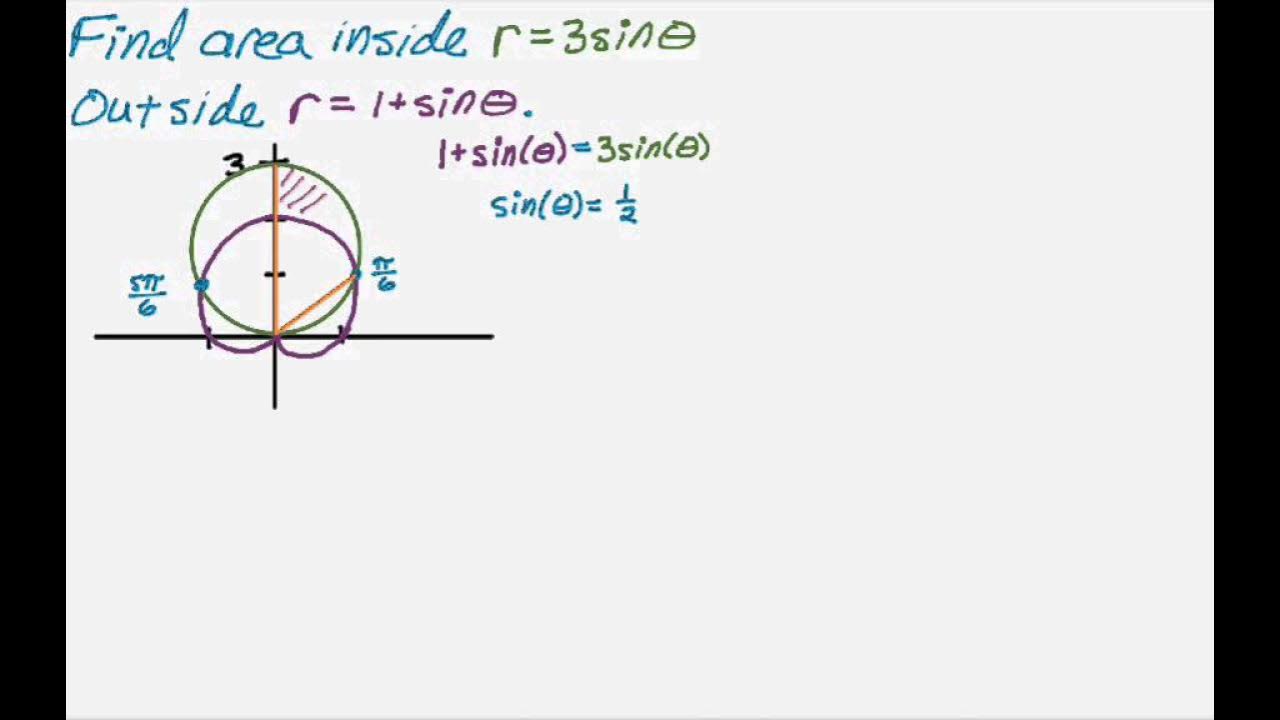
Calculus Polar Area Region Between Curves Example

How To Graph Polar Equations

Polar coordinates 2 | Parametric equations and polar coordinates | Precalculus | Khan Academy

Rate This

5.0 / 5 (0 votes)

Thanks for rating:

Related Tags
Polar GraphMathematicsTrigonometryDistance AnalysisTheta ValuesGraph SketchingSine FunctionRadius CalculationPolar CoordinatesEducational Content