Unit III: Lec 1 | MIT Calculus Revisited: Single Variable Calculus
TLDRThis MIT OpenCourseWare lecture delves into circular functions, offering an alternative perspective on trigonometry without the traditional focus on triangles. The professor redefines sine and cosine in terms of real numbers, using the unit circle, and clarifies the use of radians to avoid ambiguity between angles and lengths. The lecture also explores the calculus of these functions, deriving their derivatives and integrals, and connects circular functions to physical phenomena like simple harmonic motion, showcasing their relevance beyond geometry.
Takeaways
- 📚 The lecture introduces the concept of 'circular functions', which are an alternative way to understand trigonometric functions without the need for triangles.
- 🔍 The professor emphasizes the importance of understanding trigonometry beyond its traditional applications, such as in calculus and physics, where it becomes essential.
- 🤔 The lecture addresses a common concern about the relevance of trigonometry, explaining its broader applications beyond just surveying.
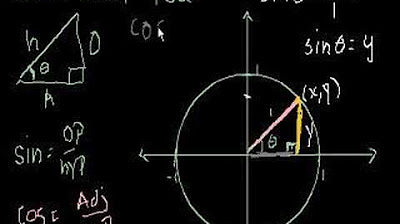
- 📐 The connection between circular functions and traditional trigonometry is established through the unit circle with a radius of 1, allowing for a new perspective on sine and cosine.
- 📈 The sine and cosine functions are redefined in terms of real numbers, mapping inputs and outputs to the real number line, rather than angles.
- 📉 The process of defining the sine and cosine of a real number 't' involves laying off 't' along the circumference of the unit circle and dropping a perpendicular to define the sine as the y-coordinate and cosine as the x-coordinate.
- 🔢 The fundamental trigonometric identity 'sine squared t' plus 'cosine squared t' equals 1 is derived from the Pythagorean theorem applied to the unit circle.
- 🔄 The concept of radian measure is introduced to eliminate ambiguity between the angle and the arc length on the unit circle, aligning the numerical value of the angle with the length of the arc.
- 🔄 The lecture explores the idea of defining trigonometric functions using different geometric figures, such as hyperbolas, to demonstrate the versatility of this approach.
- 📚 The importance of understanding the limits of 'sine t' over 't' as 't' approaches 0, and '1 - cosine t' over 't' as 't' approaches 0, is highlighted for the calculus of trigonometric functions.
- 🔧 The application of basic calculus rules to the newly defined circular functions is demonstrated, showing that once the foundational limits are understood, the derivative and integral of these functions can be found using standard calculus techniques.
Q & A
What is the main topic of the lecture?
-The main topic of the lecture is 'Circular Functions,' which is essentially a discussion on trigonometry without triangles, focusing on the connection between circular functions and traditional trigonometric functions.
Why did the professor initially take the trigonometry course lightly?
-The professor initially took the trigonometry course lightly because his high school teacher mentioned that surveyors use trigonometry, and he knew he wasn't going to be a surveyor. He later realized its importance when he encountered it in calculus and physics courses.
What is the significance of defining trigonometric functions in terms of real numbers rather than angles?
-Defining trigonometric functions in terms of real numbers allows for a more general and flexible application of these functions in various mathematical and physical contexts, including calculus, where the domain and range are sets of real numbers.
How does the professor visualize the definition of the sine function for a real number 't'?
-The professor visualizes the definition of the sine function by considering 't' as a length along the circumference of a unit circle. The sine of 't' is then defined as the y-coordinate of the point where the length 't' terminates on the circle.
What is the fundamental trigonometric identity that the professor mentions?
-The fundamental trigonometric identity mentioned by the professor is 'sine squared t' plus 'cosine squared t' equals 1, which is derived from the Pythagorean theorem applied to the unit circle.
Why is the radian measure of angles important in the context of circular functions?
-The radian measure is important because it allows for an unambiguous identification between the angle 't' and the length 't' on the unit circle, simplifying the expression of trigonometric functions and their properties.
What is the physical significance of the circular functions in the context of simple harmonic motion?
-In the context of simple harmonic motion, the circular functions describe the motion of a particle moving along the x-axis according to the rule 'x' equals 'sine kt', where 'k' is a constant and 't' is time. The acceleration of the particle is proportional to the displacement but in the opposite direction.
How does the professor explain the relationship between the sine of an angle and the sine of a real number?
-The professor explains that when using radians to measure angles, the sine of an angle 't' radians is numerically the same as the sine of the real number 't', eliminating any ambiguity between the two.
What is the derivative of the sine function with respect to a real number 'x'?
-The derivative of the sine function with respect to a real number 'x' is the cosine function, as shown by the professor through the process of differentiation and applying the limit theorems.
How does the professor use the chain rule to find the derivative of 'cosine x'?
-The professor uses the chain rule by expressing 'cosine x' as sine (pi/2 - x) and differentiating it with respect to 'x'. The derivative of 'cosine x' is then found to be minus the sine of 'x'.
What is the significance of the limits discussed in the lecture for the calculus of trigonometric functions?
-The limits discussed in the lecture, such as the limit of 'sine t' over 't' as 't' approaches 0, are crucial for finding the derivatives of trigonometric functions. These limits allow for the application of the basic rules of differentiation to the new context of circular functions.
Outlines
📚 Introduction to Circular Functions and Trigonometry
The professor begins the lecture by addressing the importance of understanding circular functions, which are essentially trigonometric functions without the need for triangles. He shares a personal anecdote about his initial skepticism towards the practicality of trigonometry, which was dispelled when he encountered it in calculus and physics. The lecture aims to explore the connection between circular functions and traditional trigonometry, emphasizing the relevance of these mathematical tools beyond surveying. The concept is introduced through the visualization of a circle with a radius of 1, laying the groundwork for defining sine and cosine in terms of real numbers rather than angles.
📐 Defining Sine and Cosine in the Context of a Circle
The professor explains how to define sine and cosine functions using a unit circle. By considering a real number 't' as a length along the circle's circumference, the sine of 't' is defined as the y-coordinate of the termination point 'P', and the cosine as the x-coordinate. This method allows for the trigonometric functions to map real numbers to real numbers, independent of angles. The explanation includes the traditional trigonometric conventions and how they translate into this new framework, including the definitions of sine and cosine for positive and negative values of 't'.
🔍 Exploring the Concept of Radian Measure
The lecture delves into the concept of radian measure, addressing the ambiguity that arises when using degrees versus radians in trigonometry. The professor illustrates how defining an angle in radians—where the angle's measure is equal to the length of the arc it subtends on a unit circle—eliminates confusion between the sine of an angle and the sine of a real number. This section also touches on the historical and mathematical reasons for adopting radians as the standard measure in trigonometry, emphasizing the clarity it brings to the definitions of trigonometric functions.
🌐 Alternative Geometric Interpretations of Trigonometric Functions
The professor discusses the flexibility in defining trigonometric-like functions using different geometric figures, such as hyperbolas, and how these definitions can vary based on the chosen method of measuring lengths along the figure. He also considers alternative ways of defining trigonometric functions on a circle, such as using radii of different lengths, and the implications these choices have on the domain of the functions. The section highlights the arbitrariness in the choice of the circle for defining trigonometric functions and the importance of careful definition to avoid ambiguity.
🔄 The Derivative of Sine and Cosine Functions
The lecture moves into the realm of calculus, specifically the computation of derivatives of sine and cosine functions. The professor outlines the process of finding the derivative of sine with respect to a real variable 'x', using the definition of the derivative and the properties of trigonometric functions. The section covers the computation of limits necessary for the derivative, such as the limit of 'sine t' over 't' as 't' approaches 0, and how these computations lead to the conclusion that the derivative of sine is cosine.
📉 Deriving the Derivative of Cosine and Its Physical Interpretation
Building on the previous discussion, the professor derives the derivative of the cosine function and touches on the physical interpretation of these derivatives in the context of simple harmonic motion. The lecture demonstrates how the second derivative of a particle's displacement in simple harmonic motion, which represents acceleration, is proportional and opposite to the displacement itself. This section reinforces the idea that trigonometric functions can be understood and applied without reference to angles, highlighting their utility in physics and engineering.
🔚 Conclusion and Preview of Future Topics
In the concluding part of the lecture, the professor summarizes the key points covered in the session, emphasizing the importance of understanding the calculus of circular functions and their physical applications. He also previews the next topic, which will be the inverse circular functions and their significance. The lecture ends with acknowledgment of the funding provided by the Gabriella and Paul Rosenbaum Foundation for the publication of the video, and a call to action for donations to support MIT OpenCourseWare.
Mindmap
Keywords
💡Circular Functions
💡Trigonometry
💡Unit Circle
💡Radians
💡Sine Function
💡Cosine Function
💡Tangent Function
💡Derivative
💡Chain Rule
💡Simple Harmonic Motion
Highlights
Introduction of circular functions as an alternative to traditional trigonometry, emphasizing their relevance in calculus and physics.
The concept of defining trigonometric functions without the need for angles, using the unit circle with a radius of 1.
Visual representation of sine and cosine as lengths on the unit circle, rather than angles, simplifying the understanding of these functions.
The explanation of how to define sine and cosine for any real number 't', mapping real numbers to real numbers.
The derivation of the tangent function as the ratio of sine to cosine, extending the concept to non-traditional inputs.
The clarification of the sine and cosine functions' behavior at specific points, such as sine of 0 being 0.
The fundamental trigonometric identity sine squared t plus cosine squared t equals 1, derived from the unit circle properties.
Graphical representation of the sine function's curve, illustrating its periodic nature and maximum/minimum values.
The invention of the radian measure to eliminate ambiguity between angle measures and the values of trigonometric functions.
The explanation of how radians allow for the sine of an angle to be numerically the same as the sine of a length.
The potential alternative to circular functions, such as hyperbolic functions, demonstrating the flexibility in defining mathematical functions.
The physical interpretation of circular functions in the context of simple harmonic motion, showing their practical applications.
The demonstration of how to derive the second derivative of a function, specifically for simple harmonic motion, using circular functions.
The connection between the physical problem of simple harmonic motion and the mathematical functions derived from the unit circle.
The application of the chain rule in calculus to find the derivative of composite functions involving sine and cosine.
The derivation of the integral formulas for sine and cosine functions, showcasing the inverse process of differentiation.
The预告 of the next lecture on inverse circular functions, indicating the continuation of the topic and its importance.
Transcripts
Browse More Related Video
Unit Circle Definition of Trig Functions

Trigonometry fundamentals | Ep. 2 Lockdown live math

Trigonometry: Unit Circle

Trigonometric Functions of Any Angle - Unit Circle, Radians, Degrees, Coterminal & Reference Angles
Hyperbolic trig functions | MIT 18.01SC Single Variable Calculus, Fall 2010
Calculus Chapter 1 Lecture 3 Taylor Series
5.0 / 5 (0 votes)
Thanks for rating: