Calculus Chapter 5 Lecture 53 Absolute & Conditional
TLDRIn this calculus lecture, Professor Greist introduces the concepts of absolute and conditional convergence in the context of infinite series. He explains that while some series are straightforward to determine as convergent or divergent, others require more nuanced tests. The lecture focuses on alternating series, which are easier to analyze due to their alternating signs. The professor presents the alternating series test, which simplifies the convergence assessment under certain conditions. He also discusses the implications of absolute and conditional convergence, noting that while absolutely convergent series are robust and can be rearranged without affecting their sum, conditionally convergent series are more delicate and can lead to different sums when terms are rearranged. The lecture concludes with a cautionary note on the potential for rearranging conditionally convergent series to yield any desired sum.
Takeaways
- 📚 The lecture introduces the concepts of absolute and conditional convergence in the context of infinite series.
- 🔍 The distinction between the two types of convergence is that absolute convergence requires the sum of the absolute values of terms to converge, while conditional convergence does not.
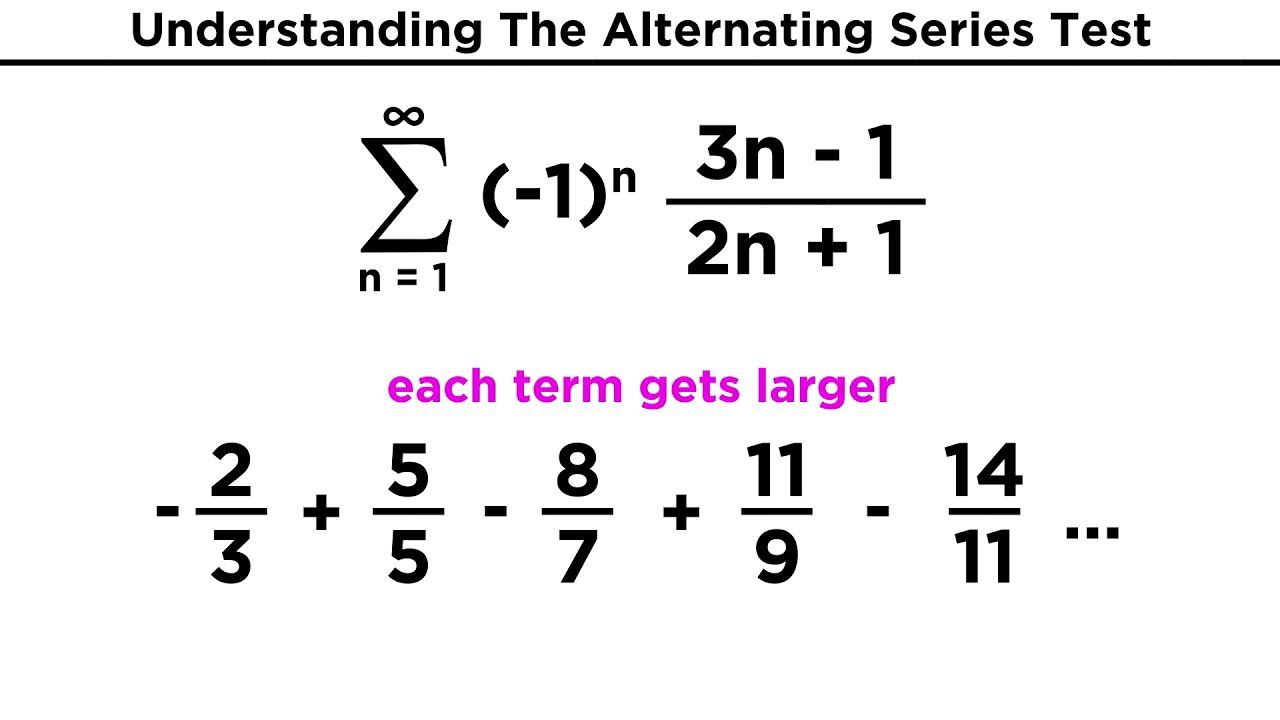
- 🔄 Alternating series, which have terms that alternate between positive and negative, are highlighted as a special case that can be easily analyzed with the alternating series test.
- 📉 The alternating series test states that if a sequence of positive terms \( a_n \) is decreasing and the limit of \( a_n \) as \( n \) approaches infinity is 0, then the alternating series converges; otherwise, it diverges.
- 📈 The script provides examples of series to demonstrate the application of the alternating series test, showing how quickly one can determine convergence without complex calculations.
- 📝 The importance of the nth term test is discussed, contrasting it with the alternating series test, where the latter is more applicable to alternating series.
- 🚫 A warning is given about the dangers of rearranging terms in conditionally convergent series, as it can lead to different sums, unlike absolutely convergent series which can be rearranged without changing the sum.
- 🔢 The script discusses the implications of the convergence tests on the series \( \sum_{n=1}^{\infty} \frac{(-1)^{n+1}}{n^p} \), showing that absolute convergence occurs for \( p > 1 \) and conditional convergence for \( p \leq 1 \).
- 📚 The concept of absolute convergence is further explained with an example involving a series with \( \frac{1}{n^2} \) terms, which converges absolutely.
- 🔑 The script emphasizes the robustness of absolutely convergent series, which allows for the rearrangement of terms without affecting the sum, a property crucial for applications in Taylor series.
- 💡 The lecture concludes with a teaser for the next lessons, hinting at the importance of understanding absolute and conditional convergence in scientific applications.
Q & A
What is the main focus of Lecture 53 by Professor Greist?
-The main focus of Lecture 53 is on absolute and conditional convergence in the context of infinite series, particularly those with both positive and negative terms.
Why are some tests for determining convergence or divergence not always obvious to apply?
-Some tests may not be obvious to apply because they may not work well in all cases, and the complexity of the series in question can make it difficult to determine the best approach to take.
What is an alternating series?
-An alternating series is a series where the terms alternate between positive and negative. It is often written in the form \( \sum_{n=1}^{\infty} (-1)^n a_n \) where \( a_n \) terms are positive.
What is the Alternating Series Test and what does it require for a series to converge?
-The Alternating Series Test states that if a sequence \( a_n \) is decreasing and positive, then the alternating series \( \sum_{n=1}^{\infty} (-1)^n a_n \) converges if and only if the limit of \( a_n \) as \( n \) approaches infinity is 0; it diverges if this limit is nonzero.
How does the convergence of an alternating series relate to the sequence of partial sums?
-The convergence of an alternating series is related to the sequence of partial sums in that if the sequence \( a_n \) is decreasing, the jumps in the partial sums get smaller, and if the limit of \( a_n \) is 0, the series converges; otherwise, if the limit is nonzero, the series diverges.
What is the difference between absolute convergence and conditional convergence?
-A series is absolutely convergent if both the sum of the terms and the sum of the absolute values of the terms converge. It is conditionally convergent if the sum of the terms converges, but the sum of the absolute values diverges.
Why is it important to distinguish between absolute and conditional convergence?
-The distinction is important because absolutely convergent series are more robust and allow for rearrangement of terms without changing the sum, whereas conditionally convergent series can be sensitive to rearrangement and may not behave as predictably.
What is an example of a series that is conditionally convergent?
-An example of a conditionally convergent series is the alternating harmonic series, \( \sum_{n=1}^{\infty} (-1)^{n+1} \frac{1}{n} \), which converges by the Alternating Series Test but the sum of the absolute values of its terms, the harmonic series, diverges.
What does it mean for a series to be divergent?
-A series is divergent if it does not converge at all, meaning that the sequence of partial sums does not approach a finite limit.
How can the properties of absolute and conditional convergence affect the application of series in the sciences?
-The properties of convergence can affect the reliability and robustness of mathematical models in the sciences. Absolute convergence ensures that a series can be manipulated more freely, while conditional convergence requires more caution due to its sensitivity to term rearrangement.
What is the significance of the series \( \sum_{n=1}^{\infty} (-1)^n \frac{\pi^n (\log n)^2 + 1}{n!} \cosh^{5/3}(n) \) in the lecture?
-This series is used as an example to illustrate the application of the Alternating Series Test. It converges because the factorial term dominates and the limit of the \( a_n \) terms is 0.
Outlines
📚 Introduction to Absolute and Conditional Convergence
Professor Greist begins lecture 53 by introducing the concepts of absolute and conditional convergence in the context of infinite series. He explains that the convergence of a series can be more complex than initially thought, especially when dealing with series that contain both positive and negative terms. The professor emphasizes the importance of the alternating series test, which simplifies the analysis of such series. He illustrates this with three series, two of which converge and one that diverges, highlighting the ease of applying the test due to the presence of the term (-1)^N. The lecture also introduces the form of alternating series and the conditions under which they converge or diverge.
🔍 Deep Dive into Alternating Series and Convergence Tests
In this section, Professor Greist delves deeper into the characteristics of alternating series, explaining that they are relatively straightforward to analyze. He demonstrates how the presence of the term (-1)^N in a series can render many traditional convergence tests ineffective, but the alternating series test remains applicable. The professor then explains the concept of absolute convergence, where both the series and the series of absolute values converge, and conditional convergence, where the series converges but the series of absolute values diverges. He uses examples to illustrate these concepts, including the alternating harmonic series and an alternating p-series, to show how the value of p affects the nature of convergence.
🚧 The Implications of Conditional Convergence and Series Rearrangement
The final paragraph of the script addresses the implications of conditional convergence and the potential dangers associated with it. Professor Greist warns that conditionally convergent series can be sensitive to the order of terms, which can lead to different sums if the series is rearranged. In contrast, absolutely convergent series are robust and can be rearranged without affecting the sum. The professor revisits the series for the natural logarithm function and demonstrates how conditional convergence can lead to seemingly paradoxical results. He emphasizes the importance of being cautious with conditionally convergent series and trusting in the stability of absolutely convergent series, especially in scientific applications.
Mindmap
Keywords
💡Convergence
💡Absolute Convergence
💡Conditional Convergence
💡Alternating Series
💡Alternating Series Test
💡Divergent Series
💡Series
💡Factorial
💡Logarithm
💡Hyperbolic Cosine
💡Rearrangements
Highlights
The lecture introduces the concepts of absolute and conditional convergence in the context of infinite series.
It explains that series with both positive and negative terms require a refined notion of convergence.
The importance of the alternating series test is emphasized, which is applicable to series with terms that alternate in sign.
The test is simple to apply but requires a decreasing sequence of positive terms.
An explanation of why the alternating series test is particularly useful due to the prevalence of alternating series.
The convergence of an alternating series is illustrated by examining the sequence of partial sums.
An example of applying the alternating series test to a series involving factorial and hyperbolic cosine terms.
Another example demonstrates the convergence of a series with terms involving logarithms to various powers.
A third example involving cube roots and higher powers is used to show divergence despite the series being alternating.
Introduction of the definitions of absolutely convergent and conditionally convergent series.
A series with only positive terms cannot be conditionally convergent, highlighting the distinction between types of convergence.
The concept that there are exactly three possibilities for a series: divergence, conditional convergence, and absolute convergence.
An example of an absolutely convergent series involving a p-series with a specific power.
The alternating harmonic series is presented as a classic example of a conditionally convergent series.
The implications of conditional convergence are discussed, warning of the potential for rearrangement to change the sum.
Absolute convergence is contrasted with conditional convergence, noting the robustness of absolutely convergent series.
The potential for rearranging terms in a conditionally convergent series to sum to any number is highlighted as a significant risk.
The importance of absolute convergence in practical applications, especially in the context of Taylor series, is emphasized.
The lecture concludes with a teaser for the next lessons, which will explore the significance of convergence types in scientific applications.
Transcripts
Browse More Related Video

Absolute and Conditional Convergence

Unit VII: Lec 3 | MIT Calculus Revisited: Single Variable Calculus

AP Calculus BC Lesson 10.9

Calculus 2 Lecture 9.6: Absolute Convergence, Ratio Test and Root Test For Series
Alternating Series, Types of Convergence, and the Ratio Test

Conditional and Absolute Convergence for Infinite Series
5.0 / 5 (0 votes)
Thanks for rating: