Calculus Chapter 2 Lecture 13 BONUS
TLDRIn this calculus lecture, Professor Greist delves into the concept of higher derivatives and their connection to Taylor series, illustrating how infinitesimal information at a specific input can predict a function's behavior at distant points. He introduces the language of operators, defining 'D' as the derivative operator and 'E' as a shift operator, and explores their algebraic properties. The lecture culminates in a profound insight: the shift operator is the exponential of the differentiation operator, and differentiation can be seen as the natural logarithm of the shift. This revelation adds another layer to the understanding of exponentiation and differentiation, emphasizing their intertwined roles in analyzing functions.
Takeaways
- 📚 The lecture introduces the concept of higher derivatives in the context of Taylor series, emphasizing how knowing derivatives at a particular input can help approximate the function's value at other points.
- 🔍 Professor Greist uses the language of operators to explain derivatives, defining the derivative operator as 'D', which takes a function and returns its derivative.
- 🎲 The script explains that operators can be raised to powers, such as 'D squared' for the second derivative and 'D cubed' for the third derivative, with 'D to the zero' being the identity operator 'I'.
- 🔢 The identity operator 'I' is likened to the number 1 in multiplication, as it leaves the function unchanged when applied.
- 🚀 The script introduces another operator 'e', which corresponds to a shift of the function, moving the graph of the function to the left by one unit when evaluated at 'x'.
- 🔄 The concept of non-integer powers of the shift operator 'e' is discussed, allowing for fractional shifts in the function's evaluation.
- 🔄 The inverse of the shift operator 'e' is also discussed, allowing for a right shift of the function, which is the inverse operation of the left shift.
- 🔗 The script connects the shift operator 'e' with the differentiation operator 'D', showing that the shift is equivalent to the exponential of the differentiation operator multiplied by the shift amount 'H'.
- 📈 The Taylor expansion is rewritten using the operator language, demonstrating the relationship between the shift operator and the derivatives of the function.
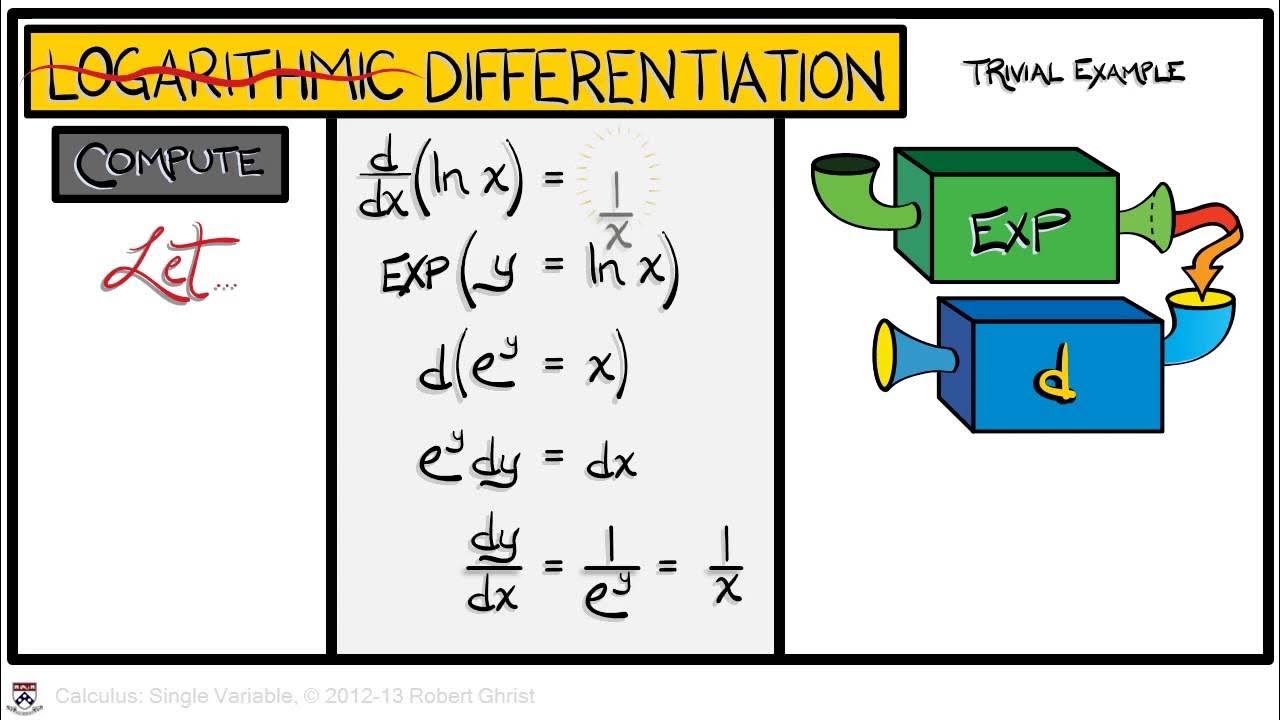
- 🤔 The lecture concludes by reflecting on the deep connection between the shift operator and the differentiation operator, suggesting that the differentiation operator can be seen as the natural logarithm of the shift operator.
- 🌐 The script invites students to consider the broader implications of these mathematical concepts, hinting at the interconnectedness of different areas of mathematics.
Q & A
What is the ultimate interpretation of higher derivatives according to the lecture?
-The ultimate interpretation of higher derivatives is in terms of Taylor series, which allows for better approximation of the function's value at a particular input when more derivatives are known at that input.
What is an operator in the context of this calculus lecture?
-An operator is a function that takes another function as its input and returns a new function as its output, with the derivative being a key example of such an operator.
What is the operator symbol for the derivative in the lecture?
-The operator symbol for the derivative is a capital 'D', which when applied to a function f, returns the derivative of f with respect to x, denoted as Df/Dx.
How is the nth derivative represented using the operator D?
-The nth derivative is represented as D to the power of n, which means applying the derivative operator successively n times.
What is the identity operator in the context of operators, and what is its symbol?
-The identity operator is an operator that returns the input function unchanged. It is denoted by the symbol 'I' and is analogous to the number 1 in multiplication.
What is the effect of the operator 'e' on a function f?
-The operator 'e' corresponds to a shift of the function f, so that when evaluated at x, it gives the value of f at x plus 1, effectively shifting the graph of f to the left by one unit.
How does the operator 'e' relate to non-integer powers?
-Non-integer powers of 'e', such as 'e' to the power of h, allow for a fractional shift of the function, evaluating f at x plus h instead of at x.
What is the inverse of the shift operator 'e'?
-The inverse of the shift operator 'e' is 'e' to the power of negative one, which performs a right shift, evaluating the function at x minus one instead of x plus one.
How is the shift operator 'e' related to the differentiation operator 'D'?
-The shift operator 'e' to the power of h is equivalent to the exponentiation of h times the differentiation operator 'D', which can be thought of as the exponential of the differentiation operator.
What does the script suggest about the relationship between the differentiation operator 'D' and the shift operator 'e'?
-The script suggests that the differentiation operator 'D' can be considered as the natural logarithm of the shift operator 'e', indicating a deep connection between shifting and differentiating.
What is the significance of the observation that the shift is the exponential of the differentiation operator?
-This observation is significant as it provides a deep insight into the algebraic relationship between shifting a function and differentiating it, showing that the shift operator is essentially the exponential of the differentiation operator.
Outlines
📚 Introduction to Higher Derivatives and Operators
Professor Greist begins Lecture 13 by delving into the concept of higher derivatives and their interpretation through Taylor series. He explains how knowing the derivatives at a specific input can help approximate the function's value at a different point. The professor introduces the concept of operators, which take a function as input and return another function as output. The derivative operator 'D' is highlighted, alongside the identity operator 'I', which acts like the number 1 in multiplication. The lecture also touches on other operators, such as 'e' for shifting functions, and explores the algebraic properties of these operators.
🔍 Deep Dive into Shift Operators and Exponential Representation
Continuing from the introduction, Professor Greist explores the concept of shift operators in more detail. He discusses how the shift operator 'e' to the power of 'H' can be evaluated at a point 'X' by considering a Taylor expansion. The professor then rewrites this in terms of operators, revealing a connection between the shift operator and the exponential function of the differentiation operator 'D'. This leads to the profound insight that the shift operator is essentially the exponential of the differentiation operator, and vice versa, the differentiation operator can be seen as the natural logarithm of the shift operator. The lecture concludes by emphasizing the ongoing exploration of the meaning behind exponentiation and differentiation, and how they provide deep insights into the nature of functions.
Mindmap
Keywords
💡Calculus
💡Taylor Series
💡Derivatives
💡Operators
💡Differentiation Operator (D)
💡Identity Operator
💡Shift Operator (e)
💡Exponential Function
💡Algebraic Manipulation
💡Natural Logarithm
Highlights
Introduction to the concept of higher derivatives in the context of Taylor series, emphasizing the importance of knowing derivatives at a particular input for function approximation.
Discussion on the algebraic properties of operators in calculus, specifically the derivative operator represented by capital D.
Explanation of how the derivative operator D can be raised to powers to represent higher derivatives, such as D squared for the second derivative.
Introduction of the identity operator, denoted by I, which represents the operation of doing nothing to a function, analogous to the number 1 in multiplication.
Introduction of the shift operator, denoted by capital E, which shifts the graph of a function to the left by one unit.
Description of the effect of applying the shift operator E multiple times, such as E squared for a double shift to the left.
Introduction of non-integer powers of the shift operator, such as E to the H, allowing for fractional shifts in the function's graph.
Explanation of the inverse shift operator, E to the negative one, which represents a right shift of the function's graph.
Discussion on the algebraic manipulation of operators, including the combination of the shift operator E and the derivative operator D.
Deep observation that the shift operator E to the H is equivalent to the exponentiation of H times the differentiation operator D.
Insight that the differentiation operator D can be considered as the natural logarithm of the shift operator E.
Connection between the shift operator and the exponential function, showing that the shift is the exponential of the differentiation operator.
Discussion on the surprising relationship between the shift and differentiation operators, and its implications in understanding the function's behavior.
Reiteration of the fundamental question of what e to the x means, and its ongoing exploration throughout the course.
Reflection on the layers of understanding in exponentiation and differentiation, and how they provide deep insights into a function.
Emphasis on the importance of knowing higher derivatives at a particular input for predicting the function's behavior far away from that input.
Final thoughts on the profound implications of the relationship between operators in calculus and their applications in mathematics.
Transcripts
5.0 / 5 (0 votes)
Thanks for rating: