Physics 8 Work, Energy, and Power (7 of 37) Inclined Plane (Friction)

Michel van Biezen
10 Sept 201308:32
EducationalLearning
32 Likes 10 Comments

TLDRThis lecture explores the relationship between work, kinetic, and potential energy, focusing on an inclined plane scenario with friction. The work done on an object is divided into potential energy, kinetic energy, and energy lost to friction. The potential energy is calculated using the formula MGH, and the friction force is determined by the normal force and the coefficient of friction. The kinetic energy is then deduced from the remaining work, leading to the final velocity of the object, illustrating the balance of energy in a physical system.

Takeaways
  • πŸ“š The lecture discusses the comparison of work, kinetic energy, and potential energy in the context of an inclined plane.
  • πŸ”„ Work done on a system is converted into potential energy, kinetic energy, and some is lost due to friction.
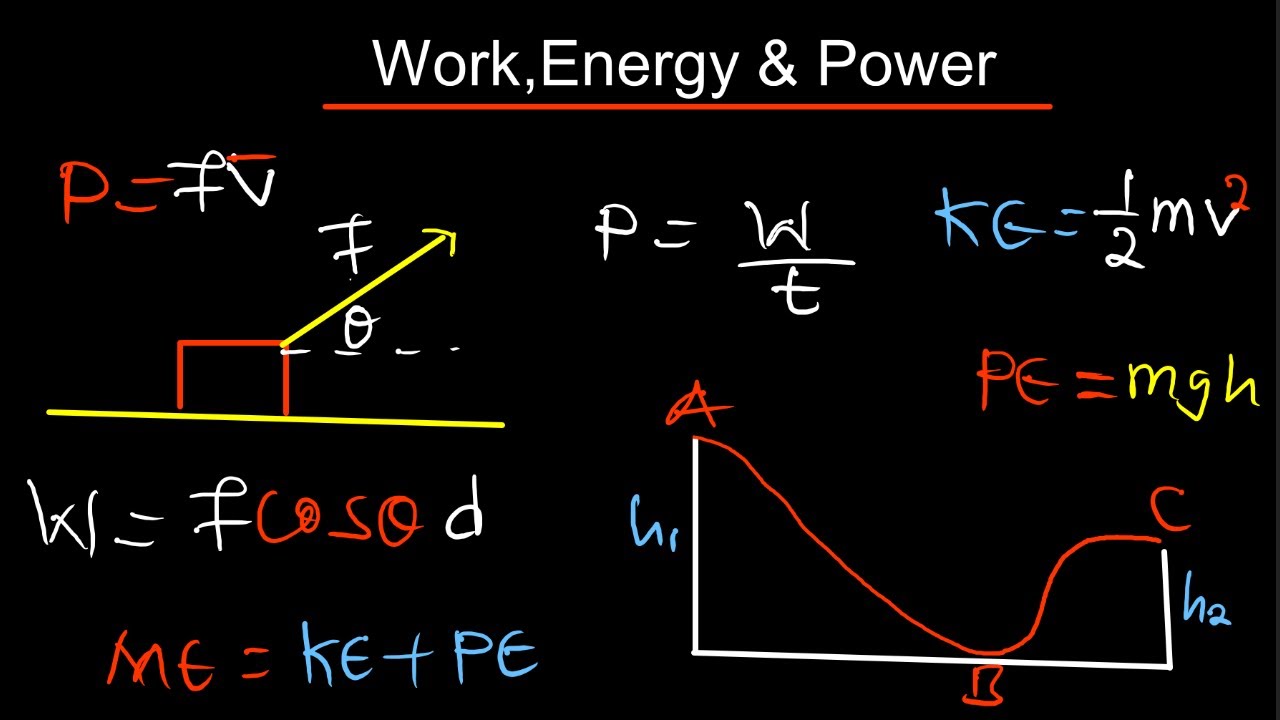
  • πŸ”’ Basic definition of work is Force Times Distance, which is applied here with a displacement of 20 meters.
  • πŸ“ The work performed is calculated as 2000 joules using a force of 100 Newtons.
  • πŸ“ˆ Potential energy gained is calculated using the formula MGH, resulting in 490 joules for a height gain of 10 meters.
  • πŸ“‰ Energy lost due to friction is determined by calculating the friction force and its work against the displacement.
  • 🧲 Friction force is calculated as the normal force (mg cosine theta) times the coefficient of friction (mu).
  • πŸ“Œ The normal force is equal to the weight of the object (mg) times the cosine of the angle (theta).
  • πŸš€ The kinetic energy of the object is calculated by subtracting the potential energy and energy lost to friction from the total work done.
  • 🏁 The final velocity of the object is determined using the kinetic energy formula, resulting in a velocity of approximately 23.15 meters per second.
Q & A

  • What is the main topic of the lecture?

    -The main topic of the lecture is comparing work, kinetic energy, and potential energy in the context of an inclined plane with friction.

  • What is the relationship between work done, potential energy, kinetic energy, and energy lost due to friction?

    -The work done on the system is equal to the sum of the potential energy, kinetic energy, and the energy lost due to overcoming friction.

  • How is work defined in the context of the lecture?

    -Work is defined as the force applied to an object times the distance the object is moved in the direction of the force, which is given by the equation Work = Force Γ— Displacement.

  • What is the displacement of the object in the example given?

    -The displacement of the object in the example is 20 meters along the inclined plane.

  • How is the height gained by the object calculated?

    -The height gained by the object is calculated by multiplying the displacement (20 meters) by the sine of the angle of inclination (30 degrees), which is 10 meters.

  • What is the formula for calculating potential energy gained by the object?

    -The potential energy gained by the object is calculated using the formula Potential Energy = Mass Γ— Gravity Γ— Height (PE = mgh).

  • What is the total potential energy gained by the object in the example?

    -The total potential energy gained by the object is 490 joules, calculated from the mass of 5 kg, gravity of 9.8 m/sΒ², and height of 10 meters.

  • How is the friction force determined in this scenario?

    -The friction force is determined by multiplying the normal force (which is mg cos ΞΈ) by the coefficient of friction (ΞΌ), where m is mass, g is gravity, and ΞΈ is the angle of inclination.

  • What is the work done by overcoming friction and how is it calculated?

    -The work done by overcoming friction is calculated by multiplying the friction force by the displacement and considering the direction (180 degrees apart), which results in a negative value indicating energy loss.

  • How much energy is lost due to friction in the example?

    -In the example, 170 joules of energy is lost due to overcoming friction.

  • How can the final velocity of the object be determined if the kinetic energy is known?

    -The final velocity of the object can be determined using the kinetic energy formula, where Kinetic Energy = 1/2 mvΒ². By rearranging the formula to solve for v (velocity), we get v = √(2 Γ— Kinetic Energy / Mass).

  • What is the final velocity of the object after moving 20 meters?

    -The final velocity of the object after moving 20 meters is approximately 23.15 meters per second.

Outlines
00:00
πŸ”§ Work, Kinetic, and Potential Energy with Friction

This paragraph introduces a lecture on the comparison of work, kinetic, and potential energy, specifically in the context of an inclined plane with friction. The work done on an object is divided into potential energy, kinetic energy, and energy lost to friction. The basic definition of work as force times distance is discussed, with a given example of moving an object 20 meters with a force of 100 Newtons, resulting in 2,000 joules of work. The height gained by the object and the potential energy calculation are also covered, using the mass, gravitational acceleration, and height to find 490 joules of potential energy. The friction force is calculated based on the normal force and the coefficient of friction, resulting in 8.5 Newtons of friction force, which leads to 170 joules of energy lost to overcome friction.

05:01
πŸ“‰ Calculating Kinetic Energy and Velocity with Friction

In this paragraph, the focus is on calculating the work done against friction and its implications on the energy balance. The work done by friction is determined by the friction force times the displacement, with the negative sign indicating energy loss, amounting to 170 joules. The remaining work is then allocated to potential and kinetic energy. The calculation of kinetic energy is detailed, showing that after accounting for potential energy and energy lost to friction, 660 joules are left for kinetic energy. The final velocity of the object is then calculated using the kinetic energy and mass, resulting in a velocity of 23 meters per second. The paragraph concludes by emphasizing the effects of work on an object, leading to increases in potential and kinetic energy, and the inevitable energy loss due to friction.

Mindmap
Keywords
πŸ’‘Work
Work in physics is defined as the product of force and the displacement in the direction of the force. In the video, work is calculated as 2,000 joules, which is the force of 100 Newtons multiplied by the displacement of 20 meters. It is a central concept as it explains the energy transfer that occurs when an object is moved against an inclined plane.
πŸ’‘Kinetic Energy
Kinetic energy is the energy an object possesses due to its motion, calculated as one-half the mass times the velocity squared. In the script, it is part of the energy partitioned from the work done on the object, and the final velocity of the block is calculated based on the kinetic energy, illustrating the concept of energy conservation.
πŸ’‘Potential Energy
Potential energy is the stored energy of an object due to its position in a force field, such as gravity. The script explains that 490 joules of the work done is converted into potential energy as the object gains height, demonstrating the conversion between different forms of energy.
πŸ’‘Inclined Plane
An inclined plane is a simple machine that allows for the raising of an object with less force than lifting it vertically. The video uses an inclined plane to discuss the distribution of work done into kinetic, potential, and lost energy due to friction.
πŸ’‘Friction
Friction is the force that resists the relative motion of solid surfaces, the sliding of objects, or the tendency of such motion. The script mentions a coefficient of friction and calculates the work done to overcome it, showing how friction can dissipate energy in a system.
πŸ’‘Coefficient of Friction
The coefficient of friction is a dimensionless scalar value that represents the ratio of the force of friction between two bodies to the force pressing them together. In the video, it is used to determine the frictional force acting on the block and the energy lost due to friction.
πŸ’‘Normal Force
The normal force is the perpendicular support force exerted by a surface that supports the weight of an object resting on it. The script calculates this force as part of the process to find the frictional force acting on the block.
πŸ’‘Displacement
Displacement is the change in position of an object. In the context of the video, the displacement is 20 meters along the inclined plane, and it is used to calculate the work done on the object.
πŸ’‘Force
Force is any interaction that, when unopposed, will change the motion of an object. The script describes a force of 100 Newtons applied to move the object along the inclined plane, which is key to the calculation of work done.
πŸ’‘Energy Conservation
Energy conservation is the principle that the total energy of an isolated system remains constant over time. The video script uses this principle to explain how the work done on the object is distributed into potential energy, kinetic energy, and energy lost to friction.
πŸ’‘Velocity
Velocity is the speed of an object in a particular direction. The script concludes with the calculation of the block's final velocity, which is derived from the kinetic energy, illustrating the direct relationship between kinetic energy and velocity.
Highlights

Introduction to comparing work, kinetic, and potential energy with an inclined plane example.

Incorporation of friction into the inclined plane scenario, affecting energy conversion.

Work done on a system is equal to the sum of potential energy, kinetic energy, and energy lost to friction.

Basic definition of work as force times distance, with displacement of 20 meters.

Calculation of work done using 100 Newtons of force over 20 meters, resulting in 2000 joules.

Determination of height gained by the object using the sine of the angle of inclination.

Calculation of potential energy gained as 490 joules using mass, gravity, and height.

Explanation of how to find the friction force using the normal force and coefficient of friction.

Determination of the friction force magnitude as 8.5 Newtons.

Calculation of work done against friction as 170 joules lost.

Equation balancing work done with potential energy, kinetic energy, and energy lost.

Calculation of kinetic energy as 1340 joules after accounting for potential energy and energy lost.

Determination of the object's final velocity using kinetic energy and mass.

Final velocity calculation resulting in 23.15 meters per second.

Illustration of how work done results in increased potential and kinetic energy, with some energy lost to friction.

Transcripts

Browse More Related Video

Work Energy and Power Full Topic

Energy Systems Clarified

What is Energy & Work in Chemistry & Physics? - [1-1-6]

Work Energy Principle

What is Kinetic Energy & Work-Energy Theorem in Physics? - [1-8]

2015 AP Physics 1 free response 3c

Rate This

5.0 / 5 (0 votes)

Thanks for rating:

Related Tags
Work DoneKinetic EnergyPotential EnergyInclined PlaneFriction ForceEnergy LossPhysics LectureEnergy ConversionForce CalculationDisplacementVelocity