Work Energy and Power Full Topic
TLDRThis educational video delves into the principles of work, energy, and power. It explains work as the product of force and displacement, highlighting the importance of direction and perpendicularity. The script further explores kinetic and potential energy, illustrating how they convert and relate to work done. It also covers power as the rate of work done and touches on energy conservation. Practical examples, such as calculating work done by friction and potential energy of a textbook, are provided to solidify understanding.
Takeaways
- 🔧 Work is defined as the product of force and displacement in the direction of the force, measured in joules (J).
- 📏 When force and displacement are in the same direction, work done is positive; in opposite directions, work is negative; perpendicular to each other results in zero work.
- 🔄 The net work done is the sum of work done by all forces, considering their direction relative to displacement.
- ⚙️ Power is the rate at which work is done, measured in watts (W), and can also be expressed in horsepower (hp).
- ⏱️ The formula for power includes both work done over time and force times velocity, with variations for forces applied at an angle.
- 🚜 In scenarios involving friction, the work done by friction is considered negative and is crucial in calculating net work and energy changes.
- ⚡️ Kinetic energy is the energy of motion, given by the formula \( \frac{1}{2}mv^2 \), where 'm' is mass and 'v' is velocity.
- ⏫ Gravitational potential energy is the energy an object possesses due to its position in a gravitational field, calculated as \( mgh \).
- 🔄 The work-energy theorem states that the net work done on an object is equal to the change in its kinetic energy.
- 🛠️ The concept of conservation of energy implies that energy can be transformed but not created or destroyed, which is essential in analyzing energy transformations in various systems.
- 📉 Non-conservation of energy occurs in scenarios where external forces, like friction, cause a loss in mechanical energy, transforming it into other forms like heat.
Q & A
What is the definition of work in physics?
-Work in physics is defined as the product of force and displacement in the direction of the force. It occurs when a force causes a displacement of an object.
How is work calculated when force and displacement are in the same direction?
-When force and displacement are in the same direction, work is calculated as the product of the force and the displacement (Work = Force × Displacement).
What is the unit of work in the International System of Units (SI)?
-The unit of work in the SI system is the joule (J), which is equivalent to one newton-meter (N·m).
What happens to the work done if the force and displacement are in opposite directions?
-If the force and displacement are in opposite directions, the work done is negative because it represents a force opposing the motion.
Why is the work done zero when force and displacement are perpendicular?
-The work done is zero when force and displacement are perpendicular because the component of the force in the direction of displacement is zero, resulting in no work being done on the object.
Can you provide an example of calculating work done against friction?
-Sure. If an object is moving due to an applied force of 100 N over a distance of 0.5 m, and there is a frictional force of 20 N opposing the motion, the work done by the applied force is positive (50 J), while the work done by the friction force is negative (-10 J), resulting in a net work of 40 J.
What is the formula for calculating work done when force is applied at an angle?
-The formula for calculating work done when force is applied at an angle (θ) is Work = F × D × cos(θ), where F is the magnitude of the force, D is the displacement, and θ is the angle between the force and displacement vectors.
How is power defined in physics and what are its units?
-Power in physics is defined as the rate at which work is done, or the amount of work done per unit time. Its SI unit is the watt (W), which is equivalent to one joule per second (J/s).
What is the relationship between watts and horsepower?
-One horsepower is equivalent to 746 watts. This relationship is used to convert between the two units of power.
Can you explain the concept of kinetic energy and its formula?
-Kinetic energy is the energy possessed by an object due to its motion. The formula for kinetic energy is KE = 0.5 × m × v², where m is the mass of the object and v is its velocity.
What is potential energy and how is it related to work?
-Potential energy is the energy an object possesses due to its position in a force field, typically related to gravity. It is related to work as the work done against gravity to raise an object results in an increase in its gravitational potential energy, calculated as PE = m × g × h, where m is mass, g is the acceleration due to gravity, and h is the height.
How can you determine the initial speed of a car involved in an accident using the work-energy theorem?
-Using the work-energy theorem, which states that the net work done on an object is equal to the change in its kinetic energy, you can calculate the initial speed of a car if you know the length of the skid mark, the coefficient of kinetic friction, and the mass of the car. The work done by friction (negative work) is equal to the change in kinetic energy from the initial to the final state (when the car comes to a stop).
Outlines
🔨 Understanding Work in Physics
The first paragraph introduces the concept of work in physics, defining it as the product of force and displacement in the same direction. It uses an example of applying a force of 100 Newtons to move an object 3 meters, resulting in 300 Joules of work. The paragraph clarifies that work is positive when force and displacement are in the same direction, negative when opposite, and zero when perpendicular. It also explains the unit of work, the Joule, and how it's derived from Newton meters.
🔧 Work Done by Applied Force and Friction
This section discusses how to calculate the work done by an applied force and friction. It explains that work is positive when the force and displacement are in the same direction and negative when they are opposite. The paragraph provides a scenario where an object moves with friction, calculating the work done by both the applied force and the friction force, and then determining the net work by adding these values.
📐 Calculating Work with Applied Force at an Angle
The third paragraph explores the concept of work when a force is applied at an angle (Theta) to the direction of displacement. It explains the use of trigonometric functions to find the component of the force that is in line with the displacement (Fx), using the sine and cosine ratios. The general formula for work in such cases is presented as F cos Theta times the displacement (D).
🏗️ Practical Examples of Work Calculations
This paragraph applies the concept of work to practical scenarios, such as calculating the work done in lifting a cement block and pulling a load on ice. It demonstrates how to use the formulas for work when forces are applied horizontally or at an angle, and how to account for friction when calculating net work.
🚜 Work and Friction in Real-World Scenarios
The fifth paragraph extends the discussion to more complex real-world examples, including the calculation of work done by an Eskimo pulling a load on ice with a horizontal force and then with a force applied at a 30-degree angle. It also addresses how to find the work done against friction and the net work in such situations.
🔄 Transitioning to Power and Energy Concepts
Moving beyond work, this paragraph introduces power, defined as the rate at which work is done. It explains the relationship between work, power, and time, and how power can be calculated using different formulas depending on the given variables. The paragraph also touches on the units of power, including watts and horsepower.
⚡️ Power Calculations in Various Situations
The sixth paragraph provides examples of calculating power in different situations, such as an electric motor pulling a truck and a motor pulling a cable up a slope. It demonstrates the use of power formulas with force, velocity, and work to find the power supplied in various scenarios.
🚀 Exploring Kinetic and Potential Energy
This paragraph delves into the concepts of kinetic and potential energy. It explains that kinetic energy is the energy of motion, calculated as half the product of mass and the square of velocity. The paragraph also introduces the idea of potential energy as the energy an object possesses due to its position.
⏳ The Relationship Between Work, Kinetic Energy, and Potential Energy
The eighth paragraph discusses the relationship between work, kinetic energy, and potential energy. It explains how work done on an object can be equated to changes in kinetic energy and how potential energy can be related to work done against gravity. The concept of elastic potential energy is also briefly introduced.
🌐 Gravitational Potential Energy and Energy Conservation
The ninth paragraph focuses on gravitational potential energy, explaining how it is calculated and how it can be transformed into other forms of energy. It also touches on the concept of energy conservation, stating that energy cannot be created or destroyed, only transformed.
🛠️ Work-Energy Theorem and Non-Conservation of Energy
The final paragraph introduces the work-energy theorem, which states that the net work done on an object is equal to the change in its kinetic energy. It also discusses situations where energy is not conserved, such as when non-conservative forces like friction are at play.
📘 Applying the Work-Energy Theorem to Real-World Problems
This paragraph applies the work-energy theorem to solve real-world problems, such as estimating the initial speed of a car from a skid mark and calculating the speed at the foot of a slope considering friction. It also addresses the concept of non-conservation of energy in the context of these problems.
Mindmap
Keywords
💡Work
💡Energy
💡Power
💡Kinetic Energy
💡Potential Energy
💡Force
💡Displacement
💡Friction
💡Conservation of Energy
💡Work-Energy Theorem
Highlights
Work is defined as the product of force and displacement in the same direction.
Work is measured in Joules, symbolized by the capital letter W, where one Newton meter equals one Joule.
When force and displacement are in opposite directions, the work done is negative.
Work done is zero when force and displacement are perpendicular to each other.
The formula for work done when force is applied at an angle is F cos Theta times displacement.
Power is defined as the rate at which work is done, measured in Watts.
The relationship between Watts and horsepower is that 1 horsepower equals 746 Watts.
Kinetic energy is the energy of an object due to its motion, given by the formula 0.5 * m * v^2.
Gravitational potential energy is the energy an object possesses due to its position, calculated as m * g * h.
Elastic potential energy is stored in a spring and is given by the formula 0.5 * k * x^2.
The work-energy theorem states that the work done by all forces on a body is equal to the change in its kinetic energy.
The conservation of energy principle states that energy cannot be created or destroyed, only transformed from one form to another.
An example of energy conversion is a child sliding down a frictionless slope, converting potential energy into kinetic energy.
In the case of non-conservation of energy, energy can be lost, such as through friction in a car accident.
The work done by friction is equal to the change in kinetic energy when an object comes to a stop.
The initial speed of a car can be estimated using the work done by friction and the length of the skid mark.
The coefficient of kinetic friction affects the speed at which a car comes to a stop on a slope.
Transcripts
Browse More Related Video

AP Physics C: Work, Energy, and Power Review (Mechanics)
AP Physics 1 review of Energy and Work | Physics | Khan Academy

A Level Physics Revision: All of Work, Energy and Power (in 18 minutes)
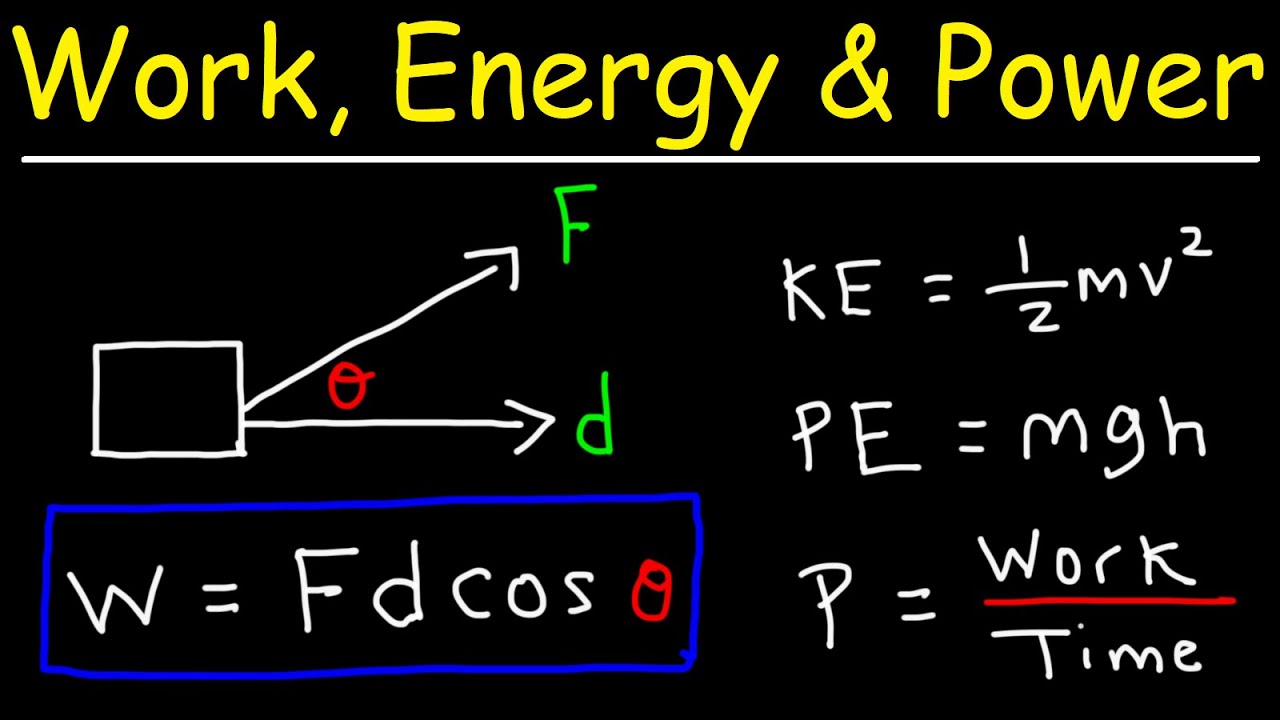
Work, Energy, and Power - Basic Introduction

Work and Energy
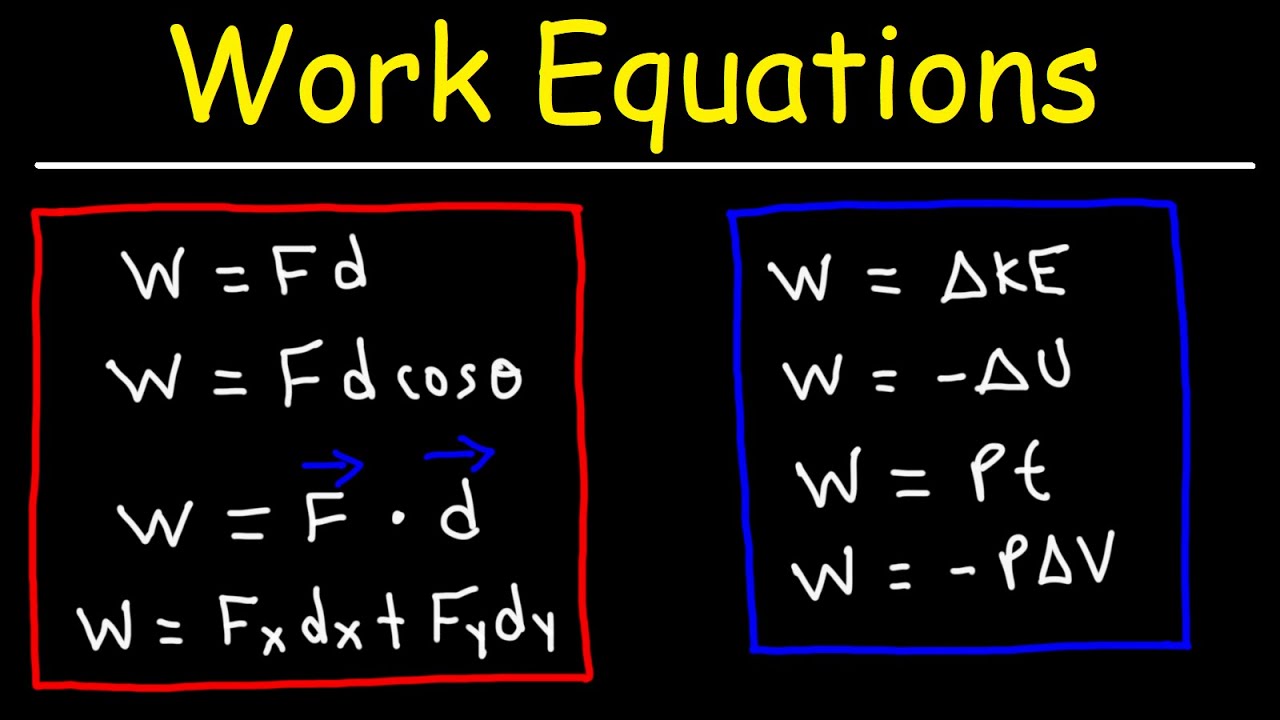
Work, Energy, & Power - Formulas and Equations - College Physics
5.0 / 5 (0 votes)
Thanks for rating: