Introduction to Elastic Potential Energy with Examples

Flipping Physics
3 Nov 201607:17
EducationalLearning
32 Likes 10 Comments

TLDRIn this educational video, the concept of elastic potential energy is explored, symbolized as PE with a subscript 'e' or U with a subscript 'e'. The video explains that elastic potential energy is stored in objects like springs and rubber bands due to temporary deformation and can be converted into kinetic or gravitational potential energy. The formula for calculating this energy is introduced, along with examples of its application in various scenarios. The importance of the spring constant, k, in determining the energy stored is highlighted, and a practical demonstration using a rubber band illustrates the calculation of elastic potential energy, emphasizing its scalar nature.

Takeaways
  • 😀 The symbol for elastic potential energy is often represented as PE with a subscript of e, or sometimes as U with a subscript of e.
  • 🔍 Elastic potential energy is the energy stored in an object due to its temporary deformation, such as in a spring.
  • 🌐 Examples of elastic potential energy include compressed or elongated springs, rubber bands in toys, and rubber balls when they hit the ground.
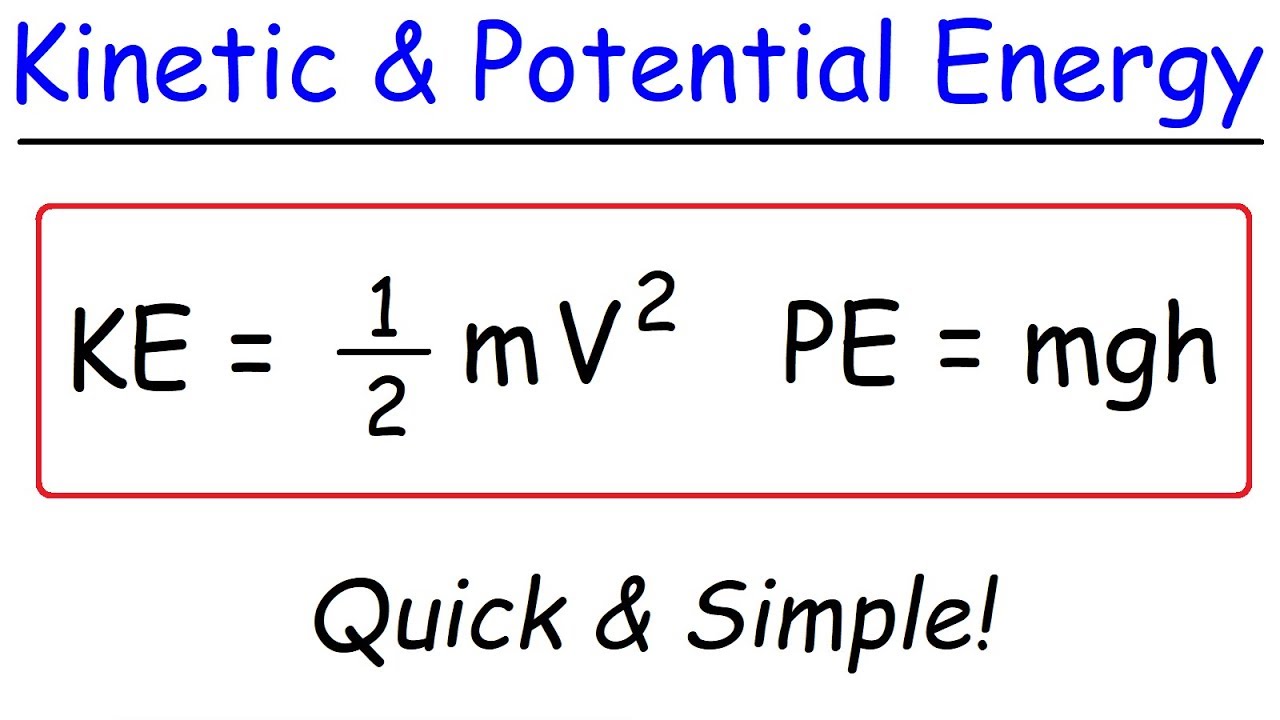
  • 📚 The formula for elastic potential energy is given by \( ext{Elastic Potential Energy} = \frac{1}{2} k x^2 \), where \( k \) is the spring constant and \( x \) is the displacement from the equilibrium position.
  • 🔧 The spring constant \( k \) measures how much a spring resists displacement, typically in Newtons per meter.
  • 🌟 A weak spring has a small spring constant, requiring less force to compress, while a strong spring has a larger spring constant, requiring more force.
  • 🤔 The spring constant of a Slinky is small because it's easy to deform, indicating less force needed for compression.
  • 🏃‍♂️ The spring inside a Pogo stick likely has a large spring constant, as it applies a significant force when used.
  • 📈 The spring constant can be determined by measuring the force exerted by a spring or rubber band at various displacements, as shown in the example with the rubber band.
  • 🚀 Elastic potential energy is a scalar quantity, similar to kinetic energy and gravitational potential energy.
  • 💡 The units for elastic potential energy are Joules, derived from the units of the spring constant (Newtons per meter) and displacement squared (meters squared).
Q & A

  • What is the symbol for elastic potential energy?

    -The symbol for elastic potential energy is PE with a subscript of e, or sometimes it is represented as U with a subscript of e.

  • What is elastic potential energy?

    -Elastic potential energy is the energy stored in an object due to its temporary deformation, such as in a spring when it is compressed or elongated.

  • Can you give an example of an object that stores elastic potential energy besides a spring?

    -Yes, a rubber band in a toy or a rubber ball when it strikes the ground and gets temporarily deformed are examples of objects that store elastic potential energy.

  • What is the equation for elastic potential energy in the context of a spring?

    -The equation for elastic potential energy is PE = (1/2)kx^2, where k is the spring constant and x is the displacement from the equilibrium position.

  • What are the units for the spring constant k?

    -The spring constant k usually has dimensions of Newtons per meter (N/m).

  • How does the spring constant k relate to the force required to compress or expand a spring?

    -The spring constant k is a measure of how much force it takes to compress or expand a spring per meter of displacement.

  • What is Hooke's Law, and how does it relate to the spring constant?

    -Hooke's Law states that the force exerted by a spring is directly proportional to its displacement from the equilibrium position, and the spring constant k is the proportionality constant in this relationship.

  • Can the spring constant be negative?

    -No, the spring constant cannot be negative as it represents a measure of resistance to displacement, which is a physical property that is inherently positive.

  • What is the significance of the displacement x being squared in the equation for elastic potential energy?

    -The displacement x is squared to ensure that the elastic potential energy is always a positive value, regardless of the direction of displacement from the equilibrium position.

  • What is the unit of elastic potential energy?

    -The unit of elastic potential energy is Joules, which is derived from the units of the spring constant (N/m) and displacement (m), resulting in Newton-meters.

  • Why is elastic potential energy considered a scalar?

    -Elastic potential energy is considered a scalar because it only has magnitude and no specific direction, unlike vectors which have both magnitude and direction.

Outlines
00:00
😀 Elastic Potential Energy Basics and Examples

The first paragraph introduces the concept of elastic potential energy, symbolized as PE with a subscript 'e' or U with a subscript 'e'. It is the energy stored in an object due to its temporary deformation, with springs being a common example. The energy can be converted into kinetic and gravitational potential energy. The script also mentions other objects like toys and rubber balls that store elastic potential energy. The formula for calculating elastic potential energy is given as PE = 1/2 k x^2, where 'k' is the spring constant and 'x' is the displacement from the equilibrium position. The spring constant is explained as a measure of a spring's resistance to displacement, with examples provided to illustrate springs with different constants. The paragraph concludes with an example of analyzing the elastic potential energy stored in a rubber band.

05:01
😀 Calculation of Elastic Potential Energy and Units

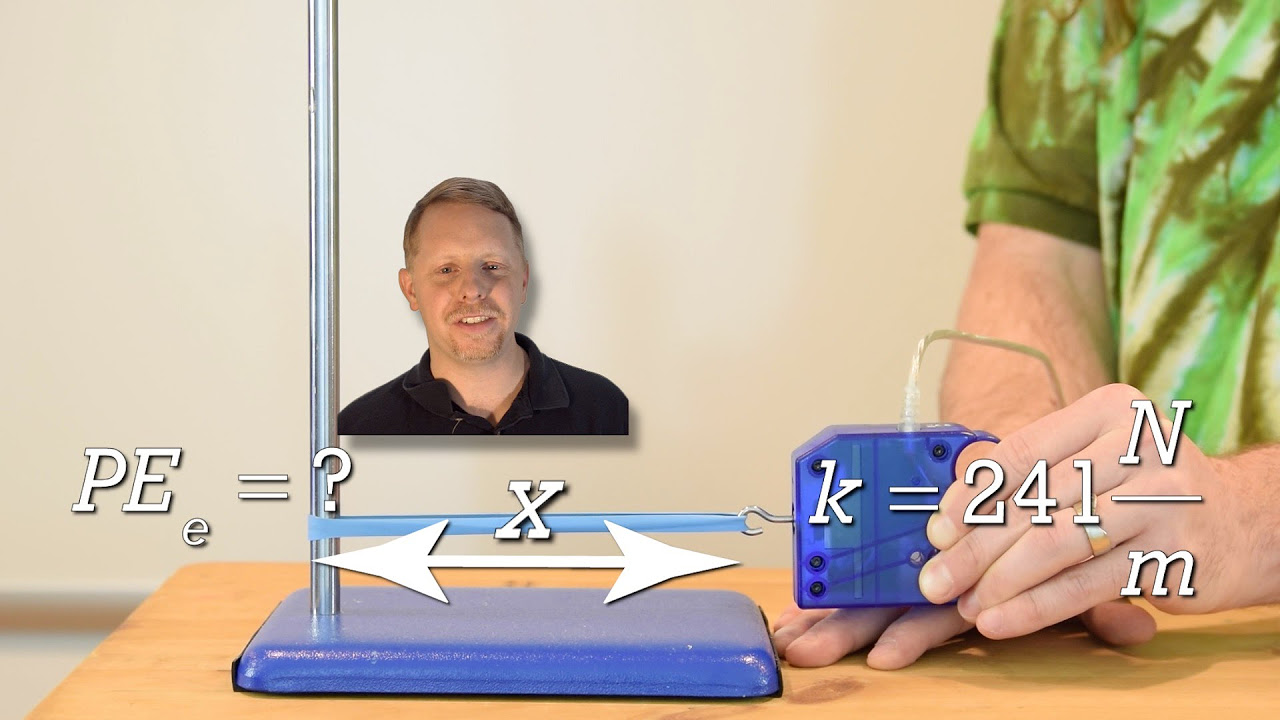
The second paragraph focuses on the calculation of elastic potential energy using the formula PE = 1/2 k x^2. It involves a practical example where a rubber band is elongated by 12 centimeters, and the spring constant is given as 241 Newtons per meter. The calculation is performed by squaring the displacement and multiplying it by half of the spring constant, resulting in 1.7 Joules of stored energy. The paragraph emphasizes the importance of units in the calculation, explaining that Newton-meters equate to Joules. It also touches on the scalar nature of elastic potential energy, similar to kinetic and gravitational potential energy, and ends with a humorous note about the floating head of Mr. Fullerton from APlusPhysics.com.

Mindmap
Keywords
💡Elastic Potential Energy
Elastic potential energy is the energy stored in an object when it undergoes a temporary deformation, such as stretching or compressing. In the video, it is explained as the energy stored in a spring when it is either compressed or elongated. The concept is central to the theme of the video, which is to understand the mechanics of energy storage and conversion in elastic materials. Examples from the script include the spring, the toy with a rubber band, and the rubber ball that stores elastic potential energy when compressed upon striking the ground.
💡Spring Constant
The spring constant, denoted as 'k', is a measure of a spring's stiffness and is defined as the force required to compress or extend the spring by one unit of length. It has units of Newtons per meter (N/m). The video uses the spring constant in the formula for elastic potential energy, emphasizing its importance in determining the amount of energy stored in a spring. Examples include the weak spring with a small spring constant of 65 N/m and the strong spring with a large spring constant of 3,300 N/m.
💡Displacement
Displacement, represented by 'x' in the script, refers to the distance an object is moved from its equilibrium or rest position. In the context of the video, displacement is the amount by which a spring is stretched or compressed. It is a key variable in the formula for elastic potential energy, indicating how much deformation has occurred and thus how much energy is stored. The script illustrates this with the example of a rubber band being pulled to the right, increasing the displacement 'x'.
💡Equilibrium Position
The equilibrium position, also called the rest position, is the position where the net force on an object is zero, and it is in a state of balance. In the video, this is the starting point from which the displacement is measured. The concept is crucial for understanding the reference point for calculating the elastic potential energy stored in a spring or rubber band.
💡Kinetic Energy
Kinetic energy is the energy an object possesses due to its motion. The video mentions that elastic potential energy can be converted into kinetic energy, as seen when a spring is released and its stored energy propels it into motion. This concept is essential for understanding energy transformation in physical systems.
💡Gravitational Potential Energy
Gravitational potential energy is the energy an object possesses due to its position in a gravitational field. The video script mentions that elastic potential energy can also be converted into gravitational potential energy, as occurs when a ball, after being struck and compressed, moves upward against gravity. This illustrates the interplay between different forms of energy in physical systems.
💡Hooke's Law
Although not explicitly defined in the video, Hooke's Law is alluded to in the context of the relationship between force and displacement in a spring. It states that the force needed to compress or extend a spring is proportional to the displacement from its equilibrium position. The video indirectly refers to this law when discussing the linear increase in force as the spring is deformed.
💡Scalar
A scalar is a physical quantity that is described by a magnitude only, without direction. The video script mentions that elastic potential energy, like kinetic and gravitational potential energy, is a scalar quantity. This means that it only has a size and does not have a directional component, which is an important distinction from vector quantities.
💡Deformation
Deformation in the context of the video refers to the change in shape or size of an object due to an applied force. Elastic deformation is temporary, meaning the object can return to its original shape or size once the force is removed. The video uses examples such as a spring, a rubber band, and a rubber ball to illustrate how objects can store elastic potential energy through deformation.
💡Conversion of Energy
The video script discusses the concept of energy conversion, where elastic potential energy can be transformed into other forms of energy, such as kinetic or gravitational potential energy. This is a fundamental principle in physics, demonstrating how energy can change forms but is conserved in a closed system.
💡Significant Digits
Significant digits are the digits in a number that carry meaningful information about its precision. The video script briefly touches on the concept when rounding the calculated elastic potential energy to two significant digits, which is an important aspect of scientific notation and data representation.
Highlights

The symbol for elastic potential energy is PE with a subscript of e or U with a subscript of e.

Elastic potential energy is the energy stored in an object due to its temporary deformation.

Common examples of elastic potential energy include springs, toys with rubber bands, and rubber balls.

Elastic potential energy can be converted to kinetic and gravitational potential energy.

The equation for elastic potential energy is given by PE = 1/2 kx^2, where k is the spring constant and x is the displacement from the equilibrium position.

The spring constant measures how much a spring resists displacement and is given in Newtons per meter.

A weak spring has a small spring constant, while a strong spring has a large spring constant.

The Slinky has a small spring constant because it is easy to deform.

The Pogo stick has a large spring constant because it applies a large force when bounced on.

An example of analyzing elastic potential energy is given using a rubber band attached to a force sensor.

The spring constant of the rubber band is determined to be 241 Newtons per meter.

Elastic potential energy is calculated as 1.7 Joules when the rubber band is elongated by 12 centimeters.

The units of elastic potential energy are Joules, derived from the units of the spring constant and displacement.

Elastic potential energy, like kinetic and gravitational potential energy, is a scalar quantity.

The concept of Hooke's Law is mentioned but not defined in this transcript.

The importance of doing homework to understand concepts like elastic potential energy is emphasized.

The transcript features a guest appearance by Mr. Fullerton of APlusPhysics.com, who assists with the rubber band example.

The transcript ends with a humorous note about owing a soda and a reminder to make the day great.

Transcripts

Browse More Related Video

(previous version) Introduction to Elastic Potential Energy with Examples

Potential Energy

Potential Energy | Science for Kids

Elastic Potential Energy - Introductory Example Problems

Kinetic Energy and Potential Energy

GCSE Physics - Elastic Potential Energy and F = ke Equations #45

Rate This

5.0 / 5 (0 votes)

Thanks for rating:

Related Tags
Elastic EnergySpring ConstantPhysics ConceptKinetic EnergyGravitational EnergyRubber BandSlinkyPogo StickHooke's LawEnergy Conversion