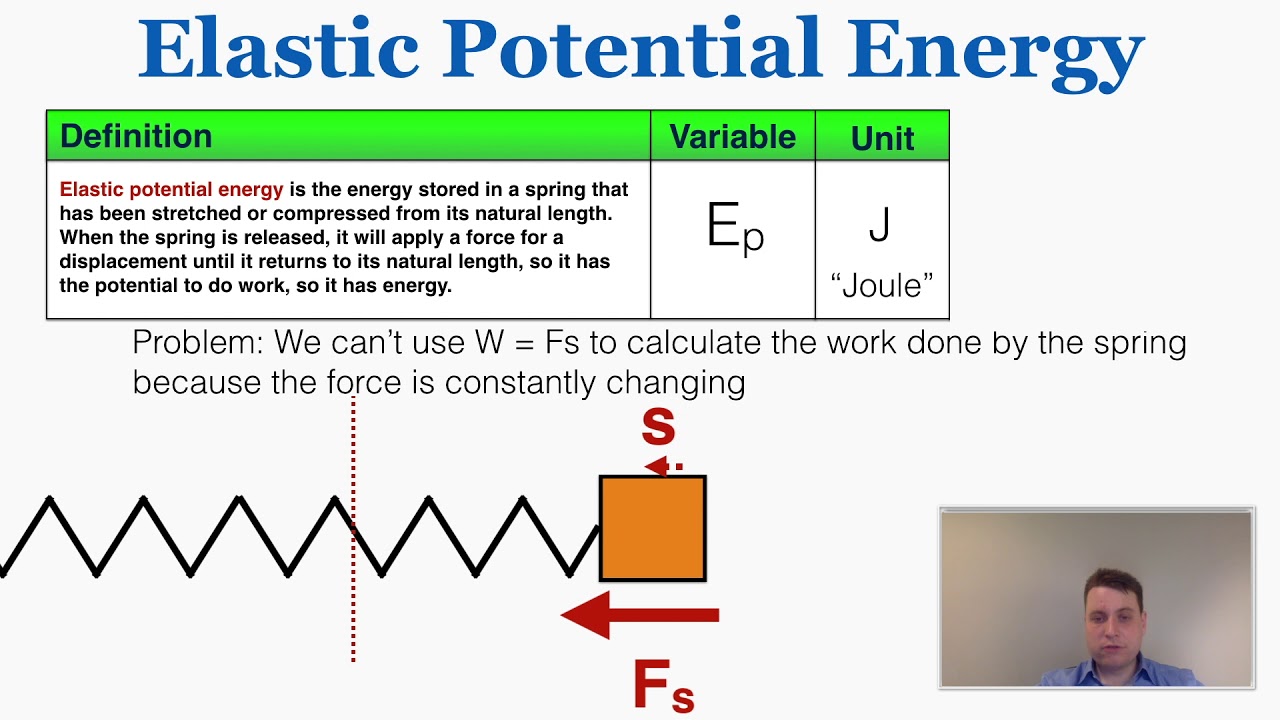
GCSE Physics - Elastic Potential Energy and F = ke Equations #45

Cognito
14 Nov 201904:26
EducationalLearning
32 Likes 10 Comments

TLDRThis video explains two fundamental equations related to elasticity: Hooke's Law (F = kx), which describes the force needed to extend a spring, and the elastic potential energy formula (E = 1/2 kx^2), illustrating the energy stored when a spring is stretched. The video uses an example to calculate the spring constant and potential energy, emphasizing the importance of understanding the elastic limit and Hooke's Law's applicability. It also highlights the graphical representation of these concepts, where the slope of the line in a force-extension graph equals the spring constant, and the area under the curve represents the elastic potential energy.

Takeaways
  • 🔍 The video discusses two fundamental equations related to elasticity: F = kx and elastic potential energy = 1/2 kx^2.
  • 🌟 The spring constant (k) is a specific value for each object, indicating its firmness or elasticity; a lower k means more elastic, while a higher k means stiffer.
  • 📐 The first equation, F = kx, links the force applied to an object to the amount it extends, with x being the extension.
  • 💡 Elastic potential energy is the energy transferred to an object when it's stretched, and it's calculated by considering only the extension squared, not the entire spring's length.
  • 📚 An example is provided where a spring with a natural length of 0.6 meters stretches to 0.8 meters under a force of 14 Newtons, illustrating the calculation of the spring constant and elastic potential energy.
  • 🔢 To find the spring constant, the formula F/x = k is rearranged and used with the given force and extension values.
  • 📉 The elastic potential energy is calculated using the formula 1/2 kx^2, with the values of k and x plugged in to find the energy stored in the spring.
  • 📈 The video explains that a graph of force against extension, where the gradient of the straight line represents the spring constant, and the area under the curve equals the elastic potential energy.
  • 🚫 It's important to note the concept of the elastic limit or the limit of proportionality, beyond which the object no longer obeys Hooke's law.
  • 👍 The video concludes by encouraging viewers to like and subscribe if they found the content useful, indicating the end of the educational segment.
Q & A

  • What are the two key equations discussed in the video?

    -The two key equations discussed are: 1) F = kx, where F is the force applied, k is the spring constant, and x is the extension of the spring. 2) Elastic potential energy = 1/2 kx^2, where k is the spring constant and x is the extension.

  • What does the spring constant (k) represent in the equation F = kx?

    -The spring constant (k) represents the stiffness of the spring. A lower k means the spring is more elastic and easier to stretch, while a higher k means the spring is stiffer and harder to stretch.

  • How is elastic potential energy defined in the video?

    -Elastic potential energy is defined as the energy transferred to an object as it is stretched. It is calculated using the formula 1/2 kx^2, where k is the spring constant and x is the extension.

  • What happens when a spring is stretched using 100 joules of energy?

    -When a spring is stretched using 100 joules of energy, this energy is transferred to the spring's elastic potential energy store. When the spring is released, it transfers this energy back out, typically in the form of kinetic energy.

  • How can you determine the spring constant of a spring if you know the force applied and the extension?

    -You can determine the spring constant by rearranging the equation F = kx to k = F/x and plugging in the known values of force and extension.

  • What is the significance of the elastic limit or the limit of proportionality in the context of Hooke's law?

    -The elastic limit or the limit of proportionality is the point at which the object stops obeying Hooke's law. Beyond this point, the relationship between force and extension is no longer linear.

  • How can you visualize the relationship between force and extension in a spring?

    -You can visualize this relationship by plotting a graph of force against extension. The gradient of the straight part of the line represents the spring constant, and the area under the curve equals the elastic potential energy.

  • What is the formula for calculating the elastic potential energy of a spring, and what does each term represent?

    -The formula for calculating the elastic potential energy is 1/2 kx^2. Here, k is the spring constant, x is the extension, and the term 1/2 represents the fact that only half of the work done is stored as elastic potential energy.

  • What is the natural length of the spring in the example provided in the video?

    -In the example, the natural length of the spring is 0.6 meters.

  • How much does the spring extend when a force of 14 Newtons is applied in the example?

    -In the example, when a force of 14 Newtons is applied, the spring extends from 0.6 meters to 0.8 meters, which is an extension of 0.2 meters.

  • What is the calculated spring constant for the example spring in the video?

    -For the example spring, the calculated spring constant is 70 Newtons per meter, obtained by dividing the force (14 Newtons) by the extension (0.2 meters).

  • What is the elastic potential energy of the spring in the example after it is stretched?

    -The elastic potential energy of the spring in the example is 1.4 joules, calculated using the formula 1/2 kx^2 with k = 70 and x = 0.2.

Outlines
00:00
🔗 Introduction to Elasticity Equations

This paragraph introduces two fundamental equations related to elasticity. The first equation, F = ke, describes the relationship between the force applied to an object and its extension, with 'k' being the spring constant, which varies among objects and indicates their stiffness or elasticity. A lower spring constant suggests more elasticity, while a higher one indicates stiffness. The second equation, elastic potential energy = 1/2 ke^2, defines the energy stored in an object when it is stretched, emphasizing that only the extension is squared, not the entire length. The paragraph also explains that elastic potential energy is the energy transferred to an object when it is stretched and can be converted into other forms, such as kinetic energy, when released.

📏 Calculating Spring Constant and Elastic Potential Energy

This paragraph demonstrates how to apply the elasticity equations through an example. A spring with a natural length of 0.6 meters is stretched to 0.8 meters under a force of 14 Newtons. The extension is calculated by subtracting the initial length from the stretched length, resulting in 0.2 meters. Using the equation F = ke, the spring constant is determined by dividing the force by the extension, yielding 70 Newtons per meter. The elastic potential energy is then calculated using the equation 1/2 ke^2, where the spring constant and extension are plugged in, resulting in 1.4 joules. The paragraph also explains the graphical representation of these concepts, where the gradient of the line in a force-extension graph represents the spring constant, and the area under the curve corresponds to the elastic potential energy.

📚 Recap and Conclusion

The final paragraph recaps the key points discussed in the video. It emphasizes the importance of understanding the elastic limit or the limit of proportionality, which is the point at which an object stops obeying Hooke's law. The video concludes with a reminder of the main concepts and an invitation for viewers to like and subscribe for more content.

Mindmap
Keywords
💡Elasticity
Elasticity refers to the property of a material to return to its original shape after being stretched or compressed. In the context of the video, it is a fundamental concept for understanding the behavior of springs and other elastic objects. The video script explains how elasticity is measured by the spring constant, which is specific to each object and indicates how stiff or elastic the object is.
💡Spring Constant (K)
The spring constant, denoted by 'K', is a measure of the stiffness of a spring. It is a specific value for each object that determines how much force is needed to extend the spring by a certain distance. A lower spring constant means the object is more elastic and easier to stretch, while a higher value indicates a stiffer object. The script uses the formula F = K * e to illustrate how the spring constant is calculated using the force applied and the extension of the spring.
💡Force (F)
Force is the push or pull upon an object resulting from its interaction with another object. In the video, force is applied to a spring, causing it to extend. The script explains that the amount of force needed to stretch a spring is directly related to the spring constant and the extension of the spring, as shown in the formula F = K * e.
💡Extension (e)
Extension is the amount by which an object, such as a spring, is stretched from its natural length. The script provides an example where a spring is stretched from 0.6 meters to 0.8 meters, resulting in an extension of 0.2 meters. This extension is crucial in calculating the spring constant and the elastic potential energy.
💡Elastic Potential Energy
Elastic potential energy is the energy stored in an elastic object, such as a spring, when it is stretched or compressed. The video script defines it as the energy transferred to an object as it is stretched, which can later be released when the object returns to its original shape. The formula used in the script is elastic potential energy = 1/2 * K * e^2, illustrating how the spring constant and extension relate to the energy stored.
💡Energy Transfer
Energy transfer refers to the movement of energy from one form to another or from one object to another. In the context of the video, when a spring is stretched, the energy used to stretch it is transferred to the spring's elastic potential energy. The script explains that if 100 joules of energy are used to stretch a spring, that energy is stored as elastic potential energy.
💡Kinetic Energy
Kinetic energy is the energy an object possesses due to its motion. The script mentions that when a spring is released after being stretched, the stored elastic potential energy is converted into kinetic energy as the spring moves back to its original position.
💡Graph
A graph is a visual representation of data, showing relationships between variables. The script describes a graph of force against extension, where the slope of the straight line represents the spring constant. This graphical representation helps to visualize the relationship between force, extension, and the spring constant.
💡Gradient
Gradient, in the context of a graph, is the rate of change of one variable with respect to another. The script explains that the gradient of the line on a force-extension graph is equal to the spring constant, indicating the stiffness of the spring.
💡Elastic Limit
Elastic limit, also known as the limit of proportionality, is the point beyond which a material no longer obeys Hooke's Law and may not return to its original shape after being deformed. The script mentions this concept to highlight the point at which the relationship between force and extension no longer holds true.
💡Hooke's Law
Hooke's Law states that the force needed to extend or compress a spring by some distance is proportional to that distance, as long as the elastic limit is not exceeded. The script refers to this law to explain the linear relationship between force and extension within the elastic limit.
Highlights

The video discusses two key equations related to elasticity.

The first equation is F = k * e, where F is the force applied, k is the spring constant, and e is the extension.

The spring constant (k) is specific to each object and measures its firmness or elasticity.

A lower spring constant indicates more elasticity, making the object easier to stretch.

A higher spring constant suggests the object is stiffer and harder to stretch.

The second equation is elastic potential energy = 1/2 * k * e^2.

Elastic potential energy represents the energy transferred to an object when it's stretched.

The energy stored in the spring is released as kinetic energy when the spring returns to its original state.

An example is given where a spring with a natural length of 0.6 meters is stretched to 0.8 meters by a force of 14 Newtons.

The extension of the spring is calculated by subtracting the natural length from the stretched length.

The spring constant is determined by rearranging the first equation and using the known force and extension.

In the example, the spring constant is calculated to be 70 Newtons per meter.

The elastic potential energy of the spring is then calculated using the second equation with the known spring constant and extension.

The calculated elastic potential energy for the example is 1.4 Joules.

A graph of force against extension illustrates the spring constant as the gradient of the straight line.

The area under the curve on the graph represents the elastic potential energy.

The elastic limit or limit of proportionality is the point where the object no longer obeys Hooke's law.

The video concludes with a recap of the key points and an invitation for viewers to like and subscribe.

Transcripts

Browse More Related Video

(previous version) Introduction to Elastic Potential Energy with Examples

Simple Harmonic Motion, Mass Spring System - Physics Full Topic

Hooke's Law and Elastic Potential Energy

Elastic Potential Energy - Introductory Example Problems

High School Physics - Springs and Hooke's Law

Elastic Potential Energy - IB Physics

Rate This

5.0 / 5 (0 votes)

Thanks for rating:

Related Tags
ElasticitySpring ConstantPotential EnergyPhysicsForceExtensionHooke's LawEnergy TransferMechanical EnergyEducational