Lecture 18 Experimental Designs; Completely Randomized Design CRD; One Way ANOVA

Benish Ali

13 Jul 202024:39

EducationalLearning

32 Likes 10 Comments

TLDRThe video script delves into the Completely Randomized Design (CRD), a fundamental experimental design used in field research, particularly in lab settings with controlled environments. It explains how treatments, representing categories of the independent variable, are randomly assigned to experimental units to measure the response of the dependent variable. The script uses an example of plant growth with different fertilizer doses to illustrate CRD's process, including replication, randomization, and statistical analysis via one-way ANOVA. The conclusion from the example suggests no significant effect of fertilizer doses on plant fresh weight, demonstrating the application and outcome interpretation of CRD.

Takeaways

🔬 The script discusses the Completely Randomized Design (CRD), a fundamental experimental design used in field research.
🧪 CRD involves assigning treatments to experimental units completely at random, assuming each unit has an equal chance of receiving any treatment.
🌱 Treatments are the different categories of the independent variable applied to see the response of the dependent variable from the experimental units.
⚖️ The purpose of an experiment is to observe the differences in the response of experimental units to various treatments, considering the role of experimental error.
🏡 CRD is suitable for homogeneous experimental units, like lab experiments where environmental conditions can be easily controlled.
📊 The script highlights the importance of replication in CRD, ensuring enough replicates of the same treatment to reduce experimental error.
🎲 The principle of randomization in CRD is crucial, as it helps in minimizing the effect of extraneous factors and reducing experimental error.
📈 The script provides an example of using CRD to study the effects of different fertilizer doses on the fresh weight of plants, emphasizing the application of one-way ANOVA for statistical analysis.
📊 The example demonstrates the process of calculating descriptive statistics, variance, and sum of squares for CRD, which are essential for ANOVA.
📉 The F max test is used to check the homogeneity of variances between treatments, a critical step before proceeding with ANOVA.
📝 The final ANOVA summary table and the interpretation of the F value indicate whether the effect of treatments is significant, with the example showing no significant effect of fertilizer doses on plant growth.

Q & A

What is a Completely Randomized Design (CRD) in experimental design?
-A Completely Randomized Design (CRD) is an experimental design where treatments are assigned completely at random to the experimental units, meaning each unit has the same chance of receiving any treatment.
What are treatments in the context of CRD?
-Treatments are the categories of the independent variable that are applied to the experimental units to observe the response of the dependent variable.
Why is it important to keep experimental units under similar conditions in CRD?
-Keeping experimental units under similar conditions helps to reduce experimental error by minimizing the effects of extraneous factors that cannot be controlled.
What is the purpose of replication in CRD?
-Replication in CRD ensures that there are enough experimental units receiving the same treatment to allow for the assessment of the treatment's effect and to reduce the impact of experimental error.
How does randomization help in reducing experimental error in CRD?
-Randomization in CRD helps in reducing experimental error by assigning treatments to experimental units in a way that assumes all units are under the same environmental conditions, thus minimizing the influence of extraneous factors.
What is the role of the response variable in CRD?
-The response variable in CRD is the dependent variable whose measurement is used to assess the effect of the treatments applied to the experimental units.
What statistical method is used to analyze data from a CRD?
-One-way ANOVA is commonly used to analyze data from a CRD, especially when there is one independent variable with multiple levels and one measurement variable.
What is the significance of the F max test in the context of CRD and ANOVA?
-The F max test is used to check the homogeneity of variances between treatments in CRD. It compares the largest variance to the smallest, and if the ratio is not significantly different from unity, it suggests that variances are homogeneous.
How is the total sum of squares calculated in ANOVA for CRD?
-The total sum of squares in ANOVA for CRD is calculated as the sum of the squared deviations of each treatment mean from the overall mean, adjusted by the correction term.
What does a non-significant F value in ANOVA indicate about the treatments in CRD?
-A non-significant F value in ANOVA for CRD indicates that there is no significant effect of the treatments on the response variable, suggesting that the different treatments do not affect the outcome.
Can you provide an example of how CRD is applied in a real-world scenario?
-An example of CRD application is in agricultural research, where different fertilizer doses are randomly assigned to plants to study their effect on the fresh weight of the plants, with the fresh weight being the response variable.

Outlines

00:00

🔬 Introduction to Completely Randomized Design (CRD)

The video script introduces the concept of the Completely Randomized Design (CRD), an experimental design used in field research. It explains the basic principles of experimental design, including the assignment of treatments to experimental units at random, with the aim of observing the response of the dependent variable. The script also mentions other types of experimental designs, such as Randomized Complete Block Design (RCBD) and Latin Square Design. The focus is on the homogeneity of experimental units and the minimization of extraneous factors to reduce experimental error. An example of applying CRD in a lab setting with 30 experimental units and 3 treatments is provided to illustrate the process.

05:01

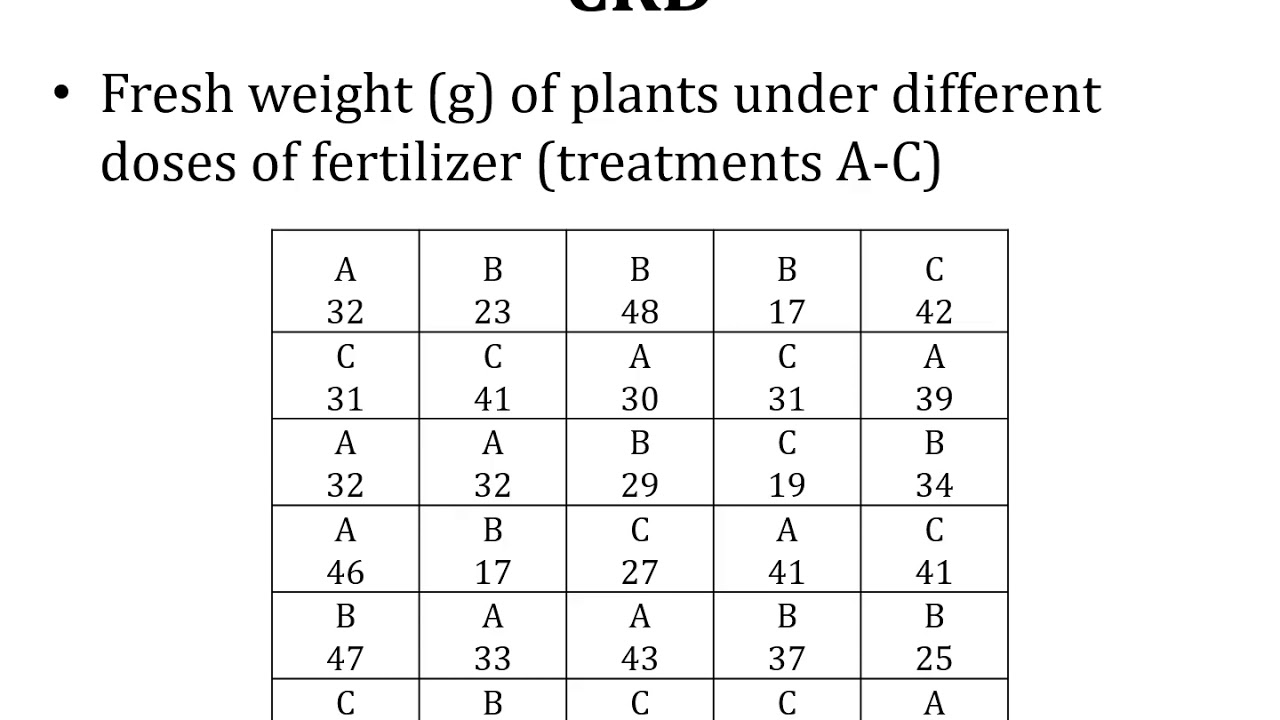
🌱 Sources of Variation in CRD and Example with Fertilizer

This paragraph delves into the sources of variation in CRD, which include treatments and experimental error. It emphasizes the importance of reducing experimental error to isolate the effects of treatments. The script uses an example of a study on the effects of different fertilizer doses on the fresh weight of plants to demonstrate how CRD is applied. The example includes the setup of the experiment, data collection, and the fulfillment of experimental design principles such as local control, replication, and randomization.

10:03

📊 Descriptive Statistics and ANOVA Preparation

The script outlines the process of statistical analysis using one-way ANOVA for the data obtained from the CRD experiment. It begins with the calculation of descriptive statistics for the different treatments, including the mean fresh weight of plants for each fertilizer dose. The script also explains the importance of testing for homogeneity of variances using the F max test before proceeding with ANOVA. The preparation for ANOVA includes the calculation of the correction term and the total sum of squares.

15:04

⚗️ Calculation of Sum of Squares and Degrees of Freedom in ANOVA

This paragraph details the steps involved in calculating the sum of squares for treatments and experimental error within the context of ANOVA. It explains how to derive the individual sum of squares for each sample and how these contribute to the total sum of squares for error. The script also covers the calculation of degrees of freedom for total, treatment, and error, which are essential components for the subsequent variance and F tests.

20:05

📉 F Test and Conclusion of ANOVA for Fertilizer Effect

The final paragraph discusses the F test, which compares the variances due to treatments and experimental error to determine significance. The script calculates the F value and compares it with the critical F value from the F distribution table to assess the significance of the treatment effect on the fresh weight of plants. The conclusion from the ANOVA indicates that there is no significant effect of different fertilizer doses on the fresh weight of plants, as the calculated F value is less than the critical value, suggesting that the variance within treatments is not significantly different from the experimental error.

Mindmap

Keywords

💡Completely Randomized Design (CRD)

Completely Randomized Design (CRD) is an experimental design where treatments are assigned to experimental units at random. It is the simplest type of experimental design and is used to test the effects of different treatments on a response variable. In the video, CRD is used to study the impact of various fertilizer doses on the fresh weight of plants. The script emphasizes that CRD is appropriate for homogeneous experimental units and controlled conditions, such as laboratory settings.

💡Experimental Units

Experimental units are the individual subjects or objects to which treatments are applied in an experiment. In the context of the video, the experimental units are the plants that receive different fertilizer treatments. The script explains that each experimental unit has an equal chance of receiving any of the treatments, which is a key aspect of CRD.

💡Treatments

Treatments are the different conditions or interventions applied to the experimental units to observe their effects on the dependent variable. In the video, treatments refer to the various doses of fertilizers assigned to the plants. The script clarifies that treatments are categories of the independent variable and are a central component of CRD.

💡Dependent Variable

The dependent variable is the outcome or response that is measured in an experiment to assess the effect of the independent variable. In the video, the fresh weight of plants serves as the dependent variable, with the script highlighting that any differences in this measurement among units receiving the same treatment are considered experimental error.

💡Experimental Error

Experimental error refers to the variation in the response variable that arises from factors other than the treatments. The script explains that this variation comes from uncontrollable extraneous factors and is an important consideration in CRD, as it can affect the accuracy of the results.

💡Homogeneous Environmental Conditions

Homogeneous environmental conditions mean that all experimental units are kept under similar circumstances to minimize the impact of extraneous factors on the experiment. The script uses this term to describe why CRD is suitable for lab experiments where conditions can be easily controlled, thus reducing experimental error.

💡Replication

Replication in an experiment refers to the practice of repeating treatments under the same conditions to ensure reliability and reduce error. The script illustrates this with the example of 30 plants, each receiving one of three fertilizer treatments, with ten replicates for each treatment, ensuring the validity of the experimental results.

💡Randomization

Randomization is the process of assigning treatments to experimental units in a random manner to control for confounding variables. The script emphasizes that in CRD, treatments are applied completely at random, as seen in the example with the 30 plants, to ensure that the experimental units are treated equally and to reduce bias.

💡One-Way ANOVA

One-Way ANOVA is a statistical method used to compare the means of three or more independent groups to determine if there are significant differences between them. The script describes using one-way ANOVA to analyze the data from the CRD experiment, comparing the mean fresh weight of plants across different fertilizer treatments.

💡Fertilizer Doses

Fertilizer doses refer to the varying amounts of fertilizer used in the experiment. The script uses this term to describe the independent variable in the example, where different doses of fertilizer are applied to plants to observe their effect on the fresh weight, which is the dependent variable.

💡Significance

In the context of the video, significance refers to the statistical importance of the observed effects or differences. The script concludes that there is no significant effect of different fertilizer doses on the fresh weight of plants, as indicated by the non-significant F-value obtained from the one-way ANOVA.

Highlights

CRD (Completely Randomized Design) is an experimental design where treatments are assigned completely at random.

Treatments represent categories of the independent variable applied to experimental units to observe the response of the dependent variable.

In CRD, each experimental unit has an equal chance of receiving any treatment, ensuring randomness in assignment.

CRD is suitable for homogeneous experimental units and controlled environments like lab experiments.

The purpose of an experiment is to observe differences in the response of experimental units to various treatments.

Variation in response among units receiving the same treatment is attributed to experimental error due to uncontrollable extraneous factors.

CRD is simple and basic, appropriate for lab experiments with easily controlled environmental conditions.

Replication in CRD ensures multiple experimental units receive the same treatment, allowing for comparison.

Randomization in CRD is crucial for reducing experimental error by assuming all units are under similar conditions.

Sources of variation in CRD are limited to treatments and experimental error, focusing on the effects of treatments.

Total variability in CRD is the sum of variability due to treatments and experimental error.

Example given demonstrates the application of CRD in studying the effects of fertilizer doses on the fresh weight of plants.

Descriptive statistics table is used for the initial analysis of data obtained from CRD.

F max test is used to check the homogeneity of variances between treatments in CRD.

One-way ANOVA is applied for statistical analysis of CRD data when there is one independent variable.

CRD results may show no significant effect of treatments if the calculated F value is less than the critical value.

ANOVA summary table organizes the results, including sources of variation, sum of squares, degrees of freedom, MS, and F values.

In the given example, the result of CRD using one-way ANOVA indicated no significant effect of different fertilizer doses on plant fresh weight.

Transcripts

Browse More Related Video

Experimental Designs; CRD; Completely Randomized Design; One-Way ANOVA

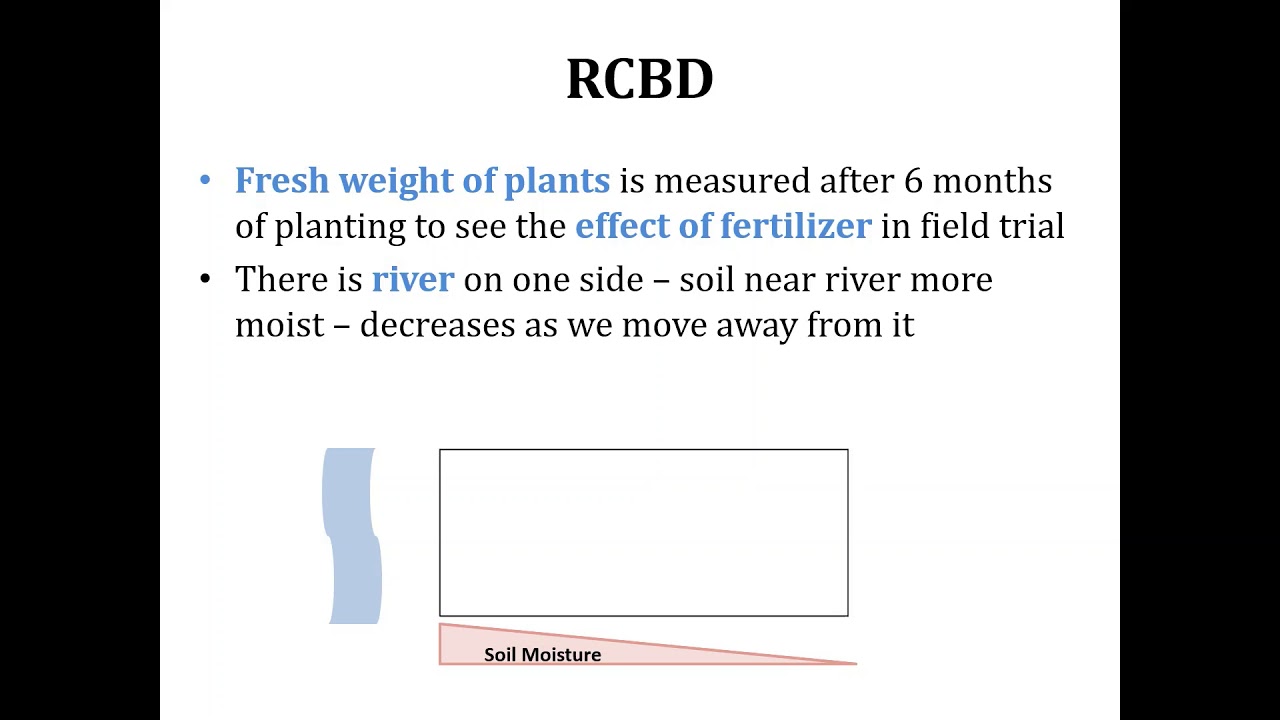
Experimental Designs; Randomized Complete Block Design; RCBD; Two-Way ANOVA

Types of Experimental Research Designs - CRD RBD RCBD LSD FD - Research Methods - Practical Research

Analyse data from experiments with completely randomised design (CRD)

Types of Experimental Designs (3.3)

Introduction to Experimental Designs; Principles; Randomization; Replication; Local Control

Related Tags

Experimental Design CRD Agriculture Randomization Fertilizer Effect Plant Growth Statistical Analysis ANOVA Homogeneous Units Research Method Lab vs Field