Types of Experimental Designs (3.3)
TLDRThis video script explores three types of experimental designs: completely randomized, randomized block, and matched pairs designs. It illustrates how each design can be applied in different scenarios, such as determining the best study environment for university students, comparing gasoline efficiency, or assessing the impact of sleep deprivation on test scores. The script explains the process of assigning experimental units, using gender as a blocking variable, and matching similar units to ensure fair and controlled comparisons.
Takeaways
- 🔬 **Completely Randomized Design**: Each experimental unit is randomly assigned to a group to receive a different treatment, with the aim to compare results across treatments.
- 📚 **Example of CRD**: A study on the best environment for studying, with three treatments (library, own room, outside) and 30 students randomly divided into three groups.
- 👥 **Randomized Block Design**: Experimental units are first grouped into blocks based on a characteristic expected to influence the response, followed by random assignment within blocks.
- 👧👦 **Gender as a Blocking Variable**: In the example, gender is used to divide students into male and female blocks before random assignment to treatments.
- 🚗 **Matched Pairs Design**: This design compares two treatments using the same or similar experimental units, ensuring a direct comparison.
- 🛣️ **Efficiency of Gasoline**: A matched pairs example where the same cars are used to test the efficiency of type A and type B gasoline.
- 💤 **Sleep Deprivation Study**: A scenario where similar experimental units (students with similar GPA) are used to compare the effects of sleep deprivation on test scores.
- 🔄 **Random Order of Treatments**: In matched pairs designs, the order of treatments is randomized to avoid order effects.
- 📊 **Comparing Results**: The outcome of experiments is to compare results within treatments and across groups to determine the effects.
- 📈 **Inference on Causation**: Matched pairs design allows for making inferences about causation, such as sleep deprivation causing lower test scores.
- 📝 **Diagrammatic Representation**: The script explains the use of diagrams to represent different experimental designs for clarity.
Q & A
What are the three types of experimental designs discussed in the video?
-The three types of experimental designs discussed are the completely randomized design, the randomized block design, and the matched pairs design.
How is a completely randomized design different from the other two designs mentioned?
-In a completely randomized design, each experimental unit is randomly assigned to a group to receive a different treatment without considering any specific characteristics of the units.
Can you explain the example given for a completely randomized design in the video?
-The example involves determining the best environment for studying, comparing a library, one's own room, and outside. Thirty university students are randomly assigned into three groups to receive these three treatments, with 10 students per group.
What is the purpose of using a randomized block design?
-A randomized block design is used when the researcher believes that a certain characteristic, like gender in the example, could influence the results. It first groups experimental units based on this characteristic before assigning them to treatments within each group.
How are experimental units assigned in a randomized block design?
-In a randomized block design, experimental units are first grouped into blocks based on a relevant characteristic, and then within each block, they are randomly assigned to different groups to receive the treatments.
What is the scenario used to illustrate the matched pairs design in the video?
-The scenario involves comparing the efficiency of two types of gasoline using three cars. Each car receives both types of gasoline, and the order of treatments is randomized.
How does a matched pairs design differ when using the same experimental units versus similar ones?
-When using the same experimental units, each unit receives both treatments. When using similar units, pairs of units with similar characteristics are created, and then each pair is split and assigned to one of the two treatments.
Why can't the same experimental units be used in the sleep deprivation and test scores example?
-The same experimental units cannot be used because having the same person take the same test twice would be unfair, as they would know the content from the first attempt.
What is the alternative method suggested in the sleep deprivation example for assigning treatments?
-The alternative method involves pairing students with similar GPAs and then randomly assigning one member of each pair to be sleep-deprived and the other to have a normal amount of sleep.
How are the results analyzed in a matched pairs design?
-In a matched pairs design, the results are analyzed by comparing the outcomes for each pair and then comparing all the results to determine the effect of the treatments.
What is the significance of comparing results within each block in a randomized block design?
-Comparing results within each block allows the researcher to control for the influence of the blocking variable, such as gender, and better isolate the effect of the treatments being studied.
Outlines
🔬 Experimental Design Overview
This paragraph introduces the concept of experimental designs, highlighting three main types: completely randomized design, randomized block design, and matched pairs design. It explains that in a completely randomized design, experimental units are randomly assigned to groups to receive different treatments, and the results are compared to assess the impact of these treatments. The paragraph also provides an example of how this design could be applied in a study to determine the best environment for studying, involving 30 university students and three different environments. It further discusses the randomized block design, which accounts for a characteristic that may influence the results, using gender as an example to demonstrate how subjects are grouped and assigned treatments within blocks. Lastly, it touches on the matched pairs design, which compares two treatments using the same or similar experimental units, with examples of testing gasoline efficiency and the impact of sleep deprivation on test scores.
📊 Recap of Experimental Designs
The second paragraph serves as a recap of the experimental designs discussed earlier. It reiterates the process of the completely randomized design, emphasizing the random assignment of experimental units to groups for treatment comparison. The randomized block design is also summarized, explaining that subjects are first grouped based on a characteristic that may affect their response to treatments, followed by a randomized assignment within these blocks. The paragraph then revisits the matched pairs design, outlining two scenarios: one where the same experimental units receive both treatments, and another where similar units are used due to constraints, such as in the case of studying the effects of sleep deprivation on test scores. The summary concludes with a simplified diagram for each design, providing a visual representation of the process and structure of the experimental setups.
Mindmap
Keywords
💡Experimental Design
💡Completely Randomized Design
💡Randomized Block Design
💡Matched Pairs Design
💡Treatment
💡Blocking Variable
💡Experimental Units
💡Random Assignment
💡GPA
💡Sleep Deprivation
💡Efficiency
Highlights
The video discusses three types of experimental designs: completely randomized design, randomized block design, and matched pairs design.
In a completely randomized design, each experimental unit is randomly assigned to a group to receive a different treatment.
The researcher compares the results from each treatment group at the end of the experiment.
An example is given where 30 university students are randomly assigned to three groups to determine the best environment for studying.
If gender is believed to influence results, a randomized block design is used, first separating subjects by gender.
In the randomized block design, subjects within each gender block are then randomly assigned to treatment groups.
A matched pairs design compares two treatments using the same or similar experimental units.
An example of a matched pairs design is determining the efficiency of two types of gasoline using the same three cars.
In the gasoline example, each car receives both types of gasoline in a randomized order to compare efficiency.
For experiments where the same experimental units cannot be used twice, similar units are matched and assigned different treatments.
An example of using similar units is a study on the effect of sleep deprivation on test scores, matching students by GPA.
In the sleep deprivation study, one student in each GPA-matched pair is sleep-deprived while the other has normal sleep.
The results of the matched pairs are compared to determine if sleep deprivation affects test scores.
A diagram is provided to illustrate the completely randomized design with any number of groups.
The randomized block design diagram shows blocks based on a characteristic, with a completely randomized design within each block.
Two scenarios of the matched pairs design are presented: using the same units and using similar units for comparison.
The matched pairs design using the same units has each unit receive both treatments in a randomized order.
The matched pairs design using similar units involves pairing up subjects and then randomly assigning each pair to a treatment.
Transcripts
Browse More Related Video

Types of Experimental Research Designs - CRD RBD RCBD LSD FD - Research Methods - Practical Research
Experimental Designs; Randomized Complete Block Design; RCBD; Two-Way ANOVA
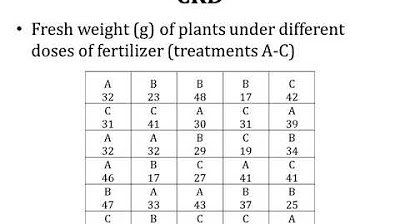
Lecture 18 Experimental Designs; Completely Randomized Design CRD; One Way ANOVA
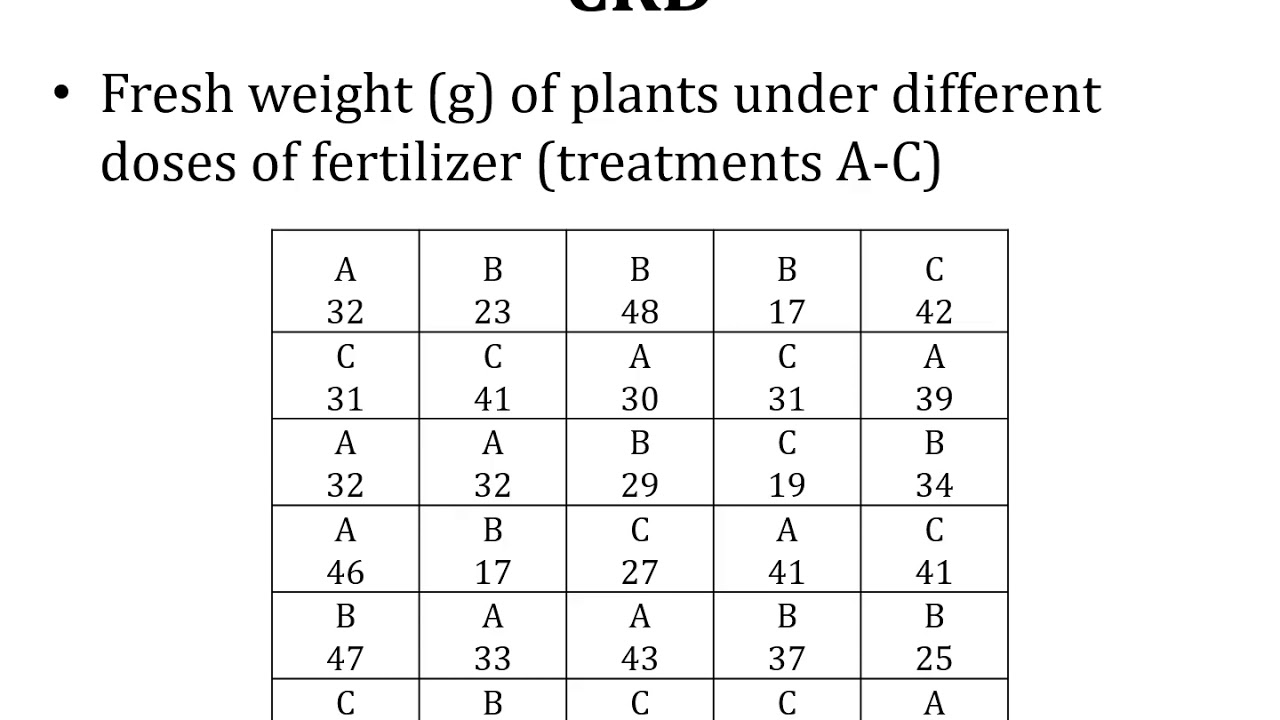
Experimental Designs; CRD; Completely Randomized Design; One-Way ANOVA
Study Designs (Cross-sectional, Case-control, Cohort) | Statistics Tutorial | MarinStatsLectures

Analyse data from experiments with completely randomised design (CRD)
5.0 / 5 (0 votes)
Thanks for rating: