Rational Expressions Review
TLDRThis comprehensive lesson delves into the intricacies of rational expressions, a fundamental algebraic skill essential for tackling trigonometric equations and simplifying trigonometric identities. The instructor emphasizes the importance of factoring and working with fractions that contain polynomials or trigonometric functions. The video covers how to multiply, add, subtract, and divide rational expressions, as well as how to simplify them by identifying and dividing out the greatest common factor. The process involves converting division problems into multiplication by the reciprocal and factoring expressions before reducing them. The lesson also addresses adding and subtracting fractions, which requires finding a common denominator, a concept that becomes more complex with binomials. Furthermore, the instructor explores complex fractions, demonstrating how to rewrite them as multiplication problems or to find a common denominator for addition and subtraction. The summary serves as a review and preparation for applying these algebraic skills in the context of trigonometry.
Takeaways
- 📚 Familiarize with rational expressions, which are fractions containing polynomials or trigonometric functions.
- 🚫 Remember that a rational expression is undefined when its denominator equals zero, which is a restriction.
- ⛔ Identify and avoid reducing fractions where terms do not share a common factor, as they are not reducible.
- ✅ Factor out the greatest common factor (GCF) from the numerator and denominator to simplify rational expressions.
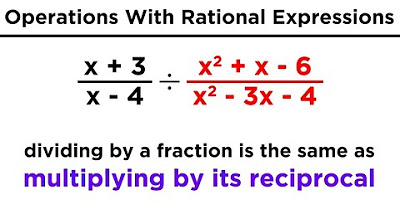
- 🔄 Convert division problems into multiplication by the reciprocal, and factor where possible to simplify further.
- 🔢 Apply the same rules for multiplying and dividing fractions to rational expressions, including factoring and reducing.
- 🔁 When adding or subtracting fractions, find a common denominator after factoring, then combine numerators and simplify.
- 🧩 For complex fractions, rewrite division as multiplication by the reciprocal of the divisor, and factor to simplify.
- 🔄 In complex fractions with addition or subtraction, find a common denominator for all terms before combining numerators.
- 📉 When dealing with binomials, treat each binomial factor as separate when determining a common denominator.
- 📈 Practice is key for mastering the algebraic skills needed for future work with trigonometric equations and identities.
Q & A
What is the main focus of the lesson in the transcript?
-The main focus of the lesson is a review of rational expressions, which is essential for solving trigonometric equations and simplifying trigonometric identities.
What is a rational expression?
-A rational expression is a fraction that contains polynomials in the numerator and/or the denominator, rather than just numbers.
Why is it important to identify restrictions in the denominator of a rational expression?
-Restrictions in the denominator are important because they can create zeros in the denominator, which is undefined in mathematics. Identifying these restrictions helps to determine the domain of the function.
What is the process of reducing a rational expression?
-Reducing a rational expression involves dividing every term in the fraction by the greatest common factor (GCF) to simplify the expression.
When can a rational expression be reduced?
-A rational expression can be reduced if all terms in the fraction contain a common factor, which is the GCF.
How does factoring relate to the process of simplifying rational expressions?
-Factoring is a preliminary step that can be used to identify common factors in a rational expression before reducing it. It helps to simplify the expression by breaking down the terms into their simplest components.
What is the difference between multiplying and dividing rational expressions?
-When multiplying rational expressions, you multiply the numerators together and the denominators together. When dividing, you multiply the first fraction by the reciprocal of the second fraction, effectively turning the division into a multiplication problem.
What is the least common multiple (LCM) and how is it used in adding or subtracting fractions?
-The least common multiple is the smallest number that all the denominators can divide into. It is used to find a common denominator for fractions, which is necessary before performing addition or subtraction.
How do you approach adding or subtracting fractions with binomial denominators?
-You need to ensure that the binomial factors are considered separately from the single terms. You find a common denominator that includes each unique binomial and single term factor from all the denominators.
What is a complex fraction and how is it simplified?
-A complex fraction is a fraction where the numerator or the denominator itself contains a fraction. It is simplified by first finding a common denominator for all terms if necessary, then focusing on simplifying the numerator, and finally reducing by dividing out common factors.
Why is it necessary to factor expressions before reducing them?
-Factoring expressions before reducing them helps to identify common factors more easily, which can then be divided out to simplify the expression. It is a step that can make the process of simplification more efficient.
Outlines
📚 Introduction to Rational Expressions and Factoring
The video begins with an introduction to rational expressions, emphasizing their importance in solving trigonometric equations and simplifying trigonometric identities. The presenter explains that rational expressions are fractions containing polynomials and possibly restrictions in the denominator, which can affect the validity of the expression. The process of simplifying fractions is discussed, along with the conditions under which rational expressions can be reduced by finding their greatest common factor (GCF). Examples are provided to illustrate when and how to reduce expressions, including the need for factoring before reduction in certain cases.
🔍 Reducing Rational Expressions and Factoring Techniques
This paragraph delves deeper into the reduction of rational expressions by dividing out the GCF. It provides a step-by-step guide on how to identify and use common factors to simplify expressions. The presenter also addresses scenarios where factoring is necessary before reduction can take place. Examples illustrate the process of reducing fractions, including those that require preliminary factoring to reveal common factors. The concept of irreducible fractions is introduced, and the importance of recognizing when a fraction cannot be simplified further is highlighted.
🤝 Multiplication and Division of Rational Expressions
The presenter moves on to the multiplication and division of rational expressions, explaining that these operations are similar to those performed with normal fractions. The process of rewriting division problems as multiplication by the reciprocal is discussed, along with the importance of factoring to simplify problems. Techniques for reducing expressions after factoring are shown, and the video emphasizes the need to look for common factors across the entire numerator or denominator to simplify the expression effectively.
🔢 Adding and Subtracting Rational Expressions
The paragraph focuses on the rules for adding and subtracting rational expressions, which requires having a common denominator. The process of finding the least common multiple (LCM) for the denominators is explained, and the importance of factoring the denominators before finding the LCM is emphasized. The presenter demonstrates how to combine like terms and simplify the numerator once a common denominator has been established. The video also clarifies common mistakes, such as incorrectly assuming a common denominator without proper factoring.
🧩 Working with Complex Fractions
The video concludes with a discussion on complex fractions, which are fractions that contain other fractions, either in the numerator or the denominator. The presenter outlines the steps for simplifying complex fractions, including rewriting division as multiplication by the reciprocal and factoring where possible. The process of finding a common denominator for all terms in a complex fraction is demonstrated, showing how to simplify the expression by focusing on the numerators once the denominators cancel each other out. The video provides examples of both friendly and less friendly complex fractions, offering strategies for each case.
📝 Summary of Algebra Skills and Application to Trigonometry
In the final paragraph, the presenter summarizes the algebra skills reviewed in the video, including simplifying fractions, multiplying, dividing, adding, and subtracting rational expressions, and working with complex fractions. The importance of these skills in the context of trigonometry is highlighted, as they will be applied when dealing with trigonometric functions and equations. The presenter reassures viewers that while the review may seem extensive, it is a necessary preparation for the upcoming trigonometric lessons and encourages viewers to practice these skills.
Mindmap
Keywords
💡Rational Expressions
💡Factoring
💡Greatest Common Factor (GCF)
💡Reduction
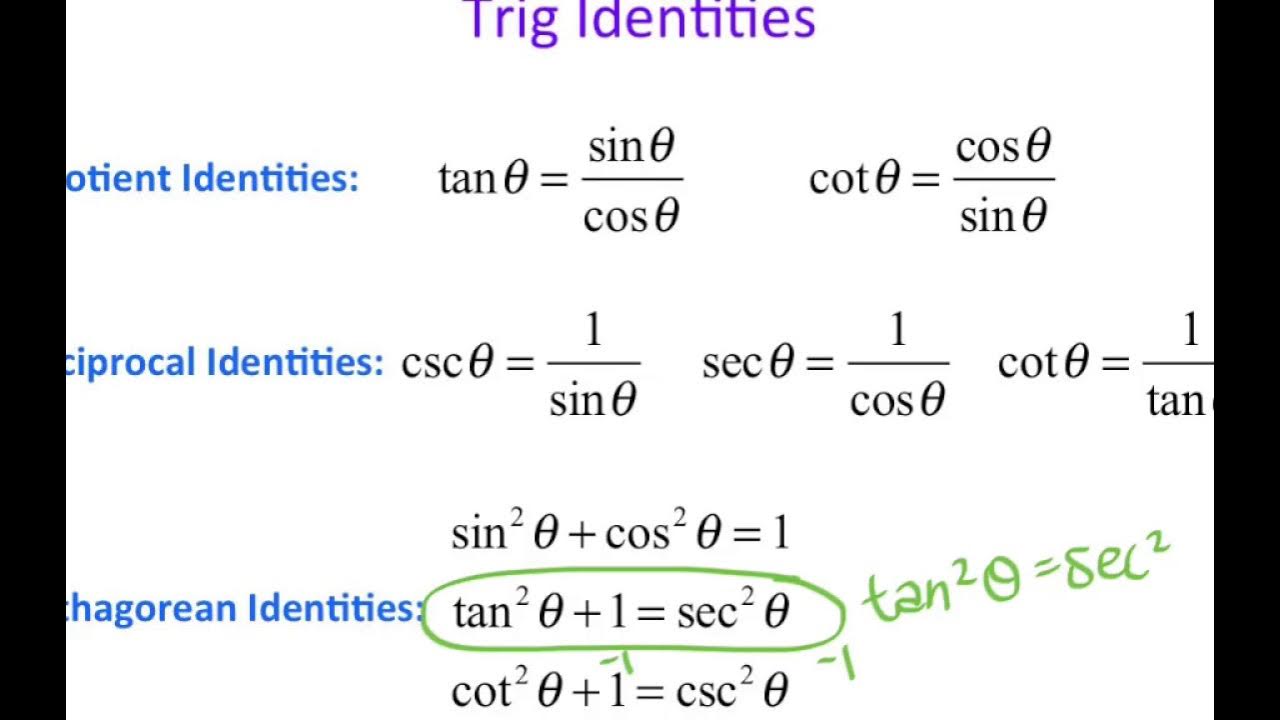
💡Trigonometric Functions
💡Least Common Denominator (LCD)
💡Complex Fractions
💡Reciprocal
💡Difference of Squares
💡Simplifying
💡Trigonometric Identities
Highlights
Introduction to rational expressions review in preparation for solving trigonometric equations and simplifying trig identities.
Emphasis on the importance of factoring and working with non-numerical fractions, which are key algebra skills for trigonometric expressions.
Explanation of the concept of rational expressions, including fractions of polynomials and trig functions, with potential restrictions.
Clarification on when a fraction is considered rational based on the denominator not creating a zero value.
Guidelines on reducing rational expressions by identifying and dividing out the greatest common factor (GCF).
Illustration of when a fraction is not reducible due to the lack of common factors among its terms.
Technique of factoring expressions before reducing, which is essential for simplifying complex fractions.
Process of simplifying fractions by dividing terms in the numerator and denominator by the GCF.
Approach to multiplying and dividing rational expressions, including the conversion of division into multiplication by the reciprocal.
Method for adding and subtracting fractions with different denominators by finding a common denominator.
Strategy for factoring denominators to find the least common multiple (LCM) for adding or subtracting fractions.
Procedure for combining numerators and keeping a common denominator after addition or subtraction of fractions.
Technique for simplifying the result of added or subtracted fractions by factoring and reducing if possible.
Handling of complex fractions by converting division into multiplication and factoring to simplify.
Solution approach for complex fractions involving addition or subtraction by finding a common denominator for all terms.
Shortcut method for complex fractions by focusing on numerators once a common denominator has been established.
Final simplification of complex fractions by canceling out common denominators and factoring the numerators.
Relevance of these algebra skills to upcoming topics in trigonometry, emphasizing their practical application.
Transcripts
Browse More Related Video
5.0 / 5 (0 votes)
Thanks for rating: