10.2.4 Regression - Outliers and Influential Points

Sasha Townsend - Tulsa
2 Dec 202005:57
EducationalLearning
32 Likes 10 Comments

TLDRIn this video, we discussed learning outcome number four for lesson 10.2, focusing on outliers and influential points in scatter plots. The goal was to help viewers define and identify outliers and influential points. We explained that an outlier is a point far from other data points, while an influential point significantly affects the regression line. We used an example involving chocolate consumption and Nobel laureates to illustrate these concepts. The video emphasized using tools like Excel to graph regression lines and determine the impact of individual data points. The session concluded with a preview of the next topic, the least squares property.

Takeaways
  • 📌 Outliers are data points that lie far away from the rest of the data in a scatter plot, and identifying them can be somewhat subjective.
  • 🔍 Influential points are those that have a strong effect on the graph of the regression line, altering its slope and intercept significantly.
  • 📈 To determine if a point is influential, one should compare the regression line with and without the point in question on the same scatter plot.
  • 🛠 The use of tools like Excel can facilitate the creation of scatter plots and regression lines to identify outliers and influential points.
  • 🍫 An example given in the script involves chocolate consumption and Nobel laureate rates, where adding a point can dramatically change the regression line.
  • 📊 The point (50, 0) is identified as both an influential point and an outlier due to its distance from other data points and its effect on the regression line.
  • 🤔 The distinction between an outlier and an influential point can sometimes be unclear, requiring visual inspection and comparison of regression lines.
  • 📉 The script emphasizes that the identification of outliers is subjective and may vary between individuals viewing the same data set.
  • 📚 The class does not delve into specific rules for identifying outliers, instead focusing on the conceptual understanding of what constitutes an outlier.
  • 🔑 The importance of visual representation is highlighted, as it can clearly show the impact of an influential point on the regression line.
  • 🔜 The script concludes with a teaser for the next lesson, which will discuss the least squares property and how it measures the 'best fit' of a regression line.
Q & A

  • What is the main focus of the video script?

    -The video script focuses on explaining the concepts of outliers and influential points in the context of scatter plots and regression lines.

  • How is an outlier defined in the context of a scatter plot?

    -An outlier is defined as a point that lies far away from all the other data points in a scatter plot, making it visually distinct from the rest of the data.

  • What is the subjectivity involved in identifying an outlier?

    -Identifying an outlier can be somewhat subjective, as different people might have different perceptions of what 'far away' means in terms of data points' distance from each other.

  • What is an influential point in the context of regression analysis?

    -An influential point is a data point that strongly affects the graph of the regression line, causing significant changes in the line's slope or position when included or excluded from the analysis.

  • How can you determine if a point is an influential point?

    -To determine if a point is influential, one should graph the regression line on a scatter plot both with and without the point in question, and observe if there are dramatic changes in the regression line.

  • What tool is suggested for creating scatter plots and graphing regression lines?

    -The script suggests using a tool like Excel, which allows for quick creation of scatter plots and graphing of regression lines.

  • What is the example given in the script to illustrate an influential point?

    -The example given is the 23 pairs of chocolate consumption and Nobel laureate rate data, where adding a point (50, 0) dramatically changes the regression line, indicating it is an influential point.

  • Why is the point (50, 0) considered both an influential point and an outlier in the example?

    -The point (50, 0) is considered both an influential point and an outlier because it significantly changes the regression line when included and is visually far away from all other data points in the scatter plot.

  • What is the least squares property mentioned at the end of the script?

    -The least squares property refers to the method used to determine the best fit line in regression analysis, which minimizes the sum of the squares of the differences between the observed values and the values predicted by the model.

  • What will be the topic of the next video in the series?

    -The next video will discuss the least squares property in more detail, explaining how the best fit line for a set of data is measured and determined.

Outlines
00:00
📊 Understanding Outliers and Influential Points

This paragraph introduces the concept of outliers and influential points in the context of a scatter plot. An outlier is defined as a data point that is significantly distant from the rest of the data, and its identification can be somewhat subjective. Influential points, on the other hand, are those that have a strong impact on the regression line's graph. To determine if a point is influential, one should compare the regression lines with and without the point in question. The example provided involves chocolate consumption and Nobel laureate rates, demonstrating how the inclusion of a particular point can dramatically alter the regression line, thus identifying it as both an outlier and an influential point.

05:02
📈 Confirming Influential Points with Regression Analysis

The second paragraph delves deeper into identifying influential points by emphasizing the necessity of graphing the regression line on a scatter plot both with and without the suspect point. It suggests using tools like Excel for this purpose. The paragraph concludes by reiterating the importance of the least squares property, which will be discussed in a subsequent video, as a method to measure the 'best fit' of the regression line to the data.

Mindmap
Keywords
💡Outliers
Outliers are data points that are significantly different from other observations in a dataset. In the context of the video, an outlier is described as a point that lies far away from all other data points on a scatter plot, making it visually distinct. The video uses the example of a point with a chocolate consumption of 50 kilograms per capita and zero Nobel laureates to illustrate an outlier, which stands out due to its extreme value compared to the rest of the data.
💡Influential Points
Influential points are data points that have a strong effect on the outcome of statistical analyses, such as the slope and position of a regression line. The video emphasizes that determining if a point is influential requires plotting the regression line with and without the point in question. If the line changes significantly, the point is considered influential, as demonstrated with the addition of the point (50, 0), which dramatically alters the regression line in the provided example.
💡Scatter Plot
A scatter plot is a graphical representation of data where individual data points are plotted on a Cartesian plane using their values for two variables. The video script discusses the importance of scatter plots in identifying outliers and influential points by visually comparing the regression line with and without certain points, as seen in the example of chocolate consumption and Nobel laureate rates.
💡Regression Line
The regression line, also known as the line of best fit, is a straight line that attempts to summarize the relationship between two variables in a scatter plot. The video explains that this line can be influenced by outliers and influential points, and the script provides a clear example of how the inclusion of a particular point can change the regression line's equation and graph.
💡Paired Sample Data
Paired sample data refers to data that is organized in pairs where each pair is related or matched in some way. In the video, the concept is mentioned in the context of identifying influential points within such data. The script does not provide a specific example but implies that paired sample data might be more prone to containing influential points that affect the regression analysis.
💡Subjective
The term 'subjective' in the video refers to the somewhat personal judgment involved in identifying outliers on a scatter plot. While there are statistical methods to define outliers, the video suggests that determining whether a point is an outlier can also be based on a viewer's perception of how far a point lies from the rest of the data, as exemplified by the varying interpretations of the point at (50, 0).
💡Excel
Excel is a widely used spreadsheet program that can create scatter plots and graph regression lines, which is mentioned in the video as a tool to help determine influential points. The script suggests that using Excel can facilitate the process of plotting the regression line with and without a specific point to see if it changes the line significantly.
💡Least Squares Property
The least squares property, which will be discussed in a subsequent video according to the script, is a method used to determine the best fit line in regression analysis. It is related to the concept of outliers and influential points because it measures how well the regression line fits the data, which can be affected by these types of points.
💡Nobel Laureate Rate
The Nobel laureate rate is a metric mentioned in the video that refers to the number of Nobel laureates per a certain population size, such as 10 million. It is used in the context of the example to illustrate the concept of outliers and influential points in the dataset of chocolate consumption versus Nobel laureate rates.
💡Chocolate Consumption
Chocolate consumption is used in the video as one variable in a dataset that is plotted against the Nobel laureate rate. The script uses this variable to create a scatter plot and to demonstrate the concepts of outliers and influential points, particularly with the example of a country with high chocolate consumption and zero Nobel laureates.
Highlights

The video discusses learning outcome number four for lesson 10.2, focusing on outliers and influential points.

The goal is to define outliers and influential points and determine their presence in a scatter plot.

An outlier is defined as a point that lies far away from other data points in a scatter plot.

Identifying an outlier can be subjective, depending on individual perception of what is 'far away'.

Influential points are those that significantly affect the regression line graph.

To determine if a point is influential, compare regression lines with and without the point.

Dramatic changes in the regression line indicate an influential point.

Excel can be used to easily create scatter plots and graph regression lines for analysis.

An example using chocolate consumption and Nobel laureate rate data illustrates the concept.

The inclusion of a point (50, 0) dramatically changes the regression line, indicating it is influential.

The point (50, 0) is also an outlier due to its distance from other data points.

The subjective nature of identifying outliers is discussed, with some points being more clearly outliers than others.

The video emphasizes the importance of graphing regression lines with and without a point to determine its influence.

The next video will discuss the least squares property and what it means for a line to be the best fit.

The least squares property will be the focus of learning outcome number five.

The video concludes with a preview of the next topic, leaving the audience curious about the least squares property.

Transcripts

Browse More Related Video

Statistics 101: Linear Regression, Outliers and Influential Observations

The Effects of Outliers and Extrapolation on Regression (2.4)

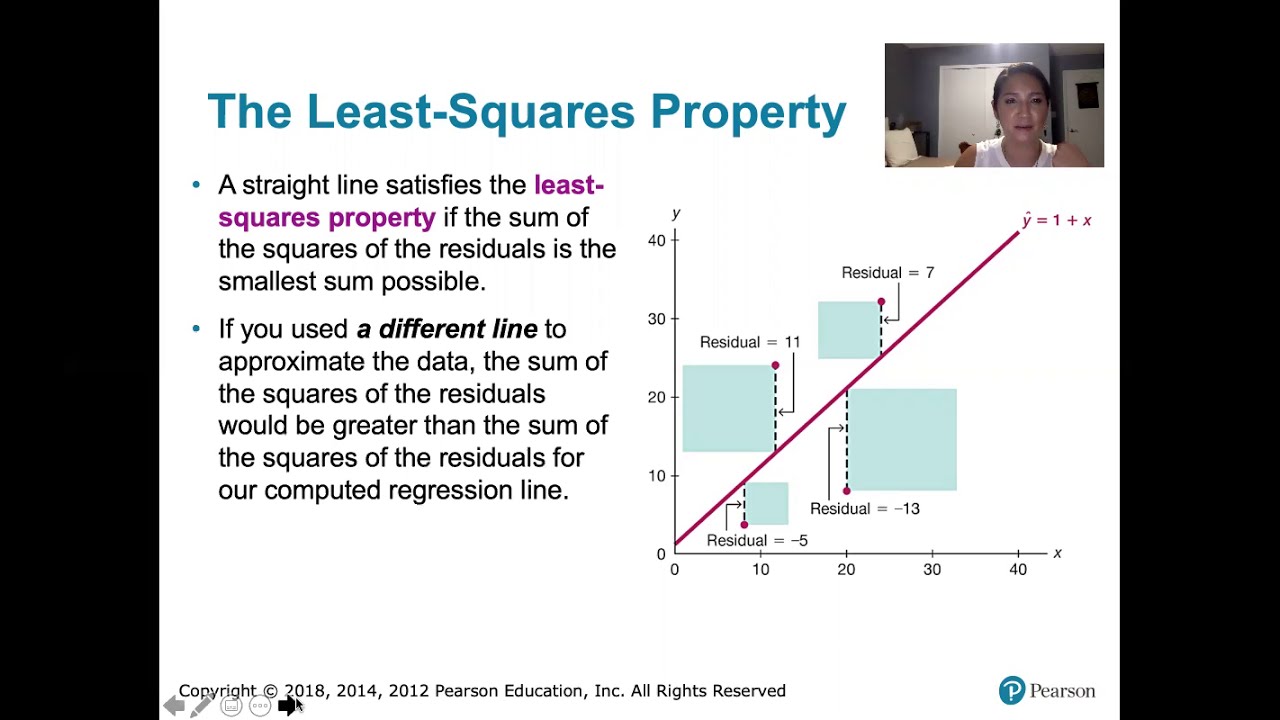
10.2.5 Regression - Residuals and the Least-Squares Property

10.2.0 Regression - Lesson Overview, Key Concepts, and Learning Outcomes

Introduction to residuals and least squares regression

Math 119 Chapter 10 part 2

Rate This

5.0 / 5 (0 votes)

Thanks for rating:

Related Tags
OutliersInfluential PointsData AnalysisRegression LineScatter PlotExcel TipsStatistical LearningChocolate DataNobel LaureatesLeast Squares