AP Calculus BC Lesson 9.7

Elizabeth Fein
10 Apr 202327:25
EducationalLearning
32 Likes 10 Comments

TLDRThis video lesson delves into the concept of polar coordinates and polar derivatives, contrasting them with rectangular coordinates. It explains how polar coordinates are represented by R and Theta, with R being the radius and Theta the angle from the x-axis. The video provides formulas for converting between polar and rectangular coordinates and demonstrates how to plot polar coordinates. It also covers the creation of polar curves and their derivatives, including examples of finding the rate of change of r with respect to Theta and the slope of the tangent line to a polar curve. The lesson concludes with a method for calculating the second derivative of a polar curve.

Takeaways
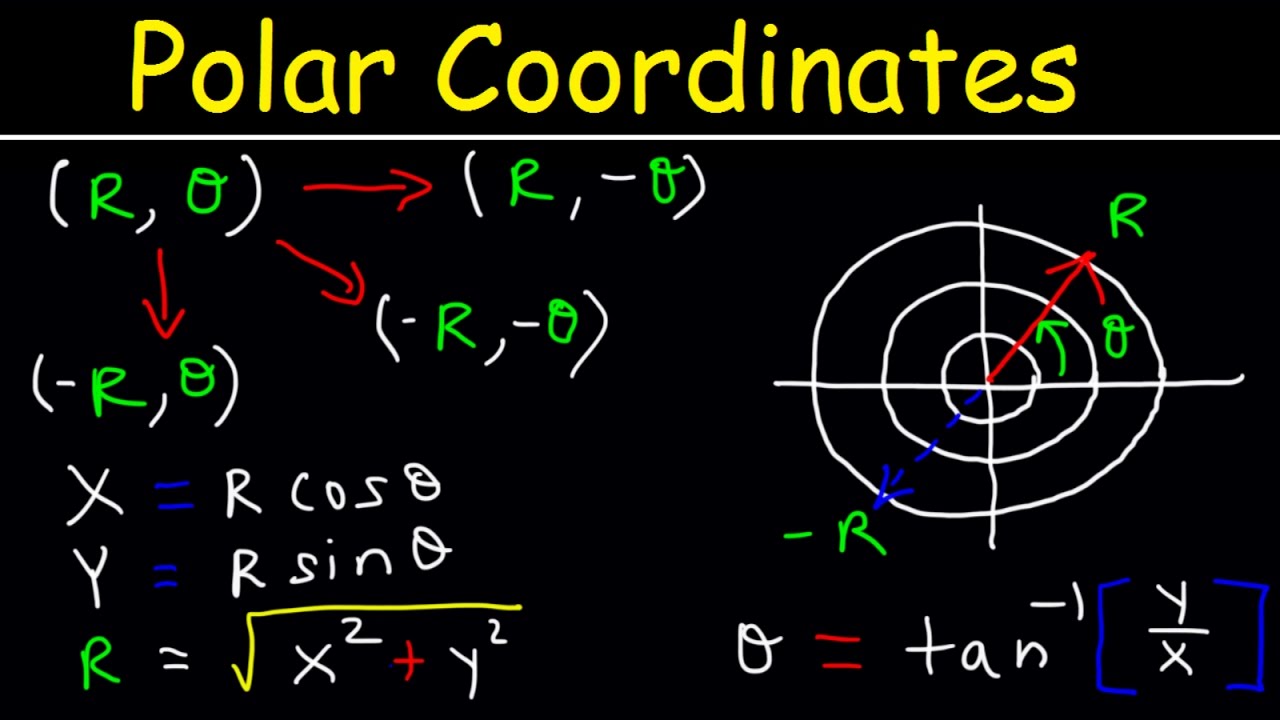
  • πŸ“ Polar coordinates are used to describe points in a circular grid format (R, Theta), with R being the radius from the center (pole) and Theta the angle from the x-axis.
  • πŸ”„ Rectangular (Cartesian) and polar coordinates can be converted using trigonometric relationships: X = R * cos(Theta) and Y = R * sin(Theta).
  • πŸ“ˆ Polar curves are equations in terms of R and Theta, allowing the plotting of shapes and patterns based on radius and angle.
  • πŸ€” Negative radius values in polar coordinates result in reflected points across the pole, maintaining the same angle but in the opposite direction.
  • πŸ“Š Graphing polar equations can be done on a calculator by switching to polar mode and inputting the equation in terms of R and Theta.
  • πŸ‘οΈ The rate of change of polar variables can be found using derivatives, with attention to whether the rate is sought for r with respect to Theta, or X and Y with respect to each other.
  • 🧭 The slope of the tangent line to a polar curve is found using the ratio dy/dx, which in polar terms translates to dy/dTheta over dx/dTheta.
  • 🌟 Polar derivatives can be complex, often requiring the use of product and chain rules, as well as careful attention to the specific variables and their relationships.
  • πŸ”’ The second derivative of a polar curve can be calculated using the formula dΒ²y/dxΒ², which involves finding the derivative of the first derivative with respect to Theta and then evaluating at a specific point.
  • πŸ“š Memorizing the conversion formulas and the relationships between polar and rectangular coordinates is crucial for solving problems involving polar coordinates and their derivatives.
Q & A

  • What are polar coordinates and how are they different from rectangular coordinates?

    -Polar coordinates are a system of expressing a position on a plane by specifying the distance from a reference point called the pole, and the angle from a reference direction, usually an axis. Unlike rectangular coordinates (Cartesian coordinates) which use x and y values to represent a point, polar coordinates use a radius (R) and an angle (Theta).

  • How can you convert a point from rectangular coordinates to polar coordinates?

    -To convert a rectangular coordinate (x, y) to polar coordinates, you calculate the radius (R) as the hypotenuse of the right triangle formed by the x and y distances from the origin, using the Pythagorean theorem (R = sqrt(x^2 + y^2)). The angle (Theta) is found using the arctangent function (Theta = atan(y/x)), taking into account the quadrant of the point to determine the correct angle.

  • What are the formulas to convert from polar coordinates to rectangular coordinates?

    -The conversion formulas from polar coordinates (R, Theta) to rectangular coordinates (x, y) are: x = R * cos(Theta) and y = R * sin(Theta).

  • How do you plot a point in polar coordinates?

    -To plot a point in polar coordinates, you start from the pole (origin) and move along the radius (R) units in the direction of the angle (Theta) from the reference axis (usually the x-axis).

  • What is a polar curve and how is it represented?

    -A polar curve is a curve defined in polar coordinates by an equation that relates R and Theta. It is represented by an equation of the form R = f(Theta), and plotting this equation for various Theta values results in the polar curve.

  • How can you find the rate of change of r with respect to Theta in a polar equation?

    -To find the rate of change of r with respect to Theta (dr/dTheta), you differentiate the polar equation R = f(Theta) with respect to Theta. This gives you the derivative dr/dTheta, which represents the rate of change of the radius with respect to the angle.

  • How do you convert a polar coordinate point into rectangular coordinates using the conversion formulas?

    -You use the formulas x = R * cos(Theta) and y = R * sin(Theta), substituting the values of R and Theta from the polar coordinate point into these formulas to find the corresponding x and y values in rectangular coordinates.

  • How do you graph polar equations on a graphing calculator?

    -To graph polar equations on a graphing calculator, you switch the calculator to polar mode using the mode button, then enter the polar equation using the appropriate function and parametric settings. The calculator will display the graph of the polar curve.

  • What is the relationship between the slope of a tangent line in polar coordinates and the derivatives dy/dx and dx/dy?

    -In polar coordinates, the slope of a tangent line is given by the ratio dy/dx = (dy/dTheta) / (dx/dTheta). This incorporates the derivatives of the polar coordinates with respect to Theta, accounting for the relationship between the changes in angle and radius.

  • How do you find the second derivative of a polar curve with respect to X?

    -To find the second derivative of a polar curve with respect to X, you use the formula d^2y/dx^2 = d(dy/dx)/dTheta, where you first find the first derivative dy/dx using the polar to rectangular conversion formulas, and then differentiate that result with respect to Theta to get the second derivative.

  • What is the instantaneous rate of change of a polar curve with respect to Theta at a specific point?

    -The instantaneous rate of change of a polar curve with respect to Theta at a specific point is found by evaluating the derivative of the polar equation with respect to Theta (dr/dTheta or dy/dTheta) at the given Theta value. This provides the rate of change at that particular point on the curve.

Outlines
00:00
πŸ“š Introduction to Polar Coordinates

This paragraph introduces the concept of polar coordinates, contrasting it with the more familiar rectangular (Cartesian) coordinates. It explains that polar coordinates are given in the format (R, Theta), where R is the radius from the origin (pole) and Theta is the angle from the x-axis. The paragraph also discusses how to convert between polar and rectangular coordinates using trigonometric relationships, specifically X = R * cos(Theta) and Y = R * sin(Theta). The importance of memorizing these formulas for the video's content is emphasized.

05:00
πŸ“ Plotting Polar Coordinates and Converting to Rectangular Form

The paragraph delves into the process of plotting polar coordinates and converting them into rectangular form. It provides examples of plotting specific polar coordinates, such as (2, pi/6) and dealing with negative radii. The conversion process is further explained with the formulas for X and Y in terms of R and Theta. The concept of polar curves is introduced, and examples of simple polar equations like R = 3 cos(2Theta) are given. The paragraph also explains how to graph polar equations using a graphing calculator and the importance of switching back to function mode when finished.

10:01
πŸ”„ Derivatives of Polar Equations

This section focuses on finding derivatives of polar equations, emphasizing the need for careful attention to the variables involved. It explains how to calculate the rate of change of r with respect to Theta, using the derivative of the given polar equation. The paragraph provides examples of finding the rate of change of X and Y with respect to Theta, using the product and chain rules. It also introduces the concept of the slope of the tangent line in polar coordinates, explaining how to calculate it using dy/dx in terms of dY/dTheta and dX/dTheta.

15:02
πŸ“ˆ Calculating Tangent Line Slopes and Second Derivatives

The paragraph discusses the calculation of the slope of the tangent line to a polar curve, using the expression dy/dx rewritten as dY/dTheta over dX/dTheta. It provides an example of finding the slope at a specific value of Theta for a given polar curve. The process of finding the second derivative of a polar curve is also explained, using the formula d^2y/dx^2. Examples are given to illustrate the calculation of the second derivative at a specific Theta value, emphasizing the use of the quotient rule and simplification of trigonometric expressions.

Mindmap
Keywords
πŸ’‘Polar Coordinates
Polar coordinates are a two-dimensional coordinate system in which each point is determined by a distance from a reference point called the pole, and an angle from a reference direction. In the video, polar coordinates are introduced as an alternative to rectangular coordinates, with the format (R, Theta) where R is the radius and Theta is the angle from the x-axis.
πŸ’‘Rectangular Coordinates
Rectangular coordinates, also known as Cartesian coordinates, are a two-dimensional coordinate system where each point is specified by its x (horizontal) and y (vertical) distances from the origin. This system is contrasted with polar coordinates in the video, where the point (3, 2) would be located three units to the right and two units up from the origin.
πŸ’‘Conversion Formulas
Conversion formulas are mathematical expressions that allow the transformation of coordinates from one system to another. In the context of the video, conversion formulas are used to switch between polar and rectangular coordinates, with the key formulas being x = R * cos(Theta) and y = R * sin(Theta).
πŸ’‘Polar Derivatives
Polar derivatives are mathematical operations that find the rate of change of a function with respect to its variables in polar coordinates. The video discusses how to find derivatives such as the rate of change of r with respect to Theta (Dr/dTheta) and how to apply these concepts to polar equations.
πŸ’‘Polar Curves
Polar curves are shapes or figures that are described by an equation in polar coordinates. These curves can represent various mathematical functions and are plotted on a polar grid. The video explains how to plot and analyze polar curves, such as those defined by equations like R = 3cos(2Theta).
πŸ’‘Graphing Calculator
A graphing calculator is an electronic device used to perform mathematical calculations and plot graphs, including those in polar coordinates. The video provides instructions on how to switch a graphing calculator to polar mode and use it to visualize polar equations.
πŸ’‘Rate of Change
The rate of change is a mathematical term that describes how quickly a quantity changes with respect to another quantity. In the video, this concept is applied to find the derivative of polar equations, such as the rate of change of r with respect to Theta or the rate of change of X and Y with respect to Theta.
πŸ’‘Tangent Line
A tangent line is a line that touches a curve at a single point without crossing it. The slope of the tangent line at a particular point on a curve can be found by taking the derivative of the curve's equation at that point. In the context of polar coordinates, the slope is often expressed as dy/dx, but in the video, it's adapted to polar functions using dy/dTheta over dx/dTheta.
πŸ’‘Chain Rule
The chain rule is a fundamental principle in calculus used to find the derivative of a composite function. It states that the derivative of a function composed of other functions is the derivative of the outer function multiplied by the derivative of the inner function. In the video, the chain rule is applied when differentiating complex polar equations involving multiple functions.
πŸ’‘Quotient Rule
The quotient rule is a calculus formula used to find the derivative of a quotient of two functions. It states that the derivative of a function divided by another function is the derivative of the numerator multiplied by the denominator minus the numerator multiplied by the derivative of the denominator, all divided by the denominator squared. The video uses the quotient rule to find the second derivative of a polar curve.
Highlights

Introduction to polar coordinates as an alternative to rectangular coordinates.

Polar coordinates are written in the format R,Θ where R is the radius and Θ is the angle from the x-axis.

Conversion between rectangular and polar coordinates using trigonometric relationships: X = R * cos(Θ) and Y = R * sin(Θ).

Plotting polar coordinates by extending the radius and rotating the specified angle from the x-axis.

Negative radius in polar coordinates results in reflection across the origin.

Identical points can have different polar representations, such as positive and negative radii leading to the same location.

Polar curves are equations in terms of R and Θ, allowing for the visualization of shapes and patterns.

Conversion from polar to rectangular coordinates using the formulas X = R * cos(Θ) and Y = R * sin(Θ).

Graphing polar equations on a calculator by switching to polar mode and inputting the polar equation.

Derivatives of polar equations, such as the rate of change of r with respect to Θ.

Using the product rule and chain rule to find derivatives of polar equations involving R and Θ.

Calculating the slope of the tangent line to a polar curve using dy/dx in terms of dY/dΘ over dX/dΘ.

Example of finding the slope of a tangent line to a polar curve with R = 5Θ² at Θ = Ο€/2.

Instantaneous rate of change of r with respect to Θ for a given polar curve and specific Θ value.

Second derivative of a polar curve using the formula dΒ²y/dxΒ² in terms of polar coordinates.

Example calculation of the second derivative of y with respect to X at Θ = Ο€/3 for a given polar curve.

Explanation of how to handle polar equations in various calculus problems, including derivatives and graphing.

Practical application of polar coordinates in understanding and solving calculus problems.

The importance of memorizing conversion formulas between polar and rectangular coordinates for problem-solving.

Transcripts

Browse More Related Video

Polar Coordinates Basic Introduction, Conversion to Rectangular, How to Plot Points, Negative R Valu

Calculus 2: Polar Coordinates (Video #30) | Math with Professor V

Lesson 14 - Polar Equations (Calculus 2 Tutor)

Polar Curves

AP Calculus BC Lesson 9.8

Lec 33 | MIT 18.01 Single Variable Calculus, Fall 2007

Rate This

5.0 / 5 (0 votes)

Thanks for rating:

Related Tags
Polar CoordinatesCalculus ConceptsCoordinate ConversionDerivativesTrigonometryGraphing TechniquesMathematics EducationPolar CurvesPolar EquationsCalculus Tutorial