Simple Harmonic Motion - IB Physics
TLDRThis lecture delves into simple harmonic motion, a periodic motion around an equilibrium point where net force is zero. It highlights objects like springs and pendulums, emphasizing the restoring force's linear relationship with displacement. The lecture explains Hooke's Law for springs, the linear restoring force in pendulums, and introduces amplitude, period, and frequency. It also covers the sine wave pattern in position-time graphs, and the relationship between velocity, acceleration, and displacement, illustrating the conservation of energy in kinetic and potential forms.
Takeaways
- 📚 Simple Harmonic Motion (SHM) is a periodic motion around an equilibrium point with no net force, characterized by a restoring force proportional to the displacement from the equilibrium position.
- 🔍 Common examples of SHM include springs and pendulums, both having an equilibrium position where the net force is zero.
- 📉 Hooke's Law states that the spring force (Fs) is linearly proportional to the displacement (x) from the equilibrium position, expressed as Fs = -kx, where k is the spring constant.
- 🌐 The restoring force in SHM is always opposite in direction to the displacement, aiming to bring the object back to the equilibrium position.
- 📏 The amplitude of SHM is the maximum displacement from the equilibrium point, not the total distance traveled by the object.
- ⏱ The period of SHM is the time taken for one complete cycle of motion, including both extremes and the return to the starting point.
- 📈 The position-time graph of an object in SHM forms a sine wave, where the amplitude can be read from the graph and the period is the time for one complete wave cycle.
- 🚀 The velocity of an object in SHM is highest at the equilibrium position and zero at the extremes, due to the restoring force's influence.
- 🔽 The acceleration in SHM is highest at the extremes (where the velocity is zero) and zero at the equilibrium position, as acceleration is the result of the restoring force.
- 🔄 The conservation of energy in SHM is evident in the constant sum of kinetic and potential energy, with kinetic energy being highest at the equilibrium and potential energy highest at the extremes.
- 📊 The force graph in SHM resembles the acceleration graph, scaled by the mass of the object, and energy graphs show the interplay between kinetic and potential energy.
Q & A
What is the definition of simple harmonic motion?
-Simple harmonic motion is the periodic motion around a central point of equilibrium where there is no net force. It involves a restoring force that acts in the opposite direction and is proportional to the displacement from the equilibrium position.
What are the two most common objects that exhibit simple harmonic motion?
-The two most common objects that exhibit simple harmonic motion are the spring and the pendulum.
What is an equilibrium position in the context of simple harmonic motion?
-An equilibrium position is a point in simple harmonic motion where there is no net force acting on the object, such as the natural length of a spring or the vertical position of a pendulum.
How does Hooke's Law relate to simple harmonic motion?
-Hooke's Law describes the restoring force in a spring, stating that the force (Fs) is equal to the spring constant (k) times the displacement (x), and it acts in the opposite direction of the displacement, which is a key characteristic of simple harmonic motion.
What is amplitude in simple harmonic motion?
-Amplitude is the maximum displacement from the equilibrium position that an object in simple harmonic motion achieves.
How is the period of simple harmonic motion defined?
-The period of simple harmonic motion is the time it takes for an object to complete one full cycle, going from one extreme to the other and back to its starting point.
What is the relationship between period and frequency in simple harmonic motion?
-The period is the inverse of the frequency. If the period is the time for one full cycle, the frequency is the number of cycles completed in one second, measured in Hertz (Hz).
What does the position-time graph of an object in simple harmonic motion typically look like?
-The position-time graph of an object in simple harmonic motion typically forms a sine wave, showing the displacement from the equilibrium position over time.
How does the velocity of an object in simple harmonic motion change as it moves through its equilibrium position and towards the extrema?
-The velocity of an object in simple harmonic motion is highest at the equilibrium position and decreases to zero at the extrema. It changes from positive to negative as the object moves past the equilibrium position.
What is the relationship between the acceleration and the displacement in simple harmonic motion?
-The acceleration in simple harmonic motion is directly proportional to the displacement and points in the opposite direction. It is zero at the equilibrium position and maximum at the extrema.
How does the conservation of energy principle apply to simple harmonic motion?
-In simple harmonic motion, the total energy (kinetic plus potential) remains constant due to the conservation of energy principle. The object exchanges kinetic energy for potential energy and vice versa as it moves, but the sum of both remains the same.
Outlines
🔄 Introduction to Simple Harmonic Motion
The script begins with an introduction to simple harmonic motion (SHM), defining it as a periodic motion around an equilibrium point where the net force is zero. The restoring force is proportional and opposite to the displacement from this point. Common examples of SHM are springs and pendulums, both having an equilibrium position with no net force. The lecture aims to break down the complex definition and discusses the role of Hooke's Law in relation to springs, emphasizing the linear relationship between the restoring force and displacement.
📏 Understanding Amplitude and Period in SHM
This paragraph delves into the concept of amplitude, which is the maximum displacement from the equilibrium position in SHM. It clarifies a common misconception about amplitude, explaining that it is not the total path length but the distance from the equilibrium to one extreme. The paragraph also introduces the period and frequency of SHM, defining the period as the time for one complete cycle and frequency as the number of cycles per second. The importance of measuring the full period, including both extremes, is highlighted to avoid common errors in calculation.
📈 Graphing SHM: Position, Velocity, and Acceleration
The script explains how to represent SHM graphically, starting with position-time graphs that form sine waves. It discusses how to derive velocity-time and acceleration-time graphs from the displacement graph, noting that the velocity is highest at the equilibrium point and zero at the extrema. Conversely, acceleration is highest at the extrema and zero at the equilibrium point. The explanation includes the relationship between the slopes of these graphs and the physical quantities they represent, emphasizing the counterintuitive nature of SHM where maximum velocity coincides with zero acceleration.
🚀 Energy Conservation in SHM
The final paragraph discusses energy in the context of SHM, focusing on the scalar nature of energy and its conservation. It describes how kinetic and potential energies vary with position and velocity, respectively, and how they sum to a constant total energy, illustrating this with graphs. The script emphasizes that in SHM, as kinetic energy increases (object moves faster), potential energy decreases, and vice versa, adhering to the principle that energy cannot be created or destroyed within a closed system.
Mindmap
Keywords
💡Simple Harmonic Motion
💡Equilibrium Position
💡Restoring Force
💡Hooke's Law
💡Amplitude
💡Period
💡Frequency
💡Position-Time Graph
💡Velocity-Time Graph
💡Acceleration-Time Graph
💡Energy Graph
Highlights
Simple harmonic motion is defined as periodic motion around a central point of equilibrium with no net force.
Restoring force in SHM acts opposite to displacement and is proportional to it.
Springs and pendulums are common examples of objects undergoing simple harmonic motion.
Equilibrium position in SHM is where the net force on the object is zero.
Hooke's Law describes the linear relationship between spring force and displacement.
The spring force is proportional to displacement and acts in the opposite direction.
Pendulum's restoring force is the component of gravity acting towards the equilibrium position.
Amplitude in SHM is the maximum displacement from the equilibrium position.
The period and frequency of SHM are related by the equation period = 1/frequency.
The full period of SHM includes motion to both extremes and back to the starting point.
Position-time graphs of SHM form a sine wave, indicating the displacement over time.
Velocity in SHM is highest at the equilibrium position and zero at the extrema.
Acceleration in SHM is zero at the equilibrium position and maximum at the extrema.
The force graph in SHM resembles the acceleration graph, scaled by the mass.
Energy graphs in SHM show kinetic and potential energy, which are conserved and sum to a constant.
In SHM, the total energy of an object remains constant due to energy conservation.
Potential energy in SHM can be elastic for springs or gravitational for pendulums.
Transcripts
Browse More Related Video
2022 Live Review 6 | AP Physics 1 | Understanding Simple Harmonic Motion
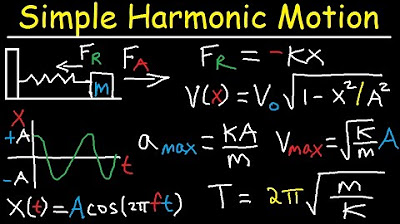
Simple Harmonic Motion, Mass Spring System - Amplitude, Frequency, Velocity - Physics Problems

8.01x - Lect 10 - Hooke's Law, Springs, Pendulums, Simple Harmonic Motion

AP Physics 1 - Simple Harmonic Motion
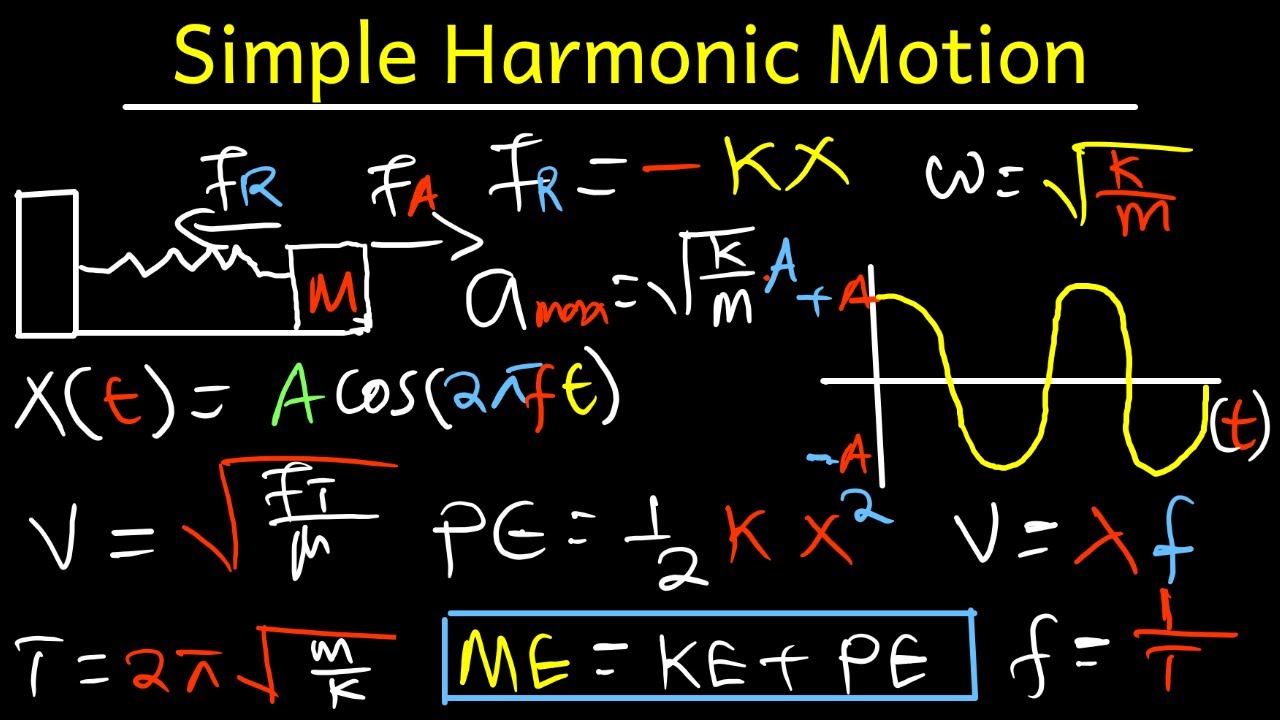
Simple Harmonic Motion, Mass Spring System - Physics Full Topic

Introduction to Simple Harmonic Motion (SHM) | General Equation and Derivation
5.0 / 5 (0 votes)
Thanks for rating: