Vectors - Higher and Foundation GCSE Maths
TLDRIn this educational video, Matt's Kitchen explores the concept of vectors in geometry, using an analogy of train journeys between villages to explain the basics of vector operations. The video demonstrates how to find vectors between points and introduces the concept of midpoints in vector terms. It guides viewers through examples, encouraging practice and understanding of vector addition, subtraction, and the representation of midpoints as vectors. The content is aimed at helping learners grasp the fundamental principles of vector geometry, with a promise of further videos on more complex topics.
Takeaways
- 📚 The video introduces the concept of vectors in geometry, explaining how to represent them with arrows and letters.
- 🚂 The script uses an analogy of villages and train lines to help understand the direction and representation of vectors.
- 🔍 Vectors are described as journeys, with the direction indicated by an arrow, and the opposite direction represented by a negative vector.
- 📝 The video explains how to find the vector from point A to B by combining the vectors from A to O and O to B, using the formula -A + B.
- 📈 The script provides a method to find the midpoint of a line segment by dividing the vector into two equal parts, represented as half of the vector.
- 📐 The midpoint of a vector is used to find the vector from the origin to a point on the line, which is half the vector from the origin to the endpoint.
- 🔢 The video offers a step-by-step approach to solving vector problems, including simplifying expressions and combining vectors.
- 📉 The script emphasizes the importance of practice in understanding the concepts of vectors and midpoints.
- 🎓 The channel offers full-length videos and tips for learning geometry, with new content released on a regular schedule.
- 💻 The video encourages viewers to engage with the content by liking, subscribing, and exploring other videos on the channel.
- 🔑 The takeaways from the script stress the need for understanding basic principles and applying them to more complex problems involving ratios and midpoints.
Q & A
What does the notation with a capital letter and an arrow above it represent in vector geometry?
-It represents the vector from point A to point B, indicating the direction and magnitude from one point to another.
How do you denote a vector when writing by hand?
-When writing by hand, instead of using a capital letter with an arrow, the letter is typically underlined to denote a vector.
What is the analogy used in the script to help understand vectors?
-The analogy compares the vectors to train journeys between three villages (A, B, and O), where the vectors represent the direction and distance of the train routes.
What is the vector from O to A called, and what does the negative of this vector represent?
-The vector from O to A is called vector A. The negative of this vector, negative A, represents the journey in the opposite direction, from A to O.
How can you describe the vector from A to B if there is no direct train line?
-You can describe the vector from A to B as the combination of going from A to O (negative A) and then from O to B (vector B), which can be written as negative A plus B.
What is the midpoint of a line segment in vector terms?
-The midpoint of a line segment is a point that is exactly halfway along the line, and in vector terms, it can be represented as half of the vector representing the entire line segment.
If M is the midpoint of OB, how would you describe the vector from O to M?
-If M is the midpoint of OB, the vector from O to M would be described as half of vector B, which can be written as -1/2 B.
How can you find the vector from A to the midpoint P of AB?
-To find the vector from A to the midpoint P of AB, you first find the vector AB (negative A plus B) and then take half of it, resulting in negative 1/2 A plus 1/2 B.
What is the vector OP if P is the midpoint of AB?
-The vector OP can be found by going from O to A (vector A) and then from A to P (negative half A plus half B), which simplifies to half A plus half B.
What is the process for finding the vector SM if S is the midpoint of AB?
-To find the vector SM, you take half of the vector representing the entire line segment AB, which has been previously calculated as negative A plus B, resulting in negative 1/2 B plus 1/2 A.
How can you describe the vector PM if P is the midpoint of AB and M is another point on the line?
-The vector PM can be described by adding the vector from A to P (negative half A plus half B) to the vector from A to B (negative A plus B), simplifying to half B plus half A.
Outlines
📚 Introduction to Vectors in Geometry
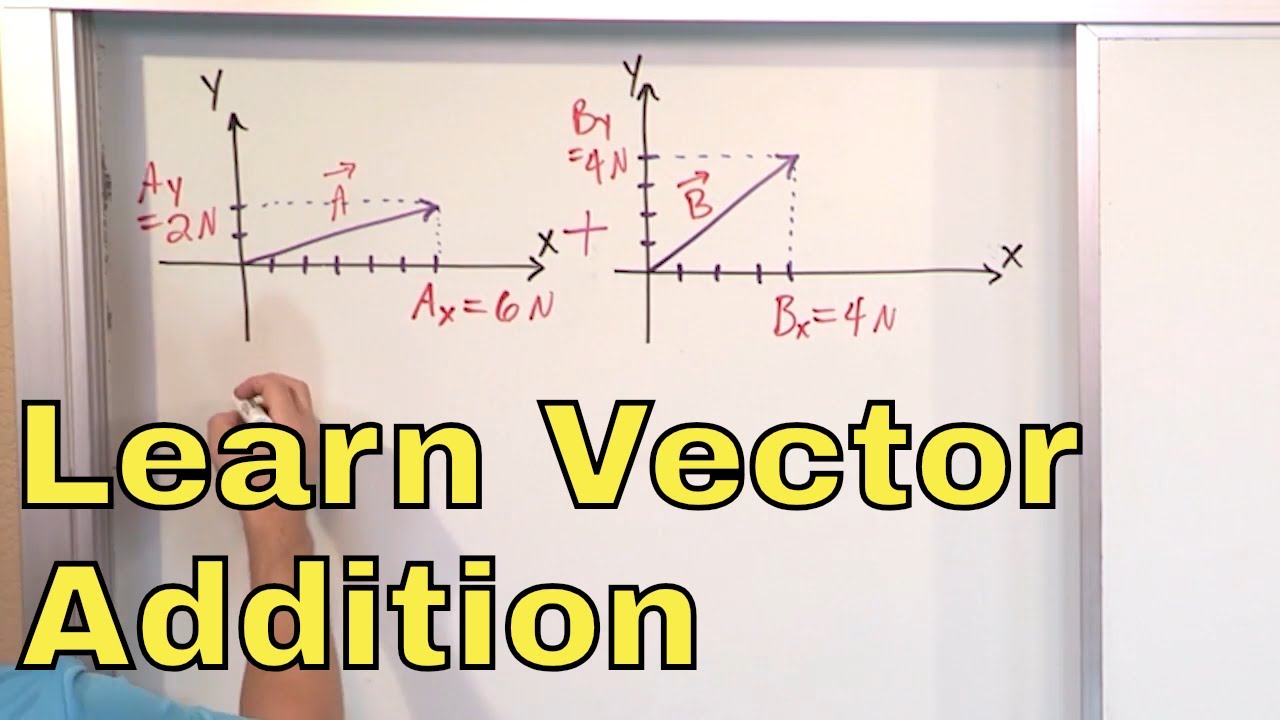
The first paragraph introduces the concept of vectors in geometry, using an analogy of villages and train lines to explain the idea of direction and magnitude in vector representation. It clarifies the notation for vectors, such as the arrow indicating the direction from point A to B, and the use of bold and underlined letters to denote vectors when written by hand. The paragraph also outlines the process of finding a vector from A to B by combining the vectors from A to O and O to B, simplifying the concept of vector addition and subtraction to describe the journey from one point to another.
🔍 Calculating Midpoints and Vectors
This paragraph delves into the application of vectors to find midpoints and to describe the vectors associated with them. It explains how to determine the vector from the midpoint of one point to another, using the previously established concept of vector addition and subtraction. The paragraph provides a step-by-step guide on how to find the vector AP, which is half of AB, and how to express OP in terms of A and P, simplifying the process to half of A plus half of B. It also includes a practice question for the viewer to solve, encouraging active learning and reinforcing the concepts discussed.
📈 Advanced Vector Problems and Practice
The final paragraph builds upon the concepts introduced earlier, tackling more complex vector problems that involve ratios and midpoints. It presents a continuation of the practice question from the previous paragraph, guiding the viewer through the process of finding vectors SM and PM. The explanation includes simplifying the vector expressions and combining them to find the final result. The paragraph concludes with an encouragement to practice and a teaser for upcoming videos that will cover additional topics in vector geometry, such as ratios and new style questions.
Mindmap
Keywords
💡Vector
💡Geometry
💡Exam Question
💡Midpoint
💡Negative Vector
💡Journey
💡Direction
💡Magnitude
💡Practice
💡Ratio
Highlights
Introduction to the concept of vectors in geometry, using an analogy of villages and train lines to explain the direction and representation of vectors.
Explanation of how to denote vectors by underlining or using bold letters when writing by hand, due to the difficulty of drawing arrows.
The use of the term 'vector' to describe the journey or direction from one point to another, with the vector's direction indicated by an arrow.
Understanding the concept of negative vectors, where the direction is reversed, represented by making the letter negative.
Illustration of how to find the vector from A to B by combining the vectors from A to O and O to B, using the concept of existing train lines.
Introduction of a second example to practice finding vectors in terms of given vectors a and b.
Explanation of the midpoint concept in geometry, relating it to the halfway point along a line segment.
How to describe the vector for the midpoint of a line segment using half of the original vector.
Demonstration of calculating the vector for a midpoint by halving the vector representing the entire line segment.
The process of finding the vector from O to P by combining the vectors from O to A and A to P.
Simplification of vector expressions by combining like terms and understanding the resulting vector.
Practice question presented for viewers to apply the concepts of vectors and midpoints.
Detailed walkthrough of solving the practice question, reinforcing the understanding of vector operations.
Discussion on the importance of practice in grasping the concepts of vectors and midpoints in geometry.
Introduction of upcoming video parts that will cover ratios and new styles of exam questions involving vectors.
Encouragement for viewers to keep practicing and to look forward to the next video for further exploration of vector concepts.
Transcripts
5.0 / 5 (0 votes)
Thanks for rating: