GCSE Physics - Moments worked examples

LovattPhysics

12 Apr 202009:02

EducationalLearning

32 Likes 10 Comments

TLDRThis instructional video offers practice moments for IGCSE physics students, focusing on balancing forces and calculating missing forces. It presents five problems where viewers must determine the unknown force, using the principle of moments around a pivot. The video guides through each problem, explaining how to equate anti-clockwise and clockwise moments to find the solution. It also emphasizes the importance of using perpendicular distances to the force for calculations. Additional exam-style questions and mark schemes are provided in the video description for further practice.

Takeaways

📚 The video is designed to help practice moments questions for IGCSE physics.
🔍 Each question presents a scenario with forces and distances, requiring the viewer to find the missing force 'F'.
⏸ Viewers are encouraged to pause the video to solve the problem themselves before the solution is shown.
🔧 The principle of balancing moments (anti-clockwise and clockwise) is central to solving the problems.
📐 The formula to find the unknown force 'F' involves equating the moments on both sides of the pivot.
📉 In the first question, the missing force 'F' is calculated by multiplying the given force by its distance from the pivot.
📈 For the second question, the unknown force 'F' is found by dividing the product of the given force and its distance by the distance of 'F' from the pivot.
🔄 The third question involves multiple forces and requires summing the moments of both clockwise and anti-clockwise forces to find 'F'.
📝 The fourth question introduces the concept of considering the direction of moments as either clockwise or anti-clockwise to find 'F'.
📐 The fifth question emphasizes the importance of using the perpendicular distance from the pivot when calculating moments.
📉 The solution for the fifth question involves dividing the sum of the moments by the perpendicular distance to find 'F'.
👍 Additional exam-style questions and mark schemes are provided in the video description for further practice.

Q & A

What is the purpose of the video?
-The video is designed to help students practice moments questions for IGCSE physics, specifically to find the missing force F in various balance scenarios.
How many forces are involved in the first example provided in the video?
-In the first example, there are two forces involved: a 20 Newton force on the left and an unknown force F on the right.
What is the formula used to find the missing force F in the first example?
-The formula used is F = (20 N * 0.6 m), which results in F being 12 Newtons.
In the second example, what force is given and what force needs to be found?
-In the second example, a force F is given at 0.2 meters from the pivot, and a 30 Newton force is 1.8 meters away. The missing force F needs to be calculated.
What is the calculated force F for the second example in the video?
-The calculated force F for the second example is 270 Newtons.
In the third example, how many forces are there and what is the method to find the missing force F?
-In the third example, there are four forces. The method to find the missing force F involves balancing the clockwise and anti-clockwise moments around the pivot.
What is the value of the missing force F in the third example?
-The value of the missing force F in the third example is 250 Newtons.
In the fourth example, how many forces are there and what is the approach to solving for the missing force F?
-In the fourth example, there are four forces. The approach involves identifying the direction of the moments (clockwise or anti-clockwise) and equating the total moments to solve for F.
What is the calculated force F for the fourth example in the video?
-The calculated force F for the fourth example is approximately 207 Newtons, rounded to three significant figures.
What is the key concept in the fifth example that differentiates it from the previous ones?
-The key concept in the fifth example is the consideration of the perpendicular distance from the pivot when calculating the moments.
What is the calculated force F for the fifth example in the video?
-The calculated force F for the fifth example is 167 Newtons, rounded to three significant figures.
Where can viewers find additional practice questions and mark schemes related to the video content?
-Viewers can find additional practice questions and mark schemes in the description of the video.

Outlines

00:00

📚 IGCSE Physics Practice: Moment of Force Calculations

This paragraph introduces a video aimed at helping students practice moment of force questions for IGCSE physics. The presenter explains the concept of balancing moments around a pivot point to solve for unknown forces. The first example involves a 20 Newton force and another force at a distance, requiring the viewer to calculate the missing force 'F'. The solution demonstrates the principle of balancing anti-clockwise and clockwise moments, resulting in a calculation that finds 'F' to be 12 Newtons.

05:00

🔍 Advanced Moment Calculation with Multiple Forces

The second paragraph continues the theme of moment calculations but introduces a more complex scenario with multiple forces and distances. The video script guides viewers through balancing the clockwise and anti-clockwise moments to find an unknown force 'F'. The example given involves forces of 30 Newtons and 60 Newtons at different distances from the pivot. The solution process involves dividing the product of the force and its distance by the unknown force's distance, yielding 'F' as 270 Newtons.

📐 Solving Complex Force Balances with Geometry

This paragraph presents a more intricate problem with additional forces and emphasizes the importance of understanding distances relative to the pivot point, not between forces. The solution involves calculating both clockwise and anti-clockwise moments with multiple forces, including a 120 Newton force at 10 meters and a 60 Newton force at 5 meters. The calculation leads to finding 'F' as 250 Newtons by equating the sum of moments and solving for the unknown.

📉 Directional Moments and Force Calculation

The fourth paragraph introduces the concept of directional moments, where the direction of the force affects whether it is considered a clockwise or anti-clockwise moment. The video script provides a detailed example with four forces and their respective distances from the pivot. The solution involves identifying the direction of each force's moment and calculating the unknown force 'F' by balancing the total moments, resulting in 'F' being approximately 207 Newtons.

📐 Perpendicular Distance in Moment Calculations

The final paragraph focuses on the importance of using the perpendicular distance from the pivot when calculating moments. The example provided involves forces with vertical directions and requires attention to the perpendicular distance for accurate moment calculation. The solution process involves dividing the product of the known force and its perpendicular distance by the unknown force's perpendicular distance, resulting in 'F' being approximately 167 Newtons.

Mindmap

Keywords

💡IGCSE

IGCSE stands for International General Certificate of Secondary Education, which is an international curriculum and examination system for 14-16 year olds. In the video, it is the qualification for which the physics practice questions are intended, indicating the educational level and the target audience.

💡Force

In physics, force is any interaction that, when unopposed, will change the motion of an object. The video script revolves around calculating unknown forces in various mechanical balance scenarios, emphasizing the concept's importance in the study of physics.

💡Moment

Moment, in the context of physics, often refers to the turning effect of a force about a particular point, known as the moment of force. The script uses the concept of moments to solve for unknown forces in lever balance problems, illustrating the principle of moments in action.

💡Pivot

A pivot is a fixed point around which a lever rotates. In the video, the pivot is the reference point from which distances are measured to calculate moments, essential for determining the balance of forces on a lever.

💡Balanced

A system is balanced when the net force and the net moment about any point are zero. The script repeatedly mentions the condition of balance to establish the equations needed to solve for the unknown forces in the physics problems.

💡Anti-clockwise

Anti-clockwise refers to the direction opposite to the movement of the hands of a clock. In the script, it is used to describe the direction of moments that would cause a clockwise rotation of the lever if not balanced by an equal anti-clockwise moment.

💡Clockwise

Clockwise is the direction of the movement of the hands of a clock. The script contrasts clockwise and anti-clockwise moments to explain how they must be equal and opposite for a lever to be in equilibrium.

💡Distance

In the context of the video, distance refers to the length from the pivot to the point where the force is applied. It is a critical factor in calculating moments, as the moment is the product of the force and the perpendicular distance to the pivot.

💡Perpendicular

Perpendicular refers to a direction at a right angle to a given line or surface. The script emphasizes the importance of using the perpendicular distance to the pivot when calculating moments, as this is the effective distance that contributes to the turning effect of the force.

💡Newton

A Newton is the SI unit of force. The script uses Newtons to quantify the magnitude of forces in the physics problems, which is necessary for calculating moments and solving for unknown forces.

💡Significant Figures

Significant figures are the digits in a number that carry meaning contributing to its precision. The script mentions rounding answers to three significant figures, which is a common practice in scientific reporting to ensure precision and clarity.

Highlights

Introduction of a video aimed at practicing moments questions for IGCSE physics.

Methodology of solving moment problems by finding the missing force F.

Explanation of balancing anti-clockwise and clockwise moments for a beam.

Solution to question 1: Calculating force F using the formula F = 20 * 0.6 Newtons.

Question 2 involves a force F at 0.2 meters and a 30 Newton force at 1.8 meters.

Solution to question 2: Determining force F as 270 Newtons using moment balance.

Question 3 introduces multiple forces and distances from the pivot.

Solution to question 3: Finding F as 250 Newtons by equating moments.

Question 4 adds complexity with four forces and varying distances.

Solution to question 4: Calculating F as 207 Newtons with careful consideration of distances and directions.

Question 5 emphasizes the importance of perpendicular distances from the pivot.

Solution to question 5: Determining force F as 167 Newtons using perpendicular distances.

The video includes a step-by-step approach to solving moment problems.

Each question provides an opportunity to pause and solve before the solution is shown.

The video concludes with additional exam-style questions and mark schemes in the description.

Encouragement for viewers to practice and good luck wishes for their studies.

Transcripts

Browse More Related Video

Moments, Torque, Toppling & Couples - GCSE & A-level Physics (full version)

Turning Forces (Moments)

Moments (part 2) | Moments, torque, and angular momentum | Physics | Khan Academy

The Principle of Moments - A Level Physics

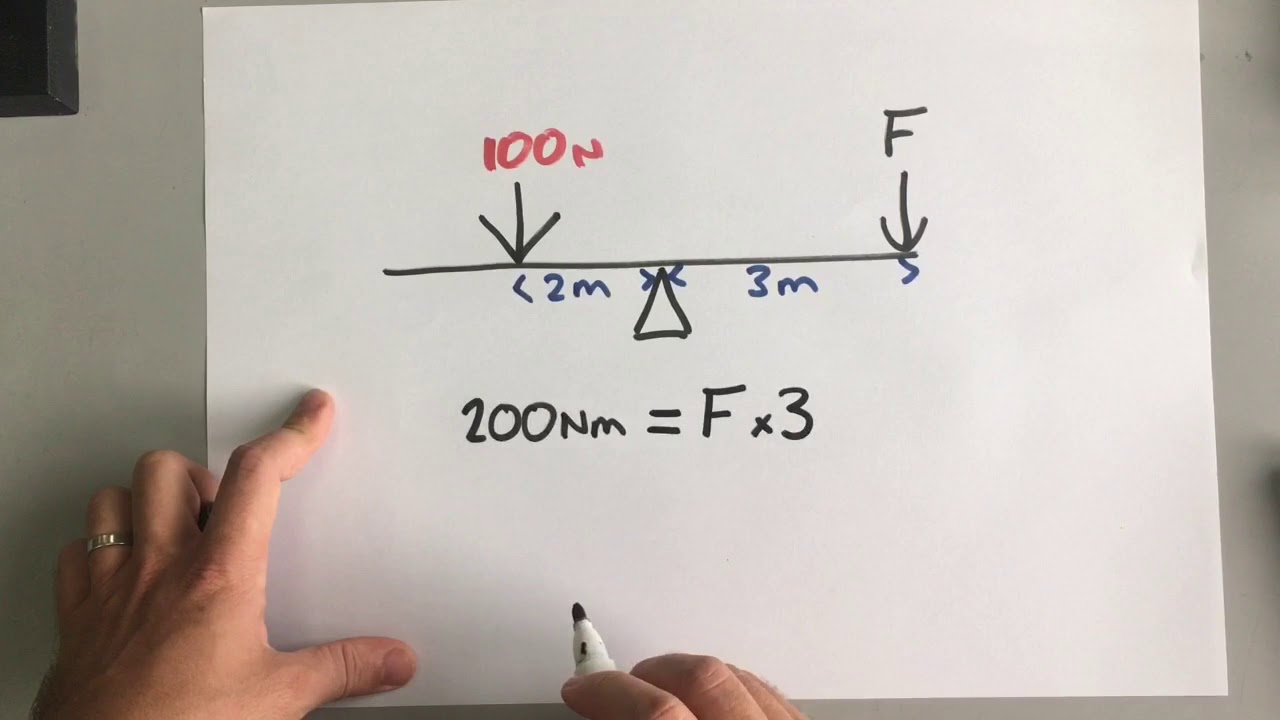
GCSE Physics - Forces 5 - Moments and Centre of Mass

Principle of Moments - Physics Revision

GCSE Physics - Moments worked examples

Takeaways

Q & A

What is the purpose of the video?

How many forces are involved in the first example provided in the video?

What is the formula used to find the missing force F in the first example?

In the second example, what force is given and what force needs to be found?

What is the calculated force F for the second example in the video?

In the third example, how many forces are there and what is the method to find the missing force F?

What is the value of the missing force F in the third example?

In the fourth example, how many forces are there and what is the approach to solving for the missing force F?

What is the calculated force F for the fourth example in the video?

What is the key concept in the fifth example that differentiates it from the previous ones?

What is the calculated force F for the fifth example in the video?

Where can viewers find additional practice questions and mark schemes related to the video content?