Calculus AB/BC – 1.7 Selecting Procedures for Determining Limits

The Algebros
13 Jun 202011:11
EducationalLearning
32 Likes 10 Comments

TLDRIn this calculus lesson, Mr. Bean introduces students to selecting procedures for finding limits, including algebraic manipulation, direct substitution, and handling indeterminate forms. He focuses on rationalizing fractions with radicals and solving complex fractions by finding common denominators. The lesson demonstrates step-by-step solutions, emphasizing the importance of precision in algebraic steps and selecting the appropriate method for each problem. Mr. Bean encourages students to practice and master these techniques for effective limit calculations.

Takeaways
  • 📚 The lesson is focused on selecting procedures in calculus to find limits, including algebraic manipulation, factoring, and canceling.
  • 🔍 Direct substitution is the first method tried for limits, but it may result in indeterminate forms like zero over zero.
  • 📐 The concept of rationalizing is introduced to deal with limits involving fractions with radicals, using the conjugate of the numerator or denominator.
  • 🔢 The process of rationalizing involves multiplying by the conjugate, which can simplify expressions using the difference of squares formula.
  • 📉 In the example given, multiplying by the conjugate simplifies the limit expression, allowing for direct substitution to find the limit as X approaches 5, resulting in 1/6.
  • 📌 The script emphasizes the importance of recognizing and correctly applying the conjugate in rationalizing expressions with radicals.
  • 🤔 The lesson also covers complex fractions, where the goal is to find a common denominator to simplify the expression before taking the limit.
  • 📝 For complex fractions, the process involves multiplying both the numerator and the denominator by the appropriate expressions to achieve a common denominator.
  • ✅ After simplifying complex fractions, the next step is to multiply by the reciprocal of the denominator to simplify the expression further.
  • 📉 The script provides examples of complex fractions where after simplification, direct substitution can be used to find the limit as X approaches a certain value.
  • 💡 The importance of precision in algebraic manipulation and careful selection of the correct procedure for each problem is highlighted throughout the lesson.
Q & A

  • What is the main topic of Mr. Bean's calculus lesson?

    -The main topic of the lesson is selecting procedures to find limits, including algebraic manipulation, rationalizing fractions with radicals, and dealing with complex fractions.

  • What is an indeterminate form in calculus?

    -An indeterminate form in calculus occurs when a limit results in an expression like 0/0 or ∞/∞, which does not provide a clear value and requires special techniques to resolve.

  • What is the conjugate in the context of rationalizing fractions with radicals?

    -The conjugate refers to a binomial where if you have a term like a - b, the conjugate would be a + b. Multiplying a binomial by its conjugate results in a difference of squares, which simplifies the expression.

  • How does Mr. Bean suggest dealing with limits that result in an indeterminate form of 0/0?

    -Mr. Bean suggests multiplying the numerator and the denominator by the conjugate of the expression in the denominator to eliminate the radicals and simplify the limit.

  • What is the process for rationalizing a fraction with a radical in the denominator?

    -The process involves multiplying both the numerator and the denominator by the conjugate of the radical in the denominator, which simplifies the expression and allows for direct substitution.

  • What is the result of the first example limit calculation in the script?

    -The result of the first example limit calculation is 1/6, after rationalizing the fraction and substituting the value of X as it approaches 5.

  • How does Mr. Bean approach teaching complex fractions?

    -Mr. Bean suggests finding a common denominator for all terms in the complex fraction, simplifying the expression, and then using the reciprocal to simplify further before applying direct substitution.

  • What is the result of the second example limit calculation with a radical in the denominator?

    -The result of the second example is -6, after rationalizing the fraction and following the steps to simplify the expression.

  • What is the strategy for dealing with complex fractions in Mr. Bean's lesson?

    -The strategy involves finding a common denominator for all fractions within the complex fraction, multiplying numerators and denominators accordingly, and then simplifying the expression before applying direct substitution.

  • What is the result of the first complex fraction limit calculation in the script?

    -The result of the first complex fraction limit calculation is -16, after simplifying the expression and substituting the value of X as it approaches 0.

  • Why is it important to factor out terms when dealing with complex fractions in limits?

    -Factoring out terms can simplify the expression and reveal common factors that can be canceled out, making it easier to apply direct substitution and find the limit.

Outlines
00:00
📚 Introduction to Calculus Limit Procedures

In this calculus lesson, Mr. Bean introduces the concept of selecting procedures to find limits, which includes algebraic manipulation techniques such as factoring and direct substitution. The lesson then delves into two advanced topics: rationalizing fractions with radicals and handling complex fractions. Mr. Bean emphasizes the importance of recognizing patterns and provides a step-by-step guide on how to deal with indeterminate forms, such as zero over zero, by multiplying by the conjugate in the case of rationalizing. The summary also covers the process of simplifying complex fractions by finding a common denominator and using reciprocals to simplify the expression before direct substitution can be applied to find the limit.

05:01
🔍 Detailed Steps for Rationalizing and Simplifying Complex Fractions

This paragraph focuses on the detailed algebraic steps required to rationalize expressions with radicals and to simplify complex fractions. Mr. Bean demonstrates the process of multiplying by the conjugate to eliminate radicals, using the difference of squares formula, and simplifying the resulting expression. He also explains how to handle complex fractions by finding a common denominator and then simplifying the expression by multiplying by the reciprocal of the denominator. The summary includes specific examples with step-by-step calculations, illustrating the process of direct substitution after simplification to find the limit of the given expressions as X approaches certain values.

10:04
🎓 Mastering Calculus Limit Techniques

The final paragraph wraps up the lesson by emphasizing the importance of precision and careful work when dealing with calculus limit problems. Mr. Bean encourages students to practice and master the techniques of direct substitution, factoring, and the use of conjugates for rationalizing expressions. He also advises students to select the most appropriate procedure for each problem and to take their time to ensure accuracy. The summary highlights the importance of reviewing algebraic steps and the application of these techniques in solving calculus limit problems, preparing students for further practice and mastery of the subject.

Mindmap
Keywords
💡Calculus
Calculus is a branch of mathematics that deals with rates of change and accumulations. In the video, calculus is the overarching subject, with the focus on 'selecting procedures' to find limits, which is a fundamental concept in understanding the behavior of functions and their outputs as inputs approach certain values.
💡Selecting Procedures
Selecting procedures refer to the various algebraic techniques used to manipulate expressions to find limits. The script mentions that these include factoring, canceling, and direct substitution. These procedures are essential for simplifying expressions before applying the limit process.
💡Rationalizing
Rationalizing is the process of eliminating radicals (square roots) from the denominator of a fraction. In the script, the teacher explains how to rationalize by multiplying the numerator and denominator by the conjugate of the denominator, which is a technique used to simplify expressions containing radicals.
💡Conjugate
The conjugate of a binomial is formed by changing the sign between the two terms. In the context of the video, the conjugate is used to rationalize expressions, as seen when the teacher multiplies by 'a minus B times a plus B' to eliminate the square root in the denominator.
💡Difference of Squares
The difference of squares is a mathematical identity that states that the difference between the squares of two numbers is equal to the product of the sum and difference of the two numbers (a^2 - b^2 = (a + b)(a - b)). In the script, this concept is used to simplify expressions after rationalizing.
💡Indeterminate Form
An indeterminate form occurs when an expression evaluates to an undefined or ambiguous result, such as 0/0. In the script, the teacher mentions that direct substitution results in an indeterminate form, which is a cue to use a different method to find the limit.
💡Complex Fractions
Complex fractions are fractions where the numerator, the denominator, or both contain fractions themselves. The script discusses how to handle complex fractions by finding a common denominator and simplifying, which is a key technique in calculus for evaluating limits.
💡Greatest Common Factor (GCF)
The greatest common factor is the largest number that divides two or more numbers without leaving a remainder. In the context of the video, the GCF is mentioned as a method to simplify complex fractions, although the teacher opts for a different approach to avoid confusion.
💡Direct Substitution
Direct substitution involves plugging the value that the variable approaches into the function to find the limit. The script emphasizes that direct substitution is faster when applicable, but it may not always be possible, especially when it results in an indeterminate form.
💡Limit
A limit is the value that a function approaches as the input approaches a certain value. The entire lesson is centered around finding limits using various algebraic techniques, illustrating the importance of limits in understanding the behavior of functions in calculus.
Highlights

Introduction to selecting procedures in calculus for finding limits.

Explanation of algebraic manipulation for limits, including factoring and canceling.

Introduction of two additional methods: rationalizing fractions with radicals and dealing with complex fractions.

Direct substitution leading to an indeterminate form of zero over zero.

Use of conjugates to rationalize expressions involving square roots in the denominator.

Demonstration of multiplying by the conjugate to eliminate radicals in a limit problem.

Simplification of expressions using the difference of squares.

Step-by-step walkthrough of a limit problem involving rationalization.

Final answer of 1/6 for the first limit problem after rationalization.

Invitation for students to try a similar problem involving a radical in the denominator.

Solution of a limit problem with a radical in the denominator, resulting in an answer of negative six.

Emphasis on the importance of correct algebraic steps and precision in solving calculus problems.

Introduction to solving complex fractions by finding a common denominator.

Method of multiplying numerator and denominator by the greatest common factor to simplify complex fractions.

Detailed example of solving a complex fraction limit problem, leading to the answer of negative 16.

Explanation of how to simplify complex fractions by multiplying by the reciprocal.

Final problem walkthrough involving complex fractions, resulting in an answer of negative 2/3.

Advice on selecting the best procedure for each limit problem based on the given expression.

Encouragement to practice and master the techniques for solving calculus limit problems.

Transcripts

Browse More Related Video

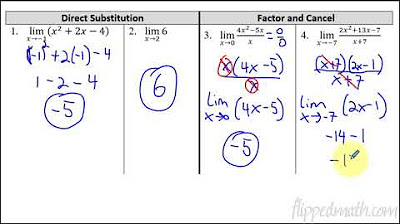
Calculus AB/BC – 1.6 Determining Limits Using Algebraic Manipulation

AP Calculus AB - 1.7 Selecting Procedures for Determining Limits

Calculus AB/BC – 6.10 Integrating Functions Using Long Division and Completing the Square

AP Calculus AB - 1.6b Determining Limits Using Algebraic Manipulation

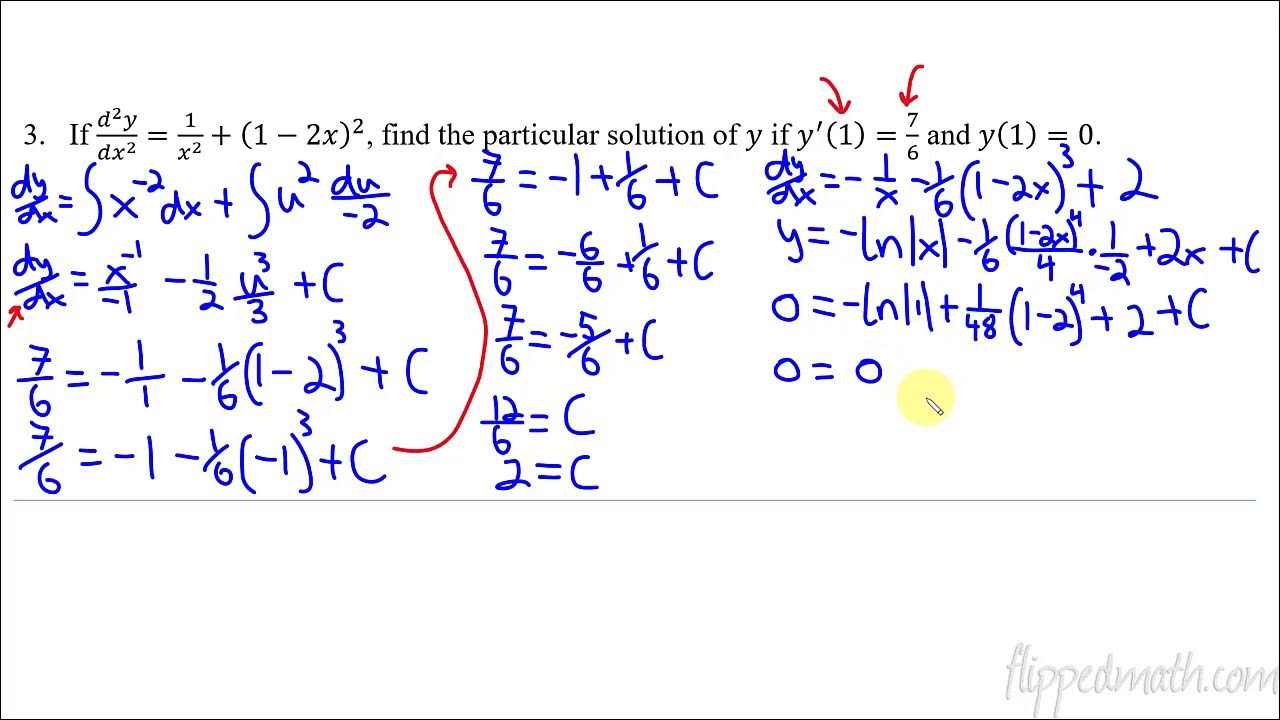
Calculus AB/BC – 7.2 Verifying Solutions for Differential Equations

Calculus AB/BC – 1.8 Determining Limits Using the Squeeze Theorem

Rate This

5.0 / 5 (0 votes)

Thanks for rating:

Related Tags
Calculus LessonsLimitsRationalizingComplex FractionsAlgebraic ManipulationDirect SubstitutionConjugate MultiplicationEducational ContentMath TutorialMr. BeanMathematics Education