Limits 01 | Introduction | CLASS 11 | JEE | PACE SERIES

Physics Wallah - Alakh Pandey

21 Feb 202156:36

EducationalLearning

32 Likes 10 Comments

TLDRThe transcript appears to be a lecture on calculus, focusing on the concept of limits. The speaker uses an informal, conversational tone and relatable analogies, such as 'neighbors' to explain complex mathematical ideas. They emphasize the importance of understanding limits in functions and their applications, suggesting that grasping this concept is crucial for a strong foundation in calculus. The lecture also touches on the different aspects of limits, including geometric and algebraic perspectives, and the influence of infinite values on limit calculations.

Takeaways

📚 The script is an educational lecture, focusing on the concept of limits in calculus, emphasizing the importance of understanding limits to grasp the concepts of calculus effectively.
🔍 It uses the analogy of 'neighbors' to explain the concept of limits, suggesting that the limit is like the 'neighbor' of a function value as you approach a certain point.
🗣️ The lecturer shares personal teaching experiences, noting that many students have misconceptions or lack of information about limits, indicating the need for clear explanations.
📈 The script discusses the geometric aspect of limits, explaining how limits are represented graphically and the significance of the right and left limits, especially in the context of a function's behavior at a point.
📚 It highlights the algebraic aspect of limits, discussing the mathematical expressions and operations involved in calculating limits, such as approaching infinity or a specific value.
👉 The importance of function concepts is stressed, as a good understanding of functions is prerequisite to understanding limits within calculus.
🤔 The script poses thought-provoking questions to the audience about the behavior of functions and their limits, encouraging active engagement and critical thinking.
📉 The concept of infinity is explored, with discussions on how it behaves in different mathematical contexts and its impact on the evaluation of limits.
📘 The lecture touches on the different forms of limits, such as infinite limits and limits at a point, and how these forms are used to solve problems in calculus.
👨‍🏫 The speaker's enthusiasm for teaching calculus is evident, with a motivational tone aimed at inspiring students to embrace the challenge of learning calculus and its complex concepts.
🎓 The script concludes with a reminder of the importance of practice and a promise to delve deeper into the topic in subsequent sessions, indicating a series of lectures or lessons.

Q & A

What is the main topic of the video script?
-The main topic of the video script is the concept of limits in calculus, with a focus on the mathematical approach and the geometric interpretation of limits.
What is the significance of 'neighbours' in the context of this script?
-In the context of this script, 'neighbours' refers to the values around a particular point on a graph, which are important in understanding the behavior of functions and their limits.
What does the instructor emphasize about learning limits for the first time?
-The instructor emphasizes that students learning limits for the first time should learn very carefully and attentively, as a strong grasp of limits will lead to a strong foundation in calculus concepts.
What is the relationship between functions and limits as discussed in the script?
-The relationship between functions and limits discussed in the script is that limits are used to understand the behavior of functions as the input approaches a certain value, which can be crucial for determining the function's value at a point or its continuity.
What is the concept of 'infinitely close' mentioned in the script?
-The concept of 'infinitely close' in the script refers to the idea that a variable can get arbitrarily close to a certain value without actually reaching it, which is fundamental in the definition of limits.
How does the script use the term 'infinity' in the context of limits?
-The script uses the term 'infinity' to describe a situation where a variable in an expression tends towards an unbounded or limitless value, which is a key concept in understanding certain types of limits.
What is the importance of understanding the right tips for limits as mentioned by the instructor?
-Understanding the right tips for limits is important because it helps students to grasp the concept more effectively and avoid common mistakes, leading to a better understanding of calculus as a whole.
How does the script differentiate between the right and left neighbors of a point?
-The script differentiates between the right and left neighbors of a point by considering the values on either side of the point. The right neighbor is the value as the input approaches from the right side, while the left neighbor is the value as it approaches from the left side.
What is the role of the function's graph in understanding limits as per the script?
-The role of the function's graph in understanding limits, as per the script, is to visually represent the behavior of the function and its approach to certain values, which helps in the geometric interpretation of limits.
How does the script relate the concept of limits to real numbers and their properties?
-The script relates the concept of limits to real numbers by discussing how functions behave as their input approaches certain real numbers, and how the properties of real numbers, such as infinity, play a role in defining and understanding limits.

Outlines

00:00

📘 Introduction to Limits and Neighbors

The speaker begins by introducing the concept of limits in calculus, drawing an analogy with the relationships between neighbors. The emphasis is on understanding the correct information about limits, which is often misunderstood. A clear understanding of neighbors (adjacent numbers) on a real number line is provided to help grasp the concept of limits.

05:00

🔍 Understanding Right and Left Neighbors

The explanation continues with a detailed analysis of right and left neighbors on a real number line. Using examples, the speaker explains how the values near a given point (e.g., x = 1) are considered its neighbors and how these values help in understanding limits. The importance of knowing both right (positive) and left (negative) neighbors is emphasized.

10:01

📈 Graphical Interpretation of Functions

This section delves into the graphical representation of functions and their values at specific points. The speaker uses a function to illustrate how its value changes at different points, particularly focusing on the right and left neighbors of a given point (e.g., x = a). This graphical understanding is crucial for comprehending limits.

15:02

🧠 Concept of Limits in Calculus

The speaker elaborates on the concept of limits by explaining the notation and meaning behind 'limit as x approaches a'. This involves studying the behavior of a function as it gets closer to a particular point. The speaker stresses the importance of understanding limits for mastering calculus concepts.

20:03

📏 Algebraic Aspects of Limits

The focus shifts to the algebraic aspects of limits, introducing seven indeterminate forms commonly encountered in calculus. These forms (e.g., 0/0, ∞/∞) require specific techniques to solve. The speaker explains that these forms behave differently based on the situation, making them challenging yet essential to understand.

25:05

🧮 Indeterminate Forms in Calculus

The speaker provides a deeper look into indeterminate forms, highlighting their tricky nature. These forms, including 0^∞ and ∞ - ∞, cannot be directly evaluated and depend on the specific context. The concept of 'limiting values' is introduced to explain how these forms are approached in calculus.

30:08

🔬 Detailed Study of Indeterminate Forms

A comprehensive explanation of the seven indeterminate forms continues, with emphasis on their behavior under different conditions. The speaker aims to make students comfortable with these forms by encouraging thorough practice and understanding of their algebraic properties.

35:08

🌊 Understanding Infinity and Its Forms

This section focuses on understanding the forms involving infinity, such as ∞/∞. The speaker uses examples to show how the values of such forms change depending on their context. The goal is to illustrate that these forms are not straightforward and require careful analysis.

40:08

🔄 Evaluating Indeterminate Forms

The process of evaluating indeterminate forms is demonstrated through examples. The speaker explains how different forms can yield different values based on their sources. This highlights the importance of context in determining the value of indeterminate forms.

45:09

🔢 Practical Examples of Indeterminate Forms

Further practical examples are provided to illustrate how indeterminate forms are evaluated. The speaker shows how to apply various algebraic techniques to simplify and solve these forms, reinforcing the concepts discussed earlier.

50:11

📊 Using Limits in Problem Solving

The application of limits in problem-solving is discussed, with examples of how to approach limit problems involving indeterminate forms. The speaker explains the step-by-step process of solving these problems using algebraic manipulations and graphical interpretations.

55:14

📚 Summary and Next Steps

The speaker summarizes the key points covered, including the understanding of limits, neighbors, and indeterminate forms. The importance of mastering these concepts for success in calculus is reiterated. The session ends with encouragement to continue practicing and exploring these topics.

Mindmap

Keywords

💡Limits

Limits are a fundamental concept in calculus, referring to the value that a function or sequence approaches as the input approaches some value. In the script, the speaker discusses limits in the context of understanding the behavior of a function as the input gets arbitrarily close to a certain point, using the term 'approaching' to illustrate this concept.

💡Infinity

Infinity is a concept that represents an unbounded quantity that is larger than any number. The script mentions 'infinity' in various contexts, such as 'infinity by infinity', to describe situations where the values are unbounded or where the function's output grows without limit as the input increases.

💡Neighborhood

The term 'neighborhood' is used in the script to describe the proximity around a certain point when discussing limits. It helps illustrate the idea that as the input value gets closer and closer to a specific point (like zero or infinity), the function's behavior can be predicted by examining its 'neighborhood'.

💡Function

A function is a mathematical relationship between two sets that assigns exactly one output for each input. The script refers to functions when explaining limits, emphasizing the importance of understanding how the function behaves as it approaches certain values.

💡Graph

The script mentions the graph of a function, which is a visual representation of the function's behavior. It is used to illustrate how limits can be visualized on a graph, showing the function's values and their trends as they approach certain points.

💡Right and Left Neighbors

These terms are used to describe the values around a certain point on either side of the number line. In the context of limits, 'right neighbor' and 'left neighbor' help explain how a function behaves as it approaches a point from the right or left, which is crucial for understanding one-sided limits.

💡Zero

Zero is a pivotal value in mathematics and is mentioned in the script in various contexts. For instance, it discusses 'approaching zero' to illustrate how a function's value gets infinitely close to zero, which is a key concept in understanding certain types of limits.

💡Positive and Negative

These terms are used throughout the script to describe the direction or sign of a number or value. In the context of limits, 'positive' and 'negative' help to clarify the behavior of a function or the value of an expression as it approaches certain limits, indicating whether the values are above or below zero.

💡Approximate

The script uses the term 'approximate' to discuss how certain values can be close to but not exactly equal to a particular number. This is relevant when talking about limits, as they often involve values that are getting closer and closer to a specific point without actually reaching it.

💡Algebraic Aspect

The algebraic aspect refers to the mathematical manipulation and symbolic representation of expressions and equations. The script mentions the algebraic aspect of limits, which involves using algebraic techniques to solve for or understand limits, making it a key part of the discussion.

💡Geometric Aspect

The geometric aspect pertains to the visual and spatial understanding of mathematical concepts. In the script, the geometric aspect of limits is discussed in relation to the graph of a function, emphasizing how the visual representation can provide insights into the behavior of the function as it approaches certain limits.

Highlights

Introduction to the concept of limits in calculus, emphasizing the importance of understanding limits for grasping calculus concepts.

The analogy of 'neighbors' to explain the concept of limits, comparing the behavior of functions as they approach a certain point.

Discussion on the right and left 'neighbors' of a function, illustrating how to determine the limit from both sides.

Explanation of the notation and terminology used in limits, such as 'approaching' and 'as x approaches'.

Clarification of common misconceptions about limits and the importance of accurate information in learning.

The significance of the function's graph in understanding limits and how the function behaves around a particular point.

Detailed exploration of the limit's geometric aspect, comparing it to the algebraic approach for a comprehensive understanding.

The impact of infinite values on limits, and how they affect the function's behavior as x approaches certain values.

Introduction to the concept of 'infinite' in mathematics, explaining its properties and its role in calculating limits.

Discussion on the different forms of infinity and their implications in mathematical calculations and limit evaluations.

The practical application of limits in real-world scenarios, emphasizing the relevance of understanding limits beyond theoretical knowledge.

Explanation of the limit calculation process, including the steps to solve limit problems and the rationale behind each step.

The role of practice in mastering the concept of limits, encouraging learners to solve a variety of limit problems.

Highlighting the importance of precision in language when discussing limits to avoid confusion and misinterpretation.

The presentation of limit problems in multiple forms, showcasing the versatility and complexity of limit calculations.

A summary of the key points covered in the session, reinforcing the understanding of limits and their significance in calculus.

Encouragement for learners to continue their studies with enthusiasm and a reminder of the importance of a thorough approach to learning calculus.

Transcripts

Browse More Related Video

Business Mathematics Calculus Midterm Review [2 Hours]

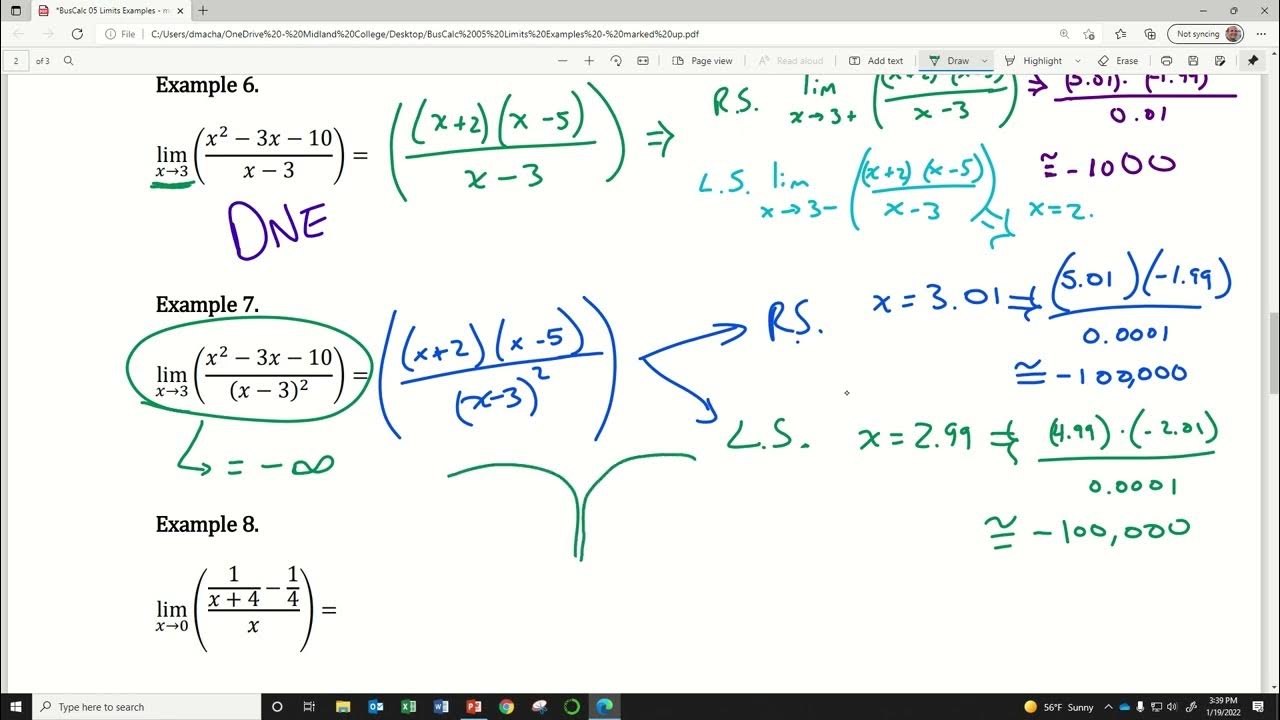
BusCalc 05 Limits Examples Pt 2

Calculus Math 133 - Lecture 2.2

AP Calculus AB - 1.5 Determining Limits Using Algebraic Properties of Limits

1.1 - Numerical and Graphical Limits

Business Calculus - Math 1329 - Section 1.5 (Part 1) - Limits and Continuity

Related Tags

Calculus Limits Math Concepts Geometry Algebra Teaching Mathematics Concept Clarity Educational Learning