Riemann Sums

RH Mathematics

25 Oct 202328:52

EducationalLearning

32 Likes 10 Comments

TLDRThis video delves into the concept of calculating the area under a graph using Riemann sums and introduces the integral notation. It covers two scenarios: areas found through geometry and those requiring calculus. The instructor explains how to approximate areas under curves like exponential functions using left, right, midpoint Riemann sums, and the trapezoidal sum, highlighting their accuracy and application. The video also discusses the properties of definite integrals, such as additivity and the effect of constants, and concludes with practice problems to reinforce the concepts.

Takeaways

📚 The video discusses the concept of area under the graph of a function, introducing the idea of Riemann sums and their use in approximating areas, especially for functions without simple geometric formulas.
📈 The script differentiates between two types of areas: those that can be calculated using geometry (like a line and part of a circle) and those that require calculus (like areas under exponential functions).
🧩 The notation for the area under the graph of a function from \( x = A \) to \( x = B \) is given as \( \int_{A}^{B} f(x) \, dx \), highlighting its connection to anti-derivatives.
📐 The video demonstrates how to find areas using geometric shapes like rectangles, triangles, and trapezoids, and how to approximate areas under curves using Riemann sums.
🔍 The script explains how to use left Riemann sums, right Riemann sums, and midpoint Riemann sums to approximate the area under a curve, each with its own method of choosing representative points for the height of the rectangles.
📊 The video provides examples of approximating integrals using Riemann sums, such as \( \int_{-1}^{1} 4x \, dx \), and discusses the limitations and inaccuracies of these approximations.
📉 The script introduces the concept of the definite integral as the exact value of the area under the graph, which is the limit of Riemann sums as the number of rectangles approaches infinity and their widths approach zero.
🔢 The video discusses the properties of definite integrals, such as the additivity of areas, the negation of areas when integrating in reverse order, and the ability to factor out constants from integrals.
🚀 The script uses the context of an airplane's fuel consumption to illustrate the practical application of integrals, showing how the integral can represent the total fuel burned over a certain time period.
📚 The video concludes with practice problems that reinforce the concepts discussed, including calculating the net signed area under a curve and understanding the properties of definite integrals.

Q & A

What is the main topic discussed in the video?
-The main topic discussed in the video is the area under the graph of a function, specifically using geometry formulas and calculus to find areas under different types of graphs.
What is the notation used for the area under the graph of a function between x = A and x = B?
-The notation used for the area under the graph of a function between x = A and x = B is the integral from A to B of f(x) dx.
Why is calculus needed to find the area under the graph of an exponential function?
-Calculus is needed to find the area under the graph of an exponential function because there is no simple geometry formula for such curved areas, unlike areas formed by lines and parts of circles.
What are the two methods mentioned for finding the area under the graph of a function?
-The two methods mentioned are using geometry formulas for simple shapes like lines and circles, and using calculus, specifically the concept of Riemann sums, for more complex shapes.
What is a Riemann sum and how is it used to approximate the area under a curve?
-A Riemann sum is a method used in calculus to approximate the area under a curve by dividing the area into rectangles and summing the areas of these rectangles. It involves taking the height of the function at certain points (like the left, right, or midpoint of each subinterval) and multiplying it by the width of the subinterval.
What is the formula for a left Riemann sum?
-A left Riemann sum for the area under the graph of a function and above the x-axis is given by the sum of the heights of the function at the left edge of each subinterval multiplied by the width of each subinterval.
What is the formula for a right Riemann sum?
-A right Riemann sum for the area under the graph of a function and above the x-axis is given by the sum of the heights of the function at the right edge of each subinterval multiplied by the width of each subinterval.
What is the formula for a midpoint Riemann sum?
-A midpoint Riemann sum for the area under the graph of a function and above the x-axis is given by the sum of the heights of the function at the midpoint of each subinterval multiplied by the width of each subinterval.
How does the trapezoidal sum differ from other Riemann sums?
-The trapezoidal sum is more accurate than other Riemann sums. It approximates the area by averaging the heights at the ends of each subinterval and multiplying by the width, effectively creating trapezoids instead of rectangles.
What are some properties of definite integrals mentioned in the video?
-Some properties of definite integrals mentioned include: the integral from A to B of f(x) dx plus the integral from B to C of f(x) dx equals the integral from A to C of f(x) dx; the integral from B to A of f(x) dx is the negative of the integral from A to B of f(x) dx; the integral from A to A of f(x) dx is zero; and the integral from A to B of k * f(x) dx can be factored out as k times the integral from A to B of f(x) dx.

Outlines

00:00

📚 Introduction to Area Under a Graph and Riemann Sums

This paragraph introduces the concept of finding the area under a graph of a function. It distinguishes between two types of graphs: those that can be analyzed using geometric formulas (like a line and part of a circle) and those that require calculus (like an exponential function). The notation for the area under a graph from x=A to x=B is introduced as the integral from A to B of f(x) dx. The connection between this notation and anti-derivatives is highlighted. The video will focus on using Riemann sums to approximate areas, starting with simple geometric shapes and moving towards more complex functions.

05:03

📐 Using Geometry to Find Areas and Riemann Sums

The paragraph discusses how to find the area under a graph using geometry for simple shapes and Riemann sums for more complex ones. It provides an example of breaking down a graph into subregions, such as rectangles and triangles, to calculate the area. The concept of a left Riemann sum is introduced, where the left edge of each subinterval is used to approximate the area under the graph. The paragraph also mentions the need for more subintervals for better approximation and the potential inaccuracies in such methods.

10:04

📉 Midpoint and Trapezoidal Riemann Sums

This paragraph explores the midpoint and trapezoidal Riemann sums as methods for approximating the area under a curve. The midpoint Riemann sum is described as using the height at the midpoint of each subinterval, which can be more accurate than left or right sums. The trapezoidal sum is introduced as the most accurate method, involving averaging the heights at the ends of each subinterval and multiplying by the width. The paragraph also discusses the importance of understanding the units of measure in integrals, especially in practical applications like calculating fuel consumption.

15:05

🔍 Understanding the Definite Integral and Riemann Sums

The paragraph delves into the true definition of the definite integral as the limit of Riemann sums as the number of rectangles approaches infinity and their widths approach zero. It emphasizes the importance of understanding this concept, which is foundational in calculus. The paragraph also discusses the properties of definite integrals, such as the additivity of areas, the negation of areas when integrating in reverse order, and the zero integral for a constant function.

20:06

📊 Properties of Definite Integrals and Practice Problems

This paragraph outlines several properties of definite integrals, such as the ability to add areas, the negation of an integral when reversing the limits, and the factorization of constants out of integrals. It also provides practice problems to illustrate these properties, involving calculating the net signed area under a graph and the effect of adding a constant to the function within the integral. The paragraph concludes with a teaser for more practice problems in future videos.

25:06

🔚 Conclusion and Further Practice

The final paragraph wraps up the discussion by summarizing the key points covered in the video and encouraging viewers to practice the concepts learned. It mentions the importance of understanding Riemann sums, the properties of definite integrals, and the practical applications of these concepts. The paragraph also hints at additional practice problems that will be covered in subsequent videos, reinforcing the need for continued learning and application.

Mindmap

Keywords

💡Area Under the Graph

The concept of 'Area Under the Graph' refers to the space enclosed by the curve of a function and the x-axis between two points. It is central to the video's theme as it introduces the idea of calculating this area using different methods, such as geometry formulas or calculus, depending on the shape of the graph. For instance, the script discusses using geometry for a line and part of a circle, and calculus for an exponential function.

💡Riemann Sum

A 'Riemann Sum' is a method used to approximate the definite integral of a function, which is another way of finding the area under the graph. The video explains that for certain functions, like exponential ones, geometry formulas are insufficient, and Riemann Sums, along with calculus, are needed. The script provides examples of left, right, and midpoint Riemann Sums to illustrate this concept.

💡Integral

An 'Integral' is a fundamental concept in calculus representing the exact value of the area under the graph of a function between two points. The video uses integral notation, such as 'integral from A to B of f(x) dx', to denote this area and explains that the integral is connected to the concept of anti-derivatives, which will be explored in subsequent lessons.

💡Geometry Formulas

In the context of the video, 'Geometry Formulas' are used to calculate the area under graphs that have shapes which can be easily broken down into recognizable geometric figures like rectangles, triangles, and trapezoids. The script mentions using these formulas for the graph on the left, which is composed of a line and part of a circle.

💡Exponential Function

An 'Exponential Function' is a mathematical function where the variable is in the exponent. The video discusses the challenge of finding the area under the graph of such a function, as it does not lend itself to simple geometric formulas and requires calculus methods, specifically Riemann Sums, for approximation.

💡Anti-derivative

An 'Anti-derivative' is a concept closely related to integration, where the process of finding an integral is essentially the reverse of differentiation. The video script hints at the connection between anti-derivatives and the area under the graph, which will be a focus of upcoming lessons.

💡Trapezoidal Sum

A 'Trapezoidal Sum' is a specific type of Riemann Sum that is used to approximate the area under the graph of a function when the function values at the endpoints of subintervals are averaged to form trapezoids. The video explains that this method is more accurate than left or right Riemann Sums and provides an example of its application.

💡Midpoint Riemann Sum

A 'Midpoint Riemann Sum' is a less common but still important method of approximating an integral, where the height of the rectangles used for the sum is based on the midpoint of each subinterval. The video mentions this method as potentially testable for AP Calculus and demonstrates its use with an example.

💡Units of Measure

'Units of Measure' in the context of the video refer to the units associated with the quantities being integrated, such as gallons per minute for fuel consumption or liters per hour for water pumped into a tank. The video emphasizes the importance of including units in the interpretation of integrals, especially in applied contexts like an airplane's flight.

💡Definite Integral

A 'Definite Integral' is the exact value of the area under the graph of a function from one point to another on the x-axis. The video provides the formal definition of a definite integral as the limit of Riemann sums as the number of rectangles approaches infinity and their widths approach zero, which is a fundamental concept in calculus.

Highlights

Introduction to the concept of area under the graph of a function and the idea of a Riemann sum.

Explanation of two cases for finding areas under graphs: using geometry formulas for simple shapes and using calculus for curved shapes.

Introduction of integral notation and its connection to anti-derivatives.

Demonstration of finding areas using geometric shapes like rectangles, triangles, and quarter circles.

Discussion on approximating areas under graphs without known formulas using Riemann sums.

Description of the left Riemann sum and its application with examples.

Explanation of the right Riemann sum and its use with more subintervals for better approximation.

Introduction to the midpoint Riemann sum and its rare usage in calculus.

Illustration of the trapezoidal sum as a more accurate approximation method.

Explanation of units of measure for integrals and their significance in practical applications.

Discussion on the meaning of integrals in the context of an airplane's fuel consumption.

Introduction to the true definition of a definite integral as the limit of Riemann sums.

Analysis of whether Riemann sums overestimate or underestimate the true area under a curve.

Properties of definite integrals, including area addition, negation, and constant factoring.

Practice problems involving the calculation of integrals with varying areas and functions.

Emphasis on including units in integral calculations, especially for AP Calculus exams.

Conclusion summarizing the importance of understanding Riemann sums and integral properties.

Transcripts

Browse More Related Video

Business Calculus - Math 1329 - Section 5.3 - The Definite Integral and Fundamental Thm of Calculus

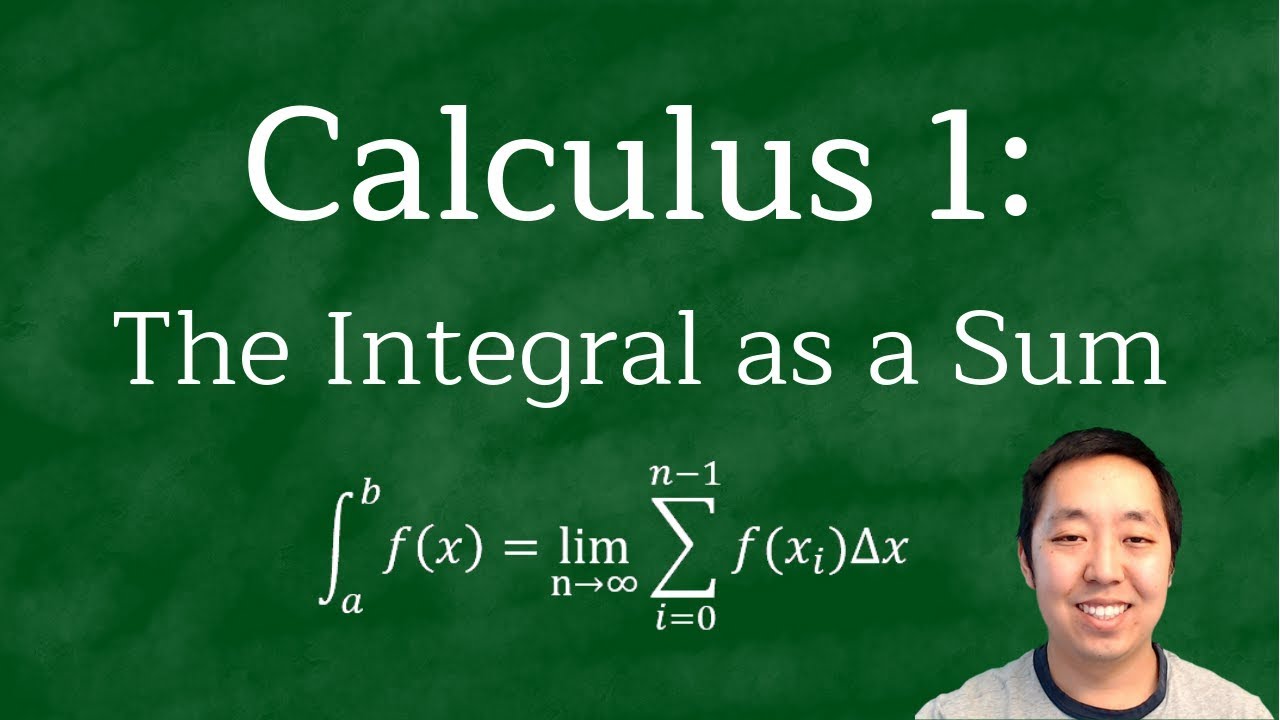
Calculus 1: Definition of the Integral Examples

Riemann and Trapezoidal Sums from Tables of Values

Riemann Sums - Midpoint, Left & Right Endpoints, Area, Definite Integral, Sigma Notation, Calculus

AP Calculus AB - 6.2 Approximating Areas with Riemann Sums

BusCalc 13.4 Riemann Sums

Related Tags

Area Calculation Graph Analysis Geometry Formulas Calculus Techniques Riemann Sums Integral Notation Exponential Functions Trapezoidal Sums AP Calculus Educational Content Math Tutorial