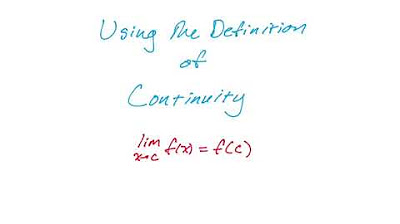Continuity - 2 Examples
TLDRThis video script discusses the concept of continuity in functions, using two examples to illustrate the process of determining if a function is continuous at a specific point. The first function, f(x), is shown to be continuous at x=1 by evaluating its limit and confirming it matches the function's value there. The second function, F(x), is analyzed at x=9, where the script explores the left and right limits and confirms the function's continuity by showing that both limits equal the function's value at x=9. The script effectively explains the mathematical process of checking for continuity, emphasizing the importance of evaluating limits from both directions.
Takeaways
- 📚 The script discusses Problem 2D from a probability worksheet, focusing on the continuity of a function at a specific point.
- 🔍 The first step in determining continuity is to check if the function is defined at the given point, which in this case is x = 1.
- 👍 The function is found to be defined at x = 1, with the value f(1) being 2, indicating a good start for continuity analysis.
- 🔄 The script then explores the behavior of the function as x approaches 1 but does not equal 1, which is crucial for understanding continuity.
- 📉 The function f(x) when x is not exactly 1 is given by the expression (x^2 - 1) / (x - 1), leading to a zero over zero indeterminate form.
- 🔑 Factoring is used to resolve the indeterminate form, revealing that the function simplifies to x + 1 as x approaches 1.
- 🎯 The limit of the simplified function as x approaches 1 is calculated to be 2, which matches the function's value at x = 1.
- 📈 Graphically, the function is shown to have no break at x = 1, with the y-value approaching 2 as x gets very close to 1 from both sides.
- 🔄 The script then moves on to Problem 2F, examining the continuity of a different function at x = 9.
- 🔢 The function at x = 9 is defined as the square root of (x + 1), which simplifies to 4 when x = 9.
- 🚦 The script investigates the limit from both the left and the right of x = 9, finding that both one-sided limits equal 4.
- 🌐 The function is confirmed to be continuous at x = 9, with no break in the function's graph, and the y-value closely approaching 4 as x approaches 9 from both directions.
Q & A
What is the first step in determining the continuity of a function at a given point?
-The first step is to check if the function is defined at the given value of x.
What is the value of f(x) when x is equal to 1 according to the script?
-When x is equal to 1, f(x) is simply equal to 2.
What is the expression for f(x) when x is not exactly 1?
-When x is not exactly 1, f(x) is expressed as (x^2 - 1) / (x - 1).
What is the result of the limit of f(x) as x approaches 1?
-The limit of f(x) as x approaches 1 exists and is equal to 2.
Why is there a 'zero over zero' case when x is close to 1 but not exactly 1?
-There is a 'zero over zero' case because both the numerator (x^2 - 1) and the denominator (x - 1) approach zero as x approaches 1.
What does the term 'continuity' imply in the context of a function?
-Continuity implies that there is no break in the function; the function is defined and the limit exists at the point of interest.
What is the function f(x) defined as when x is greater than or equal to 9?
-When x is greater than or equal to 9, f(x) is defined as the square root of (x + 1).
What is the value of f(x) when x is exactly 9 according to the script?
-When x is exactly 9, f(x) is equal to 4.
What is the expression for f(x) when x is less than 9?
-When x is less than 9, f(x) is expressed as the rational function (x^2 - 14x + 45) / (x - 9).
What is the result of the limit of f(x) as x approaches 9 from the left?
-The limit of f(x) as x approaches 9 from the left is equal to 4.
What is the result of the limit of f(x) as x approaches 9 from the right?
-The limit of f(x) as x approaches 9 from the right is also equal to 4.
Why is it necessary to consider both the left and right limits when checking for continuity at x = 9?
-It is necessary to consider both limits to ensure that the function behaves consistently as x approaches the point from both directions, which is a requirement for continuity.
Outlines
📚 Continuity Analysis at x=1
The first paragraph discusses the problem of determining the continuity of a function f(x) at the point x=1. The function is defined as 2 when x=1 and as (x^2 - 1) / (x - 1) for values of x other than 1. The speaker explains that the function is defined at x=1 and then proceeds to analyze the limit of f(x) as x approaches 1. They identify a zero-over-zero indeterminate form, which is resolved by factoring the numerator and canceling out the common factor with the denominator, resulting in a simple limit of 2 as x approaches 1. This indicates that the function is continuous at x=1, as there is no break or discontinuity in the function's value as x approaches this point.
🔍 Continuity Check at x=9 with Two-Sided Limit
The second paragraph continues the theme of continuity but shifts the focus to the point x=9. The function f(x) is defined piecewise, with one expression for x greater than or equal to 9 and another for x less than 9. The speaker first establishes that the function is defined at x=9 with a value of 4. They then examine the limit of f(x) as x approaches 9 from both the left and the right. For the left-hand limit, they simplify a rational function and find that it approaches 4 as x approaches 9 from the left. For the right-hand limit, the function simplifies to the square root of (x+1), which also approaches 4 as x approaches 9 from the right. Since both one-sided limits exist and are equal to the function's value at x=9, the speaker concludes that the function is continuous at x=9, with no breaks in the function's graph around this point.
📈 Graphical Interpretation of Continuity at x=9
The third paragraph provides a graphical interpretation of the continuity of the function at x=9. The speaker describes how the function's value approaches 4 as x gets very close to 9 from both the left and the right sides. They emphasize that there is no break in the function's graph, which confirms the continuity at x=9. This visual approach helps to reinforce the mathematical analysis from the previous paragraph, providing a clear understanding of the function's behavior around the point of interest.
Mindmap
Keywords
💡Continuity
💡Limit
💡Function
💡Factoring
💡Polynomial
💡Zero over Zero
💡Root
💡Rational Function
💡Two-Sided Limit
💡Graphical Interpretation
Highlights
Problem 2D from the probability sheet involves determining the continuity of a function at a specific point.
The function f(x) is evaluated for continuity at x = 1.
f(x) is defined at x = 1 with a value of 2.
The limit of f(x) as x approaches 1 is considered to determine continuity.
A zero over zero case is encountered when analyzing the limit.
The function simplifies to x + 1 after factoring and canceling terms.
The limit of f(x) as x approaches 1 is found to be 2, indicating continuity.
Graphical representation shows no break in the function at x = 1, confirming continuity.
Problem 2F examines the continuity of a different function at x = 9.
The function is defined at x = 9 with a value of 4.
A piecewise function requires analysis of the limit from both the left and right of x = 9.
The limit from the left as x approaches 9 is calculated.
The limit from the left is found to be 4 after factoring and simplifying.
The limit from the right as x approaches 9 is also calculated.
The limit from the right is 4, consistent with the function's value at x = 9.
The function is confirmed to be continuous at x = 9 with no break in the graph.
Transcripts
Browse More Related Video
5.0 / 5 (0 votes)
Thanks for rating: