Calculus- Lesson 8 | Derivative of a Function | Don't Memorise

Infinity Learn NEET
12 Apr 201908:20
EducationalLearning
32 Likes 10 Comments

TLDRThis educational video script delves into the concept of derivatives, which measure the rate of change of a dependent variable 'Y' with respect to an independent variable 'X'. It explains the process of differentiation, illustrating how the average rate of change converges to the instantaneous rate of change, represented as the derivative, as delta X approaches zero. The script uses a simple example of a squared function to demonstrate finding the derivative and clarifies the misconception of setting delta X to zero in calculations. It also touches on the importance of considering both positive and negative delta X values for a comprehensive understanding. The script ends with a teaser about finding the derivative of an absolute value function, encouraging viewers to continue learning.

Takeaways
  • πŸ“Š The derivative of a function measures the rate of change of a dependent variable 'Y' with respect to an independent variable 'X'.
  • πŸ” Differentiation is the process of finding the derivative of a function, which describes the rate of change at a specific point.
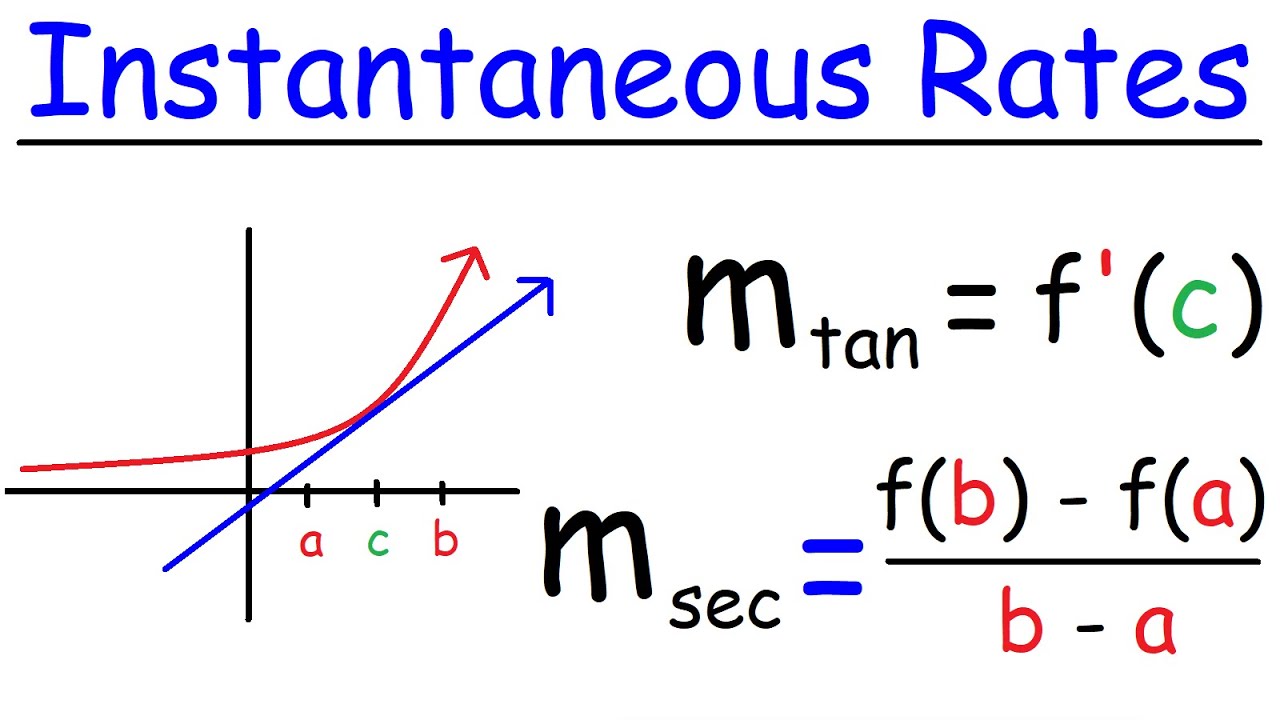
  • πŸ“ˆ The average rate of change between two values of 'X' is given by the ratio of the change in 'Y' to the change in 'X', represented as (F(X + Ξ”X) - F(X)) / Ξ”X.
  • πŸ“ As Ξ”X approaches zero, the average rate of change approaches the instantaneous rate of change, which is the derivative at point 'X'.
  • 🚫 It's incorrect to substitute Ξ”X with zero in the ratio as it would lead to a 'zero divided by zero' scenario.
  • πŸ”„ The concept of 'delta X tending to zero' means considering values of Ξ”X that are increasingly smaller, but never actually zero.
  • πŸ“š The derivative of a function at a particular point 'X' can be found by considering the average rate of change for both positive and negative increments of 'X'.
  • πŸ€” The derivative of a function at 'X' is the value that both the average rates from positive and negative increments converge to as Ξ”X approaches zero.
  • πŸ‘‰ The example given in the script demonstrates the process of finding the derivative of the function Y = X^2 at a specific point 'X'.
  • πŸ’¬ The script invites viewers to think about finding the derivative of the absolute value function at 'X' equal to zero, a topic to be covered in a future lesson.
Q & A

  • What does the derivative of a function measure?

    -The derivative of a function measures the 'rate of change' of a dependent variable 'Y' with respect to an independent variable 'X'.

  • What is the relationship between variable 'Y' and variable 'X' when 'Y' is a function of 'X'?

    -When 'Y' is a function of 'X', it means that the value of 'Y' is determined by the value of 'X', with 'Y' being the dependent variable and 'X' being the independent variable.

  • What is the process called that involves finding the derivative of a function?

    -The process of finding the derivative of a function is called Differentiation.

  • How is the average rate of change between two values of 'X' calculated?

    -The average rate of change between two values of 'X' is calculated as the ratio of the change in 'Y' to the change in 'X', also known as the slope of the secant line between the two points.

  • What does it mean for the average rate of change to approach the tangent line at 'X not'?

    -As the interval between 'X not' and 'X not plus delta X' gets smaller and closer to 'X not', the secant lines approach the tangent line at 'X not', which represents the instantaneous rate of change at that point.

  • Why can't we simply put 'Delta X' equal to zero in the average rate of change ratio?

    -Putting 'Delta X' equal to zero in the average rate of change ratio would result in a 'zero divided by zero' situation, which is undefined and does not make sense.

  • What is the significance of considering 'Delta X' tending to zero?

    -Considering 'Delta X' tending to zero allows us to find the instantaneous rate of change at 'X not', which is the derivative of the function at that point.

  • How is the derivative of a function represented in notation?

    -The derivative of a function is represented by putting a dash, or prime symbol, on the notation for the function, such as 'f'(x)'.

  • What is the example function given in the script to illustrate finding the derivative?

    -The example function given is 'Y' equal to the square of 'X', or 'Y = X^2'.

  • Why is it necessary to consider both cases when 'delta X' is greater than zero and when 'delta X' is less than zero?

    -It is necessary to consider both cases to ensure that the average rates approach the same limit as 'delta X' tends to zero, which confirms the derivative of the function at that particular value of 'X'.

  • What is the absolute value or modulus function, and how does it behave at 'X' equal to zero?

    -The absolute value or modulus function is represented by vertical bars and outputs the non-negative value of 'X'. At 'X' equal to zero, the function behaves as 'Y' equals 'X', and when 'X' is less than zero, 'Y' equals the negative of 'X'.

Outlines
00:00
πŸ“ˆ Understanding the Derivative and Differentiation

This paragraph introduces the concept of the derivative as a measure of the rate of change of a function. It explains how the dependent variable 'Y' changes with respect to the independent variable 'X', and how the derivative at a specific value of 'X' represents the instantaneous rate of change. The process of finding the derivative, known as differentiation, is summarized, highlighting the transition from the average rate of change to the instantaneous rate as the interval 'delta X' approaches zero. The paragraph uses the example of a function where 'Y' is the square of 'X' to illustrate the calculation of the derivative, emphasizing the importance of considering 'delta X' tending towards zero without actually being zero, which would lead to an undefined expression.

05:02
πŸ” Deep Dive into the Derivative Calculation and Limit Concept

This paragraph delves deeper into the process of calculating the derivative, focusing on the theoretical aspects and the limit concept. It clarifies the misconception about setting 'delta X' equal to zero in the calculation process, explaining that 'delta X' tends to zero means considering values closer and closer to zero, but never actually zero. The paragraph further discusses the importance of examining both cases where 'delta X' is greater than and less than zero to ensure the average rate of change converges to the same value, which is then identified as the derivative of the function at a particular 'X'. The example of the absolute value or modulus function is introduced, posing a question about finding its derivative at 'X' equal to zero, and promising to address it in the next lesson.

Mindmap
Keywords
πŸ’‘Derivative
The derivative of a function is a fundamental concept in calculus that measures the rate of change of the function's output with respect to its input. In the context of the video, it is used to determine how quickly the dependent variable 'Y' changes as the independent variable 'X' changes. The script explains that the derivative at a particular value of 'X' gives the instantaneous rate of change at that point, which is crucial for understanding the function's behavior.
πŸ’‘Rate of Change
Rate of change refers to how fast or slow a quantity changes with respect to another. In the video script, it is used to describe the speed at which the value of 'Y' varies as 'X' changes. The concept is central to understanding derivatives, as the derivative itself quantifies the rate of change at a specific point in the function.
πŸ’‘Dependent Variable
A dependent variable, denoted by 'Y' in the script, is a variable that depends on the value of another variable. It is the output of a function and changes in response to changes in the independent variable. The video discusses how 'Y' is a function of 'X', and the derivative helps in understanding how 'Y' changes with 'X'.
πŸ’‘Independent Variable
The independent variable, represented by 'X' in the script, is a variable that can be manipulated freely without being dependent on other variables. It is the input of a function and controls how the dependent variable 'Y' changes. The concept is essential for discussing how the derivative is calculated with respect to 'X'.
πŸ’‘Function
A function, denoted by 'F' in the script, is a mathematical relationship that associates each input (independent variable 'X') with exactly one output (dependent variable 'Y'). The video explains that the function describes how 'Y' changes with 'X', and finding the derivative of a function is a way to analyze this relationship.
πŸ’‘Differentiation
Differentiation is the process of finding the derivative of a function. It is a key operation in calculus and is used to determine the rate at which a function's value changes. The script summarizes this process and explains how it leads to the calculation of the derivative, which is a central theme of the video.
πŸ’‘Average Rate of Change
The average rate of change is the ratio of the change in the function's output to the change in its input over a specific interval. In the script, it is used to describe the slope of the secant line between two points on the function's graph. As the interval shrinks, this average rate approaches the instantaneous rate of change, which is the derivative.
πŸ’‘Secant Line
A secant line is a straight line that intersects a curve at two or more points. In the context of the video, the secant line represents the average rate of change between two values of 'X'. As the interval between these 'X' values becomes smaller, the secant line approaches the tangent line, which represents the instantaneous rate of change.
πŸ’‘Tangent Line
A tangent line is a straight line that touches a curve at a single point without crossing it. In the video, the tangent line at a particular value of 'X' is the limit of the secant lines as the interval between the 'X' values approaches zero. It represents the instantaneous rate of change at that point and is used to define the derivative.
πŸ’‘Instantaneous Rate of Change
The instantaneous rate of change is the rate at which a function's value changes at a specific point, as opposed to the average rate of change over an interval. The script explains that as delta 'X' approaches zero, the average rate of change approaches this instantaneous rate, which is the derivative of the function at that point.
πŸ’‘Limit
In calculus, a limit is the value that a function or sequence approaches as the input approaches a certain value. The script discusses the concept of the limit in the context of delta 'X' tending to zero, which is crucial for defining the derivative as the limit of the average rate of change.
πŸ’‘Modulus Function
The modulus function, also known as the absolute value function, is a mathematical function that returns the non-negative value of a number. In the script, it is used as an example to discuss the potential discontinuity in the derivative at certain points, such as 'X' equal to zero, which is a point of interest for the next lesson.
Highlights

Derivative of a function measures the 'RATE of change'.

Y is a FUNCTION of X, where Y is the dependent variable and X is the independent variable.

The derivative of a function at a particular value of X tells the RATE of change of Y with respect to X at that value.

Differentiation is the process of finding the derivative of a function.

The average rate of change between two values of X is the slope of the secant line between those points.

As the interval approaches X, secant lines approach the tangent line at X.

The limit as delta X tends to Zero of the average rate of change is the instantaneous rate of change at X.

The derivative is denoted by a dash on the notation for the function.

Putting 'Delta X' equal to zero in the ratio does not make sense as it results in 'zero divided by zero'.

To find the derivative, consider smaller and smaller values of 'Delta X', but never zero.

The derivative of Y = square of X at a particular X is found by considering the average rate for delta X tending to zero.

Substituting 'delta X' equal to zero is a convenient way to denote the limit as delta X approaches zero.

The derivative of a function at X is determined by considering both when delta X is greater than and less than zero.

The absolute value or modulus function is defined by Y being the non-negative value of X.

The derivative of the absolute value function at X equals zero requires special consideration.

The next lesson will cover finding the derivative of the absolute value function.

Transcripts

Browse More Related Video

BusCalc 18 Second Derivatives

Partial Derivatives!

Derivatives! The Limit Definition

Instantaneous Rate of Change

Introduction to calculus [IB Maths AI SL/HL]

Why the gradient is the direction of steepest ascent

Rate This

5.0 / 5 (0 votes)

Thanks for rating:

Related Tags
DerivativesDifferentiationRate of ChangeDependent VariableIndependent VariableFunction AnalysisInstantaneous SpeedAverage RateTangent LineMathematics Education