How to Explain Calculus to a 6th Grader?
TLDRThis video script offers a simplified explanation of calculus for a young audience, focusing on two core concepts: integrals and derivatives. The script begins by demystifying infinity and infinitesimals, setting the stage for understanding calculus. It then introduces integrals through the practical example of finding the area of a triangle using circular units, illustrating the transition from discrete to continuous quantities. Derivatives are explained as the slopes of tangent lines on a curve, emphasizing their role in tracking changes. The script effectively conveys the power of calculus in solving complex problems and describes it as the mathematics of change, promising a series on calculus tailored for 6th graders.
Takeaways
- 🌌 Infinity is not a number but a concept representing something unlimited, endless, or without bound.
- 🔍 An infinitesimal is a concept where something is approaching zero but not exactly equal to zero, which is crucial for understanding calculus.
- 📏 Integrals in calculus are used to find areas and volumes of irregular shapes by summing up infinitesimally small areas.
- 📐 The process of finding the area under a curve involves slicing the region into smaller rectangles and summing their areas, represented by the integral notation ∫f(x)dx from a to b.
- 💡 The concept of derivatives in calculus is about finding the slope of a curve at a specific point, which is the rate of change.
- 📈 Derivatives are calculated by considering an infinitely narrow interval where the curve can be approximated as a straight line, giving the instantaneous rate of change.
- 🚀 Calculus is referred to as the mathematics of change because it allows us to quantify and describe changes in various phenomena such as the path of a missile or stock market behavior.
- 📚 The two main concepts in calculus are integrals, which help find areas and volumes, and derivatives, which track changes in slopes of tangent lines to curves.
- 🛠 By combining integral and derivative operations with other mathematical topics, calculus becomes a powerful tool to solve complex real-world problems.
- 👨🏫 The video script aims to introduce the foundational concepts of calculus to a younger audience, emphasizing its applicability and importance in understanding change.
Q & A
What is the primary concept discussed in the first part of the response?
-The primary concept discussed in the first part is the concept of infinity, explaining that it is not a number but a concept representing something unlimited, endless, or without bound.
How does the speaker illustrate the idea that there are infinite numbers between two and three?
-The speaker illustrates this by showing that you can always add a '1' at the end of a decimal number between two and three, creating another number within that range, thus demonstrating the endless possibilities.
What is an infinitesimal and how is it related to the concept of infinity?
-An infinitesimal is a quantity that is infinitely small, represented as one over infinity. It is related to the concept of infinity as it approaches zero but is not exactly zero, which is a key concept used in calculus.
How does the speaker explain the concept of integrals using the example of a triangle?
-The speaker explains integrals by showing how to approximate the area of a triangle using circular units of different sizes (quarters, nickels, dimes), and then conceptually using an infinitely small width to represent the limit of these approximations.
What is the purpose of using smaller circular units to cover the triangle in the integral concept explanation?
-The purpose is to create a more precise estimate of the triangle's area. As the units get smaller, the gaps between them decrease, leading to a better approximation of the actual area.
Can you explain the concept of derivatives as introduced in the script?
-Derivatives in calculus represent the rate of change or the slope of a curve at a particular point. It is the limit of the slope of the curve as the interval (run) approaches zero, which is the concept of an infinitesimal.
How does the script relate the concept of derivatives to real-world scenarios?
-The script relates derivatives to scenarios such as the path of a missile, where its trajectory involves changes in direction and speed, and the behavior of the stock market, which experiences fluctuations and changes over time.
What is the significance of the integral symbol (∫) in calculus?
-The integral symbol represents the process of integration, which involves summing up infinitesimally small areas (or volumes) to find the total area under a curve or the volume of a shape.
Why are integrals and derivatives considered inverse operations in calculus?
-Integrals and derivatives are inverse operations because one undoes what the other does. Integration finds the accumulated total (area or volume), while differentiation finds the rate of change or slope at a specific point.
What is the broader implication of understanding both integrals and derivatives in calculus?
-Understanding both integrals and derivatives allows one to solve a wide range of problems involving areas, volumes, and rates of change, making calculus a powerful tool for analyzing and describing various phenomena in the world.
Outlines
🌌 Introduction to Infinity and Calculus
The first part of the video script introduces the concept of infinity, explaining that it is not a number but a concept representing something unlimited or without bound. The presenter uses the example of numbers between two and three to illustrate the endless nature of infinity. It then delves into the concept of an infinitesimal, which is one over infinity, and explains that it approaches zero but is not exactly zero. These foundational concepts are crucial for understanding the integral and derivative components of calculus. The script also introduces the idea of using smaller and smaller units to approximate areas, setting the stage for the integral concept in calculus.
📏 The Concept of Integrals in Calculus
The second paragraph focuses on the concept of integrals, which are used to calculate areas and volumes of irregular shapes. The presenter uses the analogy of covering a triangle with circular units of different sizes (quarters, nickels, and dimes) to demonstrate how smaller units can lead to a more precise estimate of an area. This leads to the idea of using infinitesimally small units, represented by 'one over infinity', to find the exact area under a curve. The script explains that integrals involve summing up the areas of these infinitesimally small rectangles, represented mathematically as the integral of a function from a lower to an upper boundary. This concept is key to solving problems that cannot be addressed with traditional algebra and geometry.
🚀 The Concept of Derivatives in Calculus
The final paragraph discusses derivatives, which are the rates of change or slopes of curves at specific points. The script begins by explaining the concept of slope with straight lines and then transitions to the challenge of finding the slope of a curve, which has varying inclines. The presenter introduces the idea of taking an infinitely small interval, where the curve can be approximated as a straight line, allowing for the calculation of the slope at that point. This slope, when the interval approaches zero, is defined as the derivative. The script highlights the practical applications of derivatives, such as tracking the path of a missile or the fluctuations of the stock market, emphasizing that calculus is the mathematics of change. The video concludes by emphasizing the importance of understanding both integrals and derivatives as the core concepts of calculus.
Mindmap
Keywords
💡Infinity
💡Infinitesimal
💡Integrals
💡Derivatives
💡Slope
💡Area
💡Volume
💡Function
💡Differential
💡Tangent
Highlights
Introduction to the concept of infinity as not a number but a concept of being unlimited, endless, or without bound.
Explanation of the infinite numbers between two and three to illustrate the idea of infinity.
Introduction of the concept of infinitesimals, where one over infinity is used to approach zero.
The use of pizza analogy to explain the concept of infinitesimals and their value approaching zero.
Transition to the main concepts of calculus: integrals and derivatives, using the concepts of infinity and infinitesimals.
Explanation of integrals as a method to find the area of shapes, using the example of covering a triangle with circular units.
The progression from using quarters to nickels to dimes to illustrate the refinement of area estimation in integrals.
Concept of making the width of columns one over infinity to represent the idea behind integrands.
Introduction of the integral notation and its meaning in summing up small areas to find the total area under a curve.
The power of calculus to solve problems in finding areas and volumes of irregular shapes that cannot be solved with regular algebra and geometry.
Introduction to the concept of derivatives as the slope of a curve at a specific point.
Explanation of how derivatives can be used to describe changes, such as the path of a missile or the stock market behavior.
The relationship between integrals and derivatives as inverse operations in calculus.
Importance of understanding the integral for finding areas and volumes, and the derivative for tracking changes in slopes.
The practical applications of calculus in describing the world's changes mathematically, emphasizing its role as the mathematics of change.
Announcement of a series on calculus for 6th graders to further explore these concepts.
Transcripts
Browse More Related Video

Unit II: Lec 2 | MIT Calculus Revisited: Single Variable Calculus
Introduction to calculus [IB Maths AI SL/HL]

Math Test Practice Test [ 30 Minutes ] Math for Business and Economics
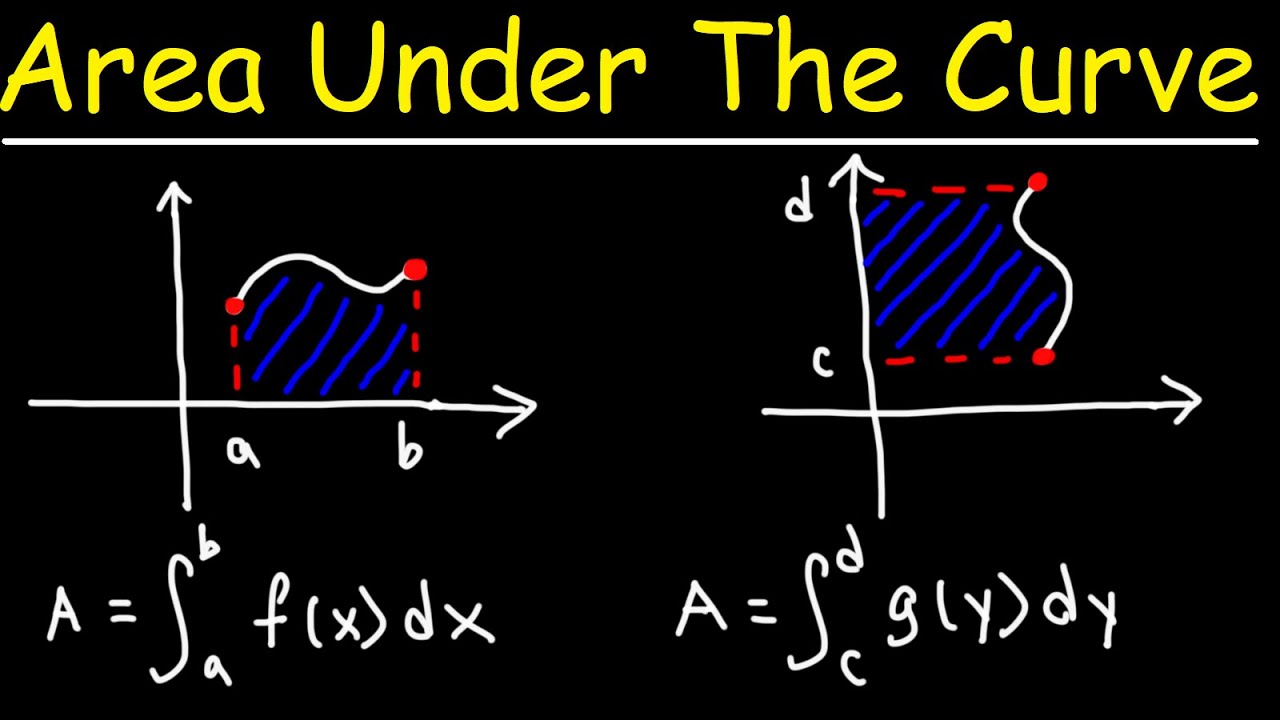
Finding The Area Under The Curve Using Definite Integrals - Calculus
Mastering Calculus: An Introduction to Integrals

Calculus II: Partial Derivatives & Integrals — Subject 4 of Machine Learning Foundations
5.0 / 5 (0 votes)
Thanks for rating: