Circular Motion: Ferris Wheel

xmtutor
9 Oct 201604:51
EducationalLearning
32 Likes 10 Comments

TLDRThis video delves into the physics of a man's weight fluctuations during a Ferris wheel ride. It explains that the man's actual weight remains constant, but the normal contact force with the floor changes due to centripetal force requirements. At the bottom, the force is greater than the man's weight, making him feel heavier; at the top, it's less, making him feel lighter. At 3 and 9 o'clock positions, the force equals his weight, provided by friction. As the wheel spins faster, these forces become more pronounced, leading to a scenario of weightlessness at the top and double the normal weight at the bottom, illustrating the fascinating interplay of forces in circular motion.

Takeaways
  • 🎢 The man's actual weight does not change during a Ferris wheel ride, but the perceived weight does due to changes in the normal contact force.
  • 🔽 At the bottom of the ride, the normal contact force is greater than the man's weight, creating a sensation of being heavier.
  • 🔼 At the top of the ride, the normal contact force is less than the man's weight, leading to a feeling of weightlessness.
  • 🌀 The centripetal force required for circular motion is provided by the difference between the normal contact force and the man's weight.
  • ↔ At the 3:00 and 9:00 positions, the net force is zero, meaning the normal contact force equals the man's weight, and friction provides the necessary centripetal force.
  • 📉 The normal contact force varies throughout the ride, being maximum at the bottom and minimum at the top, with equilibrium at the 3:00 and 9:00 positions.
  • 🚀 As the Ferris wheel spins faster, the required centripetal force increases, affecting the normal contact force at the top and bottom more significantly.
  • 💥 If the Ferris wheel spins extremely fast, the man could experience zero normal contact force at the top, leading to complete weightlessness.
  • 🤔 At high speeds, friction may not be sufficient to provide the necessary centripetal force, causing the man to slide outward until pressing against the capsule wall.
  • 💪 At the bottom position with extreme speed, the normal contact force could be twice the man's weight, making it nearly impossible to do a push-up.
  • 🧍‍♂️ The man is pressed firmly against the floor at the bottom position under high speed, due to the increased normal contact force.
Q & A

  • Why does a man's apparent weight change during a Ferris wheel ride?

    -A man's apparent weight changes due to the variation in the normal contact force between him and the floor of the Ferris wheel carriage as he moves through different positions on the wheel.

  • What is the direction of the centripetal force when the man is at the bottom of the Ferris wheel?

    -At the bottom of the Ferris wheel, the centripetal force direction is upward because the net force must also be upward to keep the man moving in a circular path.

  • Why is the normal contact force greater than the man's weight at the bottom of the ride?

    -The normal contact force is greater than the man's weight at the bottom to provide the necessary centripetal force to keep him moving in a circle, which can be represented by the equation: normal contact force - weight = m*r*ω^2.

  • What happens to the normal contact force when the man reaches the top of the Ferris wheel?

    -At the top, the centripetal force direction is downward, so the normal contact force becomes smaller than the man's weight to provide the required centripetal force, which can be represented by the equation: weight - normal contact force = m*r*ω^2.

  • Why does the man feel lighter at the top of the Ferris wheel?

    -The man feels lighter at the top because the normal contact force is reduced to be less than his weight, providing the necessary centripetal force for circular motion.

  • At what positions on the Ferris wheel does the man feel his normal weight?

    -The man feels his normal weight at the 3:00 and 9:00 positions, where the centripetal force is provided by friction, and the normal contact force equals his weight.

  • What provides the centripetal force when the man is at the 3:00 and 9:00 positions on the Ferris wheel?

    -At the 3:00 and 9:00 positions, the centripetal force is provided by friction since the normal contact force is exactly equal to the man's weight.

  • What would happen if the Ferris wheel spins at an extremely high speed?

    -If the Ferris wheel spins very fast, the required centripetal force (m*r*ω^2) increases, causing the normal contact force to increase at the bottom and decrease at the top, and the frictional force to become larger at the 3:00 and 9:00 positions.

  • Describe the situation when the angular velocity (ω) is so large that m*ω^2 equals the man's weight (mg).

    -When m*ω^2 equals mg, at the top of the Ferris wheel, the normal contact force drops to zero, resulting in the man experiencing weightlessness. At the 3:00 and 9:00 positions, friction may not be sufficient to provide the necessary centripetal force, causing the man to slide outward.

  • What would the man experience at the bottom of the Ferris wheel if the normal contact force becomes 2mg?

    -If the normal contact force is 2mg at the bottom, the man would feel twice his normal weight, making it nearly impossible to do a push-up as he would need to exert a force greater than twice his weight on the floor.

Outlines
00:00
🎡 Physics of Weight Variation on a Ferris Wheel

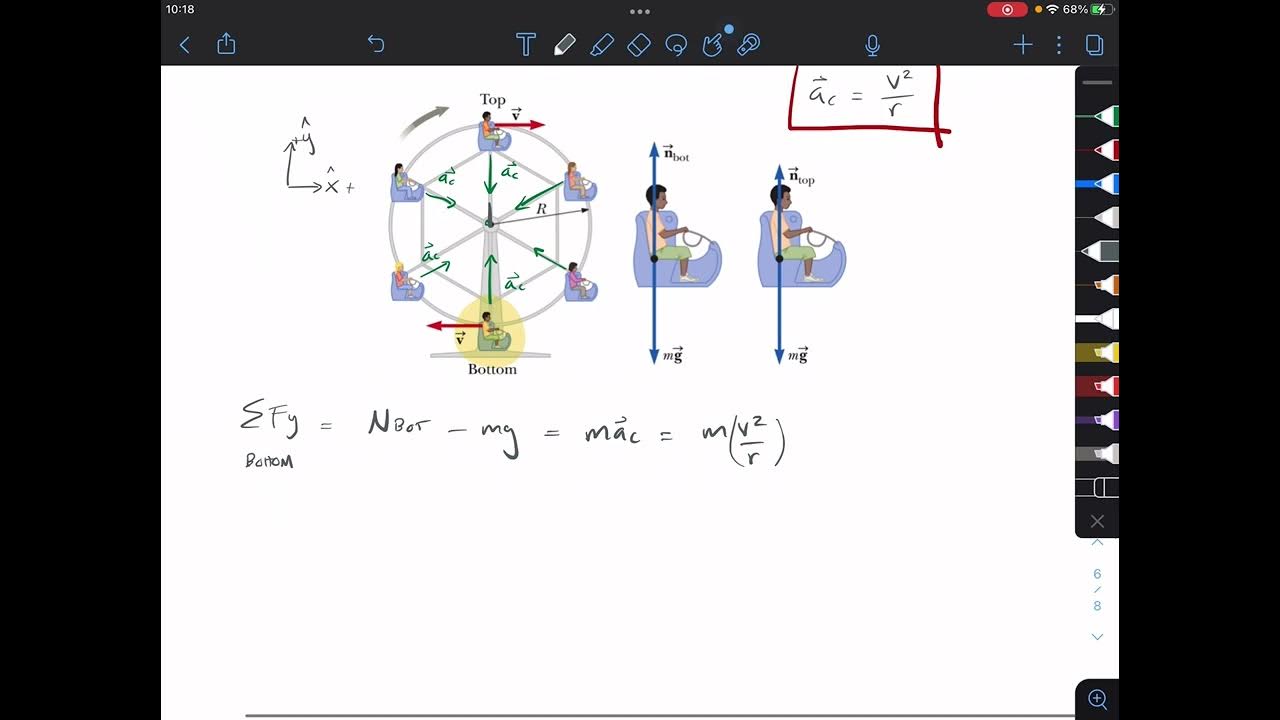
This paragraph explains the physics behind the sensation of weight change during a Ferris wheel ride. It clarifies that a person's actual weight remains constant, but the normal force exerted by the seat varies depending on the wheel's rotation and the person's position. At the bottom of the wheel, the normal force is greater than the person's weight due to the upward centripetal force required for circular motion, leading to the sensation of being heavier. Conversely, at the top, the normal force is less than the weight, causing the sensation of weightlessness. The force is exactly equal to the weight at the 3:00 and 9:00 positions, where the centripetal force is provided by friction. The paragraph also discusses extreme scenarios where the wheel spins very fast, leading to significant variations in the normal force and sensations of weightlessness or double the normal weight.

Mindmap
Keywords
💡Ferris Wheel Ride
A Ferris wheel ride refers to the experience of being on a large, vertical, rotating wheel that carries passengers in individual cabins or seats. In the video, the Ferris wheel serves as the central example to illustrate how a person's perceived weight changes during different positions on the ride. It is the basis for the discussion on centripetal force and its effects on the normal contact force between the man and the wheel.
💡Normal Contact Force
Normal contact force is the force exerted by a surface that supports the weight of an object resting on it. In the context of the video, it is the force exerted by the floor of the Ferris wheel cabin on the man. The script explains how this force varies depending on the man's position on the wheel, being maximum at the bottom and minimum at the top, and equal to the man's weight at the 3:00 and 9:00 positions.
💡Weight
Weight is the force exerted on a mass by gravity and is calculated as mass times the acceleration due to gravity (mg). The video clarifies that while a man's actual weight remains constant, the normal contact force, which he feels as 'weight', changes during the ride. This is a key concept in understanding the sensation of weightlessness and increased weight at different points on the Ferris wheel.
💡Centrifugal Force
Centrifugal force is the apparent force that pushes a mass away from the center of a circular path. The video script uses this concept to explain why the man feels lighter at the top and heavier at the bottom of the Ferris wheel. However, it's important to note that centrifugal force is a fictitious or pseudo force experienced in a rotating frame of reference.
💡Centripetal Force
Centripetal force is the actual force that keeps an object moving in a circular path, directed towards the center of the circle. In the video, it is the required centripetal force (m*r*Ω^2) that causes the changes in the normal contact force experienced by the man on the Ferris wheel. This force is essential for maintaining circular motion and is provided by the normal contact force and friction at different positions.
💡Friction
Friction is a force that resists the relative motion between two surfaces in contact. In the context of the video, friction plays a crucial role at the 3:00 and 9:00 positions on the Ferris wheel, where it provides the necessary centripetal force to keep the man moving in a circle. The script also mentions that at very high speeds, friction might become insufficient, leading to the man sliding outward.
💡Weightlessness
Weightlessness is the state in which an object appears to have no weight, often experienced in free fall or in space. The video describes a hypothetical scenario where the Ferris wheel spins so fast that at the top, the normal contact force drops to zero, resulting in the man experiencing weightlessness, as if he is hovering inside the cabin.
💡Acceleration
Acceleration is the rate of change of velocity of an object. In the video, the term is not explicitly mentioned, but it is implied in the formula for centripetal force (m*r*Ω^2), where Ω (omega) represents angular velocity. The script uses the concept of increasing acceleration (as the wheel spins faster) to explain the increasing difference between the normal contact force and the man's weight.
💡Push-Up
A push-up is a common bodyweight exercise where a person supports their body weight with their hands and toes, lowering and raising their body. The video uses the example of a push-up to illustrate the difficulty the man would face at the bottom of the Ferris wheel if the normal contact force becomes 2mg, making it nearly impossible to lift his body weight against the floor.
💡Muscle Force
Muscle force is the force generated by the contraction of muscles. The video script mentions that most people's muscles are not able to exert a force of twice their weight, which is relevant when discussing the man's inability to do a push-up at the bottom of the Ferris wheel if the normal contact force is 2mg, indicating the physical limitations of the human body in such scenarios.
Highlights

A man's perceived weight changes during a Ferris wheel ride due to variations in the normal contact force between the man and the floor.

At the bottom of the Ferris wheel, the normal contact force is greater than the man's weight due to the upward central force.

The equation for the bottom position is upward normal contact force minus weight equals the centripetal force (m*r*Ω^2).

At the top of the ride, the normal contact force is less than the man's weight because the central force is downward.

The equation for the top position is weight minus upward normal contact force equals the required centripetal force.

At the 3:00 and 9:00 positions, the normal contact force equals the man's weight, and friction provides the necessary centripetal force.

The normal contact force is not constant; it's maximum at the bottom and minimum at the top, equaling the weight only at 3:00 and 9:00 positions.

A man feels lighter when moving upward and heavier when moving downward on a Ferris wheel.

At 3:00 and 9:00 positions, the man feels his normal weight due to the balance of forces.

A slow Ferris wheel旋转速度较慢，导致人几乎感觉不到正常接触力与重力的差异。

As the Ferris wheel spins faster, the required centripetal force (m*r*Ω^2) increases, affecting the normal contact force.

At extremely high speeds, the normal contact force at the top could drop to zero, causing the man to experience weightlessness.

At high speeds, friction at the 3:00 and 9:00 positions may not be sufficient to provide the necessary centripetal force.

If the man slides outward at high speeds, the normal contact force increases to keep him in circular motion.

At the bottom position with very high speeds, the normal contact force could be twice the man's weight.

Performing a push-up at the bottom position with high speeds would be nearly impossible due to the force required.

Most people's muscles cannot exert a force of twice their weight, so the man would be pressed onto the floor.

Transcripts

Browse More Related Video

Circular motion and apparent weight

Uniform Circular Motion - 3 - Ferris Wheel

Elevator Physics Problem - Normal Force on a Scale & Apparent Weight

Normal Force on Ferris Wheel

College Physics 1: Lecture 22 - Apparent Forces, Orbits, and Gravity

How Many Different Types of Forces Are There In Physics?

Rate This

5.0 / 5 (0 votes)

Thanks for rating:

Related Tags
PhysicsFerris WheelWeight PerceptionCentrifugal ForceCentripetal ForceFrictionWeightlessnessCircular MotionForce AnalysisSpeed Impact