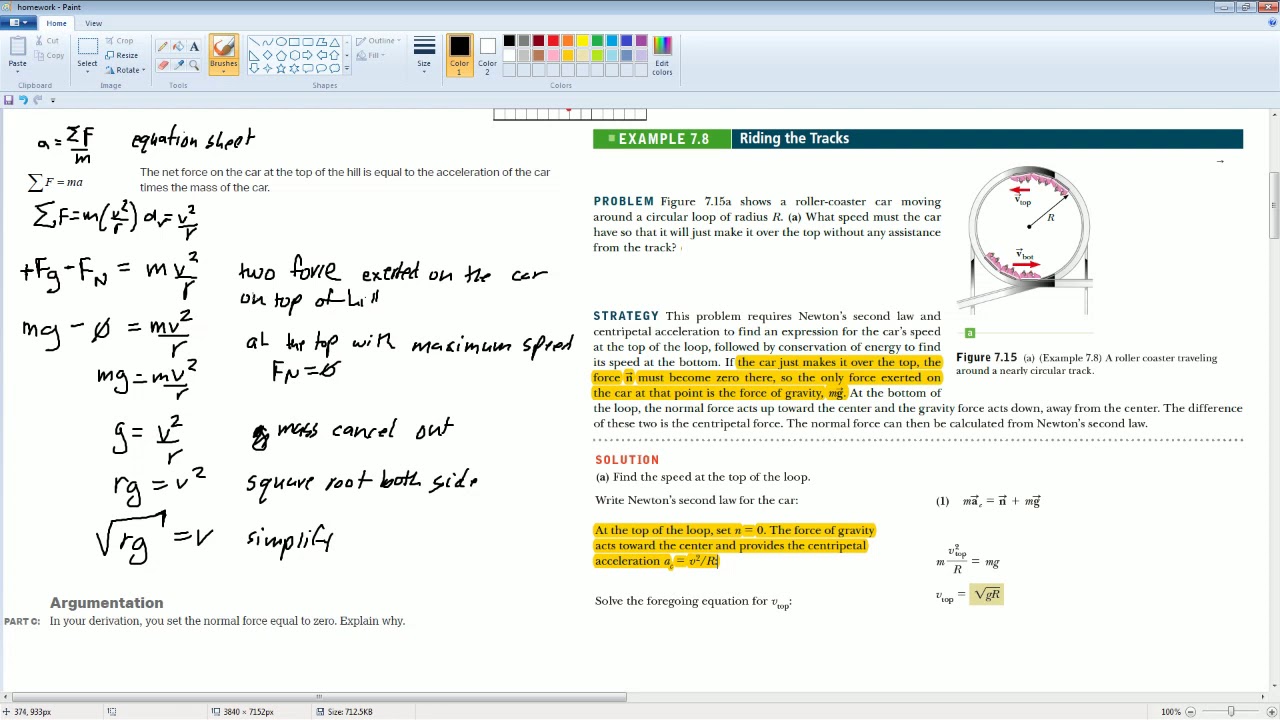
Uniform Circular Motion - 3 - Ferris Wheel

redSTEM
25 Feb 202204:55
EducationalLearning
32 Likes 10 Comments

TLDRThe script discusses a physics problem involving a child of mass m on a rotating ferrous wheel, moving in a vertical circle with a radius of 10 meters and a constant speed of 3 m/s. The goal is to calculate the normal force exerted by the seat on the child at the top and bottom of the ride. The explanation covers the concept of centripetal acceleration and the application of Newton's second law to find the normal force at different points. At the bottom, the child experiences an increased force due to the sum of weight and centripetal force, while at the top, the force is reduced as the centripetal force opposes the weight. The final calculations suggest the child feels 1.09 times their weight at the bottom and 0.908 times at the top, illustrating the effect of centripetal acceleration on perceived weight.

Takeaways
  • 🎡 The problem involves a Ferris wheel rotating with a constant velocity.
  • 👦 A child of mass m moves in a vertical circle with a radius of 10 meters at a constant speed of 3 meters per second.
  • 📏 The task is to determine the normal force exerted by the seat on the child at the bottom and top of the ride.
  • 📐 At all points, the objects on the Ferris wheel experience centripetal acceleration pointing towards the center of the circle.
  • 🧭 The coordinate system is chosen with positive y upwards and positive x to the right.
  • 🔽 At the bottom, the sum of forces in the y-direction is N_bot (upwards) minus m*g (downwards), equal to m*a_c (centripetal acceleration).
  • 🆙 At the top, the sum of forces in the y-direction is N_top (upwards) minus m*g (downwards), equal to -m*a_c (downwards centripetal acceleration).
  • ⚖️ The normal force at the bottom (N_bot) is m*v^2/r + m*g.
  • 🧮 The normal force at the top (N_top) is -m*v^2/r + m*g.
  • 📉 At the bottom, the child feels heavier due to the added centripetal force, while at the top, the child feels lighter due to the subtracting centripetal force.
  • 🔍 Calculations give N_bot = 1.09 * m*g and N_top = 0.908 * m*g.
Q & A

  • What is the problem scenario described in the transcript?

    -The problem involves a Ferris wheel rotating with a constant velocity, with a child of mass m moving in a vertical circle of radius 10 meters at a constant speed of 3 meters per second. The goal is to determine the force exerted by the seat on the child at the top and bottom of the ride.

  • What is the centripetal acceleration equation mentioned in the script?

    -The centripetal acceleration equation mentioned is a_c = v^2 / r, where v is the linear speed and r is the radius of the circular path.

  • What coordinate system is chosen in the analysis?

    -The coordinate system chosen has positive y upwards and positive x to the right.

  • How are the forces at the bottom of the ride analyzed?

    -At the bottom of the ride, the forces analyzed include the normal force pointing up (N_bottom) and the gravitational force pointing down (mg). The net force equation is N_bottom - mg = m(v^2 / r).

  • How are the forces at the top of the ride analyzed?

    -At the top of the ride, the forces analyzed include the normal force pointing up (N_top) and the gravitational force pointing down (mg). The net force equation is N_top - mg = -m(v^2 / r), considering the centripetal acceleration direction.

  • What is the expression for the normal force at the bottom of the ride?

    -The normal force at the bottom of the ride (N_bottom) is given by the equation N_bottom = m(v^2 / r) + mg.

  • What is the expression for the normal force at the top of the ride?

    -The normal force at the top of the ride (N_top) is given by the equation N_top = mg - m(v^2 / r).

  • How does the child’s apparent weight compare at the top and bottom of the ride?

    -At the bottom of the ride, the child feels heavier due to the combined effect of gravity and centripetal force, resulting in a larger normal force. At the top of the ride, the child feels lighter because the centripetal force subtracts from the gravitational force, resulting in a smaller normal force.

  • What are the numerical results for the normal forces in terms of mg?

    -The normal force at the bottom of the ride is greater than at the top of the ride due to the addition of centripetal force to the gravitational force.

Outlines
00:00
🎢 Physics of a Ferris Wheel Ride

The video script discusses a physics problem involving a child of mass m riding on a Ferris wheel that rotates with a constant velocity. The wheel has a radius of 10 meters, and the child moves in a vertical circle with a constant speed of 3 meters per second. The goal is to calculate the normal force exerted by the seat on the child at the bottom and top of the ride, which corresponds to the force the seat presses against the child. The script introduces the concept of centripetal acceleration, which is the force that keeps the child moving in a circular path, pointing towards the center of the circle. The script sets up the coordinate system and uses Newton's second law to derive the equations for the normal force at the bottom (n_bot) and top (n_top) of the ride. It explains that at the bottom, the normal force is the sum of the child's weight and the centripetal force, while at the top, it is the difference between the child's weight and the centripetal force. The script provides a conceptual overview without going into the specifics of the calculations, which are left to the viewer to solve.

Mindmap
Keywords
💡Ferrous Wheel
A ferrous wheel refers to a wheel made of iron or containing iron, which is a magnetic material. In the context of the video, it is a wheel that rotates and is part of a ride or a mechanical system. The script mentions a 'ferrous wheel' to describe the type of wheel involved in the physics problem being discussed, which is rotating with a constant velocity.
💡Constant Velocity
Constant velocity is a state of motion where an object moves at a fixed speed in a straight line. In the video, the term is used to describe the motion of the ferrous wheel and the child on the ride, emphasizing that the speed does not change over time. This is a key factor in calculating the forces experienced by the child during the ride.
💡Centripetal Acceleration
Centripetal acceleration is the acceleration experienced by an object moving in a circular path, directed towards the center of the circle. The script discusses centripetal acceleration as a force that acts on the child while they are moving in a vertical circle, which is essential for determining the normal force exerted by the seat at different points of the ride.
💡Normal Force
Normal force is the force exerted by a surface that supports the weight of an object resting on it, acting perpendicular to the surface. In the video, the normal force is the force exerted by the seat on the child at the top and bottom of the ride. It is calculated to understand how much the child 'weighs' at these points due to the combination of gravitational force and centripetal acceleration.
💡Vertical Circle
A vertical circle refers to the circular path that an object follows in a vertical plane. The script uses this term to describe the path of the child's motion on the ride, which is crucial for understanding the forces acting on the child, especially the centripetal force that keeps them in their circular path.
💡Radius
Radius is the distance from the center of a circle to any point on its circumference. In the context of the video, the radius is given as 10 meters, which is used to calculate the centripetal acceleration and the normal force experienced by the child at different points in their circular motion.
💡Linear Speed
Linear speed, or linear velocity, is the measure of an object's speed along a straight path. The script mentions a constant linear speed of 3 meters per second for the child's motion around the vertical circle, which is used in the calculations for centripetal acceleration and normal force.
💡Coordinate System
A coordinate system is a reference frame that specifies each point in a space with a set of numerical coordinates. In the script, the instructor chooses a coordinate system with positive x and y axes to facilitate the analysis of forces acting on the child. This system helps in determining the direction and magnitude of the forces.
💡Sum of Forces
Sum of forces refers to the algebraic sum of all the forces acting on an object. In the video, the sum of forces in the y-direction is used to calculate the normal force at the bottom and top of the ride. This concept is essential for understanding how the forces balance out to create the child's circular motion.
💡Weight
Weight is the force exerted on an object due to gravity and is calculated as the product of an object's mass and the acceleration due to gravity. In the script, the child's weight is a key factor in the force equations at the bottom and top of the ride, influencing how much the child feels 'heavier' or 'lighter' at different points.
Highlights

The problem involves a ferrous wheel rotating with a constant velocity.

A child of mass m moves in a vertical circle with a radius of 10 meters and a constant speed of 3 m/s.

The task is to determine the force exerted by the seat on the child at the bottom and top of the ride.

The normal force at the bottom and top of the ride needs to be calculated.

Objects rotating in a circle experience centripetal acceleration towards the center.

A coordinate system is chosen with positive y and x directions for analysis.

At the bottom of the ride, the sum of forces in the y-direction includes the normal force and gravitational force.

The equation for the bottom of the ride is n_bot - mg = m*v^2/r.

At the top of the ride, the centripetal acceleration points downward, affecting the force equation.

The equation for the top of the ride is n_top - mg = -m*v^2/r.

The normal force is solved by rearranging the force equations for both the bottom and top.

At the bottom, n_bottom = m*v^2/r + mg.

At the top, n_top = -m*v^2/r + mg.

The normal force at the top is higher than at the bottom due to the effects of centripetal acceleration.

The child feels heavier at the bottom and lighter at the top of the ride.

The normal force is calculated to be 1.09 mg at the bottom and 0.908 mg at the top.

The force units are in Newtons as it represents the normal force exerted by the seat.

The ratio of weights at the top and bottom illustrates the effect of centripetal acceleration on perceived weight.

Transcripts

Browse More Related Video

Circular motion and apparent weight

Circular Motion: Ferris Wheel

Normal Force on Ferris Wheel

AP Physics Workbook 3.E Maximum speed over the top

Uniform Circular Motion, Example #1 Ferris wheel

AP Physics Workbook 3.H The Rotor Ride

Rate This

5.0 / 5 (0 votes)

Thanks for rating:

Related Tags
PhysicsCentripetal ForceFerris WheelWeight VariationCircular MotionAccelerationEducationalScience LessonForce AnalysisMechanical Engineering