Finding Limits Numerically with a TI-84 Calculator

Chris Ozarka

22 Jul 201704:24

EducationalLearning

32 Likes 10 Comments

TLDRThis tutorial demonstrates how to use a TI-84 or TI-83 calculator to find limits numerically. The instructor guides users through inputting a function into the calculator, using the Y= function and the table settings to get values closer to the limit. They emphasize the importance of using parentheses to ensure correct order of operations and finding patterns by inputting values incrementally closer to the target limit. The tutorial is detailed and offers practical tips for both newer and older calculator models.

Takeaways

📚 The video demonstrates how to use a TI-84 or TI-83 calculator to find limits numerically.
🔢 The user is instructed to enter the function x^3 - 1 over x - 1 into the Y1 value on the calculator.
📝 It's mentioned that the TI-84 Plus has a numerator/denominator feature, simplifying function input.
👉 If the calculator doesn't have the numerator/denominator feature, parentheses must be used to ensure correct order of operations.
📈 The user is guided to use the table feature on the calculator to test values of x close to the point of interest.
🔍 The 'ask' mode is set to allow the user to input specific x values for a more controlled approach.
⚠️ The video warns against entering values too close to the point of interest without observing a pattern, as it could lead to false conclusions.
🔄 The process involves testing values on both sides of the point of interest to find a consistent pattern.
📊 As x approaches 1 from both sides, the y values should approach the limit, which in this case is 3.
📋 For older calculators, the user must use parentheses to ensure the function is entered correctly.
❓ The video invites viewers to ask questions if they have any.

Q & A

What is the purpose of the video?
-The purpose of the video is to demonstrate how to use a TI-84 or TI-83 calculator to find limits numerically.
What is the first step in using the calculator for finding limits as shown in the video?
-The first step is to press 'Y=' on the calculator and enter the function you want to evaluate numerically.
What function does the video use as an example to demonstrate finding limits?
-The video uses the function x^3 - 1 over x - 1 as an example.
Why is it important to use parentheses when entering the function into the calculator?
-Parentheses are important to ensure the correct order of operations is followed, especially if the calculator does not automatically handle numerator and denominator as a fraction.
How does the video suggest changing the table settings to have more control over the x-values?
-The video suggests pressing '2nd' then 'Window' to change the independent part from 'auto' to 'ask', allowing the user to input specific x-values.
Why does the video recommend not using the calculator's auto x-values feature?
-The auto x-values feature does not allow for the fine control needed to get values infinitesimally close to the point where the limit is being evaluated.
What is the significance of testing both sides of the point at which the limit is being evaluated?
-Testing both sides ensures that the limit exists from the left and right, which is necessary for the limit to exist at that point.
What does the video suggest to avoid when trying to find a pattern as x approaches a certain value?
-The video suggests avoiding jumping to an infinitely close value without first observing the pattern, as this could lead to a false positive.
How does the video demonstrate finding the limit of the function as x approaches 1?
-The video demonstrates by entering values of x that are increasingly closer to 1 from both sides and observing how the y-values approach 3.
Is there a difference in the process for a TI-83 calculator compared to a TI-84?
-The only difference mentioned is in setting up the fraction; the TI-83 does not have a numerator over denominator feature and requires parentheses around the numerator and denominator.

Outlines

00:00

📚 Introduction to Numerical Limits on TI-84/83 Calculators

This paragraph introduces the process of finding limits numerically using a TI-84 or TI-83 calculator. The presenter demonstrates how to input a function, specifically (x^3 - 1) over (x - 1), into the calculator's Y= function. It emphasizes the importance of using the numerator/denominator format on the TI-84 Plus, or parentheses for older models to ensure correct order of operations. The focus is on setting up the calculator to numerically approach a limit as x gets infinitesimally close to a certain value, in this case, 1.

📉 Adjusting the Table for Numerical Limit Calculation

The second paragraph explains how to adjust the calculator's table settings to gain more control over the values of x used in the limit calculation. The default table values are replaced with user-defined values that approach the limit point more closely. The presenter guides through changing the table settings to 'ask' mode, which prompts for user input of x values. The aim is to input values that are increasingly closer to 1 to observe the behavior of the function as x approaches this limit.

🔍 Observing the Function's Behavior Near the Limit Point

In this paragraph, the presenter illustrates how to input values close to 1 into the calculator's table to observe the function's behavior as x approaches 1. The values start from 0.9 and decrease in increments to get closer to 1, revealing a pattern where the function's output approaches 3. The importance of observing the pattern rather than just plugging in a very close value is highlighted to avoid false positives and ensure a correct understanding of the limit.

🔄 Testing the Function's Behavior on Both Sides of the Limit Point

The final paragraph of the script discusses the necessity of testing the function's behavior on both sides of the limit point. After observing the function's output as x approaches 1 from the left (values less than 1), the presenter then demonstrates testing for values greater than 1 to ensure a comprehensive understanding of the limit. This includes inputting values such as 1.1, 1.01, and so on, to observe how the function's output changes and to confirm the pattern identified earlier.

Mindmap

Keywords

💡TI-84 calculator

The TI-84 calculator is a graphing calculator made by Texas Instruments, commonly used in mathematics and science education. It is known for its ability to perform complex calculations and graph functions. In the video, the TI-84 is used to demonstrate how to find limits numerically, which is a fundamental concept in calculus.

💡Numerical limits

Numerical limits refer to the process of finding the value that a function approaches as the input (or a related parameter) approaches a certain point. This concept is central to the video, as it shows how to use a calculator to approximate the limit of a function without necessarily solving it algebraically.

💡Y-values

In the context of the video, Y-values represent the output of a function. The script mentions 'Y-values' when discussing how to input a function into the calculator and how to manipulate these values to find the limit as the function approaches a certain point.

💡Function

A function in mathematics is a relation between a set of inputs and a set of permissible outputs, with the property that each input is related to exactly one output. The video script provides an example of a function, 'X to the third minus 1 all over X minus 1', which is used to demonstrate finding numerical limits.

💡Numerator and denominator

The terms 'numerator' and 'denominator' refer to the top and bottom parts of a fraction, respectively. In the script, the presenter uses these terms when explaining how to input a fractional function into the calculator, emphasizing the importance of using parentheses for correct order of operations.

💡Table

In the context of the video, a 'table' refers to a feature on the calculator that allows the user to view the values of the function for different inputs. The script describes how to use the table to input specific X values and observe the corresponding Y values to find the limit.

💡Infinitesimally

The term 'infinitesimally' is used to describe values that are extremely close to, but not equal to, a certain number. In the video, the presenter uses this term to explain how to approach the value at which the limit is being taken, by using values that are infinitesimally close to that number.

💡Pattern

A 'pattern' in this context refers to the observed behavior of the function's output as the input values approach a certain point. The script emphasizes the importance of identifying this pattern to accurately determine the limit of the function.

💡Order of operations

The 'order of operations' is a set of rules that dictate the sequence in which operations in a mathematical expression should be performed. The video script mentions the importance of using parentheses to ensure that the calculator follows the correct order of operations when inputting functions.

💡Fraction

A 'fraction' is a numerical expression that represents division, consisting of a numerator divided by a denominator. The video script discusses setting up a function as a fraction on the calculator, which is crucial for correctly inputting and evaluating functions that involve division.

💡Parentheses

Parentheses are used in mathematics to group terms or expressions together, indicating that the operations within the parentheses should be performed before those outside. The script advises using parentheses when inputting functions into the calculator to ensure the correct order of operations is followed.

Highlights

Introduction to using a TI-84 or TI-83 calculator to find limits numerically.

Accessing the Y= screen to input the first function using the numerator/denominator feature on TI-84 Plus.

For calculators without the numerator/denominator feature, using parentheses for correct order of operations.

Navigating to the table to control the values of X for finding limits.

Changing the table settings to 'ask' for user input of X values.

Starting with values close to 1 to find the limit, avoiding division by zero.

Demonstration of entering values like 0.9 to get closer to the limit.

Observing the pattern as X values approach 1 and Y values approach 3.

Testing the limit from the other side by using values greater than 1.

The importance of finding a pattern rather than just using a very close value.

Using incrementally closer X values to observe the Y values approaching 3.

The process of testing limits from both sides of the point of interest.

Clarification that the method works for both TI-84 and TI-83 calculators.

Note on setting up the function with parentheses for older calculators without the fraction feature.

Emphasis on the importance of parentheses in the numerator or denominator for correct calculations.

Invitation for viewers to ask questions if they have any.

Transcripts

Browse More Related Video

Using the TI 83/84 calculator to find a derivative

Least Squares Regression Line on the TI83 TI84 Calculator

Calculus - Finding the derivative at a point using a Ti-83 or 84 calcululator

Finding Z-score Given Area - TI-84

Find Critical Value Z for Confidence Intervals with TI-84

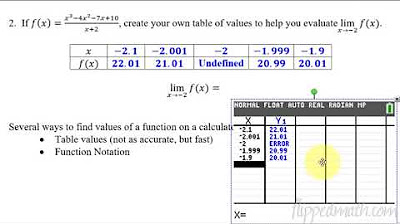
Calculus AB/BC – 1.4 Estimating Limit Values from Tables