Critical points introduction | AP Calculus AB | Khan Academy
TLDRThis video script explores the concept of maxima and minima in the context of a graphed function. It visually identifies a global maximum at a point x0, where the function's value does not exceed this peak for any x in the domain. However, no global minimum is present as the function approaches negative infinity at both ends. Local minima and maxima are also discussed, with examples provided to illustrate these points. The script then delves into the role of derivatives in identifying critical points, which are potential locations for local extrema but not necessarily minima or maxima themselves. The importance of the derivative being zero or undefined at extrema is highlighted, setting the stage for further analysis in subsequent videos.
Takeaways
- 📊 The video discusses a function depicted in yellow, with a focus on identifying its maximum and minimum values.
- 🔍 The function has a global maximum at a point x0, where f(x0) is greater than or equal to f(x) for any x in the domain.
- ⏬ The function does not have a global minimum, as it approaches negative infinity as x approaches negative and positive infinity.
- 📌 The concept of local minima and maxima is introduced, with the function having a local minimum at x1 and a local maximum at x2.
- 👀 The video suggests that local extrema can be identified by visual inspection, such as a low point for local minimum and a high point for local maximum.
- 🔄 The derivative of the function is used to further analyze the behavior at critical points, with the slope of the tangent line indicating extrema.
- 📐 At the global maximum (x0) and local minimum (x1), the derivative f'(x) is zero, indicating a horizontal tangent line.
- 🚫 At the local maximum (x2), the derivative f'(x2) is undefined due to the function's behavior, suggesting a sharp turn or cusp.
- 🤔 The video raises the question of whether all critical points (where the derivative is zero or undefined) are extrema, which is not necessarily the case.
- 🔑 Critical points are identified as places where the derivative is zero or undefined, which can help in finding potential extrema.
- 🚀 The video concludes by setting up the next discussion on how to differentiate between a minimum or maximum at a critical point.
Q & A
What is the definition of a global maximum for a function?
-A global maximum is a point on the graph of a function where the function's value is greater than or equal to its value at any other point in the domain. It is the highest point on the graph.
How can you determine if a function has a global minimum?
-A function has a global minimum if there exists a point where the function's value is less than or equal to its value at any other point in the domain. However, if the function can take on arbitrarily negative values, as it approaches negative or positive infinity, then there is no global minimum.
What is a local minimum and how can it be identified on a graph?
-A local minimum is a point on the graph where the function's value is lower than the values at points immediately surrounding it. It can be identified by observing a 'dip' or 'low point' in the graph where the function's value is less than the values of the function in a region around that point.
What does it mean for a function to have a local maximum?
-A local maximum is a point on the graph where the function's value is higher than the values at points immediately surrounding it. It can be recognized by a 'peak' or 'high point' in the graph where the function's value is greater than the values of the function in a neighborhood around that point.
Why can't we consider the endpoints of an interval as local minima or maxima?
-Endpoints of an interval are not considered local minima or maxima because the definition of a local extremum requires comparison with nearby points within the interval. At the endpoints, there are no points 'nearby' to compare with, so they do not meet the criteria for being local extrema.
What is the significance of the derivative of a function at a point?
-The derivative of a function at a point gives the slope of the tangent line to the graph of the function at that point. It indicates the rate of change of the function and can be used to determine whether the function is increasing or decreasing at that point.
How does the derivative help in identifying critical points of a function?
-Critical points are identified where the derivative of the function is either zero or undefined. These points are potential candidates for local minima, local maxima, or points of inflection, and they are where the function changes its direction of motion.
What is the relationship between a critical point and an extremum (minimum or maximum)?
-A critical point is a necessary condition for an extremum, but not a sufficient one. All non-endpoint minima and maxima are critical points, but not all critical points are minima or maxima. A critical point where the derivative is zero or undefined needs further analysis to determine if it is indeed a minimum or maximum.
Why is it not enough to conclude that a critical point is a minimum or maximum without further analysis?
-A critical point is simply where the derivative is zero or undefined. However, this does not guarantee that the point is a minimum or maximum. Further analysis, such as using the second derivative test or examining the behavior of the function around the critical point, is required to confirm whether it is indeed an extremum.
What is the purpose of visualizing the tangent line at a point on the graph?
-Visualizing the tangent line helps in understanding the slope of the function at that specific point. It provides an intuitive way to see whether the function is increasing or decreasing and can assist in identifying critical points where the slope is zero or undefined.
How does the behavior of the function as x approaches negative or positive infinity affect the existence of a global minimum?
-If the function approaches negative infinity as x approaches negative or positive infinity, it means that the function can take on any arbitrarily negative value. In such a case, there is no global minimum because there is no lowest point that the function cannot go below.
Outlines
📈 Understanding Global Maximum and Local Minima
The paragraph discusses the concept of a function's maximum and minimum values. It introduces the idea of a global maximum, which is a point where the function's value is the highest within its domain. The video uses a visual representation to identify such a point, referred to as x0, where the function's value is greater than or equal to the value of the function at any other point in the domain. The paragraph also explains that the function does not have a global minimum as it can take on arbitrarily negative values, approaching negative infinity at both ends of the domain. Furthermore, it explores the notion of local minima and maxima, which are points where the function's value is lower or higher, respectively, than the values at points in a region around them. The paragraph identifies a local minimum at x1 and a local maximum at x2, using a non-rigorous, visual approach to recognize these points. It concludes by differentiating between endpoints and points within an interval for extrema analysis.
🔍 Critical Points and Their Relation to Minima and Maxima
This paragraph delves into the derivative of a function to identify critical points, which are essential for finding minima and maxima. It explains that at points where a function has a minimum or maximum (excluding endpoints), the derivative is either zero or undefined. The paragraph uses the function's tangent line at various points to illustrate this concept, showing that at a global maximum (x0) and a local minimum (x1), the derivative is zero, while at a local maximum (x2), the derivative is undefined. The term 'critical points' is introduced to describe points where the derivative is zero or undefined. The paragraph clarifies that while every non-endpoint minimum or maximum is a critical point, not every critical point is necessarily a minimum or maximum. It uses the example of x3, a critical point with a derivative of zero, which is neither a minimum nor a maximum. The discussion sets the stage for further analysis in subsequent videos to determine whether a critical point corresponds to a minimum or maximum.
Mindmap
Keywords
💡Function
💡Global Maximum
💡Global Minimum
💡Local Minimum
💡Local Maximum
💡Derivative
💡Critical Point
💡Tangent Line
💡Slope
💡Extrema
💡Endpoint
Highlights
The function graphed in yellow is analyzed for its maximum and minimum values.
Assumption that the function decreases indefinitely as x approaches negative and positive infinity.
Identification of a global maximum at point x0 where f(x0) is greater than or equal to f(x) for any x in the domain.
Observation that there is no global minimum as the function approaches negative infinity.
Discussion on the concept of local minima and maxima and their identification on the graph.
Identification of a local minimum at point x1 where f(x1) is less than f(x) in the surrounding region.
Observation that there are no other local minima visible on the graph.
Identification of a local maximum at point x2 where f(x2) is greater than f(x) in the neighborhood around x2.
Introduction of the concept of critical points where the derivative is either zero or undefined.
Explanation that critical points at non-endpoints of an interval are potential minima or maxima.
Illustration of how the derivative at a global maximum (x0) is zero, indicating a horizontal tangent.
Illustration of how the derivative at a local minimum (x1) is also zero, indicating another horizontal tangent.
Identification of a critical point at x2 where the derivative is undefined due to the slope's discontinuity.
Introduction of the concept that not all critical points are minima or maxima, using point x3 as an example.
Teaser for the next video which will differentiate between types of critical points to determine if they are minima or maxima.
Transcripts
Browse More Related Video

Finding Relative Extrema
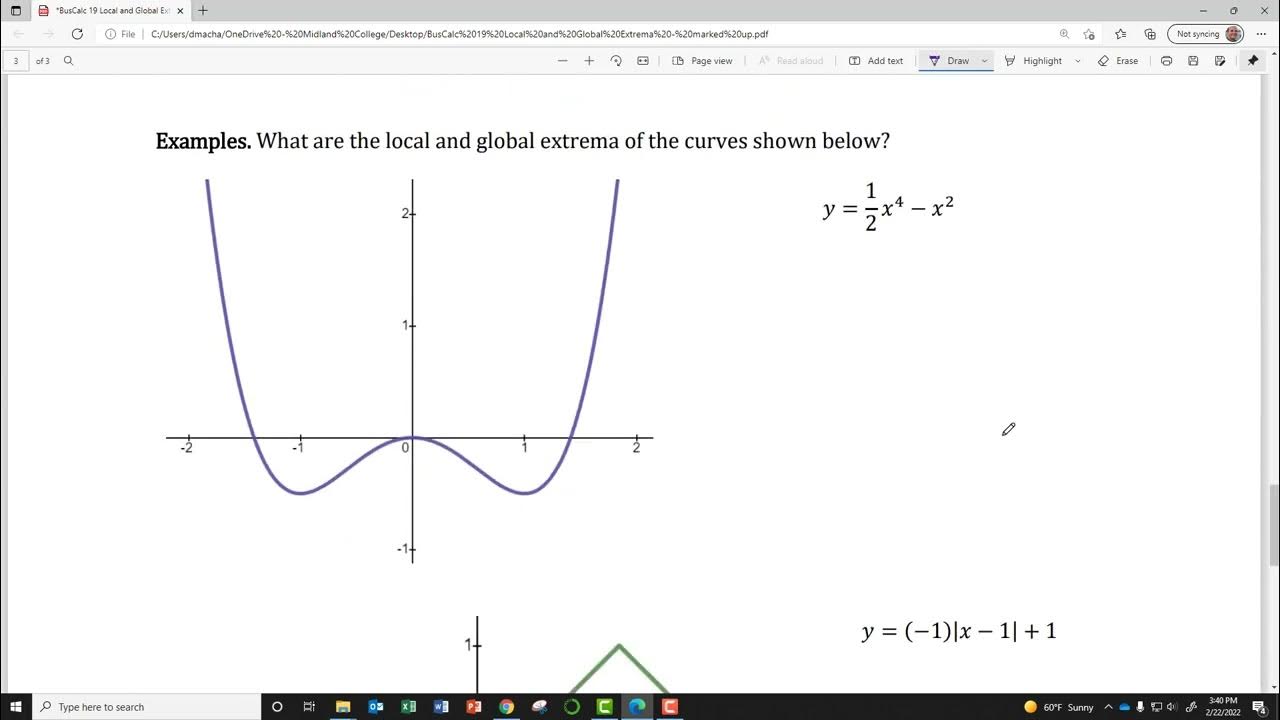
BusCalc 19 Local and Global Extrema
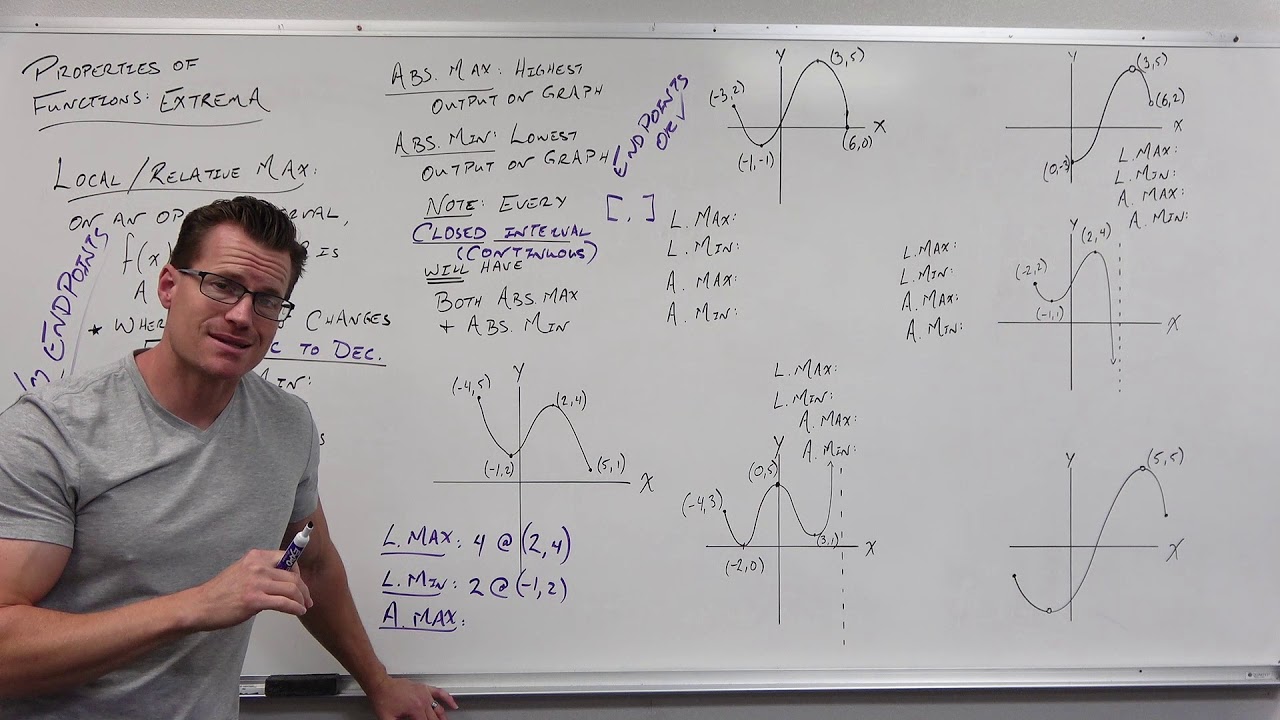
Properties of Functions - Extrema (Precalculus - College Algebra 10)

Extrema on an Interval

How to recognize relative and absolute maxima and minima | Functions | Algebra I | Khan Academy

Concavity introduction | Using derivatives to analyze functions | AP Calculus AB | Khan Academy
5.0 / 5 (0 votes)
Thanks for rating: