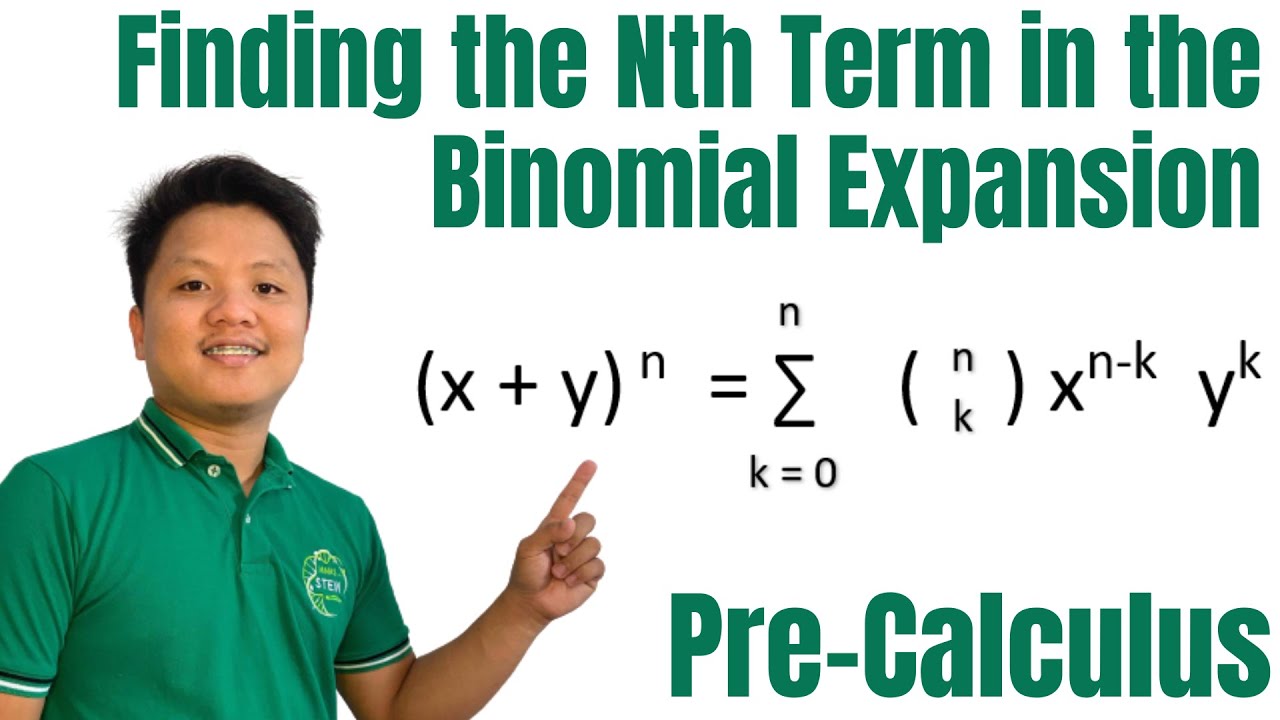
FINDING THE NTH TERM IN THE BINOMIAL EXPANSION || PRE-CALCULUS

WOW MATH
20 Nov 202106:18
EducationalLearning
32 Likes 10 Comments

TLDRThis video lesson teaches viewers how to find a specific term in the binomial expansion using a formula. The instructor demonstrates how to calculate the nth term by using the binomial coefficient and powers of variables 'a' and 'b'. Two examples are provided: finding the fifth term of (x + 2)^8, resulting in 1120x^4, and the third term of (3x - 4)^6, yielding 19440x^4. The lesson aims to help learners understand the process of binomial expansion and apply it to solve problems.

Takeaways
  • πŸ“š The video lesson is about finding the nth term in the binomial expansion.
  • πŸ” The formula for the nth term in the binomial expansion is given as \( \binom{n}{r} \cdot a^{n-r+1} \cdot b^{r-1} \).
  • πŸ“˜ An example is provided to find the fifth term of the expansion of \( (x + 2)^8 \).
  • πŸ”’ The value of n is 5, a is x, and b is 2 for the first example.
  • πŸŽ“ The process involves substituting values into the formula and simplifying the expression.
  • πŸ“ˆ The result for the first example is \( 70 \cdot x^4 \cdot 2^4 \), which simplifies to \( 1120x^4 \).
  • πŸ“ Another example is given to find the third term of the expansion of \( (3x - 4)^6 \).
  • πŸ”‘ The values for the second example are n as 6, a as 3x, and b as -4.
  • 🧩 The formula is applied again, and the term is simplified to \( 19440x^4 \).
  • πŸ“Š The final answer for the second example is \( 19440x^4 \), which is the third term of the given expansion.
  • πŸ‘ The video encourages viewers to like, subscribe, and hit the bell for more tutorial videos.
Q & A

  • What is the main topic of the video lesson?

    -The main topic of the video lesson is finding the nth term in the binomial expansion.

  • What is the general formula for the nth term in the binomial expansion?

    -The general formula for the nth term in the binomial expansion is \( \binom{n}{r} \cdot a^{n-r} \cdot b^{r} \), where \( \binom{n}{r} \) is the binomial coefficient, \( a \) and \( b \) are the terms in the binomial, and \( r \) is the term number minus one.

  • What is the binomial quantity used in the first example of the video?

    -In the first example, the binomial quantity used is \( x + 2 \) raised to the power of 8.

  • How many terms are there in the binomial expansion of the first example?

    -In the first example, the binomial expansion has 9 terms because it is raised to the power of 8.

  • What is the fifth term of the expansion of \( x + 2 \)^8 according to the formula?

    -The fifth term of the expansion of \( x + 2 \)^8 is \( 70 \cdot x^4 \cdot 2^4 \), which simplifies to \( 70 \cdot 16 \cdot x^4 \) or \( 1120x^4 \).

  • In the second example, what is the binomial quantity being expanded?

    -In the second example, the binomial quantity being expanded is \( 3x - 4 \) raised to the power of 6.

  • What is the third term of the expansion of \( (3x - 4) \)^6?

    -The third term of the expansion of \( (3x - 4) \)^6 is \( 15 \cdot 81x^4 \cdot 16 \), which simplifies to \( 19440x^4 \).

  • What is the significance of the binomial coefficient in the nth term formula?

    -The binomial coefficient \( \binom{n}{r} \) in the nth term formula determines the number of ways to choose 'r' elements from a set of 'n' elements, and it is crucial for calculating the nth term of a binomial expansion.

  • What is the purpose of the binomial expansion in mathematics?

    -The purpose of the binomial expansion in mathematics is to provide a way to expand expressions of the form \( (a + b)^n \) into a sum involving terms of \( a \) and \( b \) raised to various powers, which is useful for simplifying complex expressions and solving equations.

  • How can one find the nth term of a binomial expansion without using the formula?

    -Without using the formula, one can find the nth term of a binomial expansion by systematically applying the binomial theorem, which involves calculating each term based on the powers of \( a \) and \( b \) and their respective coefficients.

  • What is the relationship between the term number and the binomial coefficient in the nth term formula?

    -The term number in the nth term formula is directly related to the binomial coefficient as it determines the specific combination of \( a \) and \( b \) being raised to certain powers. The term number minus one gives the value of \( r \) used in the binomial coefficient calculation.

Outlines
00:00
πŸ“š Understanding Binomial Expansion Terms

This paragraph introduces the concept of finding the nth term in a binomial expansion. The speaker, Mark Awamat, explains the general formula for the nth term, which involves the binomial coefficient 'n choose r', and the powers of 'a' and 'b'. The formula is demonstrated with an example where the fifth term of (x + 2)^8 is calculated. The process involves substituting the appropriate values into the formula and simplifying to find the term. The example concludes with the calculation of 1120x^4, showcasing the application of the binomial theorem.

05:02
πŸ” Calculating Specific Terms in Binomial Expansion

The second paragraph delves into calculating specific terms of a binomial expansion, using the expansion of (3x - 4)^6 as an example to find the third term. The speaker identifies the values needed for 'n', 'r', 'a', and 'b' and applies the binomial formula. The calculation involves determining the binomial coefficient, raising 'a' and 'b' to the appropriate powers, and simplifying the expression. The result is 19440x^4, illustrating the detailed process of extracting a specific term from a binomial expansion.

Mindmap
Keywords
πŸ’‘Binomial Expansion
Binomial Expansion is a mathematical expression that represents the expansion of powers of a binomial, which is an algebraic expression with two terms. In the video, the focus is on finding the nth term in a binomial expansion, which is a fundamental concept in algebra and combinatorics. The script provides an example of finding the fifth term of the expansion of (x + 2)^8, demonstrating how to apply the binomial theorem.
πŸ’‘nth Term
The nth term refers to a specific term within a sequence or series, where 'n' denotes the position of the term. In the context of the video, finding the nth term in a binomial expansion is the primary objective. The script illustrates this by showing how to calculate the fifth term of the expansion of (x + 2)^8, which involves using the binomial formula.
πŸ’‘Binomial Formula
The Binomial Formula is a mathematical formula used to expand the powers of a binomial expression. It is represented as (a + b)^n = Ξ£ (n choose k) * a^(n-k) * b^k, where 'n choose k' is a binomial coefficient. The video discusses the application of this formula to find the nth term of a binomial expansion, as seen when calculating the fifth term of (x + 2)^8.
πŸ’‘Combination Formula
The Combination Formula is used to calculate the number of ways to choose 'k' items from a set of 'n' items without regard to the order of selection. It is represented as 'n choose k' or C(n, k) = n! / (k!(n-k)!). In the video, the combination formula is used within the binomial formula to calculate the coefficients of the terms in the expansion.
πŸ’‘Algebraic Expression
An algebraic expression is a combination of variables, constants, and operation symbols (like addition, subtraction, multiplication, and division) that represent a quantity. In the video, the algebraic expressions are binomials raised to a power, such as (x + 2)^8 and (3x - 4)^6, which are then expanded using the binomial theorem.
πŸ’‘Powers of a Binomial
Powers of a binomial refer to the result of raising a binomial to a positive integer power. In the video, the script discusses the expansion of (x + 2) raised to the power of 8 and (3x - 4) raised to the power of 6, which are examples of finding the powers of a binomial.
πŸ’‘Coefficient
In the context of a binomial expansion, a coefficient is a numerical factor that multiplies the variables in a term of the expansion. The video demonstrates how to calculate coefficients using the combination formula within the binomial formula, as seen when determining the fifth term of (x + 2)^8.
πŸ’‘Variable
A variable is a symbol that represents an unknown quantity in mathematics. In the video, 'x' is used as a variable in the binomial expressions (x + 2) and (3x - 4). The script shows how the variable 'x' is raised to different powers and how its terms are found within the expansion.
πŸ’‘Constant
A constant is a value that does not change within a given context. In the video, the constants '2' and '-4' are used in the binomial expressions (x + 2) and (3x - 4), respectively. The constants are raised to different powers during the expansion process.
πŸ’‘Substitution
Substitution is a method used in algebra where one value or expression is replaced by another. In the video, substitution is used to insert the values of 'n', 'r', 'a', and 'b' into the binomial formula to find the specific terms of the expansion, such as when calculating the fifth term of (x + 2)^8.
πŸ’‘Simplify
Simplifying is the process of making a mathematical expression more straightforward by reducing it to its simplest form. In the video, the script involves simplifying expressions after applying the binomial formula, such as simplifying (2^4) and (8 - 5 + 1) to find the final term of the expansion.
Highlights

Introduction to the binomial expansion and the formula for finding the nth term.

Explanation of the general formula for the nth term in a binomial expansion: \((a + b)^n\).

The specific formula for the nth term: \(\binom{n}{r} a^{n-r+1} b^{r-1}\).

An example to find the fifth term of the expansion of \((x + 2)^8\).

Step-by-step calculation for the fifth term using the given formula.

Substitution of values into the formula to find the fifth term.

Simplification of the expression to find the final answer for the fifth term.

Result of the fifth term calculation: \(1120x^4\).

Another example to determine the third term of the expansion of \((3x - 4)^6\).

Identification of values for \(a\), \(b\), \(n\), and \(r\) for the second example.

Application of the nth term formula to find the third term of the second example.

Calculation of the binomial coefficient and powers for the third term.

Final result of the third term calculation: \(19440x^4\).

Summary of the process to find the nth term in a binomial expansion.

Encouragement to like, subscribe, and hit the bell button for more video tutorials.

Closing remarks and sign-off from the presenter.

Transcripts

Browse More Related Video

Finding the Nth Term in the Binomial Expansion | Binomial Theorem | Pre-Calculus

BINOMIAL THEOREM || PRE-CALCULUS

Binomial Coefficients | General Formula

Binomial theorem combinatorics connection | Algebra II | Khan Academy

Calculus Divide and Integrate

Solving Quadratics by Factoring

Rate This

5.0 / 5 (0 votes)

Thanks for rating:

Related Tags
Binomial ExpansionMath TutorialEducational ContentAlgebra LessonsCombination FormulaMathematics EducationProblem SolvingVideo LessonLearning ResourceMathematics Tips