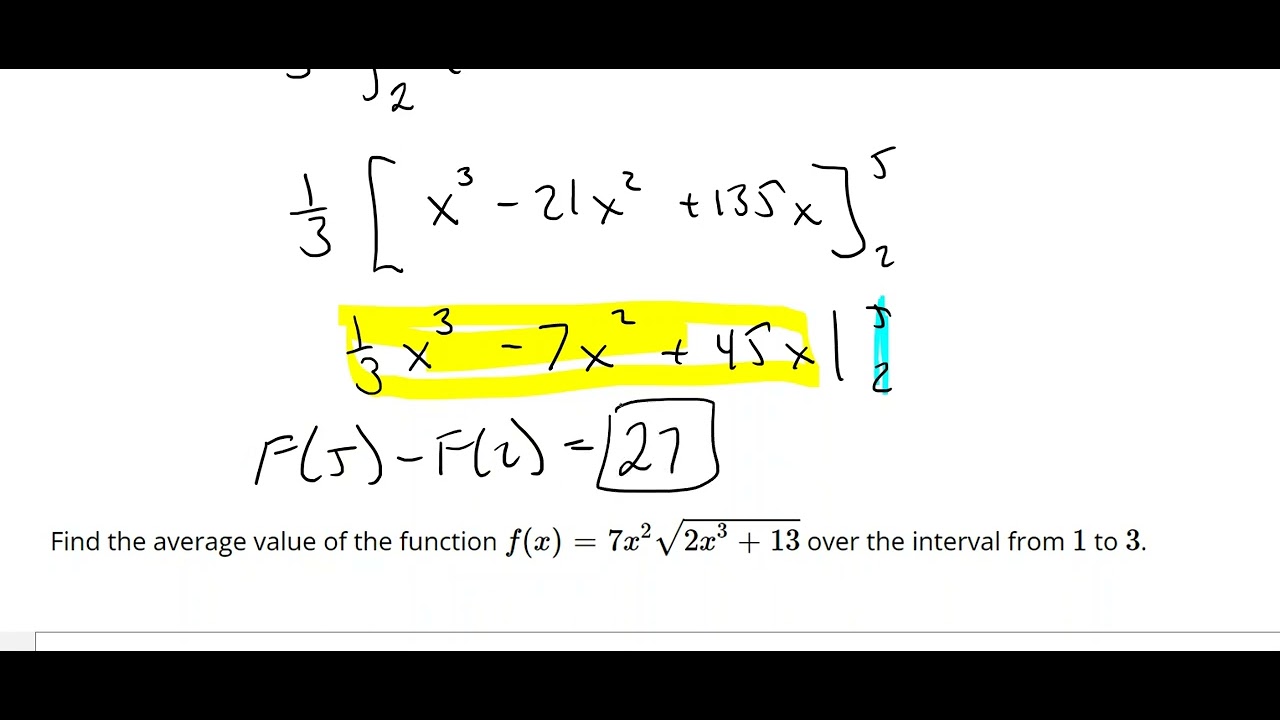
Calculus Divide and Integrate

turksvids
20 Feb 201705:48
EducationalLearning
32 Likes 10 Comments

TLDRThe video script discusses the common mathematical technique of 'divide first, then integrate' for solving integrals where the numerator's degree is greater than or equal to the denominator's degree. The presenter dives into three examples to illustrate this method. In the first example, the integral of (x^2 + 3x + 2) over (x + 3) is simplified through long division, resulting in (x + 2) over (x + 3), which is then integrated to yield (1/2)x^2 + 2x + ln|x + 3| + C. The second example involves the integral of (2x^2 - 5x + 1) over (x - 4), which after division and integration results in x^2 + 3x + 13 + ln|x - 4| + C. The third example demonstrates the integral of (x^2) over (x^2 + 4), where the presenter uses a trick of adding and subtracting the same term to simplify the integrand before integrating to get x - 2arctan(x/2) + C. The video emphasizes the importance of practice and understanding the technique, which is often followed by natural logarithm or arctangent results in the remainder term.

Takeaways
  • 📚 **Divide First, Integrate Later**: When the degree of the numerator in an integral is greater than or equal to the degree of the denominator, it's necessary to perform polynomial division before integrating.
  • 🔢 **Long Division Technique**: The video demonstrates the use of long division to simplify the integrand, which is a common method for dealing with polynomials.
  • 🛠️ **Matching Degrees**: The process of long division involves multiplying the denominator by a term that matches the degree of the numerator to facilitate the division.
  • 📉 **Cancelling Terms**: During the division, like terms in the numerator and denominator are cancelled out, simplifying the integrand.
  • 🧮 **Rewriting the Integrand**: After division, the original integrand can be rewritten in a simpler form that is easier to integrate.
  • 📈 **Integration of Resultant Terms**: Once the integrand is simplified, each term can be integrated separately, often resulting in terms like natural logarithms or arcs.
  • 🔁 **Synthetic Division Alternative**: Although synthetic division could be used for linear denominators, the video prefers long division for its clarity.
  • 📝 **Natural Log and Arc Terms**: Common outcomes of integration after division are natural logarithms and arcs, which are standard forms in calculus.
  • 🤔 **Experience Matters**: The level of experience with calculus can affect the approach to rewriting and integrating, with more experienced individuals potentially recognizing patterns more quickly.
  • ➗ **Division by Constants**: If the denominator is a constant multiple of the numerator, dividing by that constant simplifies the integrand, as shown in the example with \( x^2 + 4 \).
  • 🎓 **Plus-Minus Trick**: The video introduces a trick for rewriting the integrand by adding and subtracting the same term to match the denominator, which can simplify the integration process.
Q & A

  • What is the technique discussed in the video?

    -The video discusses the technique of dividing first and then integrating when dealing with integrals where the degree of the numerator is greater than or equal to the degree of the denominator.

  • Why is long division used in the examples?

    -Long division is used to simplify the integrand by dividing the numerator by the denominator, which helps in breaking down the integral into simpler parts that can be more easily integrated.

  • What is the first integral example given in the video?

    -The first integral example is ∫(x^2 + 3x + 2) / (x + 3) dx, which after performing long division, simplifies to ∫(x + 2) / (x + 3) dx.

  • What is the result of integrating the simplified form of the first example?

    -The result of integrating the simplified form is (1/2)x^2 + 2x + ln|x + 3| + C.

  • What is the second integral example provided in the video?

    -The second integral example is ∫(2x^2 - 5x + 1) / (x - 4) dx, which after long division, simplifies to ∫(2x + 3 + 13) / (x - 4) dx.

  • How does the video handle the second integral example?

    -The video rewrites the integrand as 2x + 3 + 13 / (x - 4) and then integrates each part, resulting in x^2 + 3x + ln|x - 4| + C.

  • What is the third integral example discussed in the video?

    -The third integral example is ∫(x^2) / (x^2 + 4) dx, which even though the degrees are the same, the video still performs division to simplify the integral.

  • What trick does the video mention for simplifying the third integral example?

    -The video mentions a 'plus 4 minus 4' trick, which involves adding and subtracting the same term to match the denominator in the numerator, thus simplifying the integral.

  • What is the final simplified form of the third integral example after applying the trick?

    -The final simplified form after applying the trick is ∫(1 - 4 / (x^2 + 4)) dx, which integrates to x - (1/2)arctan(x/2) + C.

  • What are the two common outcomes mentioned when dealing with the remainder term after division in integration?

    -The two common outcomes mentioned are ending up with a natural logarithm (ln) or an arctangent (arctan) function in the integral.

  • Why is it recommended to practice the 'plus 4 minus 4' trick?

    -Practicing the 'plus 4 minus 4' trick helps in efficiently simplifying the integrand by matching the denominator in the numerator without changing the value of the integral, which can often simplify the integration process.

  • What is the purpose of using placeholders in the long division process as mentioned in the video?

    -Using placeholders in the long division process helps to keep track of the steps and prevents errors, especially when dealing with more complex polynomial divisions.

Outlines
00:00
📚 Integrating by Division: A Common Technique

This paragraph introduces a mathematical technique for integrating functions where the degree of the numerator is greater than or equal to the degree of the denominator. The speaker demonstrates the process through an example involving the integral of ( x^2 + 3x + 2 ) over ( x + 3 ). The method involves performing long division to simplify the integrand, which then allows for easier integration. The result of the integration is expressed as ( 1/2x^2 + 2x + ln|x + 3| + C ), where ( C ) is the constant of integration.

05:03
🔍 More Division and Integration Examples

The second paragraph continues the discussion on integrating by division with two additional examples. The first example involves the integral of ( 2x^2 - 5x + 1 ) over ( x - 4 ), which after division and integration results in ( 2x + 3 + 13ln|x - 4| + C ). The second example deals with the integral of ( x^2 ) over ( x^2 + 4 ), which after applying a strategic addition and subtraction of terms to simplify the integrand, leads to the result ( x - 2 + 1/2arctan(x/2) + C ). The speaker also mentions a common trick called 'plus 1 minus 1' for simplifying the integrand, which is a useful technique for integrands that match the denominator.

Mindmap
Keywords
💡Divide first and then integrate
This is a mathematical technique used when the degree of the numerator in a rational function is greater than or equal to the degree of the denominator. The process involves performing polynomial long division to simplify the integrand before integrating. In the video, this technique is applied to several integrals to demonstrate how it simplifies the integration process.
💡Degree of a polynomial
The degree of a polynomial is the highest power of the variable in the polynomial. It is a crucial factor in determining whether to use the 'divide first and then integrate' technique. The video emphasizes that if the degree of the numerator is at least the same as the degree of the denominator, one should perform division before integrating.
💡Long division
Long division is a method used to divide one polynomial by another. It is analogous to the long division technique used with numbers and is demonstrated in the video to simplify the integrands of the given integrals. The process involves multiplying the divisor by a polynomial that will cancel out the highest degree term of the dividend, then subtracting and bringing down the next term, and repeating the process.
💡Integrand
An integrand is the function that is to be integrated in an integral. In the context of the video, after performing long division, the integrand is simplified, making the integration process more straightforward. The video shows how the original integrands are transformed into simpler forms that can be easily integrated.
💡Natural logarithm
The natural logarithm, often denoted as ln(x), is the logarithm to the base e. It is a common result when integrating functions of the form 1/x. In the video, the natural logarithm appears as part of the integrated result for the integrals involving 1/(x + 3) and 1/(x - 4), illustrating a typical outcome when integrating such functions.
💡Arctan function
The arctan function, or inverse tangent function, is the function that reverses the tangent function. It is encountered in the video when integrating the expression 4/(x^2 + 4), which after a substitution resembles the form of 1/(1 + x^2), a standard integral for which the antiderivative is arctan(x).
💡Synthetic division
Synthetic division is an alternative method to long division for dividing polynomials. It is mentioned in the video as a possible method for the division step but is not demonstrated. The video prefers long division for its illustrative purposes, although synthetic division can be quicker, especially for polynomials with a linear term in the denominator.
💡Polynomial
A polynomial is an algebraic expression consisting of variables and coefficients, involving only the operations of addition, subtraction, and multiplication, and non-negative integer exponents of variables. In the video, polynomials are the main objects being manipulated, specifically through long division, to facilitate the integration process.
💡Antiderivatives
Antiderivatives, also known as indefinite integrals, are functions whose derivative is equal to the given function. The process of finding antiderivatives is called integration. The video is centered around finding antiderivatives of various polynomial functions using the divide first and then integrate technique.
💡Integration
Integration is a fundamental operation in calculus, the inverse of differentiation, which is used to find the area under a curve or to accumulate quantities. In the video, after simplifying the integrand through division, the integration step is performed to find the antiderivative of the simplified expression.
💡Plus-minus trick
The plus-minus trick, also known as adding and subtracting, is a technique used to manipulate the numerator of a fraction to make it easier to integrate. In the video, this trick is demonstrated by adding and subtracting the same term (in this case, 4) to the numerator to create a term that matches the denominator, thus simplifying the integral.
Highlights

Discussed a common technique of dividing first and then integrating for polynomial functions.

Demonstrated the process using the integral of x squared plus 3x plus 2 over x plus 3 dx.

Performed actual long division to simplify the integrand before integrating.

Resulted in the integrand being X plus 2 over X plus 3 dx after division.

Integrated each part of the simplified integrand to get 1/2 x squared plus 2 and natural log of the absolute value of x plus 3 plus C.

Moved on to another example with the integral of 2x squared minus 5x plus 1 over x minus 4.

Highlighted the preference for long division despite the linear term in the denominator.

Revealed the common outcome of natural log or arc functions after division.

Integrated the simplified integrand to obtain x squared plus 3x plus 13 and natural log of the absolute value of x minus 4 plus C.

Tried a third example with the integral of x squared over x squared plus 4 dx, where the degrees are the same.

Used placeholders for clarity during the division process.

Simplified the integrand to 1 minus 4 over x squared plus 4 dx.

Integrated to get x plus 1/2 arctan of x over 2 plus C.

Suggested a simplification of the answer to x minus 2 or 10 of x over 2 plus C.

Introduced a trick called 'plus 4 minus 4' to match the denominator in the numerator.

Explained that adding and subtracting the same term does not change the value but simplifies the integrand.

Encouraged practice of the 'plus 4 minus 4' trick for efficiency in integration problems.

Concluded with a summary of the method and wished good luck to the viewers.

Transcripts

Browse More Related Video

Integration Technique - The Plus 1, Minus 1 Trick

Average Value of a Function

Integration by Substitution

STANDARD EQUATION OF PARABOLA TO ITS GENERAL FORM

Long Division With Polynomials - The Easy Way!

Area Between Curves: y = cos(pi*x), y = 4x^2 -1

Rate This

5.0 / 5 (0 votes)

Thanks for rating:

Related Tags
Calculus TechniquesIntegral DivisionMathematical ExamplesLong DivisionSynthetic DivisionNatural LogarithmArctan FunctionIntegration TricksMath EducationProblem SolvingAlgebraic Simplification