Binomial Coefficients | General Formula

Tambuwal Maths Class
26 Apr 202208:29
EducationalLearning
32 Likes 10 Comments

TLDRThis tutorial explains how to find the coefficients of specific terms in binomial expansions using the general formula. It covers three problems, demonstrating how to calculate coefficients for terms like 'a^3b', 'y^3', and 'x^2y^4' in respective binomial expansions, providing step-by-step simplifications and final results.

Takeaways
  • 😀 The video tutorial focuses on finding coefficients in binomial expansions using the general formula.
  • 🔍 The general formula for binomial expansion is \( n \choose r \) \( a^{n-r} \) \( b^r \), where \( n \) is the power, \( r \) is the power of \( b \), and \( a \) and \( b \) are the terms in the binomial.
  • 📚 The first problem involves finding the coefficient of \( a b^3 \) in the expansion of \( (a + b)^4 \), which is calculated using \( 4 \choose 3 \).
  • 🧩 In the first problem, the coefficient is found to be 4, derived from simplifying the binomial coefficient and the powers of \( a \) and \( b \).
  • 🔢 The second problem seeks the coefficient of \( y^3 \) in the expansion of \( (2y - 3)^5 \), where \( n = 5 \) and \( r = 2 \).
  • 📉 The coefficient of \( y^3 \) is calculated to be 720, after simplifying the expression involving factorials and powers.
  • 📚 The third problem is about finding the coefficient of \( x^2 y^4 \) in the expansion of \( (3x + 2y)^6 \), with \( n = 6 \) and \( r = 4 \).
  • 🔍 The coefficient for \( x^2 y^4 \) is determined to be 2160, following the steps of simplifying the binomial coefficient and powers.
  • 💡 The tutorial emphasizes the importance of understanding the relationship between \( n \), \( r \), and the powers of \( a \) and \( b \) in binomial expansions.
  • 🌟 The video concludes with an encouragement to share the learning and subscribe to the channel for more educational content.
Q & A

  • What is the general formula used to find the nth term of a binomial expansion?

    -The general formula used to find the nth term of a binomial expansion is \( \binom{n}{r} a^{n-r} b^r \), where \( n \) is the power of the binomial, \( r \) is the exponent of \( b \), and \( \binom{n}{r} \) is the binomial coefficient.

  • What is the coefficient of \( a^b \) to the third power in the expansion of \( (a + b)^4 \)?

    -The coefficient of \( a^b \) to the third power in the expansion of \( (a + b)^4 \) is 4. This is calculated using the formula \( \binom{4}{3} \), which simplifies to \( \frac{4!}{(4-3)! \cdot 3!} = 4 \).

  • How do you determine the value of \( r \) in the binomial expansion formula?

    -In the binomial expansion formula, \( r \) is determined by the exponent of \( b \). It is always equivalent to the power of \( b \) in the term you are looking for.

  • What is the coefficient of \( y^3 \) in the expansion of \( (2y - 3)^5 \)?

    -The coefficient of \( y^3 \) in the expansion of \( (2y - 3)^5 \) is 720. This is calculated using the formula \( \binom{5}{2} \cdot 2^3 \cdot (-3)^2 \), which simplifies to \( 5 \cdot 8 \cdot 9 = 720 \).

  • How do you find the coefficient of \( x^2 y^4 \) in the expansion of \( (3x + 2y)^6 \)?

    -The coefficient of \( x^2 y^4 \) in the expansion of \( (3x + 2y)^6 \) is 2160. This is calculated using the formula \( \binom{6}{4} \cdot 3^2 \cdot 2^4 \), which simplifies to \( \frac{6!}{(6-4)! \cdot 4!} \cdot 9 \cdot 16 = 2160 \).

  • What is the significance of the sum of the powers in a binomial expansion being equal to \( n \)?

    -The sum of the powers in a binomial expansion being equal to \( n \) ensures that the terms of the expansion correctly represent the original binomial raised to the power \( n \). It helps in identifying the correct terms and their coefficients in the expansion.

  • Why is it necessary to use the coefficients of \( a \) and \( b \) when finding a specific term's coefficient in a binomial expansion?

    -Using the coefficients of \( a \) and \( b \) is necessary because they affect the value of the term in the expansion. They are part of the general formula and contribute to the calculation of the binomial coefficient and the overall term's value.

  • How does the binomial coefficient \( \binom{n}{r} \) relate to the terms in a binomial expansion?

    -The binomial coefficient \( \binom{n}{r} \) determines the number of ways to choose \( r \) items from \( n \) items, and in the context of binomial expansions, it represents the coefficient of the term where \( b \) is raised to the power \( r \).

  • What is the role of factorials in the calculation of binomial coefficients?

    -Factorials are used in the calculation of binomial coefficients to simplify the expression. They help in reducing the calculation to a more manageable form by canceling out common factors in the numerator and the denominator.

  • Can the process of finding coefficients in binomial expansions be applied to other algebraic expressions?

    -Yes, the process of finding coefficients in binomial expansions can be applied to other algebraic expressions that involve powers and combinations, as the underlying principles of combinatorics and algebraic manipulation are universal.

Outlines
00:00
📚 Introduction to Binomial Expansion Coefficients

In this introductory segment, the tutorial begins by recapping the previous lesson on finding the nth term of binomial expansions using the general formula. The focus of this tutorial is to learn how to find specific coefficients using the same general formula. Three problems will be tackled to illustrate this concept, starting with finding the coefficient of ab^3 in the expansion of (a + b)^4. The general formula is outlined, emphasizing that the power of b is equivalent to r. The first problem's solution is then initiated, highlighting that r = 3 and demonstrating the steps to find the coefficient.

05:01
🔢 Solving the First Problem: Coefficient of ab^3 in (a + b)^4

The tutorial solves the first problem: finding the coefficient of ab^3 in the expansion of (a + b)^4. Using the formula nCr · a^(n-r) · b^r, where n = 4 and r = 3, it calculates 4C3 as 4. Detailed steps involve factorial calculations and simplifications, ultimately showing that the coefficient is 4.

🔢 Solving the Second Problem: Coefficient of y^3 in (2y - 3)^5

This section addresses the second problem: finding the coefficient of y^3 in the expansion of (2y - 3)^5. Here, n = 5 and r = 2 (since y has a power of 3 and b has a power of 2). The general formula nCr · a^(n-r) · b^r is applied, with specific values substituted. Detailed calculations show that the coefficient of y^3 is 720, achieved through factorial and power simplifications.

🔢 Solving the Third Problem: Coefficient of x^2 y^4 in (3x + 2y)^6

The tutorial solves the third and final problem: finding the coefficient of x^2 y^4 in the expansion of (3x + 2y)^6. With n = 6 and r = 4, the general formula is used again. The calculation involves 6C4, powers of the coefficients 3 and 2, and factorial simplifications. After detailed steps, the coefficient is found to be 2160. The tutorial concludes with a summary and an invitation to subscribe for more educational videos.

Mindmap
Keywords
💡Binomial Expansion
Binomial expansion refers to the process of expanding expressions that are raised to a power, typically in the form of (a + b)^n. In the video, the presenter discusses how to use the general formula for binomial expansion to find specific terms and coefficients within these expanded expressions.
💡Coefficient
A coefficient is a numerical factor that multiplies a term in an algebraic expression. In the video, the presenter focuses on finding the coefficients of specific terms in the binomial expansion, such as the coefficient of ab^3 in the expansion of (a + b)^4.
💡Combination
Combination, denoted as nCr or 'n choose r', represents the number of ways to choose r elements from a set of n elements without regard to order. It is used in the binomial theorem to determine the coefficients of the expanded terms. The presenter frequently refers to this when solving problems, such as finding the combination 4C3 in the video.
💡General Formula
The general formula for binomial expansion is ∑(r=0 to n) (nCr) * a^(n-r) * b^r, where n is the power of the binomial, and r is the specific term. The video explains how to apply this formula to find particular coefficients in binomial expansions.
💡Factorial
Factorial, denoted by n!, is the product of all positive integers up to n. It is used in calculating combinations. For example, 4! = 4 * 3 * 2 * 1. The presenter uses factorials to compute combinations needed for solving the problems in the video.
💡Exponent
An exponent indicates how many times a number (the base) is multiplied by itself. In the video, exponents are crucial as they define the powers in the terms of the binomial expansion, such as in a^3 or b^2.
💡Negative Coefficient
A negative coefficient arises when the terms in the binomial have negative values. The video explains how to handle these cases, as seen in the problem involving the expansion of (2y - 3)^5, where the coefficient of y^3 is calculated considering the negative sign.
💡Sum of Powers
In a binomial expansion, the sum of the exponents in each term equals the power of the binomial. The presenter emphasizes this point by showing that in each term of (a + b)^4, the sum of the exponents of a and b equals 4.
💡Simplification
Simplification involves reducing expressions to their simplest form. The video demonstrates this through various steps, such as cancelling out terms and reducing factorial expressions, to find the final coefficients of the terms.
💡Expansion Term
An expansion term is a specific term in the expanded form of a binomial expression. The video discusses how to identify and calculate the coefficients of specific expansion terms, such as ab^3 in (a + b)^4 and y^3 in (2y - 3)^5.
Highlights

Introduction to finding the coefficient of a term in binomial expansions using the general formula.

Explanation of the general formula: n choose r, a^(n-r), b^r.

First problem: Finding the coefficient of a*b^3 in (a+b)^4.

Calculation of the coefficient: 4 choose 3, simplified to 4.

Second problem: Finding the coefficient of y^3 in (2y-3)^5.

Determination of r as 2 for y^3 in the expansion.

Calculation of the coefficient: 5 choose 2, multiplied by 2^3 and (-3)^2.

Simplification of the coefficient to 720.

Third problem: Finding the coefficient of x^2*y^4 in (3x+2y)^6.

Determination of r as 4 for y^4 in the expansion.

Calculation of the coefficient: 6 choose 4, multiplied by 3^2 and 2^4.

Simplification of the coefficient to 2160.

Emphasis on the sum of the powers always being equal to n.

Illustration of how the coefficients are derived from the general formula.

Encouragement to share the learning with colleagues.

Invitation to subscribe to the YouTube channel for more educational content.

Transcripts

Browse More Related Video

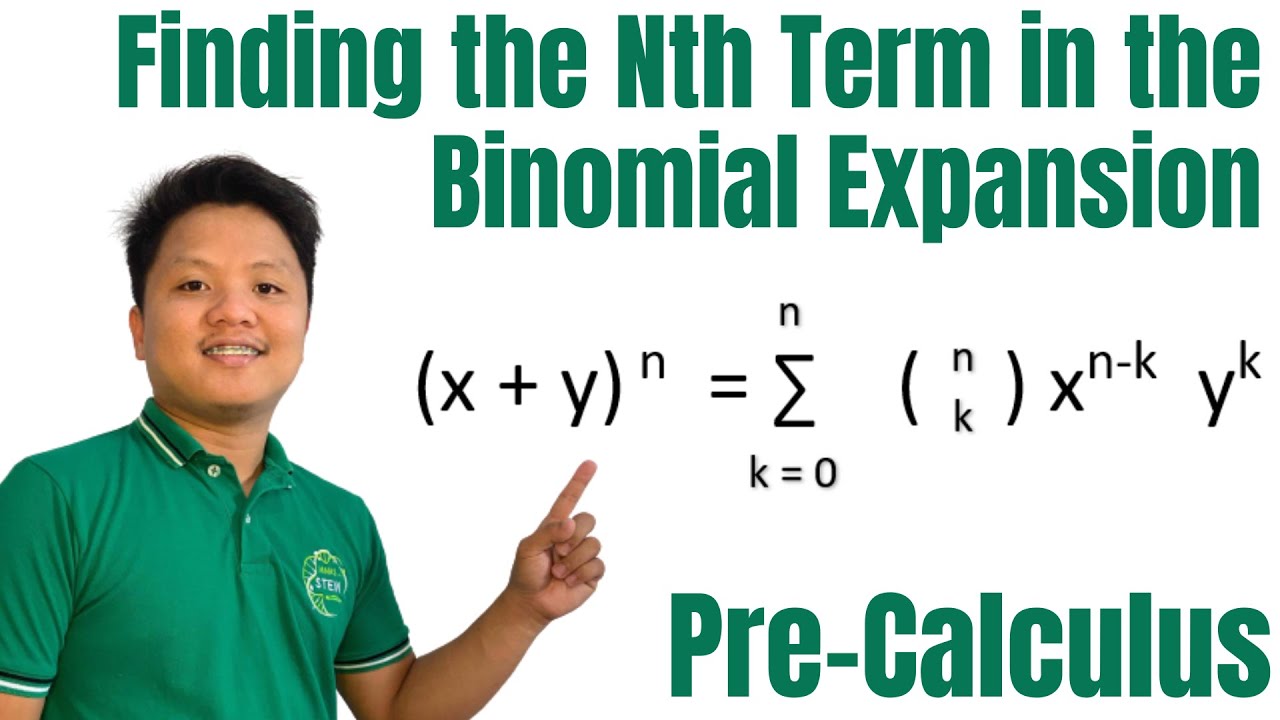
Finding the Nth Term in the Binomial Expansion | Binomial Theorem | Pre-Calculus

Binomial distribution | Probability and Statistics | Khan Academy

Finding The Probability of a Binomial Distribution Plus Mean & Standard Deviation

BINOMIAL THEOREM || PRE-CALCULUS

FINDING THE NTH TERM IN THE BINOMIAL EXPANSION || PRE-CALCULUS

Solving Quadratics by Completing the Square

Rate This

5.0 / 5 (0 votes)

Thanks for rating:

Related Tags
Math TutorialBinomial ExpansionCoefficientsAlgebraEducationLearningFormulasStep-by-StepExamplesOnline Class