What Is And How To Calculate Cohen's d?
TLDRThe video script introduces Cohen's D, a statistical measure used to quantify the magnitude of the difference between two group means. Unlike p-values, Cohen's D provides insight into the size of the effect, not just its existence. The script explains two main formulas for calculating Cohen's D: the original formula for equal group sizes and Cohen's Ds for unequal group sizes. An example calculation is provided, demonstrating how to determine the pooled standard deviation and subsequently the Cohen's D value. The video also discusses how to interpret Cohen's D values, categorizing them into small, medium, and large effect sizes based on general guidelines provided by Cohen. It emphasizes that these interpretations are not rigid and gives examples to illustrate the practical significance of different effect sizes.
Takeaways
- 📊 Cohen's D is a measure of effect size that quantifies the magnitude of the difference between two means.
- 🔍 Unlike p-values, Cohen's D provides information on the size of the effect, not just its existence.
- 📈 Cohen's D is also known as the standardized mean difference, allowing for comparison between different variables and experiments.
- ⚖️ There are two main formulas for Cohen's D: the original and Cohen's Ds, which is used for unequal group sizes.
- 🧮 The pooled standard deviation is used in the calculation because the population standard deviation is unknown.
- 📐 For equal group sizes, the original Cohen's D formula is used, which involves the mean difference divided by the pooled standard deviation.
- 📉 For unequal group sizes, Cohen's Ds formula adjusts the calculation of the pooled standard deviation to account for group size differences.
- 🌟 An example provided calculates Cohen's D for the difference in a certain protein's concentration between males and females.
- 🔢 Cohen's D values are interpreted as the number of standard deviations between the two group means.
- 📝 Cohen categorized effect sizes into small (0.2-0.5), medium (0.5-0.8), and large (>0.8), with these being general interpretations.
- 👀 Small effect sizes are typically too small to be differentiated by the naked eye, while large effect sizes are very obvious differences between groups.
Q & A
What is Cohen's D?
-Cohen's D is a type of effect size that provides a quantitative measure of the magnitude of the difference between two means. It is used to determine the size of the effect in a study, which is different from the p-value that only informs if an effect exists.
How does Cohen's D differ from a p-value?
-While a p-value can tell you if there is a statistically significant effect, it does not reveal the size of that effect. Cohen's D, on the other hand, quantifies the magnitude of the difference between two means, allowing for a better understanding of the effect size.
Why is the pooled standard deviation used in Cohen's D calculation?
-The pooled standard deviation is used because the population standard deviation is typically unknown. It provides an estimate by combining the sample standard deviations from both groups in the study.
What are the two main formulas for calculating Cohen's D?
-The two main formulas for calculating Cohen's D are the original Cohen's D and Cohen's Ds. The original formula is used when the two groups are of equal size, while Cohen's Ds is a variant used when the group sizes are unequal.
How is the pooled standard deviation calculated for the original Cohen's D formula?
-For the original Cohen's D formula, the pooled standard deviation is calculated as the square root of the average of the squared standard deviations of the two groups: sqrt((SD1^2 + SD2^2) / 2).
What is the formula for Cohen's D when groups are of unequal size?
-For unequal group sizes, Cohen's Ds is used. The pooled standard deviation is calculated differently, as the square root of the sum of the squared standard deviations, each multiplied by their respective group size minus one, divided by the total group size minus the sum of the group sizes plus one.
How do you interpret a Cohen's D value of 1.48?
-A Cohen's D value of 1.48 indicates that the means of the two groups differ by 1.48 standard deviations. This is considered a large effect size, which is quite noticeable and significant.
What are the general interpretations of Cohen's D values according to Cohen?
-According to Cohen, small effect sizes are between 0.2 and 0.5, medium effect sizes are between 0.5 and 0.8, and large effect sizes are anything over 0.8.
Why are Cohen's D values considered useful in research?
-Cohen's D values are useful because they standardize the difference between two means, allowing for comparison across different variables and experiments. This helps researchers understand the practical significance of their findings.
How can the interpretation of Cohen's D be applied in real-world scenarios?
-Cohen's D can be applied to understand the magnitude of differences in various real-world scenarios, such as the difference in average heights between age groups or the difference in test scores between different educational interventions.
What is the significance of comparing Cohen's D values across different studies?
-Comparing Cohen's D values across different studies allows researchers to understand the relative size of effects found in various experiments. This can help in determining the impact of different treatments, interventions, or phenomena being studied.
How might the interpretation of Cohen's D values be limited?
-The interpretation of Cohen's D values is limited by the fact that they are general guidelines and should not be used rigidly. The context of the study and the specific field of research may require different thresholds for what constitutes a small, medium, or large effect size.
Outlines
📊 Understanding Cohen's D as an Effect Size Measure
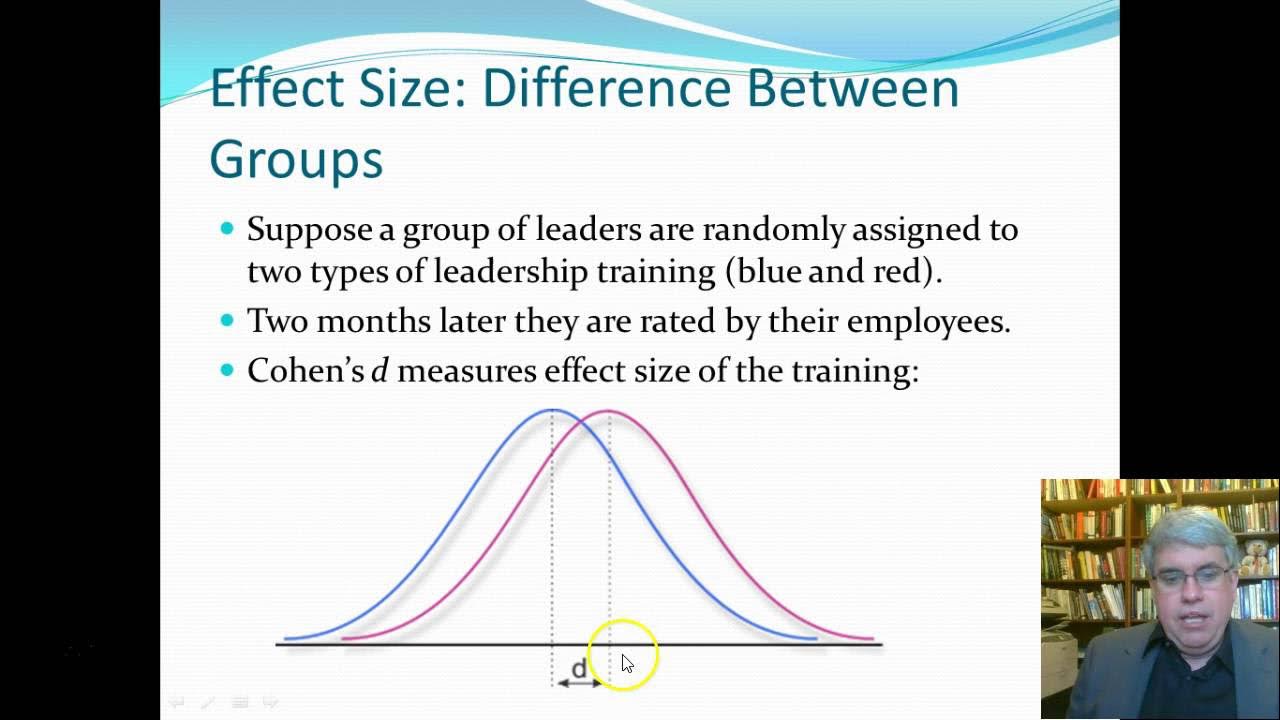
This paragraph introduces Cohen's D as a standardized measure of effect size between two means. It distinguishes Cohen's D from a p-value, emphasizing that while p-values indicate the existence of an effect, Cohen's D quantifies the magnitude of that effect. The paragraph explains the concept of a pooled standard deviation, which is used when the population standard deviation is unknown. It also outlines two main formulas for calculating Cohen's D: one for equal group sizes and another, Cohen's Ds, for unequal group sizes. An example calculation is provided for both scenarios using hypothetical data on a certain protein measured in males and females.
🔍 Interpreting Cohen's D Values and Their Significance
The second paragraph delves into the interpretation of Cohen's D values, describing them as the number of standard deviations that separate the means of two groups. It provides a contextual understanding by comparing the Cohen's D value of 1.489 to the actual difference in protein concentrations between males and females. The paragraph also outlines Cohen's original interpretation of effect sizes into small, medium, and large categories, with specific D value ranges for each. It further explains the practical implications of these effect sizes using relatable examples, such as the difference in height between different age groups of girls. The summary concludes with a reminder of the importance of Cohen's D in research and how it can be categorized into the three groups of effect sizes.
Mindmap
Keywords
💡Cohen's D
💡Effect Size
💡P-value
💡Pooled Standard Deviation
💡Standard Deviation
💡Sample Population
💡Group Sizes
💡Cohen's Ds
💡Interpretation of Cohen's D
💡Statistical Significance
Highlights
Cohen's D is a type of effect size used to measure the magnitude of the difference between two means.
Effect size differs from the p-value, as it reveals the size of the effect, not just its existence.
Cohen's D values, also known as the standardized mean difference, allow comparison between different variables and experiments.
There are two main formulas for Cohen's D: the original and Cohen's Ds, which is used for unequal group sizes.
The pooled standard deviation is used in the formulas as an estimate for the population standard deviation.
The original Cohen's D formula is used when two groups are of equal size.
Cohen's Ds formula accounts for group size differences in the calculation of the pooled standard deviation.
An example calculation is provided for Cohen's D between two groups: males and females.
The pooled standard deviation is calculated differently for equal and unequal group sizes.
Cohen's D value represents the number of standard deviations between the two group means.
Cohen's D values can be interpreted as small, medium, or large effect sizes.
Small effect sizes are generally too small to be differentiated by the naked eye.
Medium effect sizes are just large enough to be seen by the naked eye.
Large effect sizes represent very obvious differences between groups.
Cohen provided general interpretations for small, medium, and large effect sizes, but they should not be used rigidly.
The example given compares the difference in height between different age groups of girls to illustrate effect sizes.
Cohen's D is a valuable tool for researchers to quantify and interpret the magnitude of differences between groups.
Understanding Cohen's D and its calculation helps in the practical application of statistical analysis in research.
Transcripts
Browse More Related Video
5.0 / 5 (0 votes)
Thanks for rating: