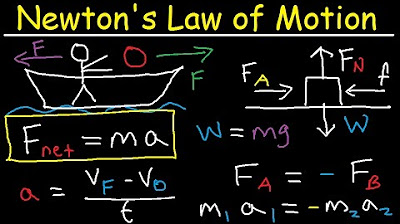
More on Newton's third law | Forces and Newton's laws of motion | Physics | Khan Academy
TLDRThe video script delves into the nuances of Newton's Third Law, often misconceived as 'for every action, there's an equal and opposite reaction.' It clarifies that the law pertains to forces being equal in magnitude and opposite in direction, acting on different objects. The misconception that forces cancel each other out is debunked by explaining that while forces are equal, accelerations differ due to mass. The script also dispels the idea of a delay in the creation of partner forces, emphasizing their instantaneous nature. It uses the example of a box on a table to illustrate how to identify third law partner forces by reversing the labels of interacting objects. The distinction between third law forces and other equal and opposite forces, like those balancing an object in rest, is also highlighted. The summary serves to correct common misunderstandings and provides a clear understanding of Newton's Third Law and its implications.
Takeaways
- 📚 Newton's Third Law is often misinterpreted as 'for every action, there's an equal and opposite reaction', but it's more accurately described as 'for every force, there's an equal and opposite force'.
- 🔍 The 'equal' in the law means the forces are the same in magnitude, and 'opposite' means they have opposite directions, emphasizing the vector nature of forces.
- 🤔 A common misconception is that these forces might cancel each other out, but they don't because they act on different objects.
- 📌 To clarify, if object A exerts force F on object B, then object B must exert an equal and opposite force (-F) on object A.
- 🚫 Forces described by Newton's Third Law cannot cancel out because they are applied to different objects, which is a critical distinction.
- 🌌 Even if two objects are vastly different in size, the forces they exert on each other must be equal in magnitude, as per Newton's Third Law.
- 🔄 The law applies universally and instantaneously, with no delay in the creation of the partner force, regardless of the speed at which the forces are applied.
- ⚖️ While forces may be equal, the resulting accelerations can differ due to the mass of the objects involved, as acceleration is force divided by mass.
- 🕰 Newton's Third Law holds true at every moment in time, ensuring that action and reaction forces are always equal and opposite, no matter the scenario.
- 🔍 To identify third law partner forces, list both objects involved and reverse the labels to find the corresponding force on the second object.
- ❌ Not all equal and opposite forces are third law partner forces; they must be identified correctly by considering the objects they act upon.
Q & A
What is the common misconception about Newton's Third Law that is often cited?
-The common misconception is that for every action, there is an equal and opposite reaction. This is too vague to be useful and doesn't accurately represent the law's specifics regarding forces and objects.
How can Newton's Third Law be more precisely phrased?
-More precisely, Newton's Third Law states that for every force, there is an equal and opposite force. This emphasizes that the forces are equal in magnitude and opposite in direction.
Why doesn't the world's forces cancel each other out according to Newton's Third Law?
-The forces do not cancel each other out because they are exerted on different objects. The action and reaction forces are on different objects, which is a critical aspect of Newton's Third Law.
If two forces are equal and opposite, why does one object accelerate while the other remains still?
-Even if the forces are equal and opposite, the resulting accelerations can differ because acceleration is the net force divided by the mass. A more massive object will experience less acceleration than a less massive one when the same force is applied.
How does Newton's Third Law apply to the forces between a planet and a star?
-According to Newton's Third Law, the force that a planet exerts on a star is equal in magnitude and opposite in direction to the force that the star exerts on the planet, regardless of their relative masses.
Is there a delay in the creation of the reaction force in Newton's Third Law?
-No, there is no delay. The reaction force is instantaneously generated as soon as the action force is applied, according to Newton's Third Law.
How can one identify the partner force in Newton's Third Law?
-To identify the partner force, list both objects involved in the interaction and then reverse the labels of the force to find the partner force. The partner force is always on the second object and in the opposite direction.
Why are the forces exerted by a table on a box and vice versa not considered third law partner forces?
-The forces exerted by a table on a box and by the box on the table are not third law partner forces because they can cancel each other out when there is no acceleration. Third law partner forces are always on different objects and cannot cancel out.
What is the difference between forces that are equal and opposite due to the second law and third law partner forces?
-Forces that are equal and opposite due to the second law occur when the net force on an object is zero, resulting in no acceleration. In contrast, third law partner forces are always equal and opposite, regardless of the acceleration, and they act on different objects.
Can the magnitude of the forces in Newton's Third Law be unequal if the objects have different properties?
-No, the magnitude of the forces in Newton's Third Law must be equal, regardless of the objects' properties such as size or charge. The law dictates that the forces are equal in magnitude and opposite in direction.
What is the role of mass in determining the acceleration of an object when forces are applied?
-The mass of an object determines its acceleration when a force is applied. A larger mass will result in a smaller acceleration for the same applied force, as acceleration is inversely proportional to mass when the force is constant.
Outlines
🧐 Clarifying Newton's Third Law: Action and Reaction
The paragraph discusses common misconceptions about Newton's Third Law, emphasizing that it is not as straightforward as often believed. The law is frequently summarized as 'for every action, there's an equal and opposite reaction,' but this is deemed too vague. A more precise formulation is 'for every force, there's an equal and opposite force.' This means the forces are equal in magnitude but opposite in direction. The paragraph clarifies that these forces act on different objects, hence they do not cancel each other out. It also dispels the notion that forces might cancel out all motion in the universe, illustrating that forces are exerted on different objects, such as object A by object B and vice versa. The summary stresses the importance of understanding that Newton's Third Law involves pairs of forces that are always on different objects, which is critical for grasping the law's implications accurately.
🚀 The Consequences of Equal and Opposite Forces
This paragraph explains why equal and opposite forces, as described by Newton's Third Law, do not result in equal outcomes. It clarifies that even though forces are equal, the resulting accelerations may differ due to the masses of the objects involved. Acceleration is defined as the net force divided by mass, hence different masses can lead to different accelerations, even when the forces are the same. The paragraph also debunks the myth that there might be a delay in the creation of the reaction force; according to Newton's Third Law, the forces are always equal and opposite at every moment in time, regardless of the speed at which they are applied. The instantaneous nature of these forces is highlighted, using the example of a person kicking a wall to illustrate that the reaction force from the wall occurs at the same time as the action force applied by the foot. The summary also addresses the challenge of identifying third law partner forces, suggesting listing both interacting objects and reversing the labels as a method to determine the partner force accurately.
🌌 The Universality and Identification of Newton's Third Law Forces
The final paragraph underscores the universality of Newton's Third Law, which applies to all situations regardless of the objects' sizes, charges, or other properties. It reiterates that the forces involved in Newton's Third Law are always equal in magnitude and opposite in direction, acting on two different objects. The process of identifying the third law partner force is simplified by reversing the labels of the interacting objects. The paragraph also differentiates between forces that might be equal and opposite but are not third law partner forces, such as when the net force is zero due to the second law of motion, indicating no acceleration. Using the example of a box on a table, the paragraph shows how the force exerted by the table on the box and the force exerted by the box on the table are third law partner forces, which remain equal and opposite regardless of the situation. However, the force of the table on the box does not have to be the partner force to the gravitational force exerted by the Earth on the box; instead, the partner force to gravity is the force the box exerts on the Earth. The summary reminds us that while some forces may appear to be third law partner forces, they are only so if they act on different objects and are equal and opposite in direction and magnitude at every given moment.
Mindmap
Keywords
💡Newton's Third Law
💡Action and Reaction
💡Force Vectors
💡Magnitude
💡Direction
💡Acceleration
💡Mass
💡Instantaneous
💡Misconceptions
💡Universality
💡Identifying Forces
Highlights
Newton's Third Law states that for every force, there is an equal and opposite force
The forces are equal in magnitude and opposite in direction
The forces act on different objects, not the same object
Forces are vectors, so they have both magnitude and direction
Common misconception is thinking forces cancel out, but they act on different objects
Forces are always equal and opposite at every moment in time
Forces are instantaneously generated, there is no delay
Equal forces on different objects can result in different accelerations due to different masses
Forces are always equal between two interacting objects, regardless of their sizes or properties
Identifying third law partner forces involves listing both objects and reversing the labels
Forces that are equal and opposite but act on the same object are not third law partner forces
Third law partner forces never cancel out because they act on different objects
The forces between the Earth and an object, like a planet, are always equal and opposite
The reason a more massive object doesn't move as much is due to its larger mass resulting in lower acceleration
An example of identifying third law partner forces is the force between a box and the Earth
The force the table exerts on a box and the force the box exerts on the table are third law partner forces
Forces that seem equal and opposite may not be third law partner forces if they act on the same object
Newton's Third Law is a fundamental principle that applies universally to all forces and objects
Transcripts
5.0 / 5 (0 votes)
Thanks for rating: