Moment of Inertia Introduction and Rotational Kinetic Energy Derivation

Flipping Physics
2 Sept 201808:39
EducationalLearning
32 Likes 10 Comments

TLDRThe video script explores the concept of kinetic energy and its two forms: translational and rotational. It begins with a discussion on the equation for kinetic energy, which is one-half mass times velocity squared. The conversation then shifts to rotational kinetic energy, emphasizing that it's not the center of mass's velocity that matters but the energy of each small piece within a rotating object. The script introduces the concept of moment of inertia, which is the sum of mass times the square of the distance from the axis of rotation for each piece of the object, and is likened to 'rotational mass.' The video uses an analogy of eggs in an egg carton to illustrate how the moment of inertia affects the ease or difficulty of causing angular acceleration. It concludes by highlighting the difference between moment of inertia and inertial mass, and how the former is more influenced by the distance from the axis of rotation rather than the mass itself.

Takeaways
  • 📚 The equation for kinetic energy is one-half mass times velocity squared (KE = 1/2 mv^2).
  • 🚴 For a rotating object like a bike wheel, the center of mass may not move, but the object still has kinetic energy due to its rotation.
  • 🔄 Kinetic energy of a rotating object is not calculated using the center of mass velocity, but rather the sum of kinetic energies of all small parts that make up the object.
  • ⚙️ Tangential velocity (v) is related to angular velocity (ω) by the equation v = rω, where r is the radius or distance from the axis of rotation.
  • 📐 The Moment of Inertia (I) is a measure of an object's resistance to angular acceleration, calculated as the sum of mass times the square of the distance from the axis of rotation for each small piece of the object.
  • ↕️ Moment of Inertia is also known as 'rotational mass' and is symbolized by a capital 'I'.
  • 🔄 The rotational kinetic energy of an object is given by one half times the moment of inertia times the square of the angular velocity (KE_rot = 1/2 Iω^2).
  • 🏃 Translational Kinetic Energy is the energy associated with the motion of the center of mass of an object as it moves through space.
  • 🤔 The concepts of inertial mass (resistance to linear acceleration) and moment of inertia (resistance to angular acceleration) are distinct, though related.
  • 🥚 By moving the mass further from the axis of rotation, the moment of inertia—and thus the resistance to angular acceleration—increases, even if the total mass remains the same.
  • 📈 The distance from the axis of rotation (r) has a greater impact on the moment of inertia than the mass of the individual pieces because it is squared in the calculation.
  • 📉 Tripling the distance from the axis of rotation increases the moment of inertia nine times, whereas tripling the mass only triples it.
Q & A

  • What is the basic equation for kinetic energy?

    -The basic equation for kinetic energy is Kinetic energy equals one-half mass times velocity squared (KE = 1/2 mv^2).

  • Why does the statement that a rotating wheel with a stationary center of mass has zero kinetic energy not make sense?

    -It doesn't make sense because kinetic energy is the energy of motion, and even though the center of mass isn't moving, the wheel is still in motion due to rotation, so it should have kinetic energy.

  • How should we calculate the kinetic energy of a rotating object like a wheel?

    -Instead of using the center of mass, we should consider the kinetic energy of all the individual small pieces that make up the wheel. The total kinetic energy is the sum of the kinetic energies of all these small pieces.

  • What is the relationship between tangential velocity and angular velocity?

    -The relationship between tangential velocity (v) and angular velocity (ω) is given by the equation v = rω, where r is the radius or the distance from the axis of rotation.

  • What is the term used to describe the sum of the mass of each small piece times the square of its distance from the axis of rotation?

    -The term used is Moment of Inertia (I), which is a measure of an object's resistance to rotational acceleration.

  • How is the rotational kinetic energy of an object defined mathematically?

    -The rotational kinetic energy (KE_rot) is defined as one half times the moment of inertia (I) of the object, times the square of the angular velocity (ω) of the object (KE_rot = 1/2 Iω^2).

  • What is the difference between translational kinetic energy and rotational kinetic energy?

    -Translational kinetic energy is the energy associated with the motion of the center of mass of an object moving from one point in space to another, while rotational kinetic energy is the energy associated with an object rotating around its axis.

  • Why is the moment of inertia also referred to as 'rotational mass'?

    -The moment of inertia is referred to as 'rotational mass' because it takes the place of the inertial mass in the rotational kinetic energy equation, representing the measure of an object's resistance to angular acceleration.

  • What is the role of inertial mass in the context of the kinetic energy equations?

    -Inertial mass is a measure of an object's resistance to acceleration. In the context of kinetic energy equations, it is a factor in the translational kinetic energy equation, representing the object's resistance to linear acceleration.

  • How does the location of mass within a system affect its moment of inertia?

    -The location of mass within a system greatly affects its moment of inertia. The further the mass is from the axis of rotation, the larger the moment of inertia, and the more difficult it is to cause the system to angularly accelerate.

  • Why does the distance from the axis of rotation (r) in the moment of inertia equation have a larger influence than the mass of each particle?

    -The distance from the axis of rotation (r) is squared in the moment of inertia equation, which means that it has a much larger influence on the moment of inertia than the mass of each particle. Doubling the distance from the axis of rotation quadruples the moment of inertia, whereas doubling the mass only doubles it.

  • What is the significance of the moment of inertia in understanding the behavior of rotating objects?

    -The moment of inertia is significant because it quantifies the resistance of a rotating object to changes in its state of rotation. A higher moment of inertia means that more torque is required to change the angular velocity of the object, making it more stable and resistant to angular acceleration.

Outlines
00:00
🧐 Understanding Kinetic Energy and Rotational Motion

The first paragraph introduces the concept of kinetic energy and its equation, which is one-half mass times velocity squared. It then discusses a scenario involving a rotating bike wheel, highlighting that despite the wheel's motion, its center of mass remains stationary, leading to a seemingly zero kinetic energy. The conversation emphasizes that the kinetic energy equation does not apply to this situation as it does not account for the type of kinetic energy the wheel possesses. The speakers delve into the concept of rotational kinetic energy, explaining that it involves considering the kinetic energy of all the individual small pieces that make up the wheel. They introduce the equation for rotational kinetic energy, which involves the moment of inertia and the square of the angular velocity. The moment of inertia is defined as the sum of the mass of each small piece times the square of its distance from the axis of rotation. The paragraph concludes with a comparison between translational and rotational kinetic energy, and an explanation of the moment of inertia as 'rotational mass'.

05:05
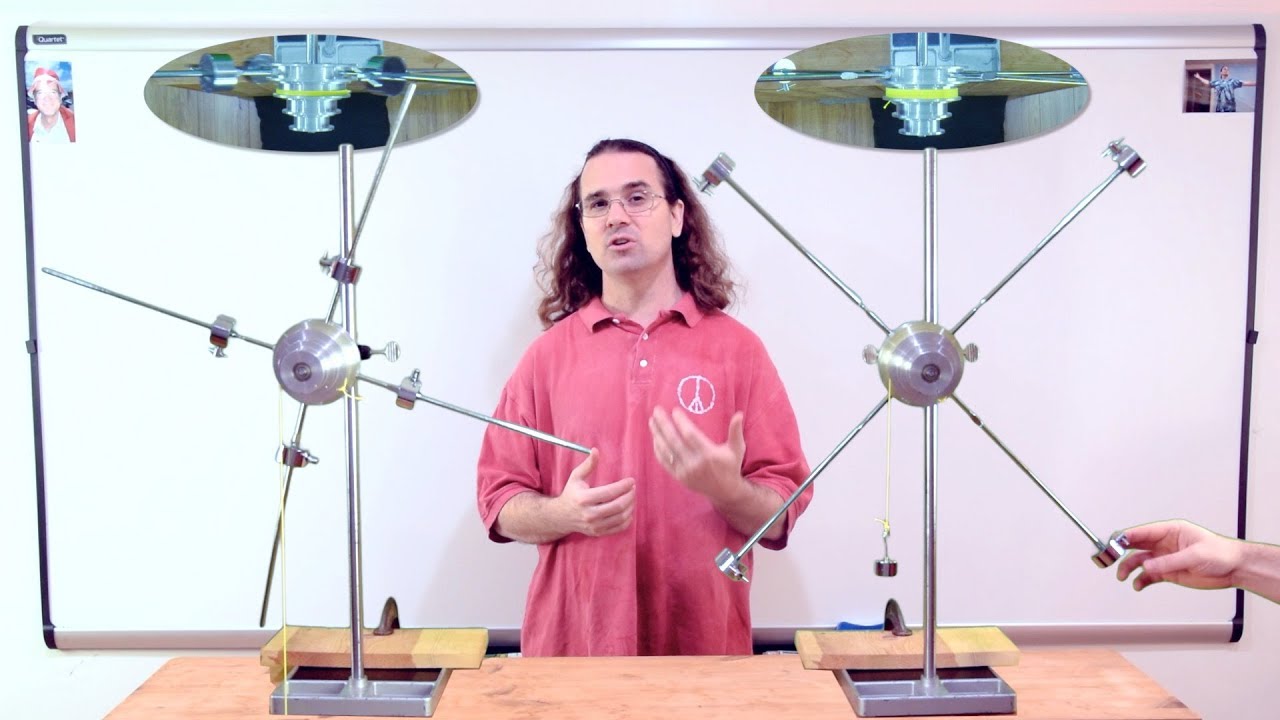
🥚 Demonstrating Moment of Inertia with an Egg Carton Example

The second paragraph uses an egg carton with two eggs to illustrate the concept of moment of inertia, or 'rotational mass'. It explains that the moment of inertia is a measure of an object's resistance to angular acceleration, and it increases with the square of the distance from the axis of rotation. By moving the eggs from the center to the ends of the egg carton, the distance from the axis of rotation ('r') increases, which in turn increases the moment of inertia. This makes it more difficult to rotate the eggs, even though the total mass of the system remains the same. The paragraph emphasizes the importance of the distance from the axis of rotation in determining the moment of inertia, as it is squared in the equation. The example demonstrates that increasing the distance has a more significant effect on moment of inertia than increasing the mass of the particles involved.

Mindmap
Keywords
💡Kinetic Energy
Kinetic energy is the energy that an object possesses due to its motion. It is defined as one-half the product of the object's mass and the square of its velocity (KE = 1/2 mv^2). In the video, the concept is introduced to discuss the energy of motion, both in a translating object and a rotating wheel, leading to the distinction between translational and rotational kinetic energy.
💡Center of Mass
The center of mass is the point at which the mass of an object can be considered to be concentrated for the purpose of analyzing its motion. In the script, it's mentioned that for a rotating bike wheel, the center of mass is not moving, which initially leads to a misconception about the wheel's kinetic energy.
💡Translational Kinetic Energy
This is the kinetic energy associated with the motion of the center of mass of an object as it moves from one point in space to another. It is distinct from rotational kinetic energy and is calculated using the formula KE = 1/2 mv^2, where m is the mass and v is the linear velocity of the object's center of mass. The video clarifies this concept by contrasting it with rotational kinetic energy.
💡Rotational Kinetic Energy
This is the kinetic energy possessed by an object due to its rotation. It is calculated differently from translational kinetic energy and is given by the formula KE = 1/2 Iω^2, where I is the moment of inertia and ω is the angular velocity. The video explains this concept by examining the kinetic energy of a rotating wheel, which is not simply zero even when its center of mass is stationary.
💡Moment of Inertia
The moment of inertia (I) is a measure of an object's resistance to rotational acceleration about a particular axis and is calculated as the sum of the product of each small mass element and the square of its distance from the axis of rotation. It is a key concept in the video, where it is used to calculate the rotational kinetic energy of a wheel and is likened to 'rotational mass'.
💡Angular Velocity
Angular velocity (ω) is a measure of the rate of rotation of an object, expressed in radians per unit time. It is a vector quantity that defines the angular speed and direction of rotation. In the script, it is used to relate the tangential velocity of points on a rotating wheel to its rotation speed.
💡Tangential Velocity
Tangential velocity is the linear velocity of a point moving along the circumference of a circle, and it is determined by the radius of the circle and its angular velocity (v = rω). The video uses this concept to connect the rotational motion of a wheel to the linear motion of points on its edge.
💡Inertial Mass
Inertial mass is a measure of an object's resistance to acceleration when a force is applied. It is a fundamental concept in physics that describes why more massive objects are harder to move or stop once in motion. The video uses this concept to draw a parallel with moment of inertia, which is a measure of resistance to rotational acceleration.
💡Rigid Body
A rigid body is an idealized object in which the distance between all pairs of particles remains constant, meaning it does not change shape when rotated or when forces are applied. The video discusses the wheel as a rigid body, which allows for the assumption that all parts of the wheel share the same angular velocity.
💡Egg Carton Example
The egg carton example is used in the video to illustrate the concept of moment of inertia. By moving the eggs within the carton, the distance of the mass from the axis of rotation changes, which in turn affects the moment of inertia and the ease with which the system can be rotated. This practical example helps to solidify the abstract concept of moment of inertia.
💡Distance from Axis of Rotation
The distance (r) of a mass from the axis of rotation is squared in the moment of inertia equation, which means it has a significant impact on the object's resistance to rotational acceleration. The video emphasizes that this distance is more influential in determining the moment of inertia than the mass itself, as increasing the distance has a squared effect on the moment of inertia.
Highlights

Kinetic energy is defined as one-half mass times velocity squared.

A rotating object like a bike wheel has kinetic energy even if its center of mass is not moving.

The total kinetic energy of a rotating wheel is the sum of the kinetic energies of all its small pieces.

Each small piece of the wheel has a tangential velocity due to the rotation.

Tangential velocity is related to angular velocity by the equation: Tangential velocity equals radius times angular velocity.

The moment of inertia (I) is the sum of mass times the square of the distance from the axis of rotation for each small piece of the object.

Rotational kinetic energy is calculated as one half times the moment of inertia times the square of the angular velocity.

Translational kinetic energy is the energy associated with the motion of the center of mass of an object.

The moment of inertia can also be thought of as 'rotational mass', indicating the object's resistance to angular acceleration.

Inertial mass is a measure of an object's resistance to acceleration.

The moment of inertia is influenced more by the distance from the axis of rotation squared than by the mass of each particle.

Moving the mass further from the axis of rotation increases the moment of inertia, making it harder to cause angular acceleration.

The egg carton example demonstrates how changing the location of masses affects the moment of inertia and rotational acceleration.

The inertial mass of a system remains constant even when the location of its masses changes.

The distance from the axis of rotation (r) is squared in the moment of inertia equation, emphasizing its significant impact on the object's rotational properties.

Tripling the distance from the axis of rotation increases the moment of inertia nine times, whereas tripling the mass only triples it.

The discussion provides a clear understanding of the concepts of kinetic energy, moment of inertia, and their practical implications.

Transcripts

Browse More Related Video

Moment of Inertia | Physics with Professor Matt Anderson | M12-05

Pure Rolling Motion | Physics with Professor Matt Anderson | M12-12

AP Physics 1 - Rotational Kinetic Energy

College Physics 1: Lecture 25 - Moment of Inertia and Rotational Dynamics

AP Physics Lecture on Moment of Inertia. Watch this before 7.E

Demonstrating Rotational Inertia (or Moment of Inertia)

Rate This

5.0 / 5 (0 votes)

Thanks for rating:

Related Tags
Kinetic EnergyMoment of InertiaPhysicsRotational MotionEducationalAngular VelocityTangential VelocityRigid BodyEgg Carton AnalogyInertia